Schrodinger Equation - A simple derivation

DrPhysicsA
19 Jan 201207:35
EducationalLearning
32 Likes 10 Comments

TLDRThe script presents a simplified derivation of the Schrödinger equation, starting with the total energy concept and using a wave function in the form of ψ = e^(i(kx - ωt)). Through differentiation and substitution, the time-independent Schrödinger equation is derived, followed by the time-dependent version. The explanation connects wave mechanics with quantum mechanics, highlighting the significance of Planck's constant and the wave-particle duality.

Takeaways
  • 📝 The Schrodinger equation is derived from the principle that total energy is the sum of kinetic and potential energy.
  • 🌊 The wave function used in the derivation is of the form ψ = e^(i(kx - ωt)), representing a wave with wave number k and angular frequency ω.
  • 🔄 Differentiating the wave function with respect to x yields an expression involving i, k, and the original wave function ψ.
  • 📐 When differentiating the wave function with respect to x again, the term ∂²ψ/∂x² emerges, which is key in the equation.
  • 🌐 The relationship K = P/ħ (h-bar) is used, where K is the wave number, P is momentum, and ħ is the reduced Planck's constant.
  • 🔄 Substituting the expression for K into the second derivative term leads to the equation involving the momentum operator P squared and the Planck's constant.
  • 💡 The time-independent Schrodinger equation is derived by equating the energy eigenvalue (E) to the sum of P²/2m and the potential energy (U).
  • 🕒 The time-dependent Schrodinger equation is introduced by considering the time derivative of the wave function and its relationship with energy and frequency.
  • 🔄 The frequency ω is related to the energy eigenvalue E by the equation E = ħω, which is used to further derive the time-dependent equation.
  • 🌐 The final form of the time-dependent Schrodinger equation is obtained by substituting the expressions for the time derivative of the wave function into the equation.
  • 📈 The derivation provided is a simplified explanation of the complex mathematical framework behind quantum mechanics.
Q & A

  • What is the total energy composed of in the context of this script?

    -In the context of this script, the total energy is composed of kinetic energy and potential energy.

  • How is the total energy mathematically represented in the script?

    -The total energy is mathematically represented as half the product of mass (M) and velocity (V) squared plus the potential energy (U), which can be written as E = (1/2)MV^2 + U.

  • What is the significance of the wave function ψ in the derivation?

    -The wave function ψ, represented as ψ = e^(i(KX - ΩT)), is significant as it is the form used to describe the quantum state of a particle, which is essential in deriving the Schrödinger equation.

  • What is the role of the term 'K' in the wave function?

    -The term 'K' in the wave function represents the wave number, which is related to the momentum (P) through the relation K = P/ħ, where ħ is the reduced Planck's constant.

  • How is the time-independent Schrödinger equation derived from the given wave function?

    -The time-independent Schrödinger equation is derived by differentiating the wave function with respect to X, applying the relation between K and P, and equating the resulting expression to the total energy E, which leads to the equation: -ħ²(∂²ψ/∂x²) = P²ψ/(2m) + Uψ.

  • What is the time-dependent Schrödinger equation?

    -The time-dependent Schrödinger equation is a version of the Schrödinger equation that includes time-varying factors, and it is derived by differentiating the wave function with respect to time T, leading to the equation: iħ(∂ψ/∂t) = -ħ²(∂²ψ/∂x²) + Uψ.

  • How is the reduced Planck's constant (ħ) related to the momentum (P) and the wave number (K)?

    -The reduced Planck's constant (ħ) is related to the momentum (P) and the wave number (K) through the relation K = 2π/λ, where λ is the wavelength, and ħ = P/K.

  • What is the significance of the relation E = P²/(2m) + U in the derivation?

    -The relation E = P²/(2m) + U is significant as it represents the total energy of the particle in terms of its momentum and potential energy, which is crucial for equating the differentiated wave function to the total energy in the derivation of the Schrödinger equation.

  • How does the script connect the concept of energy to the wave function?

    -The script connects the concept of energy to the wave function by equating the total energy of the particle to the energy represented by the wave function, which includes both its kinetic and potential energy components.

  • What is the role of Planck's constant in the derivation of the Schrödinger equation?

    -Planck's constant, and specifically the reduced Planck's constant (ħ), plays a fundamental role in the derivation of the Schrödinger equation as it relates the momentum and the wave number, and appears in the differential equations that describe the time-dependent and time-independent behaviors of the wave function.

  • Why is the form of the wave function e^(i(KX - ΩT)) chosen for this derivation?

    -The form of the wave function e^(i(KX - ΩT)) is chosen because it represents a general solution to the wave equation that can describe the quantum state of a particle in terms of its momentum and energy, which is essential for the derivation of the Schrödinger equation.

Outlines
00:00
📚 Derivation of the Time-Independent Schrödinger Equation

This paragraph begins with an introduction to the derivation of the Schrödinger equation. It starts by recalling the concept that total energy is the sum of kinetic and potential energy, expressed as E = (1/2)mv² + U, where m is mass, v is velocity, and U is potential energy. The wave function is introduced as ψ = e^(iKX - ωt), with K being the wave number and ω the angular frequency. By differentiating this wave function with respect to X and using the relations between momentum (P), energy (E), and the reduced Planck constant (ħ), the paragraph derives the time-independent Schrödinger equation: -ħ²/2m * (d²ψ/dx²) + Uψ = Eψ. The explanation is mathematical and assumes prior knowledge of the concepts involved.

05:03
🕒 Extension to the Time-Dependent Schrödinger Equation

The second paragraph extends the discussion to the time-dependent Schrödinger equation. It begins by introducing the concept that E equals ħω, where ω is the angular frequency. The wave function is then differentiated with respect to time (t), leading to the expression for the time evolution of the wave function. By substituting the relationship between energy and frequency, and applying the previously derived time-independent equation, the paragraph arrives at the time-dependent Schrödinger equation: iħ(dψ/dt) = -ħ²/2m * (d²ψ/dx²) + Uψ. This equation describes how the quantum state evolves over time, which is crucial for understanding quantum dynamics.

Mindmap
Keywords
💡Schrodinger Equation
The Schrodinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. In the video, the derivation of the time-independent and time-dependent forms of the equation is explained. It is central to understanding quantum systems and is used to determine the wave function of particles.
💡Total Energy
Total energy, in the context of the script, refers to the sum of kinetic and potential energy of a system. It is a fundamental concept in classical mechanics and is extended to quantum mechanics. The script begins by reminding viewers that total energy is half the mass times the velocity squared plus potential energy, which is a precursor to the quantum mechanical energy operator.
💡Kinetic Energy
Kinetic energy is the energy of motion, defined as half the product of an object's mass and the square of its velocity. In the video, kinetic energy is used to introduce the concept of energy in quantum systems and is a part of the derivation of the Schrodinger equation.
💡Potential Energy
Potential energy is the stored energy of a system due to its position or configuration. In the script, it is part of the total energy equation and is crucial for understanding how energy changes in quantum systems are described by the Schrodinger equation.
💡Wave Function
The wave function, denoted as ψ (psi), is a mathematical description of the quantum state of a system. It contains all the information about a physical system and is central to the interpretation of quantum mechanics. In the video, a specific form of the wave function, e^(i(kx - ωt)), is used to derive the Schrodinger equation.
💡Differentiation
Differentiation is a mathematical operation that finds the rate at which a function changes with respect to its variable. In the video, differentiation with respect to position (x) and time (t) is used to manipulate the wave function and derive the Schrodinger equations.
💡Planck's Constant
Planck's constant, denoted as ħ (h-bar), is a fundamental constant in quantum mechanics that relates the energy of a photon to its frequency. It appears in the script as a factor in the wave function and is crucial in the formulation of the Schrodinger equation.
💡Momentum
Momentum, represented by the symbol 'p', is the product of an object's mass and its velocity. It is a key concept in classical mechanics and has a direct correspondence in quantum mechanics, where it is related to the wave function through the de Broglie hypothesis.
💡De Broglie Wavelength
The de Broglie wavelength, associated with the wave-particle duality of particles, is the wavelength of the wave associated with a moving particle. It is given by the formula λ = h/p, where h is Planck's constant and p is the momentum of the particle. In the video, this concept is used to define the wave number k.
💡Energy Operator
The energy operator is a differential operator used in quantum mechanics to represent the total energy of a system in terms of its wave function. It is derived from the classical energy expression and is a key component in the formulation of the Schrodinger equation.
💡Time-Independent
Time-independent refers to a property of a system or equation where the outcome does not change with time. In the context of the video, the time-independent Schrodinger equation describes the stationary states of a quantum system, which do not depend on time.
💡Time-Dependent
Time-dependent means that a property or system changes with time. In the video, the time-dependent Schrodinger equation accounts for the evolution of the quantum state over time, which is essential for describing dynamic systems.
Highlights

Derivation of the Schrodinger equation using simple concepts.

Total energy is the sum of kinetic and potential energy.

The equation for total energy is E = (1/2)mv^2 + U.

Differentiating the wave function with respect to x.

The wave function is given by ψ = e^(i(kx - ωt)).

Differentiating the wave function with respect to x squared.

The relationship between k and p: k = p/ħ.

The derivation of the time-independent Schrodinger equation.

The time-independent Schrodinger equation is given by -(ħ^2/2m)∂^2ψ/∂x^2 + Uψ = Eψ.

Derivation of the time-dependent Schrodinger equation.

The time-dependent Schrodinger equation is given by iħ(∂ψ/∂t) = (-ħ^2/2m)∂^2ψ/∂x^2 + Uψ.

The relationship between E, p, and ω: E = p^2/2m + U and E = ħω.

The wave function's form is crucial for the derivation process.

Understanding the role of Planck's constant in the derivation.

The use of complex exponential functions in the wave function.

The significance of the de Broglie wavelength λ in defining k.

The derivation process involves both spatial and temporal differentiation.

The connection between the energy of the system and the wave function.

The final form of the time-dependent Schrodinger equation.

Transcripts

Browse More Related Video

How Schrodinger Came Up With His Famous Equation (But EASIER)

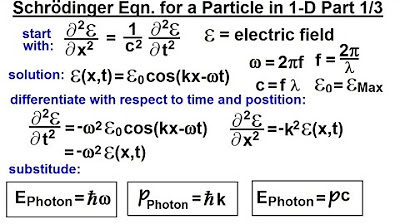
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (5 of 92) The Schrodinger Eqn. in 1-D (1/3)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (14 of 92) Time & Position Dependencies 3/3

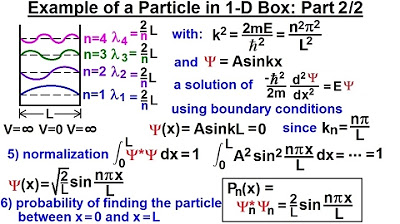
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

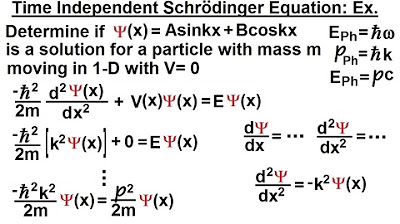
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (15 of 92) Time & Position Dependencies Ex.*

What Is Quantum Mechanics Explained

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsPhysicsTutorialSchrodingerEquationWaveFunctionKineticEnergyPotentialEnergyMathDerivationEducationalContentPhysicsEnthusiastsQuantumTheory