A Nice Math Olympiad Geometry Challenge | Circle inside a semicircle | Find the radius of the circle
TLDRThe video script presents a geometrical problem involving a circle inscribed within a semicircle. Given the lengths of CD (3 units) and BD (4 units), the goal is to calculate the radius of the circle. By using principles of symmetry, circle chord properties, and the Pythagorean theorem, the script guides through a series of calculations to ultimately determine the radius. The solution involves setting up equations based on the relationship between the radii, chords, and the segments of the circle and semicircle, solving for the unknown radius, and concluding with a positive value of 3/2 units for the radius of the circle.
Takeaways
- 📐 The problem involves a circle inside a semicircle with given lengths CD (3 units) and BD (4 units).
- 🔍 By symmetry, if CD is extended to point E, then CD = DE = 3 units.
- 🔶 The relationship between chords in a circle is used: aod * bod = cod * ed.
- 🤔 The diameter of the semicircle is AB, and its radius (uppercase R) is calculated as (aD + BD) / 2.
- 📐 The radius of the circle (lowercase r) is found by solving a quadratic equation involving the lengths of the chords and segments.
- 🌐 The centers of the semicircle and circle are denoted as P and Q, respectively.
- 🔼 The perpendicular from the center of a circle to a tangent at a point of contact is used to find the lengths of segments PQ and QM.
- 📐 Pythagorean theorem is applied to find the length of PQ.
- 🔢 A quadratic equation in terms of the radius r is formed and solved to find the value of the circle's radius.
- 🌀 The discriminant of the quadratic equation is calculated to find the possible values of the radius.
- 🎯 The positive value of the radius is selected as lengths are positive.
Q & A
What is the given information about the circle and semicircle in the problem?
-The problem describes a circle inscribed in a semicircle. The length of the chord CD is 3 units, and the length of the diameter BD of the semicircle is 4 units.
How does the symmetry of the circle help in finding the length of DE?
-Due to the symmetry of the circle, if CD is extended to point E, then CD will be equal to DE, which is 3 units in length.
What is the relationship between the lengths of chords a and CD in the circle?
-The product of the lengths of chords a and CD (a * d) is equal to the product of the lengths of chords a and BD (a * b), which is 9/4 units squared.
How is the radius of the semicircle calculated?
-The radius of the semicircle (denoted as R) is half the length of the diameter AB. It is calculated using the formula R = (a/2 + BD/2), where a is the length of the chord intersecting the semicircle at point D, BD is the diameter of the semicircle, and the result is 25/8 units.
What is the significance of the center of the semicircle (P) and the circle (Q) in the problem?
-The centers P and Q are significant because they are used to calculate the length of PQ, which is the distance between the centers of the two touching circles. This distance is crucial for finding the radius of the circle (r).
How is the length of PQ determined?
-The length of PQ is determined by subtracting the radius of the circle (r) from the radius of the semicircle (R), which is calculated to be 25/8 units. So, PQ = (25/8) - r.
What is the relationship between the lengths of DB, DP, and the radius of the circle (r)?
-DB is the length of the diameter of the semicircle minus PB, which is calculated as 4 - (25/8), resulting in 7/8 units. DP is the length DB minus PB, which is also 7/8 units. These lengths are used in the Pythagorean theorem to find the radius of the circle (r).
How is the radius of the circle (r) ultimately calculated?
-The radius of the circle (r) is calculated by solving a quadratic equation derived from the Pythagorean theorem and the relationships between the lengths of the chords and radii. The equation is 2r^2 + 9r - 18 = 0, and the positive solution for r is 3/2 units.
Why is the negative solution for the radius discarded?
-The negative solution for the radius is discarded because a radius, being a length, must be a positive value. Therefore, only the positive solution is considered valid for the radius of the circle.
What is the final answer for the radius of the circle?
-The final answer for the radius of the circle is 3/2 units.
How does the Pythagorean theorem play a role in solving this problem?
-The Pythagorean theorem is used to relate the lengths of the segments PQ, PM, and QM, which are essential in deriving the equation to solve for the radius of the circle.
What is the significance of the angle 90° in the problem?
-The angle of 90° is significant because it indicates that the radius and the tangent to the circle at the point of contact are perpendicular to each other, which is a property of circles and is used in the derivation of the Pythagorean relationship.
Outlines
📐 Geometrical Analysis of a Circle and Semicircle
The paragraph discusses the process of finding the radius of a circle given a circle inside a semicircle. It starts by describing the setup, where CD and BD are known lengths, and uses symmetry to establish equal lengths. The paragraph then applies the properties of circles, specifically the relationship between chords and the product of their segments intersected by another line. By equating areas and using algebraic manipulation, the radius of the semicircle (r) and the circle (R) are calculated. The paragraph concludes with the calculation of the distance between the centers of the semicircle and the circle, using Pythagorean theorem and the established values.
📐 Further Geometrical Calculations and Relations
This paragraph continues the geometrical analysis by focusing on the relationship between the radius of the circle (r), the semicircle (R), and the distance between their centers (PQ). It introduces new points M and N to further illustrate the geometrical relationships and uses the properties of tangents and radii to establish equations. The paragraph then proceeds to solve for the unknown radius (r) using algebraic methods, including the application of Pythagorean theorem and quadratic equations, ultimately finding the positive value for r.
📐 Solving for the Radius with Quadratic Equations
The paragraph delves into the algebraic aspect of the problem, setting up a quadratic equation to find the radius (r) of the circle. It starts by simplifying the quadratic equation and then solving it using the quadratic formula. The process involves identifying the coefficients of the equation and calculating the discriminant. The paragraph concludes by finding the two possible values for r, but since the radius cannot be negative, it discards the negative solution, leaving the positive value as the correct answer.
🎶 Final Solution and Positive Radius Confirmation
In the final paragraph, the solution for the radius of the circle is confirmed to be positive, as a length cannot be negative. The paragraph concludes the geometrical and algebraic analysis by reiterating the calculated radius of the circle, which is 3/2. The inclusion of a music emoji suggests a conclusion to the problem-solving process, possibly indicating a sense of accomplishment or relief.
Mindmap
Keywords
💡Circle
💡Semicircle
💡Radius
💡Symmetry
💡Chord
💡Diameter
💡Tangent
💡Pythagorean Theorem
💡Quadratic Equation
💡Intersection
💡Perpendicular
Highlights
The problem involves finding the radius of a circle given a circle inside a semicircle with specific measurements.
CD is given as 3 and BD is 4, which are used to find the radius.
By extending CD to point E, symmetry is used to establish that CD equals ED, both being 3.
A property of circles is applied where the product of two chords intersecting at a point is equal to the product of the other two chords.
The radius of the semicircle (uppercase R) is calculated using the diameter (AB) and the segments AD and BD.
The center of the semicircle is denoted as P and the center of the circle as Q.
PQ is shown to pass through the common point of contact (S), which is perpendicular to the tangent at that point.
The relationship between the radii (uppercase R and lowercase r) and the lengths PS, Qs, and PQ is established.
A right-angled triangle is formed with PQ, PM, and QM, and Pythagoras' theorem is applied to find the values.
A quadratic equation is formed to solve for the radius (r) of the circle.
The quadratic equation is solved to find the two possible values of the radius, but only the positive value is considered as it represents a length.
The final answer for the radius of the circle is given as 3/2.
The method used combines geometric properties, algebraic manipulation, and the application of Pythagoras' theorem.
The problem demonstrates the application of mathematical concepts to solve real-world geometrical problems.
The solution process is logical and methodical, providing a clear step-by-step guide to finding the radius.
The video is an example of educational content that teaches viewers how to approach and solve complex geometric problems.
Transcripts
Browse More Related Video
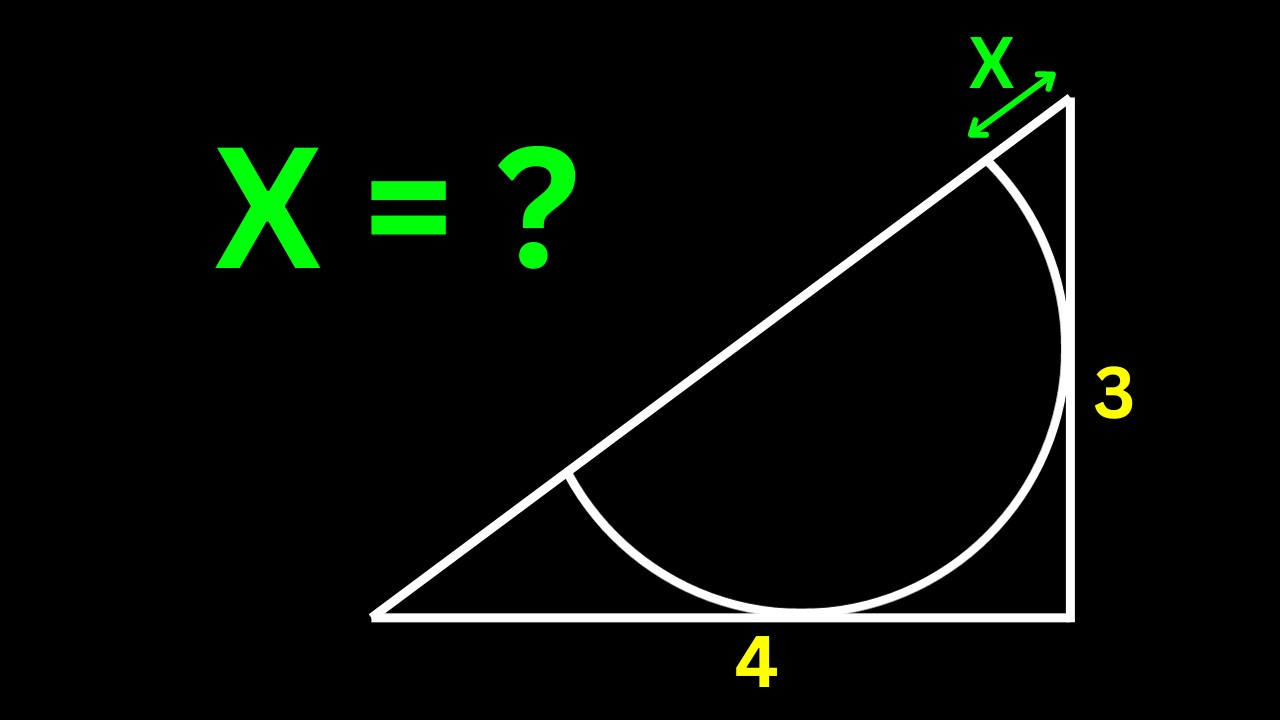
Canada Math Olympiad Problem | Best Math Olympiad Problems | Geometry
A square is inscribed in a circle with radius = 5, what is the area of the square?

Hungary Math Olympiad Problem | Best Math Olympiad Problems | Geometry Problem
Distance Formula
EQUATION OF CIRCLE IN STANDARD FORM | PROF D

What are radians? Simply explained
5.0 / 5 (0 votes)
Thanks for rating: