Optimisation Grade 12: Maximum Volume Cone
TLDRIn this educational video transcript, the speaker addresses the challenge of remembering mathematical formulas, particularly for calculating the volume and surface area of a cone. They suggest that students should memorize these formulas, as they are not always provided in exams. The speaker then guides the audience through the process of finding the maximum volume of a cone given the sum of its radius and height is 14. They use calculus to derive the formula for the cone's volume, set up a quadratic equation, and solve for the height that maximizes the volume, concluding that the optimal height is approximately 4.67 units.
Takeaways
- 📚 The script discusses the challenge of remembering mathematical formulas for calculating volume and surface area, especially for shapes like a cone.
- 💡 It suggests that students should take the time to learn and memorize these formulas, as they are commonly used and not provided in exams.
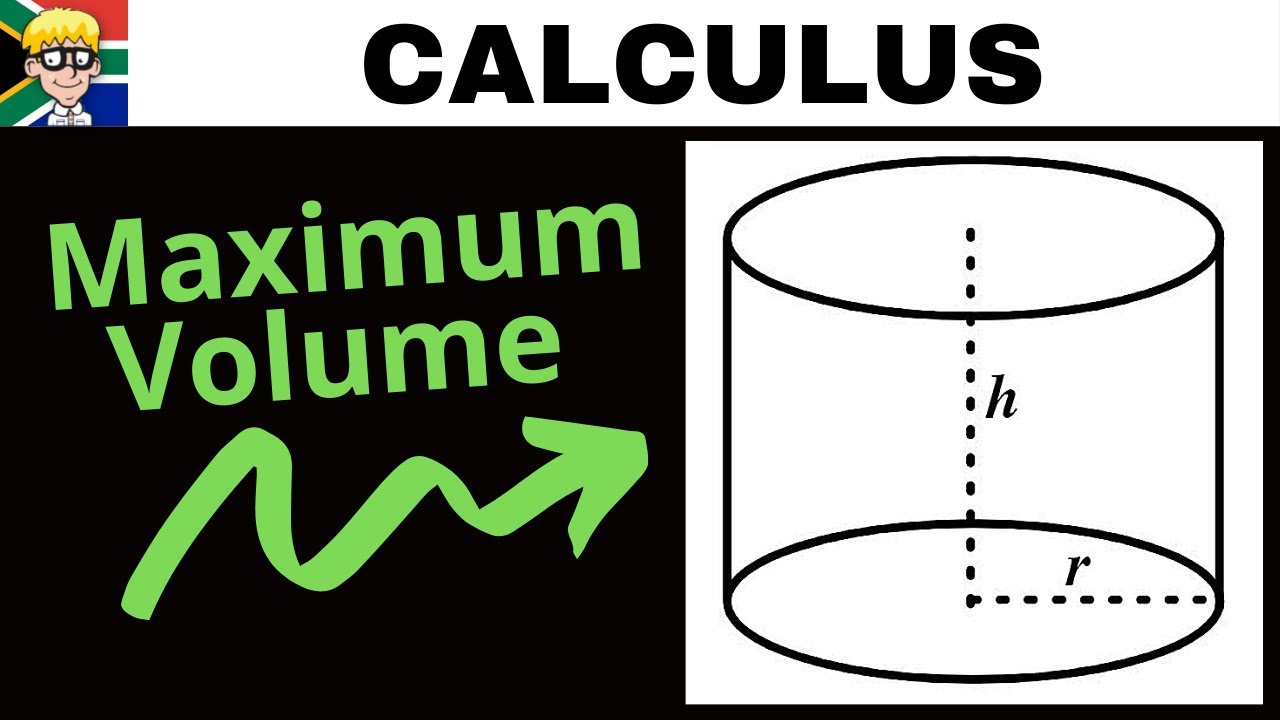
- 📐 The speaker provides a hint that the volume of a cone is similar to that of a cylinder, which is the area of the base times the height, divided by three.
- 🔍 The script emphasizes that pi is a constant and not a variable, simplifying the formula for the volume of a cone to \( \frac{1}{3} \pi r^2 h \).
- 🔢 The problem at hand involves determining the value of 'h' (height) that maximizes the volume of the cone, given that the sum of the radius and height is 14.
- 📝 The script recommends isolating the variable 'h' by expressing the radius 'r' as \( 14 - h \), and then substituting it into the volume formula.
- 📉 To find the maximum volume, the script explains to take the first derivative of the volume equation with respect to 'h' and set it equal to zero.
- 🧩 The derivative simplifies to a quadratic equation, which can be solved using the quadratic formula or by factoring, if possible.
- 🔑 The script points out that one of the potential solutions for 'h' is discarded because it would result in a zero radius, which is not feasible for a cone.
- 🎯 The correct value of 'h' that maximizes the volume of the cone is found to be approximately 4.67, not 14, ensuring a positive radius and height.
- 📚 The overall message is the importance of understanding and applying mathematical concepts and formulas to solve real-world problems, such as optimizing the volume of a cone.
Q & A
Why does the speaker suggest remembering the formulas for the volume and surface area of shapes?
-The speaker suggests remembering the formulas because they are often not provided in exams, and having access to these formulas in real life is similar to having access to them in the exam, emphasizing the importance of memorization for practical use.
What is the main topic discussed in the script?
-The main topic discussed in the script is finding the value of 'h' that maximizes the volume of a cone, including the process of deriving and solving the related mathematical equation.
What is the formula for the volume of a cone as mentioned in the script?
-The formula for the volume of a cone mentioned in the script is \( \frac{1}{3} \pi r^2 h \), where 'r' is the radius of the base and 'h' is the height of the cone.
Why does the speaker say that pi (π) is not a variable?
-The speaker says that pi is not a variable because it is a constant number approximately equal to 3.14, and it does not change or vary in mathematical calculations.
What is the relationship between the volume of a cone and the volume of a cylinder with the same radius and height?
-The volume of a cone is one-third of the volume of a cylinder with the same radius and height.
What additional information is given about the cone in the script to help find the value of 'h'?
-The additional information given is that the sum of the radius 'r' and the height 'h' of the cone is 14, which can be expressed as \( h + r = 14 \).
How does the speaker simplify the volume formula to have only 'h' as the variable?
-The speaker simplifies the volume formula by expressing the radius 'r' in terms of 'h' as \( r = 14 - h \) and then substituting this expression into the volume formula.
What mathematical method is used to find the maximum volume of the cone?
-The method used to find the maximum volume of the cone is calculus, specifically taking the first derivative of the volume equation with respect to 'h' and setting it equal to zero.
Why can't the height 'h' be 14 according to the script?
-The height 'h' cannot be 14 because, according to the given information, the sum of the radius and height must be 14. If 'h' were 14, the radius 'r' would be 0, which would not form a cone but a straight line with zero volume.
What is the value of 'h' that maximizes the volume of the cone according to the script?
-The value of 'h' that maximizes the volume of the cone is approximately 4.67, as determined by solving the quadratic equation derived from the first derivative of the volume formula.
Outlines
📚 Maximizing Cone Volume with Geometry
The speaker addresses the challenge of remembering geometric formulas, particularly for calculating the volume and surface area of a cone, which is a common issue for grade 12 students. The speaker suggests that while it would be helpful to have formulas provided in exams, this is not always the case, and thus encourages students to take the time to learn and memorize these formulas. The main focus of the paragraph is to find the value of 'h' that maximizes the volume of a cone. The speaker provides a hint that the volume of a cone is one-third of the volume of a cylinder with the same base area and height. Using the given relationship between the radius (r) and height (h) of the cone, which is the sum of r and h equals 14, the speaker guides through the process of substituting r with (14 - h) in the volume formula and then taking the derivative to find the maximum volume. The paragraph concludes with the setup for finding the maximum volume by setting the derivative equal to zero.
🔍 Solving the Cone Volume Optimization Problem
This paragraph continues the discussion on finding the maximum volume of a cone, focusing on the mathematical process of optimization. The speaker explains the process of taking the first derivative of the volume formula with respect to 'h' and setting it equal to zero to find the critical points. After simplifying the derivative and eliminating the constant π, the speaker arrives at a quadratic equation. The quadratic formula is then used to solve for 'h', yielding two possible solutions. However, the speaker points out that one of the solutions, h = 14, is not feasible because it would imply a cone with a zero radius, which is not practical. The correct solution, h = 4.67, is identified as the one that maximizes the volume of the cone, given the constraint that the sum of the radius and height is 14. The paragraph concludes with the correct value of 'h' that achieves the maximum volume of the cone.
Mindmap
Keywords
💡Volume
💡Surface Area
💡Cone
💡Formulas
💡Derivative
💡Maximum
💡First Derivative
💡Quadratic Formula
💡Cylinder
💡Optimization
💡Pi (π)
Highlights
The question is tricky for many grade 12 students as they often forget formulas for volume and surface area of shapes like cones.
It is suggested that formulas should be provided in exams as they are easily accessible in real life.
The speaker recommends spending 30 minutes to find and memorize the formulas for shapes like cones.
The volume of a cone is the same as that of a cylinder with the same base area and height, divided by 3.
The volume formula for a cone is derived by comparing it to a cylinder and dividing by 3.
The problem involves two variables, radius (r) and height (h), with the constraint that r + h = 14.
To find the maximum volume, isolate one variable, in this case height (h), using the given constraint.
Substitute the expression for r (14 - h) into the volume formula to get an equation in terms of h.
Simplify the volume equation to get a cubic equation in terms of h.
To find the maximum volume, take the first derivative of the volume equation with respect to h and set it to zero.
The derivative simplifies to a quadratic equation in terms of h.
Solve the quadratic equation using the quadratic formula to find the possible values of h.
The height value of 14 is not feasible as it would result in a cone with zero radius and volume.
The correct height value that maximizes the volume of the cone is approximately 4.67.
The process involves memorizing formulas, substituting variables, simplifying equations, and applying calculus to find maximums.
The speaker emphasizes the importance of understanding the relationship between a cone and a cylinder for volume calculations.
The method demonstrates a practical approach to solving geometry problems by connecting them to real-life scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: