AP Physics B Kinematics Presentation #17

The New Jersey Center for Teaching and Learning
26 Jun 201204:05
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript discusses a physics problem about an object moving with a constant acceleration of 5 meters per second squared. It evaluates different statements about the object's motion, including its velocity, distance traveled, and acceleration changes. Through kinematic equations, it is determined that the correct statement is that the object's velocity increases by 5 meters per second each second. The analysis dismisses other options, such as constant velocity, moving 5 meters per second, and changing acceleration, confirming the object's consistent velocity increase due to constant acceleration.

Takeaways
  • 🚀 An object moves with a constant acceleration of 5 meters per second squared.
  • ❌ The object's velocity stays the same is incorrect because constant acceleration means changing velocity.
  • 📉 The object moves 5 meters each second is incorrect as the position changes quadratically with respect to time.
  • 📏 Using the equation x = 1/2 * a * t^2, the object moves 2.5 meters in one second.
  • 🛑 The object's acceleration increasing by 5 meters per second squared each second is incorrect due to constant acceleration.
  • 🛑 The object's acceleration decreasing by 5 meters per second squared each second is also incorrect.
  • 📈 The object's velocity increases by 5 meters per second each second is correct.
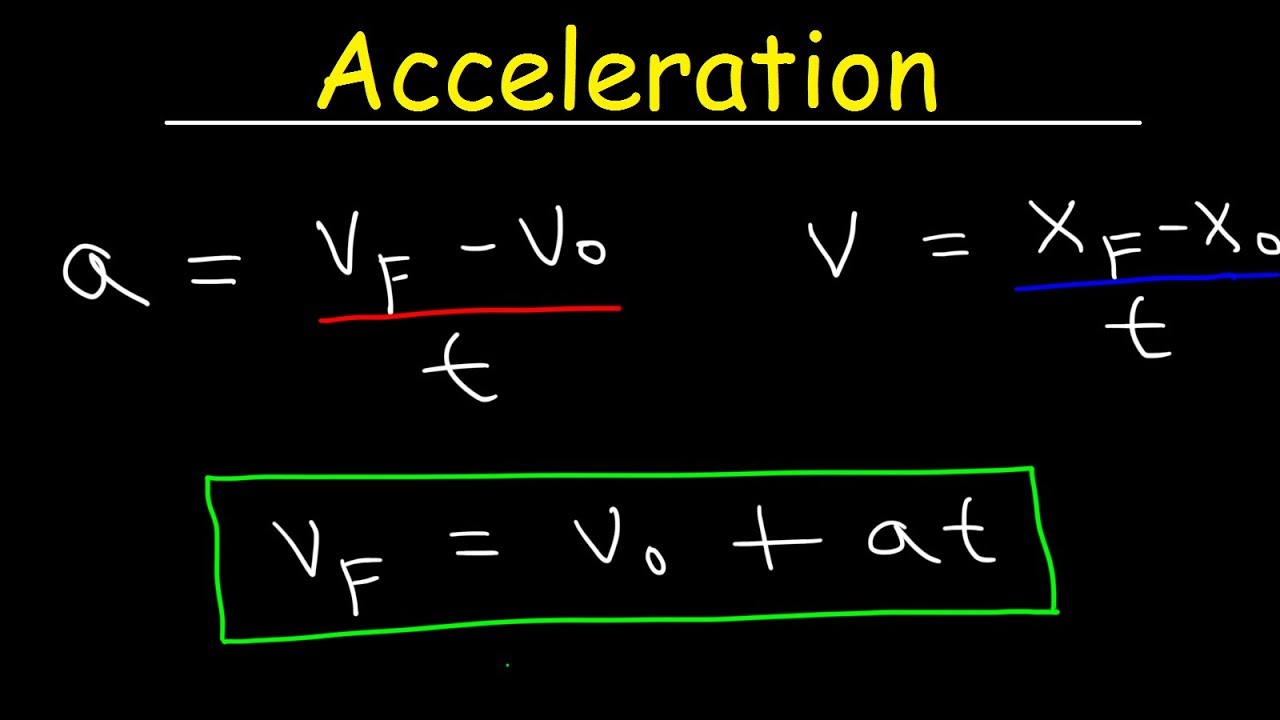
  • 📐 Using the kinematic equation v = v_0 + a*t confirms the velocity increase.
  • 🕒 If the initial velocity is zero, after one second, the velocity becomes 5 meters per second.
  • ✔️ The correct statement is that the object's velocity increases by 5 meters per second each second.
Q & A

  • What is the given acceleration of the object?

    -The object has a constant acceleration of 5 meters per second squared.

  • Why is the statement 'the object's velocity stays the same' incorrect?

    -The statement is incorrect because with a constant acceleration, the object's velocity changes over time according to the kinematic equation v = v_0 + at.

  • Why does the object not move 5 meters each second?

    -The object does not move 5 meters each second because the distance covered is given by x = 0.5at^2. With an acceleration of 5 meters per second squared, it moves 2.5 meters in the first second.

  • Why is the statement 'the object's acceleration increases by 5 meters per second squared each second' incorrect?

    -This statement is incorrect because the problem states that the acceleration is constant at 5 meters per second squared, so it does not increase.

  • Why is the statement 'the object's acceleration decreases by 5 meters per second squared each second' incorrect?

    -This statement is incorrect because the problem specifies a constant acceleration of 5 meters per second squared, so it does not decrease.

  • Why is the statement 'the object's velocity increases by 5 meters per second each second' correct?

    -The statement is correct because with a constant acceleration of 5 meters per second squared, the object's velocity increases by 5 meters per second every second.

  • How does the kinematic equation v = v_0 + at explain the change in velocity?

    -The equation v = v_0 + at shows that velocity changes linearly with time when acceleration is constant. For a = 5 m/s^2 and v_0 = 0, the velocity increases by 5 meters per second every second.

  • What is the distance covered by the object after 1 second?

    -The distance covered by the object after 1 second is 0.5 * 5 * (1)^2 = 2.5 meters.

  • If the initial velocity is zero, how do we calculate the final velocity after a certain time?

    -The final velocity can be calculated using the equation v = at. For example, after 1 second with an acceleration of 5 meters per second squared, the final velocity is 5 * 1 = 5 meters per second.

  • What assumptions are made about the initial conditions in the script's analysis?

    -The analysis assumes that the initial position x_0 is zero meters and the initial velocity v_0 is zero meters per second.

  • How does the quadratic nature of the position equation x = 0.5at^2 affect the distance traveled over time?

    -The quadratic nature of the position equation means that the distance traveled increases with the square of the time, resulting in greater distances over longer time intervals.

Outlines
00:00
🚀 Explanation of Constant Acceleration

The object in question moves with a constant acceleration of 5 meters per second squared. Several statements are evaluated to determine their truthfulness based on this constant acceleration. The key points discussed include: - Statement A, which claims the object's velocity stays the same, is refuted using the first kinematic equation. - Statement B, which asserts the object moves 5 meters each second, is disproved using the third kinematic equation, showing the actual distance is 2.5 meters in one second. - Statement C is incorrect as it suggests increasing acceleration, while the problem states constant acceleration. - Statement D is also incorrect as it implies decreasing acceleration, contradicting the constant acceleration given. - Statement E is verified as true, showing that the object's velocity increases by 5 meters per second each second, confirmed by the first kinematic equation.

Mindmap
Keywords
💡Constant acceleration
Constant acceleration refers to a steady increase in velocity over time. In the video, it is mentioned that an object has a constant acceleration of 5 meters per second squared, meaning the object's velocity increases at a consistent rate. This concept is crucial for understanding the motion being described, as it underpins the calculation of the object's changing velocity and position.
💡Velocity
Velocity is the speed of an object in a specific direction. In the context of the video, the object's velocity changes due to the constant acceleration, following the equation v = v₀ + at. The discussion emphasizes that the object's velocity does not stay the same, contradicting option A in the problem statement.
💡Kinematic equation
Kinematic equations are used to describe the motion of objects. The video refers to the first and third kinematic equations: v = v₀ + at and x = x₀ + v₀t + 1/2at², respectively. These equations are used to determine the final velocity and position of the object over time, given initial conditions and constant acceleration.
💡Position
Position refers to the location of an object at a particular time. The video explains that the object's position can be calculated using the third kinematic equation, considering initial position and velocity as zero. It demonstrates that the object does not move 5 meters each second, as stated in option B, but rather follows a quadratic relationship with time.
💡Initial velocity (v₀)
Initial velocity (v₀) is the velocity of an object at the start of a time period. In the video, the initial velocity is considered zero for simplicity. This concept is important for calculating changes in velocity and position over time under constant acceleration.
💡Final velocity (v)
Final velocity (v) is the velocity of an object at the end of a time period. The video illustrates how final velocity is calculated using the equation v = v₀ + at. With a constant acceleration of 5 meters per second squared, the final velocity increases by 5 meters per second for every second that passes.
💡Distance
Distance refers to the total path length covered by an object. In the video, it is clarified that the distance covered by the object increases over time due to acceleration, and it does not remain constant at 5 meters per second as option B incorrectly suggests.
💡Acceleration
Acceleration is the rate at which an object's velocity changes. In the context of the video, the object experiences a constant acceleration of 5 meters per second squared. This means the object's velocity increases steadily, which is key to understanding why option C (which suggests increasing acceleration) is incorrect.
💡Quadratic relationship
A quadratic relationship in this context refers to the position of the object depending on the square of time (t²) when acceleration is constant. The video explains that the object's position is given by x = 1/2 at², showing that the distance covered increases more rapidly over time, not linearly.
💡Option E (correct answer)
Option E states that the object's velocity increases by 5 meters per second each second, which is correct. The video confirms this by explaining that with a constant acceleration of 5 meters per second squared, the velocity increases consistently. This aligns with the kinematic equation v = v₀ + at, validating option E as the accurate description of the object's motion.
Highlights

The object moves with a constant acceleration of 5 meters per second squared.

The object's velocity does not stay the same because acceleration dictates that velocity is changing.

The first kinematic equation is used to show that final velocity is different from initial velocity with constant acceleration.

The object's position can be calculated using the third kinematic equation.

Initial position and velocity are assumed to be zero to simplify the calculation.

The position of the object after 1 second is calculated as 2.5 meters, not 5 meters, indicating that position is quadratic with respect to time.

The statement that the object moves 5 meters each second is incorrect.

The statement that the object's acceleration increases by 5 meters per second squared each second is incorrect.

The statement that the object's acceleration decreases by 5 meters per second squared each second is incorrect.

The problem states a constant acceleration of 5 meters per second squared, so neither an increase nor a decrease in acceleration is correct.

The object's velocity increases by 5 meters per second each second.

The final velocity after 1 second is calculated as 5 meters per second using the first kinematic equation.

The increase in velocity is consistent with the acceleration, confirming that velocity increases linearly with time under constant acceleration.

Choice E, which states that the object's velocity increases by 5 meters per second each second, is correct.

All other choices (A, B, C, and D) are incorrect based on the given information and calculations.

Transcripts

Browse More Related Video

Why distance is area under velocity-time line | Physics | Khan Academy

AP Physics B Kinematics Presentation #12

Physics - Acceleration & Velocity - One Dimensional Motion

College Physics 1: Lecture 8 - Acceleration

motion graphs explained

Airbus A380 take-off time | One-dimensional motion | Physics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
KinematicsPhysicsAccelerationVelocityConstant AccelerationEquationsMotionScience EducationNewton's LawsProblem Solving