Geometric Optics 2
TLDRThis educational script delves into the principles of optics, focusing on total internal reflection and its applications in fiber optics and everyday objects like glass edges and magnifying glasses. It explains Snell's law, the critical angle, and the behavior of light through lenses, detailing how lenses form real and virtual images based on their curvature and the object's distance. The script also explores the use of lenses in devices like cameras and telescopes, and how the lens power is measured in diopters, providing a comprehensive overview of optical phenomena and their practical implications.
Takeaways
- 🌟 Total internal reflection occurs when light travels from a medium with a higher index of refraction to one with a lower index, and the angle of incidence is greater than the critical angle, causing all light to be reflected back into the denser medium.
- 🔍 The critical angle can be calculated using Snell's Law, where the sine of the angle of transmission equals the ratio of the refractive indices of the two media, and the sine of 90 degrees is 1.
- 💧 In water, the critical angle for transitioning to total internal reflection when moving to air is approximately 48.75 degrees, based on the refractive index of water being 1.33.
- 🌌 Total internal reflection is responsible for the visibility of the sky when underwater at certain angles and the sudden disappearance of the sky's view beyond the critical angle, creating a 'perfect mirror' effect.
- 🌐 Fiber optics utilize total internal reflection to transmit light signals over long distances, with light entering at an angle that keeps it confined within the glass fiber.
- 💡 High-power LED lights around the edges of glass can create a total internal reflection effect, keeping the light within the glass unless an obstruction like a hand or ink is introduced, a phenomenon known as frustrated total internal reflection.
- 🔑 Understanding Snell's Law and the concept of the index of refraction is fundamental to the function of lenses, which can be used to focus or diverge light rays depending on their curvature.
- 👓 Positive or converging lenses, such as those found in reading glasses or magnifying glasses, are thicker at the center and thinner at the edges, and can be used to correct vision or enlarge images.
- 🔍 Negative or diverging lenses, such as those in some eyeglasses for myopia, are thinner at the center and thicker at the edges, and can only form virtual, upright, and smaller images.
- 📚 The power of a lens is measured in diopters, with positive values indicating converging lenses and negative values indicating diverging lenses.
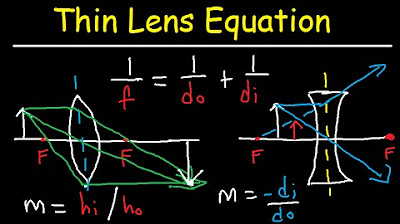
- 🔄 The thin lens equation (1/Do + 1/Di = 1/F) is similar to the mirror equation but has different signs for the distances, allowing for the calculation of image formation based on the object distance and lens focal length.
Q & A
What is total internal reflection?
-Total internal reflection is a phenomenon where light traveling from a medium with a higher index of refraction to one with a lower index of refraction is completely reflected back into the higher index medium when the angle of incidence exceeds a certain critical angle.
What is the critical angle in the context of total internal reflection?
-The critical angle is a special angle at which the angle of incidence is such that the refracted ray travels exactly along the boundary between the two media, making the angle of refraction 90 degrees.
How can you mathematically determine the critical angle for light going from water to air?
-The critical angle can be determined using Snell's Law, where the sine of the critical angle (θc) is equal to the ratio of the refractive indices of air (nT) to water (nI), or θc = arcsin(nT / nI). For water to air, it would be arcsin(1 / 1.33).
What is the critical angle for light going from water to air?
-The critical angle for light going from water to air is approximately 48.75 degrees, calculated using the formula arcsin(1 / 1.33).
What is the practical application of total internal reflection in fiber optics?
-In fiber optics, total internal reflection is used to keep light signals confined within the fiber, allowing for efficient transmission of data over long distances with minimal loss.
What is frustrated total internal reflection?
-Frustrated total internal reflection occurs when the light that is being totally internally reflected is 'pulled out' of the medium by some external means, such as touching the surface or applying ink, which disrupts the conditions for total internal reflection.
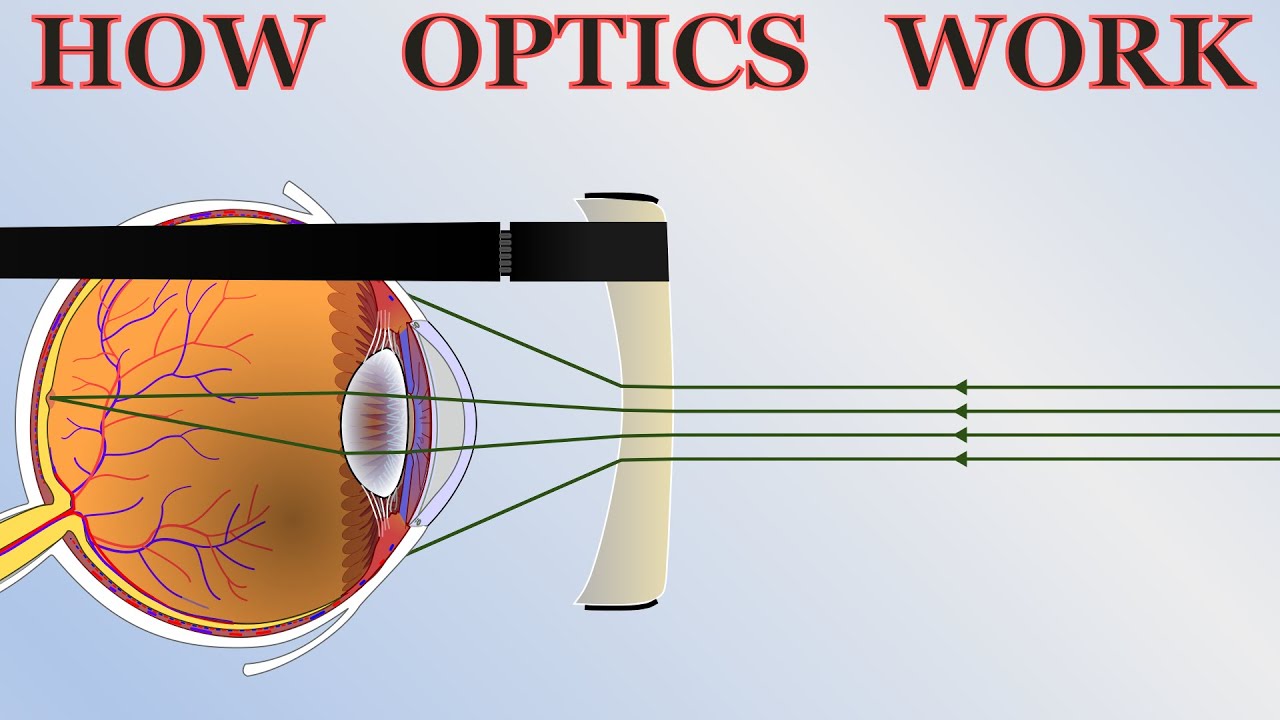
How does the shape of a lens affect the path of light passing through it?
-The shape of a lens, whether convex (bulging outward) or concave (curving inward), will cause light to bend or refract as it passes through the lens. Convex lenses converge light rays towards a focal point, while concave lenses diverge them.
What are the three rules for image formation by a lens?
-The three rules are: 1) Rays parallel to the optic axis are refracted through the focal point on the other side of the lens. 2) Rays passing through the lens's focal point continue in a direction parallel to the optic axis. 3) Rays passing through the center of the lens continue without changing direction.
What is the difference between a real image and a virtual image in the context of lenses?
-A real image is formed where actual light rays converge and can be projected onto a screen, whereas a virtual image is formed where the light rays appear to diverge from and cannot be projected onto a screen; it is only visible by looking into the lens.
How does the position of an object relative to the focal length of a lens affect the nature of the image formed?
-When an object is placed beyond twice the focal length of a lens, a real, inverted, and reduced image is formed. When the object is within the focal length, a virtual, upright, and magnified image is formed.
What is the unit used to measure the power of a lens?
-The power of a lens is measured in diopters, which is the reciprocal of the lens's focal length in meters.
How can you use reading glasses to view a partial solar eclipse safely?
-By covering reading glasses with tinfoil and poking a small hole in it, you can project an image of the Sun onto a piece of paper at a safe distance, allowing you to view the partial eclipse without directly looking at the Sun.
Outlines
🌊 Total Internal Reflection Explained
The paragraph introduces the concept of total internal reflection, a phenomenon where light traveling from a medium with a higher index of refraction to one with a lower index is completely reflected back into the denser medium at a certain angle, known as the critical angle. The critical angle is calculated using Snell's law, where the sine of the angle of incidence equals the ratio of the indices of refraction. The script uses the example of light traveling from water to air, with the critical angle calculated to be approximately 48.75 degrees. This concept is demonstrated practically through an experiment in a swimming pool, where the water surface acts as a perfect mirror at angles above the critical angle.
🔍 Applications of Total Internal Reflection
This section discusses the applications of total internal reflection in everyday life and technology. It describes an experiment where looking underwater at an angle reveals the sky until the critical angle is reached, after which the water surface acts as a mirror. The paragraph also mentions the use of total internal reflection in fiber optics, which is fundamental to internet communication, and in edge-lit glass displays, where light is kept inside the glass by the same principle. The concept of frustrated total internal reflection is introduced, where adding a foreign substance to the glass surface can pull light out of the glass, demonstrating the sensitivity of total internal reflection to surface conditions.
👓 Understanding Lenses and Their Properties
The script delves into the principles of lenses, starting with the effects of a curved piece of glass on light paths. It explains how a lens can be designed to focus light rays to a single point, known as the focal point, creating a thin lens. The types of lenses are categorized based on their curvatures as convex, concave, plano-convex, and plano-concave. The importance of lens design in creating various optical elements like telescopes, microscopes, and camera lenses is highlighted, emphasizing the complexity of correcting for aberrations and achieving a good image with multiple lenses.
📐 The Three Rules for Image Formation by Lenses
This paragraph outlines the three rules for determining the image formation by lenses, which are analogous to the rules for mirrors. The rules are: 1) Parallel rays to the optic axis will converge at the focal point after passing through the lens, 2) Rays passing through the lens's focal point will exit parallel to the optic axis, and 3) Rays passing through the center of the lens will continue without bending. These rules are applied to form a real, inverted image on a screen or film, demonstrating the lens's ability to create a real image based on the object's position relative to the lens.
🌳 Image Formation with a Converging Lens
The script explores the concept of image formation when an object is placed within the focal length of a converging lens. It explains that the image formed in this scenario is virtual, upright, and magnified. The explanation includes the application of the lens rules to determine the location of the virtual image, emphasizing that the rays appear to diverge from a point behind the lens, creating the perception of an upright, magnified image that cannot be projected onto a screen.
👁️ Power of Lenses and Magnifying Effects
This section discusses the power of lenses, measured in diopters, which is the reciprocal of the focal length in meters. It explains how positive lenses, such as reading glasses, can be used as magnifying glasses and how their power is indicated on the prescription. The paragraph also provides a practical tip on using reading glasses with tin foil to safely view partial solar eclipses by creating a pinhole effect, demonstrating the versatility of lenses in everyday applications.
🔦 Negative Lenses and Their Image Formation
The script describes negative or diverging lenses, which form virtual, upright, and reduced images. It explains that the focal points of a negative lens are on the same side as the light source, and the lens causes light rays to diverge, creating the illusion that they originate from a point behind the lens. The unique characteristic of negative lenses is that they can only form virtual images, which has implications for their use in optical devices and survival scenarios, such as starting a fire.
📚 The Thin Lens Equation and Its Application
This paragraph introduces the thin lens equation, which is analogous to the mirror equation, with the formula 1/Do + 1/Di = 1/F, where Do is the object distance, Di is the image distance, and F is the focal length. The script demonstrates how to use the thin lens equation to calculate the image distance for a given object distance and focal length, using an example of photographing a tree with a camera lens. It illustrates the practical application of the lens equation in determining the position of the image formed by a lens.
🔬 Further Exploration of the Thin Lens Equation
The final paragraph reiterates the thin lens equation and its significance in optics. It emphasizes the importance of understanding the sign conventions for measuring distances in the lens equation and provides a step-by-step calculation for determining the image distance when the object is placed at a known distance from the lens. The explanation reinforces the concept that as the object distance increases, the image distance approaches the focal length, which is why modern cameras can focus on objects at various distances effectively.
Mindmap
Keywords
💡Total Internal Reflection
💡Index of Refraction
💡Critical Angle
💡Snell's Law
💡Fiber Optics
💡Lens
💡Converging Lens
💡Diverging Lens
💡Focal Length
💡Power of a Lens
💡Magnification
Highlights
Total internal reflection occurs when light travels from a high index medium to a low index medium and reaches an angle where it no longer exits the medium.
The critical angle for total internal reflection is calculated using Snell's law, where the sine of the angle equals the ratio of the indices of refraction.
In water-to-air scenarios, the critical angle is approximately 48.75 degrees, beyond which light is entirely reflected within the water.
Total internal reflection can be observed in everyday experiences, such as seeing the sky when underwater at certain angles.
Fiber optics utilize total internal reflection to transmit light signals over long distances, forming the backbone of the internet.
High power LED strips around the edge of a glass can demonstrate total internal reflection, keeping light inside the glass.
Frustrated total internal reflection occurs when an object on the glass surface pulls light out of the glass, visible as a change in light reflection.
Lenses can be made from curved glass, which bends light according to Snell's law and the lens's shape, creating a focus point.
Different types of lenses, such as convex, concave, plano-convex, and plano-concave, have distinct curvatures and light bending properties.
Lenses can correct vision, create telescopes, and microscopes by shaping glass to manipulate light effectively.
The three rules for lens imaging include: parallel rays go through the focus, rays through the focus go parallel, and rays through the center do not bend.
Blocking part of a lens reduces the image brightness but does not change its location or quality, demonstrating the collective effect of many rays.
Converging lenses, or positive lenses, are used in reading glasses and can be identified by their ability to start fires due to light convergence.
The power of a lens is measured in diopters, with positive values indicating converging lenses and negative values indicating diverging lenses.
Negative lenses, or diverging lenses, only form virtual images that are upright and smaller than the object, as seen with biconcave lenses.
The thin lens equation relates the object distance, image distance, and focal length, essential for understanding image formation in optics.
Practical applications of the thin lens equation include calculating image distances for cameras and understanding focus in various optical devices.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: