Ray Diagrams
TLDRThis educational video explores the concept of ray diagrams, focusing on spherical mirrors, specifically concave and convex mirrors, and their properties. It explains how the position of an object relative to the focal point determines the type of image formed: real or virtual, and its orientation: upright or inverted. The script delves into the principles of magnification, the mirror and lens equations, and the power of lenses measured in diopters. It also contrasts the effects of convergent and divergent lenses on image formation, providing a clear guide to understanding optical phenomena.
Takeaways
- 🔍 The video discusses ray diagrams, focusing on spherical mirrors, particularly concave mirrors, and their imaging properties.
- 📏 The principal axis is a horizontal line used as a reference in the ray diagrams for mirrors.
- 🔴 The focal point is the point where parallel rays converge after reflecting off a mirror, and the focal length is the distance between the mirror and this point.
- 🏹 When an object is placed outside the focal point, a real image is formed because the rays converge at a point behind the mirror.
- 🔄 The image formed is inverted relative to the object, with the magnification being greater than one, indicating an enlarged image.
- 🔑 The sign of the image distance (di) is used to determine the location of the image relative to the mirror, with positive values indicating the image is on the left side.
- 🌌 If the object is placed inside the focal point, an upright virtual image is formed, which is larger than the object and appears on the opposite side of the mirror.
- 📐 The center of curvature is located at twice the focal length from the mirror, and rays from the object to the center of curvature help in forming the image.
- 🔊 The video also covers convex mirrors, which always produce virtual, upright, and reduced images due to their negative focal length.
- 📚 Essential equations for mirrors and lenses include the mirror equation (1/f = 1/do + 1/di) and the magnification formula (m = hi/ho or m = -di/do).
- 👓 The power of a lens is calculated using the formula power = 1/f, measured in diopters, and requires converting focal lengths from centimeters to meters.
Q & A
What is the principal axis in the context of spherical mirrors?
-The principal axis is a horizontal line used as a reference in ray diagrams for spherical mirrors. It passes through the focal point and the center of the mirror.
What is the focal length in relation to a concave mirror?
-The focal length is the distance between the focal point of a concave mirror and the mirror itself. It is a key parameter in determining the properties of the image formed by the mirror.
How is the height of the object (ho) defined in ray diagrams?
-The height of the object (ho) is the perpendicular distance from the top of the object to the principal axis in a ray diagram.
What is the difference between a real and a virtual image in the context of mirrors?
-A real image is formed where actual light rays converge, whereas a virtual image is formed where the extensions of the diverging light rays appear to meet. Real images can be projected onto a screen, but virtual images cannot.
How does the position of the object relative to the focal point affect the type of image formed by a concave mirror?
-If the object is placed outside the focal point, a real, inverted, and possibly enlarged image is formed. If the object is placed inside the focal point, an upright, enlarged, and virtual image is formed.
What is the significance of the radius of curvature in relation to a spherical mirror?
-The radius of curvature is twice the focal length for a spherical mirror. It is the distance from the mirror to the center of curvature, which is an important parameter in lens and mirror calculations.
What is the relationship between the magnification of an image and the height of the image (hi) compared to the height of the object (ho)?
-The magnification (M) is the absolute value of the ratio of the image height (hi) to the object height (ho), i.e., M = |hi/ho|. If M > 1, the image is enlarged; if M < 1, the image is reduced.
What is the mirror equation and how is it used?
-The mirror equation is 1/f = 1/do + 1/di, where f is the focal length, do is the object distance, and di is the image distance. It is used to calculate the position and properties of the image formed by a mirror or lens.
What is the difference between a convex and a concave mirror in terms of focal length and the type of image they produce?
-A concave mirror has a positive focal length and can produce both real and virtual images depending on the object's position. A convex mirror has a negative focal length and always produces a virtual, upright, and reduced image.
How is the power of a lens calculated and what unit is it measured in?
-The power of a lens is calculated using the formula power = 1/f, where f is the focal length in meters. It is measured in diopters, which is meters to the minus one (m⁻¹).
What are the key differences between a convergent and a divergent lens in terms of focal length and the type of image they produce?
-A convergent lens has a positive focal length and can produce both real and virtual images based on the object's position. A divergent lens has a negative focal length and always produces a virtual, upright, and reduced image.
Outlines
🔍 Understanding Ray Diagrams with Spherical Mirrors
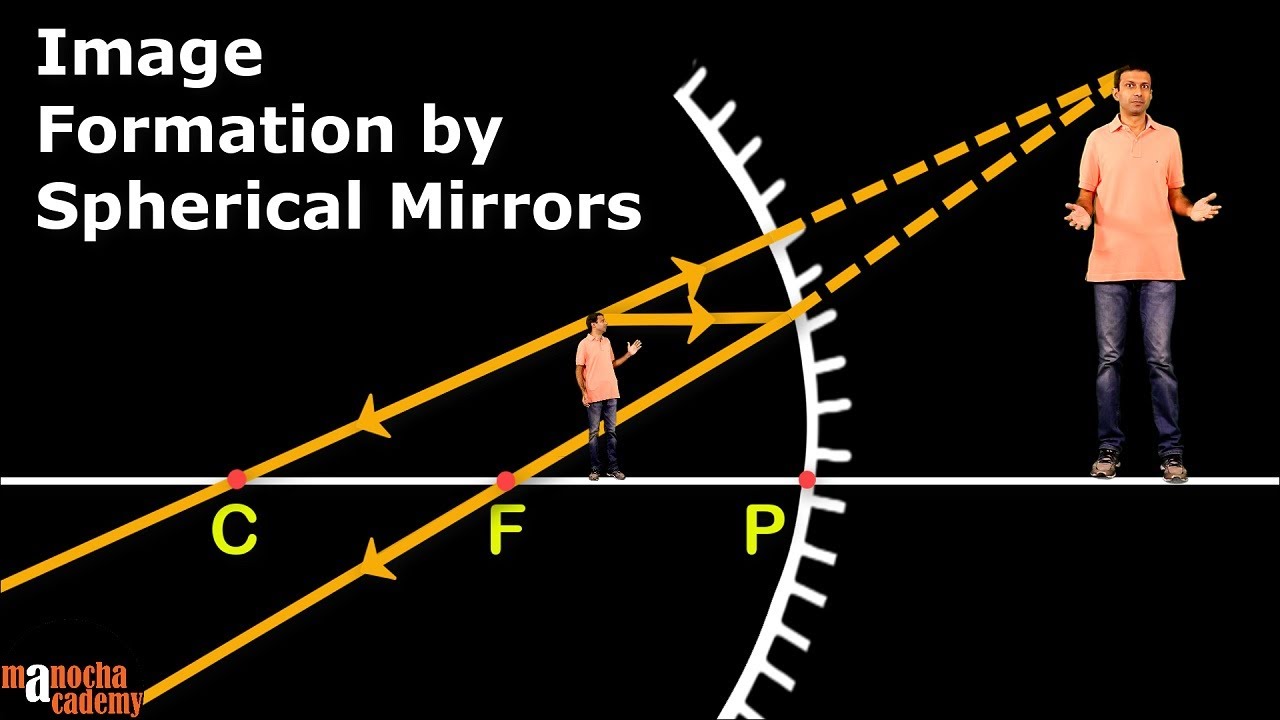
The first paragraph introduces the concept of ray diagrams, specifically focusing on spherical mirrors, particularly concave mirrors. It explains the principal axis, focal point, and focal length, and demonstrates how to draw an object outside the focal point to create a real image. The image formed is real, inverted, and enlarged, with the magnification being greater than one. The paragraph also discusses the sign conventions for distances and the relationship between the image and object heights, concluding with the characteristics of a virtual image formed when the object is placed within the focal point of a concave mirror.
📚 Exploring Mirror and Lens Equations
The second paragraph delves into the equations associated with mirrors and lenses. It starts with the mirror equation, which is applicable for both mirrors and lenses, and explains the significance of the focal length, whether positive for a concave mirror or negative for a convex mirror. The paragraph also covers the concept of magnification, power in diopters, and the relationship between image height and object height. It distinguishes between a convergent lens, which can produce both real and virtual images, and a divergent lens, which always produces a virtual image. The summary includes an example of a virtual image formed by a convex mirror and the characteristics of a real image formed by a convergent lens.
🎥 Practical Examples of Image Formation with Lenses
The final paragraph provides practical examples of image formation using lenses. It first discusses the use of a divergent lens to form a virtual, upright, and reduced image. Then, it contrasts this with a convergent lens, which, when the object is placed beyond the focal point, forms a real, inverted, and enlarged image. The paragraph concludes with a brief mention of how ray diagrams can be used to locate the image, summarizing the video's content on the principles of image formation with mirrors and lenses.
Mindmap
Keywords
💡Ray Diagrams
💡Spherical Mirror
💡Principal Axis
💡Focal Point
💡Focal Length
💡Real Image
💡Virtual Image
💡Magnification
💡Inverted Image
💡Convex Mirror
💡Lens
💡Mirror Equation
💡Power of a Lens
Highlights
Introduction to ray diagrams and their use in understanding spherical mirrors.
Explanation of the principal axis and focal point in the context of a concave mirror.
Description of the focal length and its measurement from the mirror to the focal point.
Placement of the object outside the focal point and its impact on the ray paths.
Ray tracing technique to determine the location of the image formed by a mirror.
Identification of a real image by the convergence of light rays.
Significance of the image distance (di) and its relation to the orientation of the image.
Characteristics of an inverted image and the calculation of magnification.
Difference between real and virtual images based on the direction of light convergence.
Placement of the object inside the focal point and its effect on image formation.
Concept of the center of curvature and its distance from the mirror.
Formation of an upright virtual image when the object is within the focal point.
Visual representation of light rays in virtual images and their apparent convergence.
Introduction to convex mirrors and their distinct focal point characteristics.
Behavior of light rays with convex mirrors and the formation of reduced virtual images.
Equations for mirrors and lenses, including the mirror equation and magnification formula.
Explanation of the power of a lens and its calculation in diopters.
Differentiation between convergent and divergent lenses based on focal length.
Ray diagram examples for divergent lenses and the resulting virtual, reduced, and upright images.
Ray diagram examples for convergent lenses and the conditions for real and virtual image formation.
Conclusion summarizing the use of ray diagrams for image location in mirrors and lenses.
Transcripts
Browse More Related Video

Concave Mirrors and Convex Mirrors Ray Diagram - Equations / Formulas & Practice Problems
Multiple Two Lens System with Diverging and Converging Lens
Convex and Concave Lenses
Thin Lens Equation Converging and Dverging Lens Ray Diagram & Sign Conventions
GCSE Physics - How to Draw Ray Diagrams #70
Spherical Mirrors
5.0 / 5 (0 votes)
Thanks for rating: