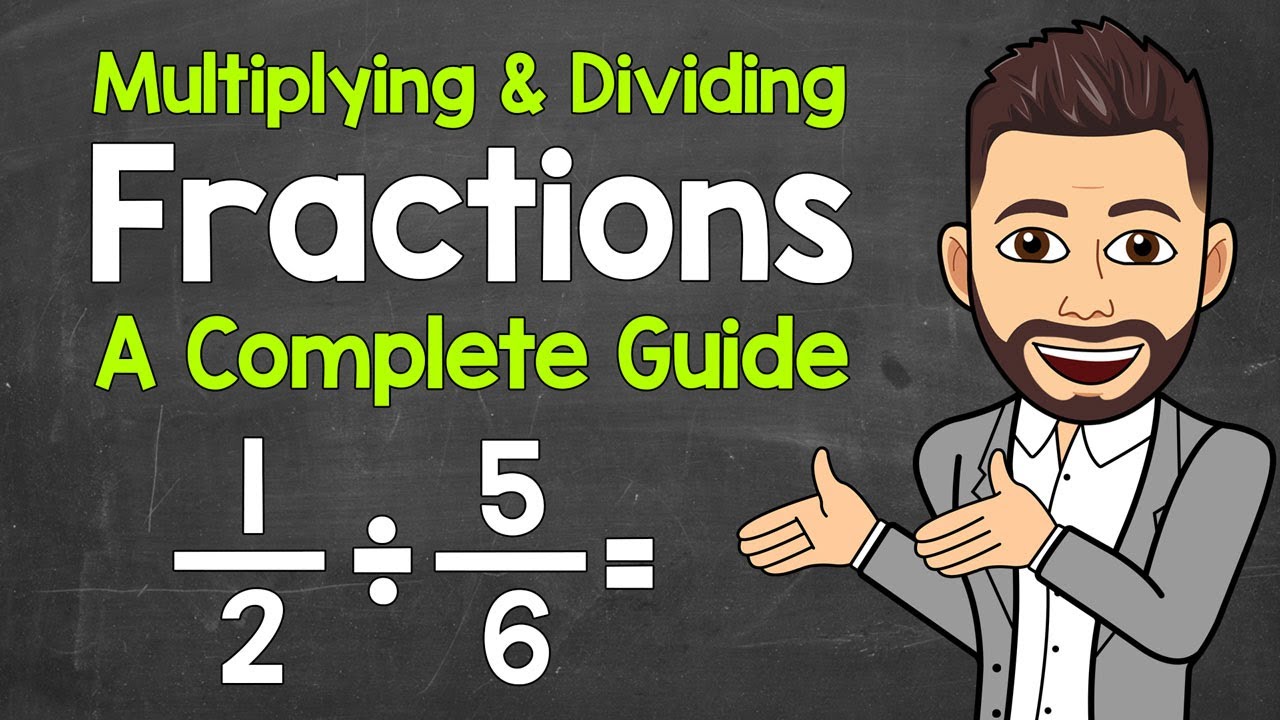
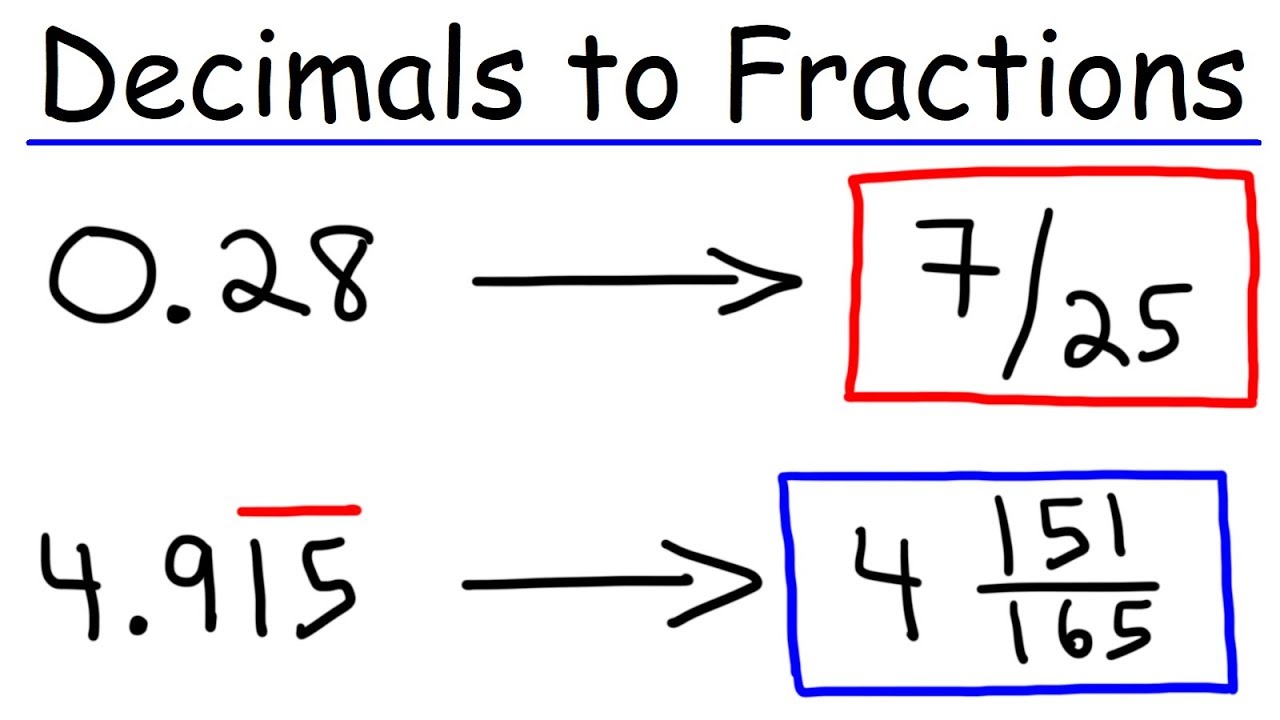
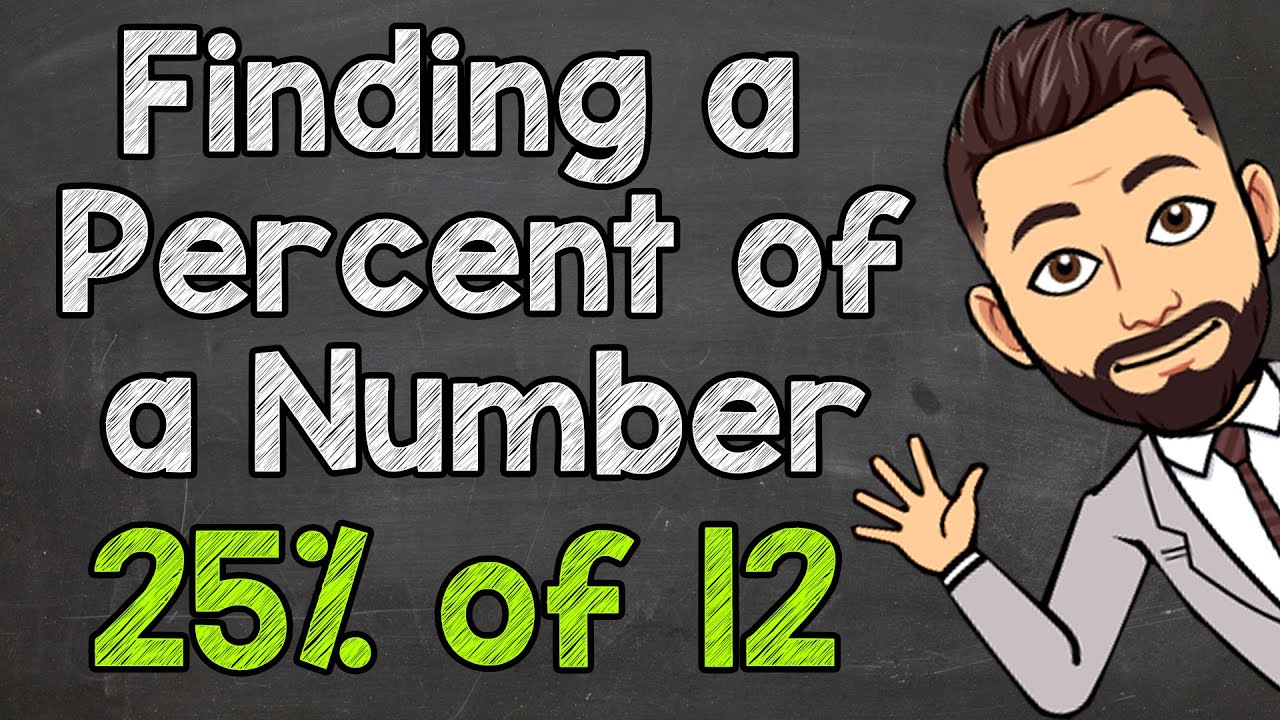
Understanding Fractions, Improper Fractions, and Mixed Numbers

Professor Dave Explains
8 Aug 201706:13
EducationalLearning
32 Likes 10 Comments

TLDRProfessor Dave delves into the concept of fractions, explaining them as parts of a whole derived from division. He starts with basics, differentiating between cases where dividends are larger or smaller than divisors, leading to whole numbers or fractions, respectively. Using a pizza analogy, Dave illustrates how fractions like one eighth or one fourth represent portions of a whole. He touches on equivalent fractions, comparing fractions with different denominators, and introduces improper fractions and mixed numbers. The video culminates in practical applications, such as calculating the number of pizzas needed for a party, demonstrating how to manipulate and convert fractions in everyday scenarios.

Takeaways
  • 😀 Fractions represent pieces or portions of a whole unit, like slices of a pizza
  • 😊 You can compare fractions by drawing models and seeing which piece covers a larger area
  • 👍 Equivalent fractions represent the same value even if written differently
  • 😉 Improper fractions have a numerator larger than the denominator and can be converted to mixed numbers
  • 🧐 Mixed numbers contain both whole units and fractional pieces, like 3 and 3/4 pizza
  • 🤓 To order an exact number of pizzas, convert fractions of pizzas to total slices then full pizzas
  • 🥳 Multiply the whole number of pizzas by slices per pizza, then add extra slices
  • 😯 Fractions can be greater than 1 if the numerator is larger than the denominator
  • 🤔 Common denominators make comparing the relative sizes of fractions straightforward
  • 🤯 Various methods like models, decimals, or common denominators allow fraction comparison
Q & A

  • What are the three components needed to perform division?

    -The three components needed to perform division are a dividend, a divisor, and a quotient.

  • How is a fraction represented when the divisor is larger than the dividend?

    -When the divisor is larger than the dividend, the result is a fraction that has a value between zero and one, representing a piece or fraction of one.

  • What example is used to explain how fractions work?

    -A pizza cut into equal slices is used as an example to explain how fractions work.

  • What does one eighth of a pizza represent?

    -One eighth of a pizza represents one slice out of eight equal slices of the whole pizza.

  • How can fractions be equivalent, as illustrated with the pizza example?

    -Fractions can be equivalent if they represent the same portion of a whole in different ways, such as one fourth being the same as two eighths of a pizza.

  • What can be done to compare fractions with different denominators?

    -To compare fractions with different denominators, one can visually represent them, convert to decimal notation, or find the least common denominator.

  • What is an improper fraction, and can you give an example?

    -An improper fraction is when the numerator is larger than the denominator, indicating a value greater than one. An example is four thirds.

  • How are mixed numbers related to improper fractions?

    -Mixed numbers are another way to represent improper fractions by combining whole numbers with fractions, such as converting four thirds into one and one third.

  • How do you determine the number of whole pizzas to order based on the number of slices needed?

    -You calculate the total number of slices needed, divide by the number of slices per pizza to determine whole pizzas, and adjust for any additional slices to get a mixed number or improper fraction representation.

  • What mathematical operations are illustrated when converting between improper fractions and mixed numbers?

    -The operations illustrated include multiplication, division, and simplification of fractions to convert between improper fractions and mixed numbers.

Outlines
00:00
😀 Introducing Fractions

Paragraph 1 introduces the concept of fractions as the result of division when the quotient is not a whole number. It explains how fractions represent a part or fraction of one whole using the example of pizza slices. It discusses fractions less than one with different denominators and equivalent fractions.

05:04
😀 Checking Comprehension on Fractions

Paragraph 2 indicates it is time to check comprehension on fractions before moving on.

Mindmap
Keywords
💡fraction
A fraction represents a part of a whole. Fractions are important in understanding division problems where the dividend is not evenly divisible by the divisor, resulting in a quotient that is a fraction of a whole number. Fractions help represent the portions of a pizza being divided and eaten.
💡denominator
The denominator is the bottom number in a fraction. It represents the total number of equal parts something is divided into. For example, if a pizza is cut into 8 slices, each slice is 1/8 of the pizza and 8 is the denominator.
💡numerator
The numerator is the top number in a fraction. It represents the number of parts being considered. For example, if you eat 3 out of 8 slices of pizza, the numerator is 3.
💡equivalent
Equivalent fractions represent the same amount even if the numbers are different. For example, 1/4 and 2/8 are equivalent because they represent the same portion of the whole.
💡improper fraction
An improper fraction has a numerator larger than its denominator. For example, 4/3 is an improper fraction because the numerator is greater than the denominator. Improper fractions have a value greater than 1.
💡mixed number
A mixed number contains both a whole number and a fraction, such as 1 1/3. It combines an improper fraction with a whole number for a more readable form.
💡reduce
To reduce a fraction means to simplify it to lowest terms. For example, 2/8 reduces to 1/4 by dividing the numerator and denominator by 2.
💡least common denominator
The least common denominator (LCD) is the smallest denominator that all fractions being considered can be converted to, allowing for easy comparison.
💡decimal
A decimal represents a fraction with a denominator that is a power of 10. Decimals provide an exact representation of a fractional amount.
💡divide
Division splits a quantity into equal parts. Fractions represent the result when division does not result in a whole number.
Highlights

First significant research finding

Introduction of innovative methodology

Key conclusion and practical application

Transcripts

Browse More Related Video

Math Antics - Ratios And Rates

Multiplying and Dividing Fractions | A Complete Guide | Math with Mr. J

Understanding Fractions

Decimals to Fractions

(2 and 3/4) plus 1/2 times 5/3 = ? What’s the first thing you should be thinking in this problem?

How to Divide Fractions – 6th Grade Math

Rate This

5.0 / 5 (0 votes)

Thanks for rating: