Worked example: Taylor polynomial of derivative function | AP Calculus BC | Khan Academy

Khan Academy
13 Oct 201405:22
EducationalLearning
32 Likes 10 Comments

TLDRThis video script discusses using a second-degree Taylor polynomial to approximate the derivative of a function, g, at x=1. It explains the process of constructing the polynomial using given values of g and its derivatives at x=2, and then evaluates the approximation for g'(1), resulting in an approximate value of three.

Takeaways
  • 📚 The script discusses the application of a second-degree Taylor polynomial to approximate the derivative of a function at a specific point.
  • 🔍 The function g and its derivatives are given at x equals two, with g(2) = 3, g'(2) = 1, g''(2) = -1, and g'''(2) = 2.
  • 📈 The task is to approximate g'(1) using the second-degree Taylor polynomial centered at x = 2.
  • 📝 The general formula for a second-degree Taylor polynomial for a function f(x) centered at x = a is f(a) + f'(a)(x - a) + f''(a)(x - a)^2 / 2!.
  • 🔑 The approximation of g'(x) at x = 1 involves substituting the given values of g and its derivatives at x = 2 into the Taylor polynomial formula.
  • 🔍 The script emphasizes the importance of correctly identifying the function and its derivatives to be used in the approximation.
  • 📘 The script provides a step-by-step guide on how to set up and simplify the Taylor polynomial for the given function g.
  • 📊 The script simplifies the Taylor polynomial by substituting the values of g(2), g'(2), g''(2), and g'''(2) into the formula.
  • 🧩 The final simplified form of the approximation for g'(x) is g'(x) ≈ 1 - (x - 2) + (x - 2)^2.
  • 📌 The script concludes by evaluating the approximation at x = 1, resulting in g'(1) ≈ 3.
  • 🤓 The script encourages viewers to pause and attempt the problem themselves, fostering active learning and engagement.
Q & A

  • What is the given value of g(2) in the script?

    -The given value of g(2) is 3.

  • What is the value of the first derivative of g evaluated at x equals 2?

    -The first derivative of g, denoted as g'(2), is equal to 1.

  • What is the value of the second derivative of g evaluated at x equals 2?

    -The second derivative of g, denoted as g''(2), is negative one.

  • What is the value of the third derivative of g evaluated at x equals 2?

    -The third derivative of g, denoted as g'''(2), is 2.

  • What is the task the script is asking us to perform?

    -The task is to use the second degree Taylor polynomial, centered at x equals 2, to approximate g'(1), not g(1).

  • What is the general form of a second degree Taylor polynomial for a function f(x) centered at x equals a?

    -The general form is f(x) ≈ f(a) + f'(a) * (x - a) + f''(a) * (x - a)^2 / 2!.

  • How is the second degree Taylor polynomial for g'(x) derived in the script?

    -It is derived by using the given values of g'(2), g''(2), and g'''(2), and substituting them into the general form of the second degree Taylor polynomial for g'(x).

  • What is the simplified form of the second degree Taylor polynomial for g'(x) centered at x equals 2?

    -The simplified form is g'(x) ≈ 1 - (x - 2) + (x - 2)^2.

  • How is the approximation for g'(1) calculated in the script?

    -The approximation for g'(1) is calculated by substituting x = 1 into the simplified second degree Taylor polynomial for g'(x).

  • What is the final approximation for g'(1) obtained in the script?

    -The final approximation for g'(1) is 3.

  • What is the significance of using a second degree Taylor polynomial for approximation?

    -The second degree Taylor polynomial provides a closer approximation to the function's value and its derivatives than a linear approximation, especially when the function has a curvature around the point of interest.

Outlines
00:00
📚 Deriving the Second Degree Taylor Polynomial for Function Approximation

The script introduces a mathematical problem involving the function g and its derivatives at x equals two. Given the values of g(2), g'(2), g''(2), and g'''(2), the task is to use the second degree Taylor polynomial, centered at x=2, to approximate g'(1). The script encourages viewers to attempt the problem before revealing the solution. The general formula for a second degree Taylor polynomial is provided, and then it's applied specifically to the function g'(x). By substituting the given values into the formula, the script derives the approximation for g'(1), which simplifies to a polynomial expression. The final evaluation of this polynomial at x=1 yields an approximation of g'(1) equal to 3.

05:01
🔍 Evaluating the Second Degree Taylor Series Approximation

This paragraph summarizes the process of using the second degree Taylor series approximation for g'(x), centered at x=2, to estimate the value of g'(1). The script confirms that the approximation was successfully calculated and evaluated, resulting in a value of 3 for g'(1). The paragraph concludes with a hope that the viewer found the mathematical exploration enjoyable, emphasizing the educational and engaging aspect of the content.

Mindmap
Keywords
💡Function g
In the context of the video, 'Function g' refers to a mathematical function for which various properties are being discussed. It is the central object of study in the video, with its values and derivatives at a specific point being the focus. The script mentions 'g of two is equal to three,' indicating the function's value at x=2, which is a key piece of information for constructing the Taylor polynomial.
💡Derivative
The derivative of a function represents the rate of change of the function's output with respect to its input. In the video, the derivative of 'Function g' at x=2 is given as 'G prime of two is equal to one,' which is essential for the Taylor polynomial approximation. Derivatives play a crucial role in understanding the local behavior of the function around a given point.
💡Second Derivative
The second derivative of a function is the derivative of the first derivative, providing information about the function's concavity and rate of change of the slope. In the script, 'The second derivative of g evaluated two is negative one,' which is used in the second degree Taylor polynomial to capture the curvature of the function at x=2.
💡Third Derivative
The third derivative is the derivative of the second derivative and gives information about the function's higher-order changes in curvature. The script states 'The third derivative of g evaluated at two is two,' which is used to complete the second degree Taylor polynomial for 'Function g'.
💡Taylor Polynomial
A Taylor polynomial is an approximation of a function near a given point using the derivatives of the function at that point. The script discusses using a 'second degree Taylor polynomial, centered at x equals two' to approximate 'g prime of one,' illustrating the application of Taylor polynomials in approximating function values.
💡Second Degree
The term 'second degree' in the context of the Taylor polynomial refers to the polynomial's order, which is determined by the highest power of (x - a) used in the polynomial, where 'a' is the center point. The script mentions 'second degree place, because it's x minus two squared,' indicating the polynomial includes terms up to (x - 2)^2.
💡Centered at x equals two
In the script, 'centered at x equals two' refers to the point around which the Taylor polynomial is constructed. This is the reference point for the approximation, and all derivatives used in the polynomial are evaluated at this point. The script uses this concept to explain the construction of the approximation for 'g prime of one'.
💡Approximation
Approximation in this video refers to the process of estimating a function's value or behavior using a simpler mathematical expression, such as a Taylor polynomial. The script's main task is to 'use the second degree Taylor polynomial to approximate g prime of one,' which is the goal of the mathematical process described.
💡Two Factorial
In mathematics, 'two factorial' (written as 2!) is the product of all positive integers up to 2, which is 2 * 1 = 2. The script mentions 'two factorials is, two times one or it's just two,' which is used in the denominator of the second term of the Taylor polynomial formula.
💡G Prime of One
The term 'g prime of one' refers to the derivative of the function 'g' evaluated at x=1. The script's ultimate goal is to approximate this value using the second degree Taylor polynomial centered at x=2. The script concludes with an approximation of 'g prime of one is going to be approximately equal to three' using the constructed polynomial.
Highlights

Introduction of a task to approximate g'(1) using the second degree Taylor polynomial centered at x=2.

Given values: g(2) = 3, g'(2) = 1, g''(2) = -1, and g'''(2) = 2.

Explanation of the general form of a second degree Taylor polynomial for a function f(x).

The formula for the second degree Taylor polynomial is f(x) ≈ f(2) + f'(2) * (x - 2) + f''(2) * (x - 2)^2 / 2!.

Adapting the formula to approximate g'(x) instead of f(x).

The second derivative of g'(x) is the third derivative of g(x), which is g'''(2).

Substituting the given values into the formula to find the approximation for g'(x).

Simplification of the approximation formula to g'(x) ≈ 1 - (x - 2) + (x - 2)^2.

Evaluating the approximation at x = 1 to find g'(1).

The calculation process for g'(1) using the approximation formula.

Result of the approximation: g'(1) ≈ 3.

Emphasis on the use of Taylor series for approximation in calculus.

The practical application of the second degree Taylor series in approximating derivatives.

The importance of understanding the derivatives of the function being approximated.

The method of evaluating the approximation at a specific point, in this case x = 1.

The final approximation result is consistent with the given g'(2) value.

Encouragement for viewers to pause and attempt the problem themselves before the solution is presented.

The educational approach of providing a step-by-step guide through the problem-solving process.

Transcripts

Browse More Related Video

2023 AP Calculus BC FRQ #6

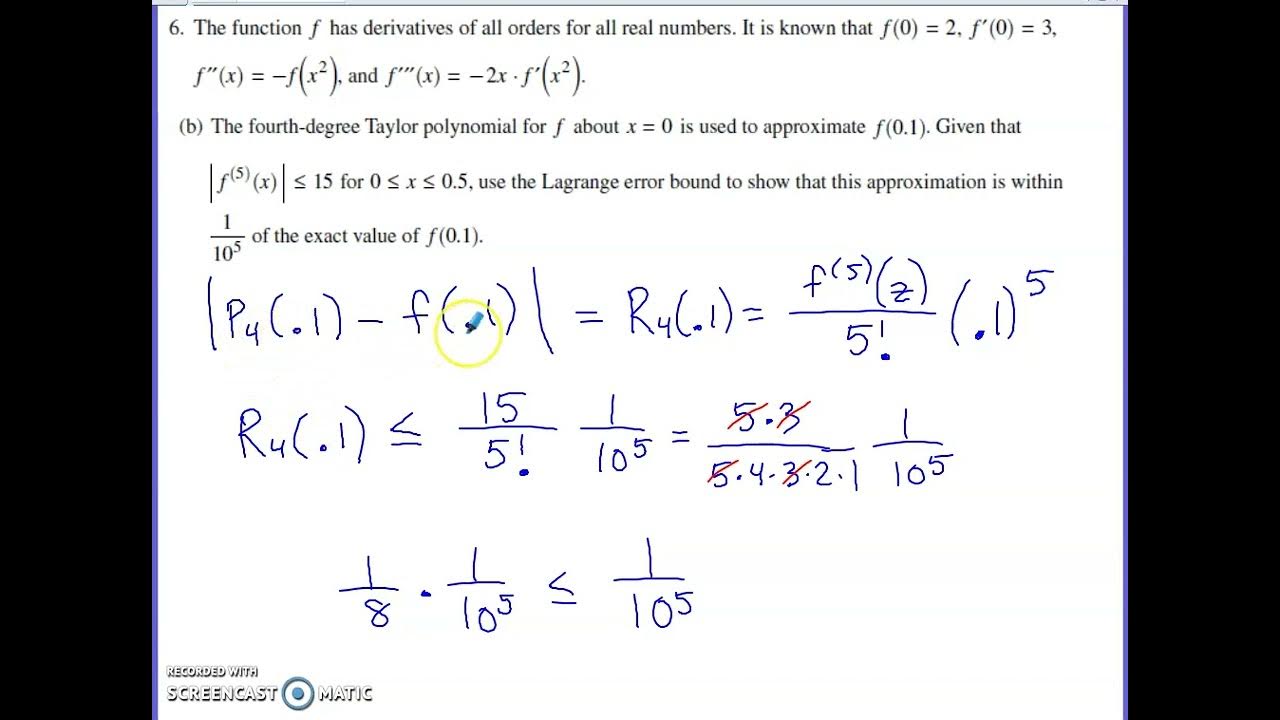
2023 AP Calculus BC Free Response Question #6

Avon High School - AP Calculus BC - Topic 10.12 - Example 2

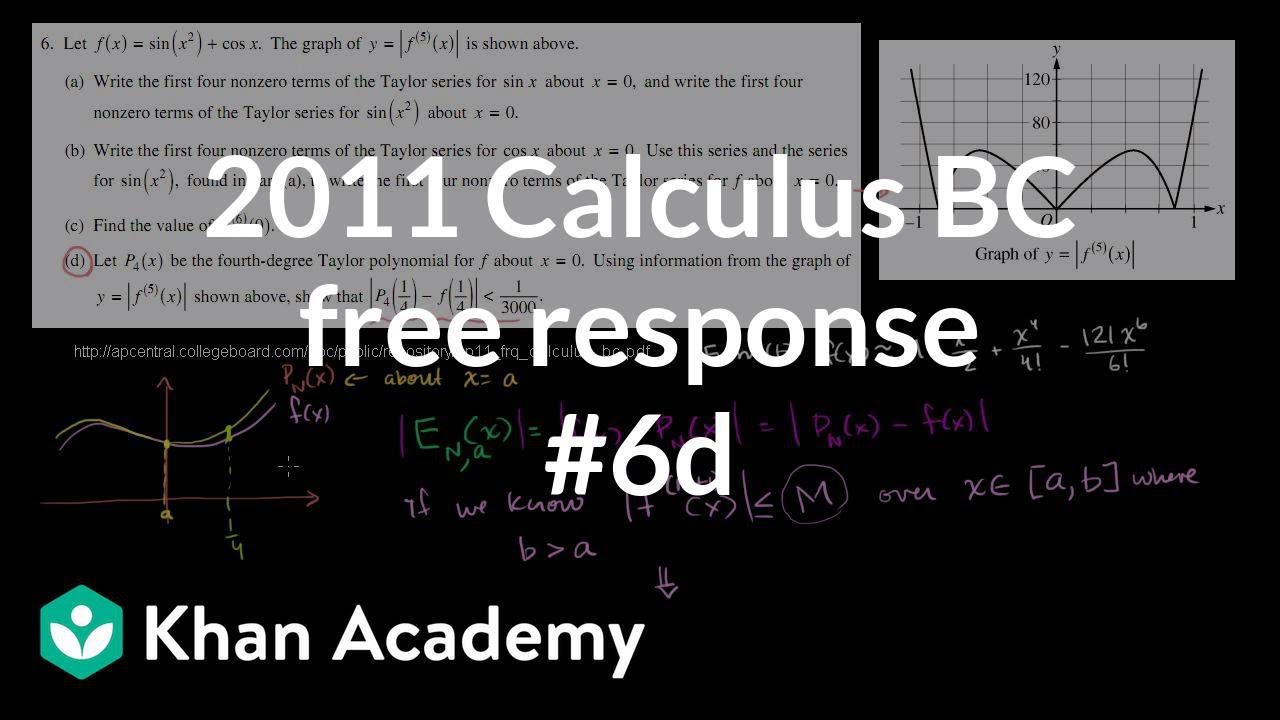
2011 Calculus BC free response #6d | AP Calculus BC | Khan Academy

2011 Calculus BC free response #6b | AP Calculus BC | Khan Academy

Analyzing concavity (algebraic) | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Taylor SeriesDerivative ApproximationMathematicsCalculusFunction AnalysisPolynomialsEducational ContentLearning ToolMath TutorialDerivative Functions