2011 Calculus BC free response #6d | AP Calculus BC | Khan Academy
TLDRThe video script discusses the concept of approximating functions using Taylor polynomials, specifically focusing on a fourth-degree Taylor polynomial centered at x equals 0. It explains how to bound the error of the approximation at x equals 1/4 using the properties of the function's fifth derivative. By applying the bound, the video demonstrates that the absolute value of the difference between the polynomial and the function at 1/4 is less than 1/3,000, showcasing the effectiveness of Taylor approximations.
Takeaways
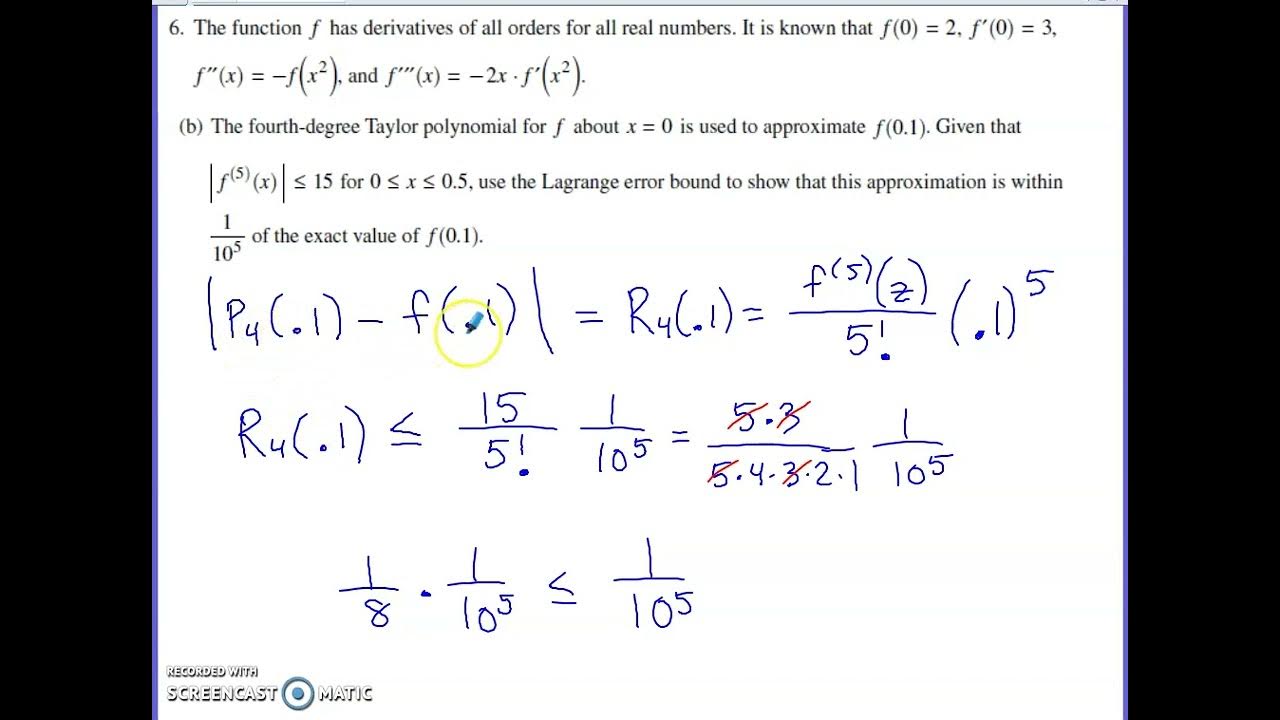
- 📚 The problem involves using a fourth degree Taylor polynomial, P_sub_4(x), to approximate a function f(x) at x=1/4.
- 🔢 The goal is to show that the absolute value of the difference between the polynomial approximation and the actual function at x=1/4 is less than 1/3000.
- 📈 The script provides a graph of the absolute value of the fifth derivative of f(x), which is crucial for bounding the error.
- 🌟 The key concept is that the error of an n-th degree polynomial approximation can be bounded using the (n+1)-th derivative of the function.
- 📊 The error function, or remainder, is the difference between the actual function f(x) and the polynomial approximation P(x).
- 🔍 The error is bounded by M*(x-a)^(n+1)/(n+1)!, where M is the maximum value of the (n+1)-th derivative over the interval [a, b].
- 🎯 In this case, a=0 and b=1/4, and the fifth derivative of f(x) is shown to be less than or equal to 40 over the interval [0, 1/4].
- 🧮 The calculation involves finding the value of the error at x=1/4, which is less than 1/3000 based on the given information.
- 📝 The script emphasizes the importance of knowing the properties of Taylor approximations and their bounded errors for solving such problems.
- 🏆 The problem is a good example of applying Taylor series approximations and understanding their error bounds, which is useful in calculus.
- 💡 The script suggests that knowing the boundedness of the error remainder is crucial for solving this problem efficiently, especially in the context of an exam.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the application of Taylor polynomials for approximating functions and bounding the error of such approximations.
What is the degree of the Taylor polynomial discussed in the script?
-The degree of the Taylor polynomial discussed in the script is four.
What is the significance of the absolute value of the fifth derivative in the problem?
-The absolute value of the fifth derivative is significant because it is used to determine the bound on the error of the function approximation at x equals 1/4.
How does the script visualize the concept of bounding the error?
-The script visualizes the concept of bounding the error by drawing a graph to represent the function and the polynomial approximation, and then showing the absolute value of the difference between the two at x equals 1/4.
What is the general formula used to bound the error of a Taylor polynomial approximation?
-The general formula used to bound the error of a Taylor polynomial approximation is (M * (x - a)^(n+1)) / (n+1)! where M is the maximum value of the (n+1)th derivative over the interval, and a and x are points within that interval.
What is the role of the (n+1)th derivative in the Taylor polynomial approximation?
-The (n+1)th derivative plays a crucial role in determining the error bound of the Taylor polynomial approximation. It provides the maximum value used in the error bounding formula.
How does the script determine the value of M for the given problem?
-The script determines the value of M by observing the graph of the absolute value of the fifth derivative and noting that it is definitely less than 40 over the interval between 0 and 1/4.
What is the interval [a, b] mentioned in the script?
-In the context of the script, the interval [a, b] is used to define the range over which the (n+1)th derivative of the function is bounded. For the specific problem discussed, a is 0 and b is 1/4.
What is the final bound on the error found in the script?
-The final bound on the error found in the script is less than 1 over 3,000.
How does the script emphasize the importance of knowing the properties of the (n+1)th derivative?
-The script emphasizes the importance of knowing the properties of the (n+1)th derivative because it is essential for bounding the error in the Taylor polynomial approximation, which is a key aspect of the problem being solved.
What advice does the script give for understanding the proof of the error bounding formula?
-The script advises viewers to watch another video from the Khan Academy Calculus playlist that covers the proof of the error bounding formula from first principles.
Outlines
📈 Error Bound of Taylor Polynomial
The narrative in paragraph1 centers on determining the error bound of a fourth-degree Taylor polynomial approximation of a function f at x=1/4. It introduces the problem by referencing a graph of the absolute value of the fifth derivative of f. The speaker then explains the concept of bounding the error between the polynomial and the function at x=1/4, using a graphical explanation to illustrate the polynomial's behavior around the origin. The paragraph transitions into a general discussion on bounding the error of Taylor polynomial approximations, referencing the speaker's previous videos that delve into the theoretical underpinnings of these concepts.
🔍 Applying Error Bound Theory
Paragraph2 elaborates on the specific task of bounding the error for the fourth-degree Taylor polynomial. It begins by recalling the polynomial's formula obtained in an earlier part of the discourse and then connects this to the concept of bounding the error using a maximum value M derived from the fifth derivative of f. The speaker calculates the bound for the error at x=1/4, using a hypothetical maximum value M and the principles discussed previously, ultimately showing that this error is less than 1 over 3,072, and hence less than the required 1 over 3,000.
🔢 Calculating Specific Error Bound
In paragraph3, the focus shifts to the detailed calculation and simplification of the error bound established in the previous paragraph. The speaker meticulously walks through the mathematical process of dividing terms to simplify the expression, eventually deriving that the error at x=1/4 is less than 1 over 3,072. This is concluded to be less than 1 over 3,000, satisfying the problem's requirement and confirming the polynomial’s approximation accuracy at the specified point.
Mindmap
Keywords
💡Taylor Polynomial
💡Absolute Value
💡Derivative
💡Error Function
💡Bounding
💡Remainder
💡Degree of Polynomial
💡Interval
💡Factorial
💡Graph
💡Approximation
Highlights
The problem involves using a fourth degree Taylor polynomial to approximate a function around x equals 0.
The absolute value of the difference between the polynomial and the function evaluated at 1/4 is to be determined.
A graph of the function and the polynomial can help visualize the approximation error.
The error of the function approximation at x equals 1/4 is the focus of the problem.
The general method to bound the error involves knowing properties of the (n+1)th derivative of the function.
For a fourth degree polynomial approximation, the error at any x is related to the fifth derivative of the function.
The error can be bounded if the (n+1)th derivative is less than or equal to a maximum value over a certain interval.
The Taylor polynomial for f, p sub 4 of x, is given as 1 plus x squared over 2 plus x to the fourth over 4.
The error at 1/4 is to be bounded using the given information about the fifth derivative of f.
The graph of the absolute value of the fifth derivative of f is provided to help find the maximum value over the interval from 0 to 1/4.
The maximum value of the fifth derivative is estimated to be less than 40 over the interval from 0 to 1/4.
The error at 1/4 is calculated to be less than or equal to 40 times 1/4 to the fifth power over 5 factorial.
Simplifying the expression, the error at 1/4 is found to be less than 1 over 3,072.
The problem statement asks to show that the error at 1/4 is less than one over 3,000.
The calculated error of less than 1 over 3,072 is indeed less than 1 over 3,000, satisfying the problem's requirement.
The solution process emphasizes the importance of understanding the properties of derivatives for polynomial approximations.
The problem demonstrates the practical application of Taylor polynomials in approximating functions and bounding errors.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: