2023 AP Calculus BC FRQ #6

turksvids
10 May 202306:40
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses a problem from the 2023 AP Calculus BC Exam, focusing on a series problem involving a function f with derivatives of all orders. The task is to find the fourth derivative of f and write the fourth degree Taylor polynomial at x equals zero. The video provides a step-by-step approach to calculate the derivatives, starting with the given information about f(0), f'(0), and higher derivatives. It then uses the product rule to find the fourth derivative, which is shown to be -6 when evaluated at zero. The Taylor polynomial is constructed using the known derivatives, resulting in a simplified polynomial of 2 + 3x - x^2 - (1/4)x^4. Part B of the problem involves using the fourth degree polynomial to approximate f(0.1), with an error bound provided by the LaGrange error bound. The video demonstrates that the approximation error is within the specified tolerance. Finally, the script introduces a new function G and derives its second degree Taylor polynomial around x equals zero, using the given information about G and its derivative. The video concludes by simplifying the polynomial for G.

Takeaways
  • 📚 The video discusses problem number six from the 2023 AP Calculus BC Exam, which is a series problem.
  • 🔍 The function f has derivatives of all orders for all real numbers, with specific values given for f(0), f'(0), f''(x), and f'''(x).
  • 🌟 Part A requires finding the fourth derivative of f and writing the fourth degree Taylor polynomial at x=0, showing all work.
  • ✅ The fourth derivative of f at zero is found to be -6 by applying the product rule and evaluating at x=0.
  • 📝 The fourth degree Taylor polynomial for f at x=0 is constructed using the known derivatives and is simplified to 2 + 3x - x^2 - (1/4)x^4.
  • 🔢 Part B involves using the fourth degree polynomial to approximate f(0.1) and applying the LaGrange error bound with a maximum fifth derivative value of 15.
  • 📉 The approximation error is shown to be less than 1/10^5 of the exact value, ensuring the approximation is within the specified tolerance.
  • 🆕 A new function G is introduced in Part C, with g(0) given and g'(x) defined as e^x * f(x).
  • 🔧 The second degree Taylor polynomial for G at x=0 is found by calculating G'(0) and G''(0), then applying the Taylor series formula.
  • 📐 The polynomial for G simplifies to 4 + 2x + (5/2)x^2, which is derived from the given function and its derivatives.
  • 💡 The video provides a comprehensive walkthrough of a series problem from the AP Calculus BC Exam, emphasizing the importance of understanding derivatives and Taylor polynomials.
  • ⚙️ The process of finding higher-order derivatives, constructing Taylor polynomials, and applying error bounds is clearly explained, making the video a useful resource for exam preparation.
Q & A

  • What is the main topic of the video?

    -The video focuses on solving problem number six from the 2023 AP Calculus BC Exam, which is a series problem.

  • What is the first step in finding the fourth derivative of the function f?

    -The first step is to apply the product rule using the known third derivative, which involves differentiating the first part and multiplying by the second part, and vice versa.

  • What is the value of the fourth derivative of f at x equals zero?

    -The fourth derivative of f at x equals zero is -6, which is found by evaluating the expression f'(0) * (-2), given that f'(0) is 3.

  • How is the fourth degree Taylor polynomial for f at x equals zero written?

    -The fourth degree Taylor polynomial is written as f(x) ≈ 2 + 3x - x^2 - (1/4)x^4, where the coefficients are derived from the function's derivatives evaluated at x=0.

  • What is the purpose of using the LaGrange error bound in Part B of the problem?

    -The LaGrange error bound is used to show that the approximation of f(0.1) using the fourth degree polynomial is within a certain error margin, given the maximum value of the fifth derivative on the interval [0, 0.1].

  • How is the error in the approximation of f(0.1) using the fourth degree polynomial calculated?

    -The error is calculated using the formula for the LaGrange error bound, which involves the maximum value of the absolute fifth derivative (M), the interval length raised to the power of the degree of the polynomial plus one, and divided by the factorial of that degree.

  • What is the maximum value given for the absolute fifth derivative in the LaGrange error bound?

    -The maximum value given for the absolute fifth derivative is 15.

  • What is the function G defined as in Part C of the problem?

    -The function G is defined such that G(0) is 4 and G'(x) is e^x * f(x).

  • What is the value of G'(0) in the function G?

    -The value of G'(0) is 2, which is calculated as e^0 * f(0), given that f(0) is 2.

  • How is the second derivative of G, denoted as G''(x), found?

    -G''(x) is found using the product rule, which involves the first derivative of the second part plus the second derivative of the first part, evaluated at x=0.

  • What is the second degree Taylor polynomial for G at x equals zero?

    -The second degree Taylor polynomial for G at x equals zero is G(x) ≈ 4 + 2x + (5/2)x^2, which is derived from the function's derivatives evaluated at x=0.

Outlines
00:00
📚 Calculus Series Problem Overview

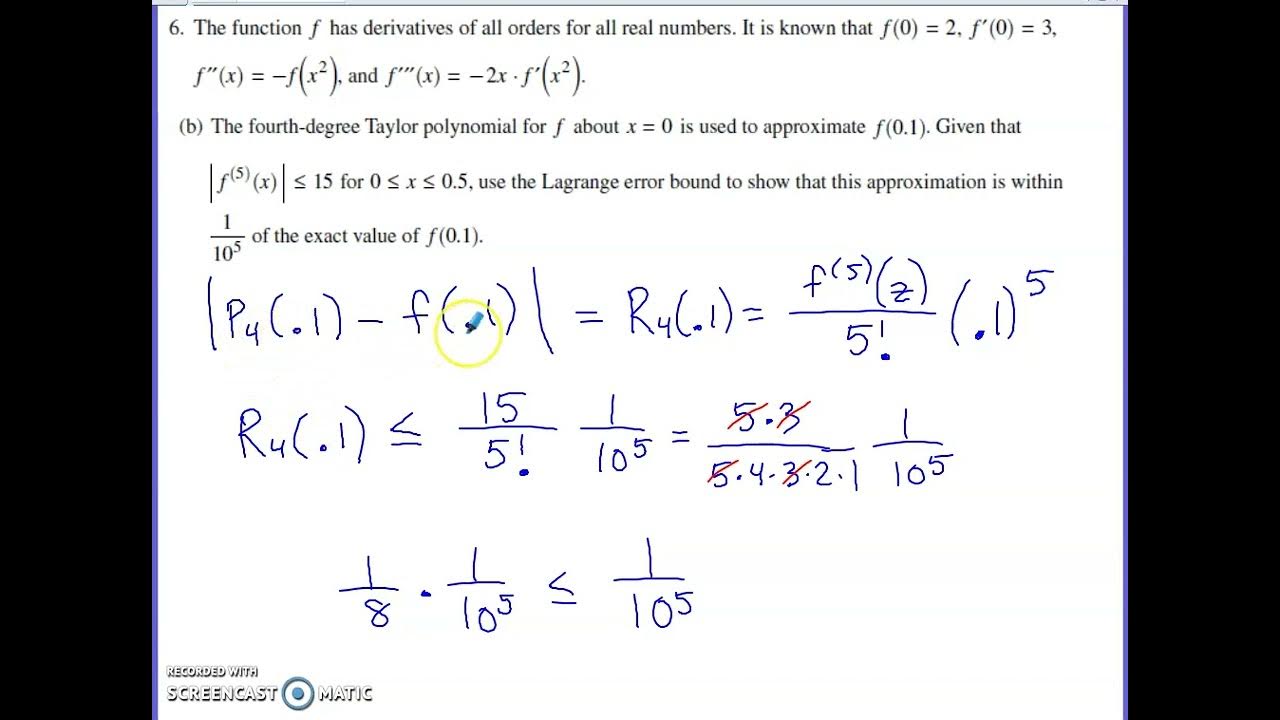
This paragraph introduces a series problem from the 2023 AP Calculus BC Exam. The focus is on a function 'f' with derivatives of all orders for real numbers. Key known values of the function and its derivatives at x=0 are provided, including F(0)=2, F'(0)=3, F''(x)=-F(x^2), and F'''(x)=-2x*F'(x^2). The task involves finding the fourth derivative, writing the fourth degree Taylor polynomial for F at x=0, and using the LaGrange error bound to show the approximation error for a given interval.

05:02
🔍 Deriving the Fourth Derivative and Taylor Polynomial

The paragraph outlines the process of finding the fourth derivative of the function 'f' using the product rule and the known third derivative. It then proceeds to construct the fourth degree Taylor polynomial at x=0, incorporating the values of the function and its first three derivatives at that point. The polynomial is simplified to a more manageable form for further use.

🔢 LaGrange Error Bound Application

This section deals with Part B of the problem, which involves using the fourth degree Taylor polynomial to approximate the value of F at x=0.1. It employs the LaGrange error bound to demonstrate that the approximation is within a certain tolerance, given the constraint on the absolute value of the fifth derivative. The calculation involves finding the maximum value of the fifth derivative over the interval [0, 0.1] and using it to bound the error of the approximation.

🎓 Constructing the Taylor Polynomial for a Related Function G

The final paragraph introduces a new function 'G' with given values for G(0) and G'(x), and the task is to find the second degree Taylor polynomial for G about x=0. It involves calculating G'(0) and G''(0), then using these values to formulate the polynomial. The paragraph concludes with the simplified form of the Taylor polynomial for G.

Mindmap
Keywords
💡AP Calculus BC Exam
AP Calculus BC Exam is a standardized test offered by the College Board that covers advanced topics in calculus. It is a significant part of the video's theme as the entire discussion revolves around solving a problem from this exam. In the script, the video focuses on the series problem from the 2023 edition of the exam.
💡Derivatives
Derivatives in calculus represent the rate at which a function changes at a certain point. They are fundamental to the video's content, as the problem involves finding and using various orders of derivatives to construct a Taylor polynomial. The script mentions the first, second, third, and fourth derivatives of the function f(x).
💡Taylor Polynomial
A Taylor polynomial is a representation of a function as an infinite sum of terms calculated from the values of its derivatives at a single point. It is central to the video's narrative, as the task is to write the fourth degree Taylor polynomial for a given function at x equals zero. The script details the process of finding this polynomial using known derivatives of the function.
💡Product Rule
The product rule is a fundamental theorem in calculus used to find the derivative of a product of two functions. It is used in the video to find the fourth derivative of the function f(x). The script illustrates the application of the product rule when differentiating the expression involving f(x) and x.
💡Chain Rule
The chain rule is a method for finding the derivative of a composite function. It is mentioned in the script when discussing the derivative of f(x) squared, which is a composite of f(x) and the square function. The chain rule is essential for correctly differentiating such expressions in the context of the video.
💡Lagrange Error Bound
The Lagrange error bound provides an estimate of the error between the actual value of a function and its polynomial approximation within a given interval. It is used in the video to show that the approximation of the function f at x equals 0.1 is within a certain tolerance. The script calculates the error bound using the maximum value of the fifth derivative over the interval from 0 to 0.1.
💡Function Evaluation
Function evaluation is the process of determining the value of a function at a specific point. It is a key concept in the video, as the task involves evaluating the function f(x) and its derivatives at x equals zero. The script provides specific values for f(0), f'(0), and higher derivatives at this point.
💡Factorial
Factorial, denoted by 'n!', is the product of all positive integers less than or equal to n. It is used in the video when writing out the terms of the Taylor polynomial, where factorials appear in the denominators of the coefficients. The script includes factorials in the calculation of the coefficients for the polynomial terms.
💡Series
A series in mathematics is the sum of the terms of a sequence. In the context of the video, the series problem involves working with Taylor series, which is a representation of a function as an infinite sum of its derivatives at a point. The script discusses the series in relation to the Taylor polynomial approximation of the function f.
💡Function Approximation
Function approximation is the process of finding a simpler function that behaves similarly to a more complex one, often for the sake of easier calculation or analysis. The video's main theme involves approximating the function f using its Taylor polynomial. The script demonstrates how to approximate f at x equals 0.1 using the fourth degree polynomial.
💡e to the x
e to the x, where e is the base of the natural logarithm, is a common mathematical function that appears in the video as part of the definition of a new function g(x). The script introduces g(x) = e^x * f(x), which is used to derive the second degree Taylor polynomial for g about x equals zero.
Highlights

The video discusses problem number six from the 2023 AP Calculus BC Exam, which is a series problem.

Function f has derivatives of all orders for all real numbers.

Given that F(0) is 2, F'(0) is 3, and F''(x) is negative f(x) squared.

The third derivative F''' is negative two x times F'(x) squared.

Part A requires finding the fourth derivative and writing the fourth degree Taylor polynomial for F at x=0.

The fourth derivative is found using the product rule and evaluated at zero to get -6.

The fourth degree Taylor polynomial for F at x=0 is approximated as 2 + 3x - x^2 - (1/4)x^4.

Part B involves approximating F(0.1) using the fourth degree polynomial and the LaGrange error bound.

The absolute value of the error is shown to be less than 1/10^5 of the exact value using the fifth derivative's maximum value of 15.

The LaGrange error bound is simplified to demonstrate the approximation's accuracy.

A new function G is introduced with g(0) = 4 and G'(x) = e^x * F(x).

The second degree Taylor polynomial for G about x=0 is derived using given values and product rule.

G'(0) is calculated to be 2, and G''(0) is found to be 5.

The second degree Taylor polynomial for G at x=0 is written as 4 + 2x + (5/2)x^2.

The video provides a comprehensive walkthrough of calculus problems, including the application of Taylor polynomials and error bounds.

The presenter emphasizes the importance of showing work and understanding the underlying calculus concepts.

The video concludes with a positive note, wishing viewers good luck in their calculus endeavors.

Transcripts

Browse More Related Video

2023 AP Calculus BC Free Response Question #6

2011 Calculus BC free response #6d | AP Calculus BC | Khan Academy

The Lagrange Error Bound for Taylor Polynomials

Worked example: Taylor polynomial of derivative function | AP Calculus BC | Khan Academy

2006 AP Calculus AB Free Response #6

Avon High School - AP Calculus BC - Topic 10.12 - Example 3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusSeries ProblemTaylor PolynomialError BoundDerivativesMathematicsExam PrepEducational ContentProduct RuleLaGrangeCalculus Tutorial