Math 14 HW 6.2.27-T Find the percentage of men meeting the height requirement.

Fiorentino Siciliano
5 Oct 202214:23
EducationalLearning
32 Likes 10 Comments

TLDRThe script discusses a survey on gender height distributions, focusing on amusement park character height requirements. It calculates the percentage of men within the 57-63 inch range, finding only 4.11% meet the criteria, suggesting most characters are likely female. It then explores new height requirements excluding the tallest 50% and shortest 5% of men, determining the range to be 63.4 to 70.1 inches.

Takeaways
  • 📊 A survey reveals the heights of women are normally distributed with a mean of 64.2 inches and a standard deviation of 4.2 inches.
  • 🚹 The same survey indicates that men's heights are normally distributed with a mean of 70.1 inches and a standard deviation of 4.1 inches.
  • 🎢 The amusement park has height requirements for live characters, which are a minimum of 57 inches and a maximum of 63 inches.
  • 📈 The script involves sketching a normal distribution curve to visualize the area of interest for the height requirements.
  • 🔢 The process includes converting the given height requirements into Z-scores for men to find the percentage meeting the criteria.
  • ⚖️ Z-scores are calculated using the formula \( \frac{(X - \mu)}{\sigma} \), where \( X \) is the height, \( \mu \) is the mean, and \( \sigma \) is the standard deviation.
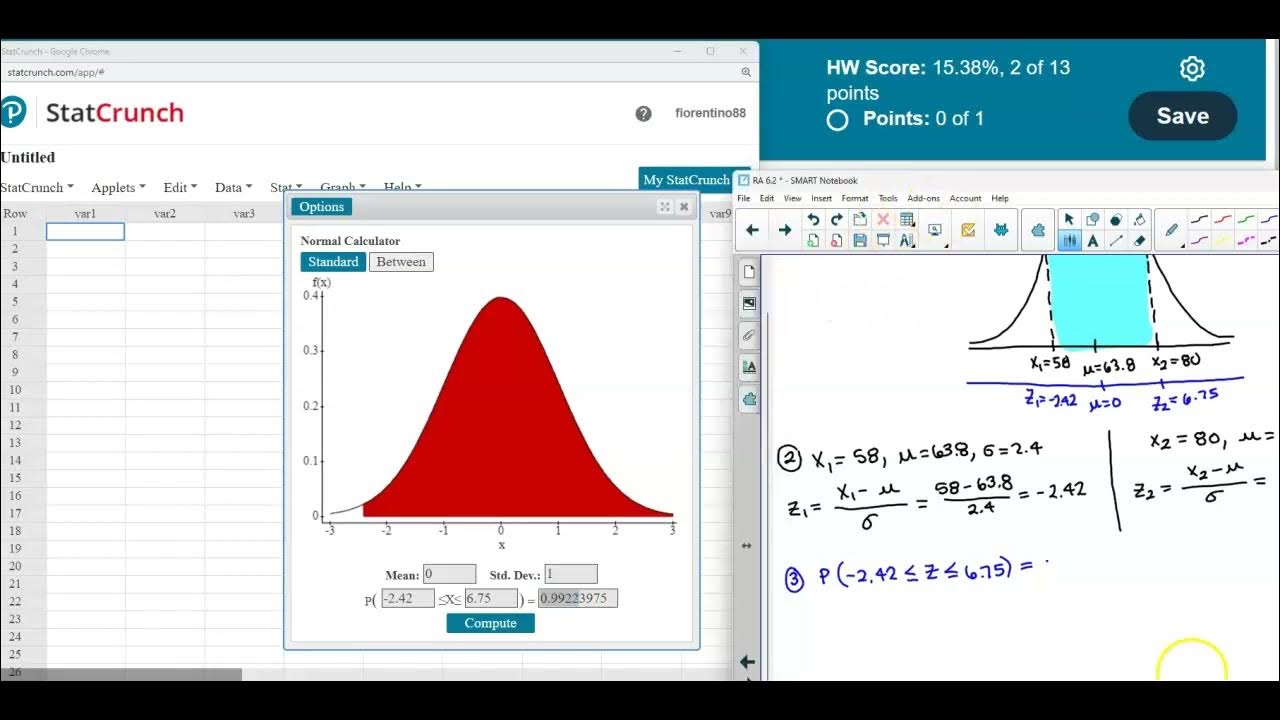
  • 📉 The Z-score for the lower height limit (57 inches) is approximately -3.20, indicating a significant deviation from the mean.
  • 📈 The Z-score for the upper height limit (63 inches) is approximately -1.73, which is closer to the mean than the lower limit.
  • 🔮 Using StatCrunch, the probability (or area under the curve) between these Z-scores is found to be 0.0411.
  • 👦 The percentage of men meeting the height requirement is 4.11%, suggesting that most characters at the amusement park are likely to be women.
  • 🆕 Part B of the script discusses changing the height requirements to exclude only the tallest 50% and shortest 5% of men.
  • 📏 The new height requirements are calculated to be a minimum of 63.4 inches and a maximum of 70.1 inches, excluding the extremes of the male height distribution.
Q & A

  • What is the mean height for women according to the survey?

    -The mean height for women is 64.2 inches.

  • What is the standard deviation for women's height in the survey?

    -The standard deviation for women's height is 4.2 inches.

  • What is the mean height for men according to the survey?

    -The mean height for men is 70.1 inches.

  • What is the standard deviation for men's height in the survey?

    -The standard deviation for men's height is 4.1 inches.

  • What are the minimum and maximum height requirements for live characters at the amusement park?

    -The minimum height requirement is 57 inches, and the maximum is 63 inches.

  • Why is it necessary to convert the height requirements into Z-scores?

    -Converting the height requirements into Z-scores allows us to find the corresponding probabilities on the standard normal distribution, which helps in determining the percentage of the population that meets the requirements.

  • What is the Z-score for the minimum height requirement of 57 inches for men?

    -The Z-score for the minimum height requirement of 57 inches is approximately -3.20.

  • What is the Z-score for the maximum height requirement of 63 inches for men?

    -The Z-score for the maximum height requirement of 63 inches is approximately -1.73.

  • What percentage of men meet the height requirement of the amusement park based on the survey?

    -Approximately 4.11 percent of men meet the height requirement of the amusement park.

  • What does the low percentage of men meeting the height requirement suggest about the gender composition of the characters at the amusement park?

    -The low percentage suggests that most of the characters are likely to be women, as they have a higher proportion of individuals within the specified height range.

  • If the height requirements are changed to exclude only the tallest 50 percent and the shortest five percent of men, what are the new height requirements?

    -The new height requirements would be a minimum of 63.4 inches and a maximum of 70.1 inches.

  • How are the new height requirements determined for the amusement park?

    -The new height requirements are determined by finding the Z-scores that correspond to the 5th percentile (shortest five percent) and the 50th percentile (tallest 50 percent) on the standard normal distribution, and then converting these Z-scores back to height measurements using the mean and standard deviation of men's height.

Outlines
00:00
📊 Statistical Analysis of Height Requirements

This paragraph discusses a survey's findings on the normal distribution of women's and men's heights, with specific mean and standard deviation values provided. It also addresses the height requirements for live characters at an amusement park, which range from a minimum of 57 inches to a maximum of 63 inches. The task involves calculating the percentage of men who meet these requirements by visualizing the data on a normal distribution curve, determining the mean, and converting the height requirements into z-scores. The process of finding the shaded area between the z-scores on the curve is explained, which represents the percentage of men fitting the height criteria.

05:02
🔢 Calculating the Probability of Meeting Height Requirements

The second paragraph continues the analysis by focusing on the probability calculation for men meeting the amusement park's height requirements using a statistical tool called StatCrunch. It details the steps to input the z-scores into the tool and compute the probability of the shaded area between the two z-scores, which corresponds to the height range of 57 to 63 inches. The result is a 4.11% probability, suggesting that most characters at the amusement park are likely to be women since the majority of men do not meet the height requirement. The paragraph also introduces a new scenario where the height requirements change to exclude only the tallest 50% and shortest 5% of men, prompting a new analysis for the revised height limits.

10:05
📐 Redefining Height Requirements for Amusement Park Characters

In the final paragraph, the script outlines the process of redefining the height requirements for amusement park characters by excluding the tallest 50% and shortest 5% of men. The normal distribution curve is sketched, and the new probabilities are marked on the curve. The task involves finding the z-scores corresponding to these probabilities and then converting them back to the original height scale using the mean height and standard deviation of men. The new height requirements are calculated to be a minimum of 63.4 inches and a maximum of 70.1 inches, providing a clear understanding of the revised criteria for character selection.

Mindmap
Keywords
💡Normal Distribution
Normal distribution, also known as Gaussian distribution, is a probability distribution that is characterized by a symmetrical bell-shaped curve. In the context of the video, it is used to describe the distribution of women's and men's heights. The video explains that both women and men's heights follow a normal distribution with different means and standard deviations, which is essential for understanding the percentage of individuals meeting certain height criteria.
💡Mean
The mean, often referred to as the average, is a measure of central tendency in statistics. It is calculated by adding up all the values in a data set and then dividing by the number of values. In the video, the mean height for women is given as 64.2 inches, and for men, it is 70.1 inches. These means are crucial for determining the center of the normal distribution curves for height.
💡Standard Deviation
Standard deviation is a measure that indicates the amount of variation or dispersion in a set of values. A low standard deviation indicates that the values tend to be close to the mean, while a high standard deviation indicates that the values are spread out over a wider range. In the script, the standard deviation for women's height is 4.2 inches, and for men, it is 4.1 inches, which helps in understanding the spread of height data around the mean.
💡Z-Score
A Z-score is a standard score that indicates how many standard deviations an element is from the mean. It is used to standardize the data points and compare them on the same scale. In the video, Z-scores are calculated for different height values to determine the proportion of the population that falls within specific height ranges, which is essential for analyzing the height requirements for amusement park characters.
💡Amusement Park
An amusement park is a recreational area with games, rides, and other entertainment attractions. In the video, the amusement park serves as a context for discussing height requirements for live characters. The script mentions specific minimum and maximum height requirements that characters must meet, which is a central theme of the video.
💡Height Requirement
Height requirements are specific minimum and maximum height limits set for certain roles or jobs, such as the live characters at an amusement park. In the script, the initial height requirements are a minimum of 57 inches and a maximum of 63 inches. These requirements are analyzed to determine the percentage of men who meet these criteria.
💡Percentage
A percentage is a way of expressing a number as a fraction of 100. It is used to describe proportions or comparisons. In the video, the percentage is used to express the proportion of men who meet the height requirements for amusement park characters. The script calculates that 4.11 percent of men meet the initial height criteria.
💡StatCrunch
StatCrunch is a statistical software tool used for data analysis, including the calculation of probabilities and z-scores. In the video, StatCrunch is used to find the area under the normal distribution curve between specific z-scores, which helps determine the percentage of men within the desired height range.
💡Bell Curve
A bell curve is a graphical representation of a normal distribution, so named because of its bell shape. In the video, the bell curve is used to visualize the distribution of men's heights and to identify the areas corresponding to the height requirements for amusement park characters.
💡Gender Implication
The term 'gender implication' refers to the inference or conclusion drawn about gender based on certain data or observations. In the video, the result of the height requirement analysis suggests that most characters at the amusement park are likely women, as the majority of men do not meet the height criteria.
💡New Height Requirements
In the second part of the video, 'new height requirements' refer to the revised criteria for the height of amusement park characters, which exclude the tallest 50 percent and the shortest five percent of men. The script calculates the new minimum and maximum height requirements based on these exclusions.
Highlights

A survey reveals the normal distribution of women's and men's heights with specific means and standard deviations.

Women's heights have a mean of 64.2 inches and a standard deviation of 4.2 inches.

Men's heights have a mean of 70.1 inches and a standard deviation of 4.1 inches.

Amusement park character height requirements range from a minimum of 57 inches to a maximum of 63 inches.

The process involves sketching a normal curve to visualize the area of interest for men's heights.

Z-scores are calculated for the minimum and maximum height requirements to standardize the data.

Z1 is calculated as -3.20 for the 57-inch height requirement.

Z2 is calculated as -1.73 for the 63-inch height requirement.

Statcrunch is used to find the probability between the two Z-scores.

Only 4.11% of men meet the height requirement, suggesting most characters are likely women.

Part B explores changing height requirements to exclude the tallest 50% and shortest 5% of men.

A new normal distribution curve is sketched with the updated height requirements.

Z-scores for the new requirements are calculated using Statcrunch.

Z1 for the new minimum requirement is -1.64, corresponding to 5% of the data.

Z2 for the new maximum requirement is 0.00, corresponding to the 50% mark.

The new minimum height requirement is calculated to be 63.4 inches.

The new maximum height requirement remains at the mean height of 70.1 inches.

The analysis provides insights into the gender distribution of amusement park characters based on height requirements.

Transcripts

Browse More Related Video

Math 14 6.2.25 Find the percentage of women meeting the height requirement.

Math 14 HW 6.4.15-T Using the Central Limit Theorem

The Most Depressing Graph EVER!

AP Physics B Kinematics Presentation General Problems #11

Projectile Motion - Projectile Fired Horizontally

2016 AP Calculus AB Free Response #5

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Height AnalysisAmusement ParkGender RatioStatistical SurveyZ-ScoresNormal DistributionCharacter RolesHeight RequirementsData VisualizationProbability Calculation