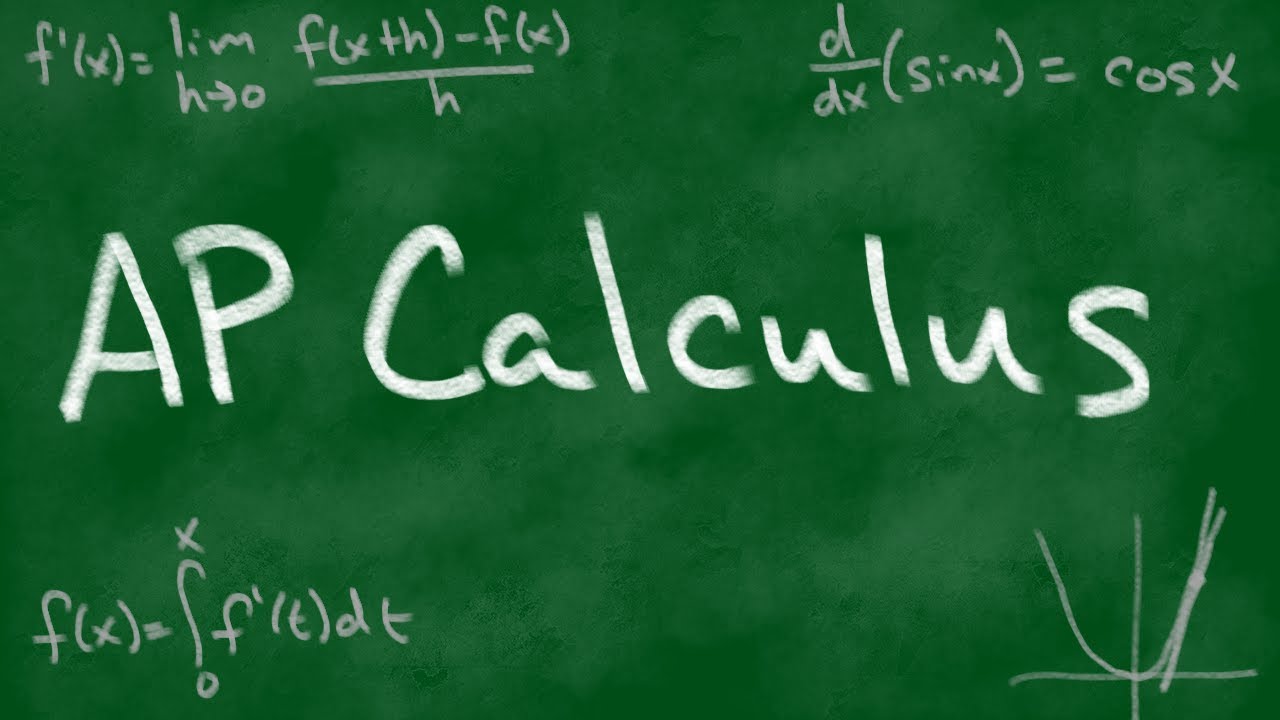2016 AP Calculus AB Free Response #5
TLDRIn this engaging video, Alan from Bottle Stem continues his series on AP Calculus 2016 free response questions, focusing on question number five. He tackles the problem of finding the average value of the radius of a funnel with a height of ten inches and a radius given by the function R(H) = √(3 + H^2), where R and H are measured in inches. Alan demonstrates the process of integration to find the average radius and then moves on to calculate the volume of the funnel by summing the volumes of infinitesimally thin discs. He also addresses a rate of change problem, determining the rate at which the height of liquid in the funnel is decreasing when the liquid's height is three inches and the radius is decreasing at a rate of 150 inches per second. Despite the complexity and the lack of a calculator, Alan manages to solve the problems step by step, providing a detailed walkthrough of the mathematical process. He concludes by sharing his thoughts on the difficulty of the question and offers additional help through his Twitch or Discord channels for those interested in math and physics.
Takeaways
- 📚 The video is a continuation of AP Calculus 2016 free response questions, focusing on question number five.
- 📏 The problem involves a funnel with a height of ten inches, and the radius of its cross-sections varies with height H according to a given formula.
- ⭕ The average value of the funnel's radius is calculated by integrating the radius function from 0 to 10 and then dividing by the interval width (10).
- 🧮 The integral to find the average radius involves the function R(H) = 1/(23 + H^2), which is integrated and simplified to find the average value.
- 🚀 The volume of the funnel is determined by summing the volumes of infinitesimally thin discs, each with a variable radius and thickness.
- 🔢 The volume calculation requires integrating the area of the disc (πR^2) times its thickness (DH) over the height of the funnel.
- ⏳ A rate of change problem is presented, asking for the rate at which the height of the liquid in the funnel is changing with respect to time when the liquid's height is three inches and the radius is decreasing at a rate of 150 inches per second.
- 🔄 To solve for the rate of change in height, the derivative of the radius with respect to time (dr/dt) is used, applying the chain rule to find dh/dt.
- 📉 The rate of change of the liquid's height with respect to time (dh/dt) is found to be negative two-thirds inches per second at the given conditions.
- 🎯 The presenter successfully calculates the average radius, volume, and rate of change, indicating a good understanding of calculus concepts.
- 📈 The presenter notes that the 2016 AP Calculus questions felt more tedious numerically compared to the 2017-2018 ones.
- 💻 The video concludes with an offer for free homework help on Twitch or Discord for those interested in learning more about math and physics.
Q & A
What is the formula given for the radius of the funnel at a certain height H?
-The radius of the funnel at height H is given by the formula R = 1/(23 + H^2), where R and H are in inches.
How is the average value of the radius of the funnel calculated?
-The average value of the radius is calculated by integrating the radius function R(H) with respect to H from 0 to 10, and then dividing by the width of the interval, which is 10 in this case.
What is the final result of the integral for the average radius of the funnel?
-The final result of the integral is approximately 30 + 1000/200, which simplifies to 30 + 5, giving a total of 35 inches.
How is the volume of the funnel calculated?
-The volume of the funnel is calculated by slicing it into thin discs and summing up the volumes of these discs. The volume of each disc is found by multiplying the area of the disc (πR^2) by its thickness (dH).
What is the expression for the volume of a single disc in the funnel?
-The volume of a single disc is given by the expression π * (1/(23 + H^2))^2 * dH.
What is the final expression for the volume of the funnel?
-The final expression for the volume of the funnel is π/400 * (9H + 2H^3 + 1/5 * H^5) evaluated from H = 0 to H = 10.
What is the result of the volume calculation for the funnel?
-The result of the volume calculation is π/40 * 22090 cubic inches.
What is the rate of change of the radius of the liquid surface with respect to time at H equals 3 inches?
-The rate of change of the radius with respect to time at H equals 3 inches is given as -1/5 inch per second.
How is the rate of change in the height of the liquid with respect to time calculated?
-The rate of change in the height of the liquid with respect to time (dh/dt) is calculated using the chain rule, where dr/dt is known and H is the height at which the rate is being calculated.
What is the rate of change in the height of the liquid with respect to time at H equals 3 inches?
-At H equals 3 inches, the rate of change in the height of the liquid with respect to time (dh/dt) is -2/3 inches per second.
What does Alan offer for those who need help with homework or want to learn about math and physics?
-Alan offers free homework help on platforms like Twitch or Discord for those who have homework questions or want to learn about different parts of math and physics.
What is the final parting message from Alan to the viewers?
-Alan thanks the viewers for watching, asks them to leave a comment, like, or subscribe, and informs them that he will see them in the final AP Calculus free response for the exam.
Outlines
📚 Calculus Problem: Funnel's Average Radius and Volume
In the first paragraph, Alan discusses an AP Calculus 2016 free response question focused on a funnel with a height of ten inches. The funnel's radius at any height H is given by a specific function. Alan calculates the average value of the funnel's radius by integrating the radius function from 0 to 10 and dividing by the interval width. He then moves on to calculate the volume of the funnel by slicing it into discs and summing their volumes. This involves integrating a function of the radius squared over the same interval. Alan mentions the complexity of the math involved and the absence of a calculator, which makes the process more challenging.
💧 Rate of Change in Liquid Height within a Funnel
The second paragraph deals with a rate of change problem concerning the liquid inside the funnel. At a specific instant when the liquid's height is three inches, the radius of the liquid surface is decreasing at a rate of 150 inches per second. Alan uses the given radius function and the rate of change of the radius to find the rate of change of the liquid's height with respect to time. He applies the chain rule to differentiate the equation and solves for the rate of change in height (dh/dt), which he calculates to be negative two-thirds inches per second. Finally, Alan discusses the scoring guideline for the problem and expresses satisfaction with his solution, noting the algebra involved was more complex than expected.
Mindmap
Keywords
💡AP Calculus
💡Free Response Questions
💡Integral
💡Volume
💡Rate of Change
💡Chain Rule
💡Disc Method
💡Average Value
💡Funnel
💡Cross-Sections
💡Twitch
💡Discord
Highlights
Alan is discussing AP Calculus 2016 free response question number five.
The problem involves finding the average value of the radius of a funnel with a height of ten inches.
The radius of the funnel at height H is given by the function R(H) = √(3 + H^2).
The average value of the radius is calculated by integrating R(H) from 0 to 10 and dividing by the interval width.
Alan simplifies the integral to 1/200 * (3H + 1/3 * H^3) evaluated from 0 to 10.
The final result for the average radius is approximately 30.1667 inches.
Next, Alan calculates the volume of the funnel by slicing it into discs and summing their volumes.
The volume of a single disc is given by π * R^2 * dH, where R is the radius and dH is the disc thickness.
The volume integral is set up as π/400 * (9 + 6H^2 + H^4) * dH from 0 to 10.
After evaluating the integral, the volume of the funnel is found to be (π/4) * 20909 cubic inches.
A rate of change problem is addressed, where the liquid in the funnel is draining, and the radius decreases at a rate of 150 inches per second when H = 3.
The derivative dr/dt is given as -1/5, and Alan uses it to find dh/dt using the chain rule.
The rate of change in the height of the liquid with respect to time, dh/dt, is calculated to be -2/3 inches per second.
Alan provides the scoring guideline for the question, which matches his calculations.
He comments on the difficulty level of the question, noting it to be more tedious than previous ones.
Alan offers free homework help on Twitch or Discord for those interested in learning math and physics.
He encourages viewers to comment, like, or subscribe for more educational content.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: