4.2.2 Addition and Multiplication Rules - The Addition Rule

Sasha Townsend - Tulsa
7 Oct 202009:46
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the addition rule in probability, teaching two methods: an intuitive approach using Venn diagrams and an algebraic method. It emphasizes avoiding double counting of outcomes where both events occur. The script illustrates the rule with the example of choosing a jack or a heart from a deck of cards, demonstrating how to calculate the probability correctly by either counting outcomes or using the formula P(A or B) = P(A) + P(B) - P(A and B).

Takeaways
  • 📚 The video discusses Learning Outcome Number Two from Lesson 4.2, focusing on understanding and applying the addition rule in probability.
  • 🔍 The addition rule helps to find the probability of event A or event B or both occurring, emphasizing the importance of not double-counting outcomes.
  • 📈 The intuitive approach to the addition rule involves adding the number of ways each event can occur and then dividing by the total number of outcomes, while avoiding double-counting overlaps.
  • 📊 A Venn diagram is used to illustrate the concept, showing the overlap between two events and how to calculate the combined probability without double-counting.
  • 🧩 The algebraic or formulaic approach to the addition rule is mathematically equivalent to the intuitive approach, using the formula P(A or B) = P(A) + P(B) - P(A and B).
  • 🔢 The formulaic approach subtracts the probability of both events occurring to account for the overlap, ensuring that outcomes are counted only once.
  • 🃏 An example using a deck of cards is provided to demonstrate the application of the addition rule, with events being choosing a jack or a heart.
  • 👁 The example clarifies the importance of recognizing and accounting for the overlap, such as the Jack of Hearts, which is both a jack and a heart.
  • 🔑 The key to the addition rule is to add the probabilities of individual events while being cautious about not counting overlapping outcomes twice.
  • 📝 The script emphasizes that both the intuitive and formulaic methods lead to the same result, reinforcing the understanding of the addition rule.
  • 🤔 The video aims to ensure viewers understand the addition rule, its application, and the importance of avoiding double-counting in probability calculations.
Q & A

  • What is the addition rule in probability?

    -The addition rule in probability is used to find the probability that either event A occurs, event B occurs, or both occur. It allows for the calculation of the combined probability of two events without overlap.

  • What are the two approaches to applying the addition rule discussed in the video?

    -The two approaches to applying the addition rule are the intuitive approach and the formulaic or algebraic approach. Both methods convey the same concept but present it in different ways.

  • How does the intuitive approach to the addition rule work?

    -The intuitive approach involves adding the number of ways event A can occur to the number of ways event B can occur, ensuring that outcomes are not double-counted. This is often illustrated using a Venn diagram to visualize the overlap between the two events.

  • What is the formulaic or algebraic approach to the addition rule?

    -The formulaic approach uses the formula P(A or B) = P(A) + P(B) - P(A and B), which accounts for the overlap between events A and B by subtracting the probability of both events occurring together.

  • Why is it important to avoid double-counting outcomes in the addition rule?

    -Double-counting outcomes would lead to an inflated probability that does not accurately represent the likelihood of the events occurring. It's crucial to ensure each outcome is counted only once for an accurate probability calculation.

  • Can you give an example of how to use the addition rule with a Venn diagram?

    -In the script, the example of choosing a jack or a heart from a deck of cards is used. The Venn diagram would have two circles representing the events A (choosing a jack) and B (choosing a heart), with the overlap representing the jack of hearts, which is counted only once.

  • What is the significance of the sample space in the context of the addition rule?

    -The sample space represents all possible outcomes of an experiment. When applying the addition rule, the sum of the probabilities of the individual events (minus the overlap) is divided by the total number of outcomes in the sample space to find the combined probability.

  • How many cards are in a standard deck and how are they distributed among suits?

    -A standard deck has 52 cards, distributed evenly among four suits: spades, clubs, hearts, and diamonds, with each suit containing 13 cards.

  • What is the probability of choosing a jack from a deck of cards?

    -Since there are 4 jacks in a deck of 52 cards, the probability of choosing a jack is 4 out of 52.

  • What is the probability of choosing a heart from a deck of cards?

    -There are 13 hearts in a deck of 52 cards, so the probability of choosing a heart is 13 out of 52, which simplifies to 1 out of 4.

  • How can you find the probability of choosing either a jack or a heart from a deck of cards without using the formula?

    -You can count the number of ways to choose a jack (4) and the number of ways to choose a heart (13), then add them together and subtract the overlap (jack of hearts) to get 16. Then divide by the total number of cards (52) to get the probability.

  • What are some key points to remember when using the addition rule?

    -Key points include recognizing when to use the addition rule (probabilities of 'or' events), adding the number of ways each event can occur, being cautious not to double-count overlapping outcomes, and if using the formula, subtracting the probability of both events occurring together.

Outlines
00:00
📚 Understanding the Addition Rule for Probabilities

This paragraph introduces the concept of the addition rule in probability theory, which is used to calculate the likelihood of two events, A or B, occurring. It explains two methods of applying the rule: an intuitive approach using a Venn diagram to visualize the overlapping outcomes, and a formulaic approach using algebra. The Venn diagram helps to ensure outcomes are not double-counted by subtracting the overlap from the total. The formulaic approach is shown to be equivalent to the intuitive one, with the formula P(A or B) = P(A) + P(B) - P(A and B), where P(A and B) accounts for the overlap to avoid double-counting.

05:03
🃏 Applying the Addition Rule with a Deck of Cards Example

The second paragraph provides a practical example of applying the addition rule using a deck of cards. It discusses calculating the probability of drawing either a jack or a heart, or both. The example clarifies the need to consider the total number of cards and the distribution across suits. It explains how to count the number of ways each event can occur, being careful to avoid double-counting the jack of hearts, which is both a jack and a heart. The paragraph demonstrates both the intuitive counting method and the formulaic calculation, showing that both methods yield the same result, which is the simplified probability of 4 out of 13.

Mindmap
Keywords
💡Addition Rule
The Addition Rule is a fundamental concept in probability theory that allows for the calculation of the likelihood of either one event or another, or both, occurring. In the video, it is defined as a method to find the probability of event A or event B or both happening. It's central to the video's theme, as it underpins the intuitive and formulaic approaches discussed. For example, the script explains that when using the Addition Rule, one must add the probabilities of A and B but subtract the probability of their intersection to avoid double-counting outcomes.
💡Probability
Probability is a measure of the likelihood that a particular event will occur, expressed as a number between 0 and 1. In the context of the video, probability is calculated using the Addition Rule to determine the chance of one event or another happening. The script uses the example of choosing a jack or a heart from a deck of cards to illustrate how probabilities are calculated and adjusted using the Addition Rule to account for overlapping outcomes.
💡Intuitive Approach
The Intuitive Approach refers to a method of understanding or solving problems based on common sense or general reasoning, rather than formal mathematical procedures. In the video, this approach is used to explain the Addition Rule by visually representing events A and B on a Venn diagram and ensuring outcomes are counted once. The script emphasizes that this approach leads to the same result as the formulaic method, providing a more accessible entry point for understanding the concept.
💡Formulaic Approach
The Formulaic Approach involves using a mathematical formula to solve a problem. In the video, this approach is contrasted with the intuitive method but is shown to yield the same result. The script presents a formula for the Addition Rule: P(A or B) = P(A) + P(B) - P(A and B), which mathematically ensures that outcomes are not double-counted, aligning with the principles explained through the intuitive approach.
💡Venn Diagram
A Venn Diagram is a visual tool used to illustrate the relationships between sets, particularly the overlap between two sets. In the video, a Venn Diagram is used to represent events A and B, showing the distinct outcomes of each event and the shared outcomes where they overlap. The script uses the Venn Diagram to demonstrate the process of adding the probabilities of A and B while carefully excluding the overlap to avoid counting outcomes twice.
💡Sample Space
The Sample Space is the set of all possible outcomes of an experiment. In the video, the sample space is the entire deck of 52 cards when discussing the probabilities of drawing a jack or a heart. The script explains that the total number of outcomes in the sample space is used as the denominator when calculating probabilities, emphasizing the importance of this total in ensuring accurate probability calculations.
💡Outcomes
Outcomes refer to the individual results that can occur in an experiment or event. In the video, outcomes are the specific cards that can be drawn from a deck, such as the jack of hearts or the queen of spades. The script discusses how to count these outcomes correctly when applying the Addition Rule, ensuring that shared outcomes between events A and B are not double-counted.
💡Overlapping Outcomes
Overlapping Outcomes are results that are common to two or more events. In the video, the jack of hearts is an example of an overlapping outcome, as it is both a jack and a heart. The script explains the importance of identifying and correctly accounting for these outcomes when using the Addition Rule to prevent them from being counted more than once in probability calculations.
💡Deck of Cards
A Deck of Cards is the complete set of playing cards used in various card games. In the video, a standard deck of 52 cards is used as an example to illustrate the application of the Addition Rule. The script details the composition of the deck, with four suits each containing 13 cards, including the jacks and hearts, to provide a concrete context for calculating probabilities.
💡Suits
Suits refer to the categories into which the cards in a deck are divided, typically spades, clubs, hearts, and diamonds in a standard deck. The video uses suits to organize the sample space of the deck of cards and to identify the specific cards that can be drawn as outcomes. The script explains that there are 13 cards in each suit, including the jacks, which are used in the example to calculate the probability of drawing a jack or a heart.
Highlights

The video discusses the addition rule for calculating the probability of two events occurring.

Two approaches to applying the addition rule are presented: an intuitive approach and a formulaic or algebraic approach.

The intuitive approach involves adding the number of ways each event can occur and dividing by the total outcomes, ensuring no double-counting.

A Venn diagram is used to illustrate the overlapping outcomes between two events.

The formulaic approach is shown to be equivalent to the intuitive approach, with the overlap accounted for by subtraction.

An example using a deck of cards is provided to demonstrate the application of the addition rule.

The example explains how to calculate the probability of choosing a jack or a heart from a deck of cards.

The importance of understanding the structure of a deck of cards for probability calculations is emphasized.

The calculation process is detailed, showing how to avoid double-counting the jack of hearts.

The video demonstrates both the intuitive and formulaic methods for calculating the probability of choosing a jack or a heart.

The final probability is simplified to 4 out of 13, showing the equivalence of both methods.

Key points for using the addition rule are summarized, including avoiding double-counting and using the rule for 'or' scenarios.

The video stresses the importance of recognizing when to apply the addition rule in probability problems.

The transcript concludes with an invitation for further help, indicating a supportive learning environment.

The video's educational value lies in clearly explaining complex probability concepts using relatable examples.

The use of visual aids like the Venn diagram enhances understanding of the addition rule.

The video's practical application of the addition rule to a common scenario makes the concept more accessible.

Transcripts

Browse More Related Video

Probability Tree Diagrams

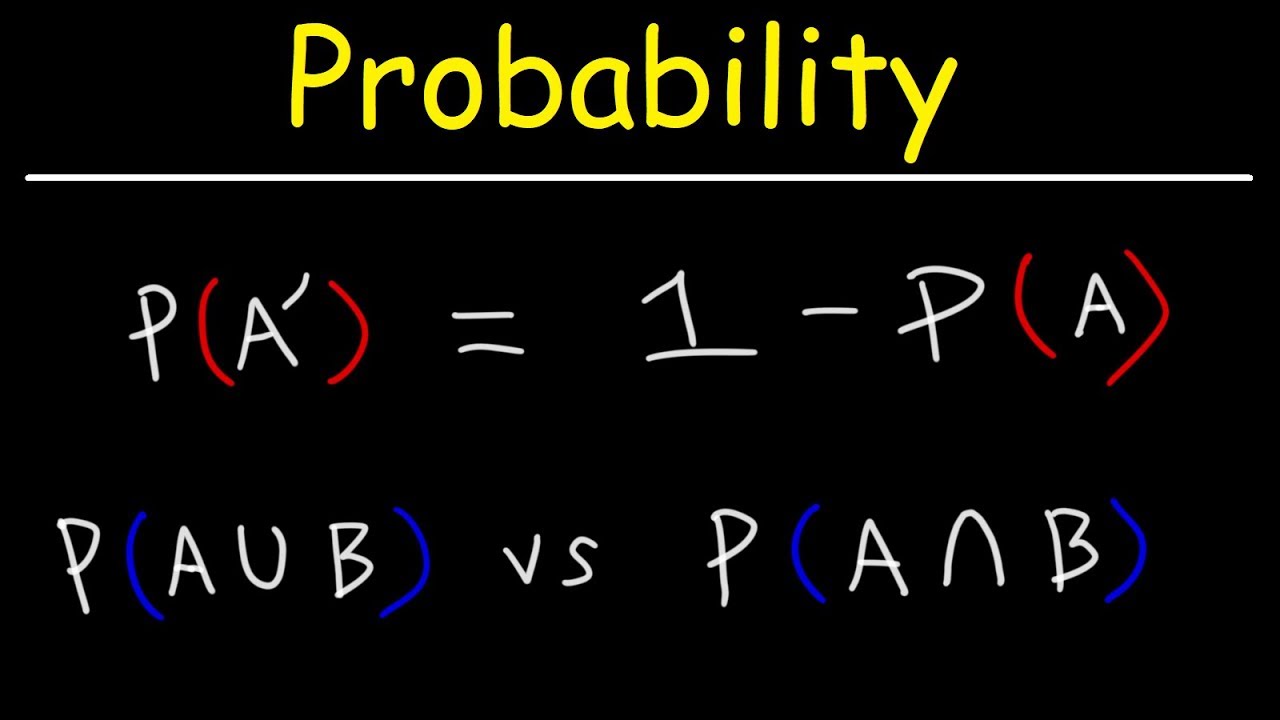
Probability of Complementary Events & Sample Space

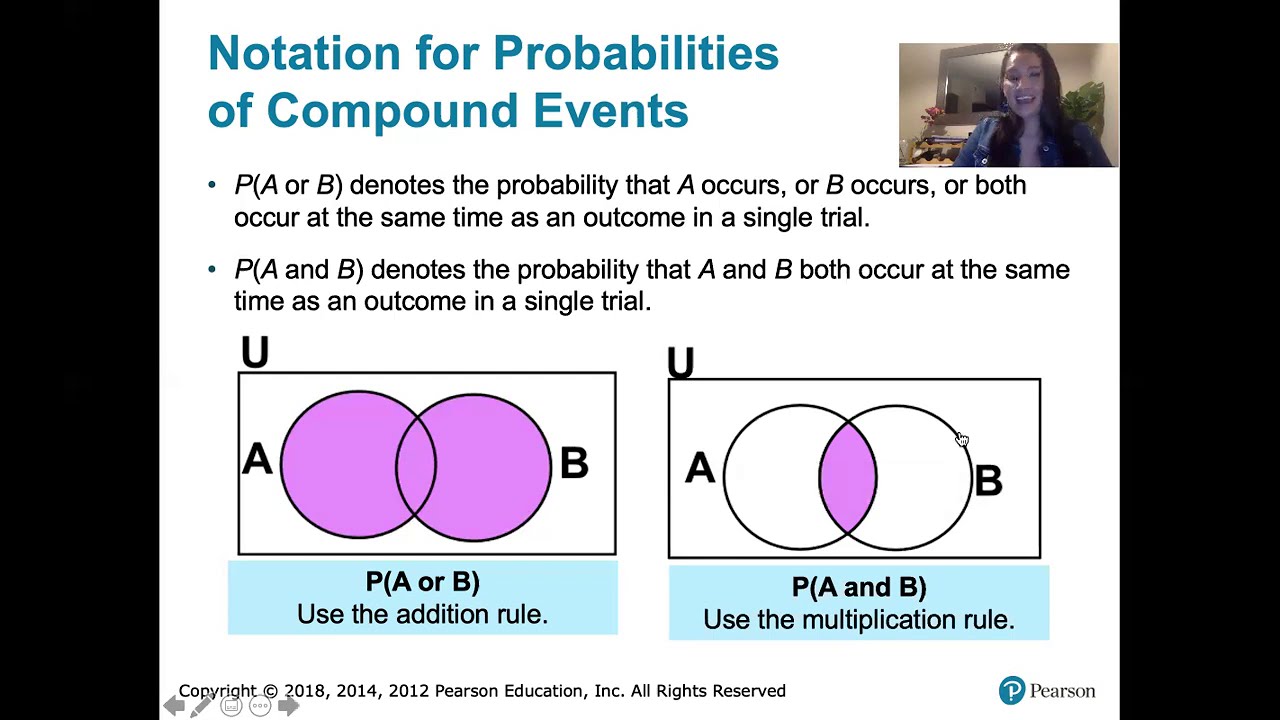
4.2.1 Addition and Multiplication Rules - Simple and Compound Events, and Their Probabilities

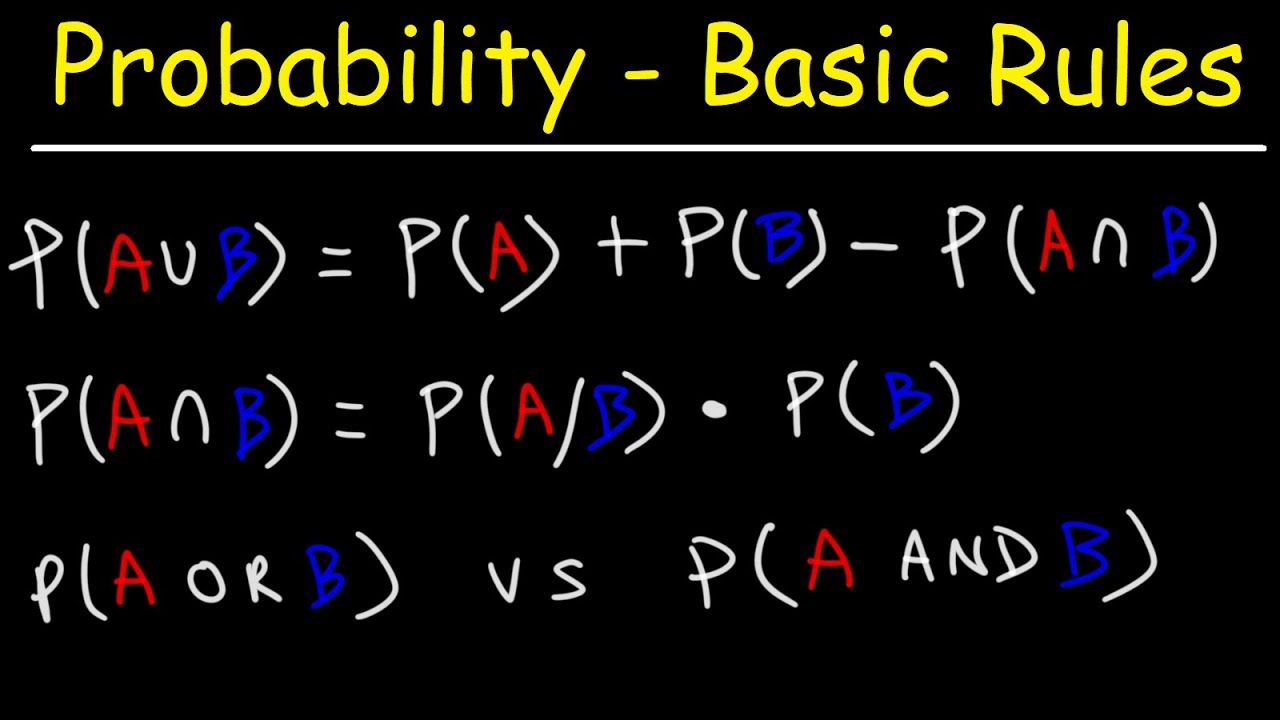
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events

Bernoulli Distribution

Bernoulli, Binomial and Poisson Random Variables

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityAddition RuleIntuitive ApproachFormulaic ApproachVenn DiagramCard DeckJackHeartSample SpaceProbability CalculationLearning Outcome