Polar Coordinates and Graphing Polar Equations

Professor Dave Explains

7 Feb 201810:45

EducationalLearning

32 Likes 10 Comments

TLDRThe video explains polar coordinates as an alternative to the traditional rectangular coordinate system. Points are plotted by a distance R from a central pole or origin and an angle θ from the polar axis. Converting between polar and rectangular coordinates involves trigonometric relationships. Plotting graphs in polar coordinates is especially useful for circles, semicircles, and other curved shapes based on the trig functions. The video gives examples of plotting points and graphing equations in the polar coordinate system, as well as practice problems for converting between the two systems.

Takeaways

😀 Polar coordinates define a point by its distance from the origin (radius) and the angle it makes with the polar axis (theta)
👉 Rectangular coordinates use X and Y values; polar coordinates use R and theta values to locate a point
📏 It's easy to convert between rectangular and polar coordinate systems using trig functions
🔀 A point can have multiple valid sets of polar coordinates, unlike rectangular coordinates
📐 Plotting points in the polar coordinate system involves going to the angle theta, then the radius R
✏️ Converting equations between rectangular and polar forms involves substituting X with R cos(theta) and Y with R sin(theta)
🏹 Graphing circles is much easier using polar coordinates compared to rectangular
🖍 Random trig functions plotted in polar mode can create interesting shapes like rose curves
❗️ The pole is located at the origin and the polar axis extends horizontally from it
👍 Polar coordinates are extremely useful for graphing certain equations, like circles

Q & A

What are the two coordinates used in polar coordinates?
-In polar coordinates, the first coordinate tells us a radius, or a distance from the origin, and the second coordinate tells us an angle from the polar axis to a line segment that contains the point.
Can the angle in polar coordinates be represented in degrees or radians?
-Yes, the angle in polar coordinates can be represented in either degrees or radians.
How can the same point have different valid polar coordinates?
-The same point can have different valid polar coordinates because we can add multiples of 2π to the angle coordinate and get the same point. We can also switch the sign of the R value and add multiples of 2π.
What information do we need to convert between polar and rectangular coordinates?
-To convert between polar and rectangular coordinates, we need the equations x = Rcosθ and y = Rsinθ, as well as the Pythagorean theorem R^2 = x^2 + y^2 and tangent θ = y/x.
Why are polar coordinates useful for graphing circles?
-Polar coordinates make graphing circles easier because a circle equation has a simple form like R = 5 in polar coordinates, whereas the rectangular form contains x^2 + y^2 and is more complex.
How can we plot a polar equation by plugging in points?
-We can plug in values for θ, like multiples of π/6, calculate the corresponding R values, and plot the resulting points to construct the graph.
What kinds of shapes can complex polar equations produce?
-More complicated polar equations can produce loops, rose curves, and other intricate and beautiful shapes.
How can we check our work in converting between coordinate systems?
-We can convert a point from polar to rectangular coordinates and back again to check that we get the same original point, verifying the process.
What is the advantage of using rectangular vs. polar coordinates?
-Rectangular coordinates uniquely specify points, while polar coordinates are better for representing circles, symmetry, and periodic functions.
What other contexts are polar coordinates used in besides graphing?
-Polar coordinates are used in navigation, physics, engineering, and complex number planes in higher math.

Outlines

00:00

😀 Introducing Polar Coordinates

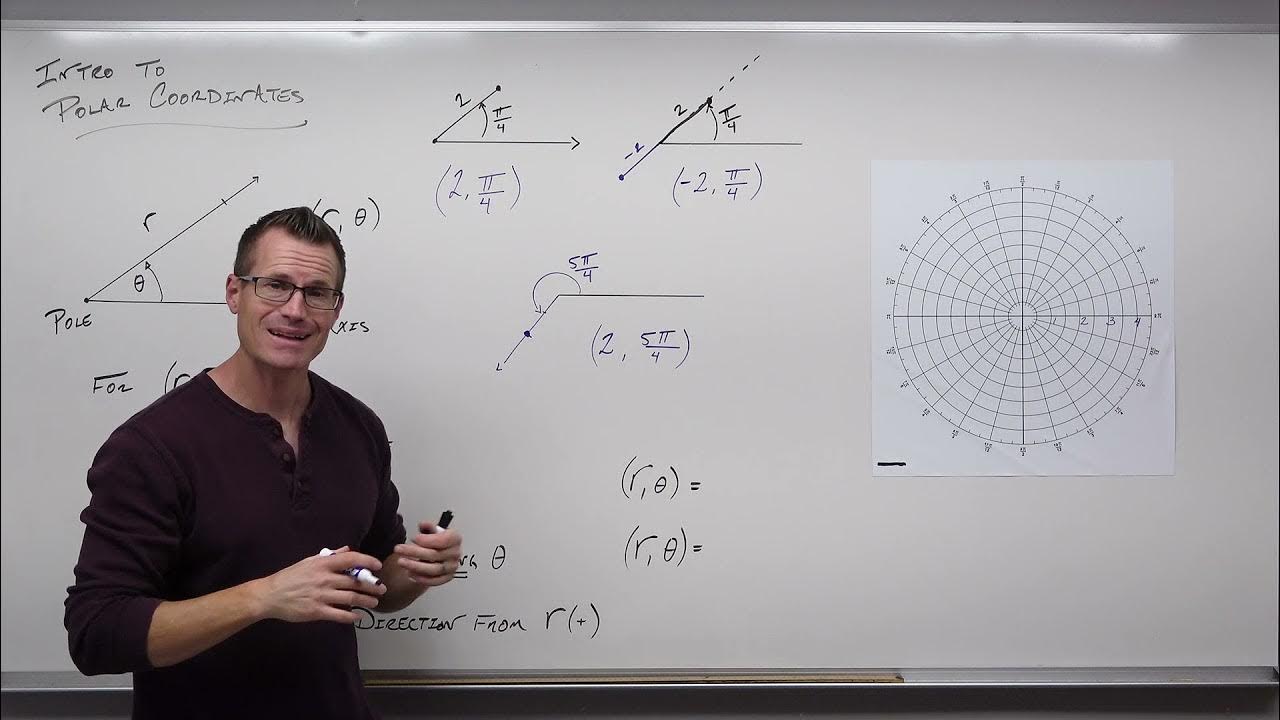
This paragraph introduces the concept of polar coordinates as an alternative to rectangular coordinates for plotting points on a plane. It explains that polar coordinates consist of an R value representing the distance from the origin and a theta value representing the angle from the polar axis. It gives examples of plotting points using polar coordinates and notes that points can have multiple valid coordinate representations.

05:05

😀 Converting Between Coordinate Systems

This paragraph explains how to convert equations and points between rectangular and polar coordinate systems using trigonometric identities. It shows how circles are easier to represent in polar coordinates. It also briefly discusses graphing polar equations and using a graphing calculator to visualize different polar graphs.

Mindmap

Keywords

💡Polar coordinates

A coordinate system used to locate points on a plane. Instead of the rectangular x and y coordinates, polar coordinates use an angle θ and a radius or distance r from a central point called the pole or origin. Polar coordinates allow for easy representation of circles, spirals, and other shapes.

💡Rectangular coordinates

The standard x and y coordinate system on a Cartesian plane, where x represents the horizontal position and y represents the vertical position. Polar coordinates provide an alternative way to locate points.

💡Unit circle

A circle with a radius of 1 unit, used to easily look up trigonometric values for common angles. Understanding the unit circle helps in working with and graphing polar coordinates.

💡Radius

In polar coordinates, the radius r represents the distance from the origin point. It is one of two values needed to plot a point, the other being the angle θ.

💡Angle

In polar coordinates, the angle θ indicates the direction from the polar axis to the point being located. Together with the radius r, this angle uniquely identifies each point.

💡Quadrants

The four sections of the coordinate plane divided by the x and y axes. Polar coordinates can represent points in all quadrants using positive or negative values for the radius r.

💡Graphing

Plotting equations on a coordinate plane. Polar equations often produce circles, roses, spirals, and other exotic shapes compared to the simpler lines and parabolas graphed in rectangular coordinates.

💡Coordinate conversion

Switching between rectangular and polar coordinate systems for the same point or equation. Converting between the two allows leveraging the advantages of each system.

💡Trigonometry

Using trig functions like sine, cosine, and tangent to analyze relationships between angles and side lengths in triangles. Trigonometry is key to converting between rectangular and polar coordinates.

💡Equation conversion

Transforming equations expressed in x and y coordinates into the radius r and angle θ used in the polar system. This allows graphing equations in polar coordinates more easily.

Highlights

Polar coordinates use radius and angle to plot points rather than x and y coordinates.

In polar coordinates, most points have multiple valid coordinate representations.

Converting between polar and rectangular coordinates involves basic trigonometry.

Polar coordinates make graphing circles much simpler.

Polar coordinates are best suited for plotting circular or radial patterns.

Graphing polar equations by plugging in values is an easy way to plot simple cases.

More complex polar equations can create beautiful and intriguing graphs.

Any point on the coordinate plane can be represented by rectangular or polar coordinates.

Polar coordinates consist of a radius R and angle theta.

Negative radius values in polar coordinates refer to the opposite direction.

Rectangular equations can be converted to polar form using trigonometric identities.

Graphing complex polar equations by plugging in values is an insightful exercise.

Understanding polar coordinates builds on knowledge of trigonometry and the unit circle.

Polar coordinates offer an alternate, useful perspective for understanding functions.

Converting between coordinate systems broadens understanding of geometric relationships.

Transcripts

Browse More Related Video

Introduction to Polar Coordinates (Precalculus - Trigonometry 36)

Ch. 8.1 Polar Coordinates

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

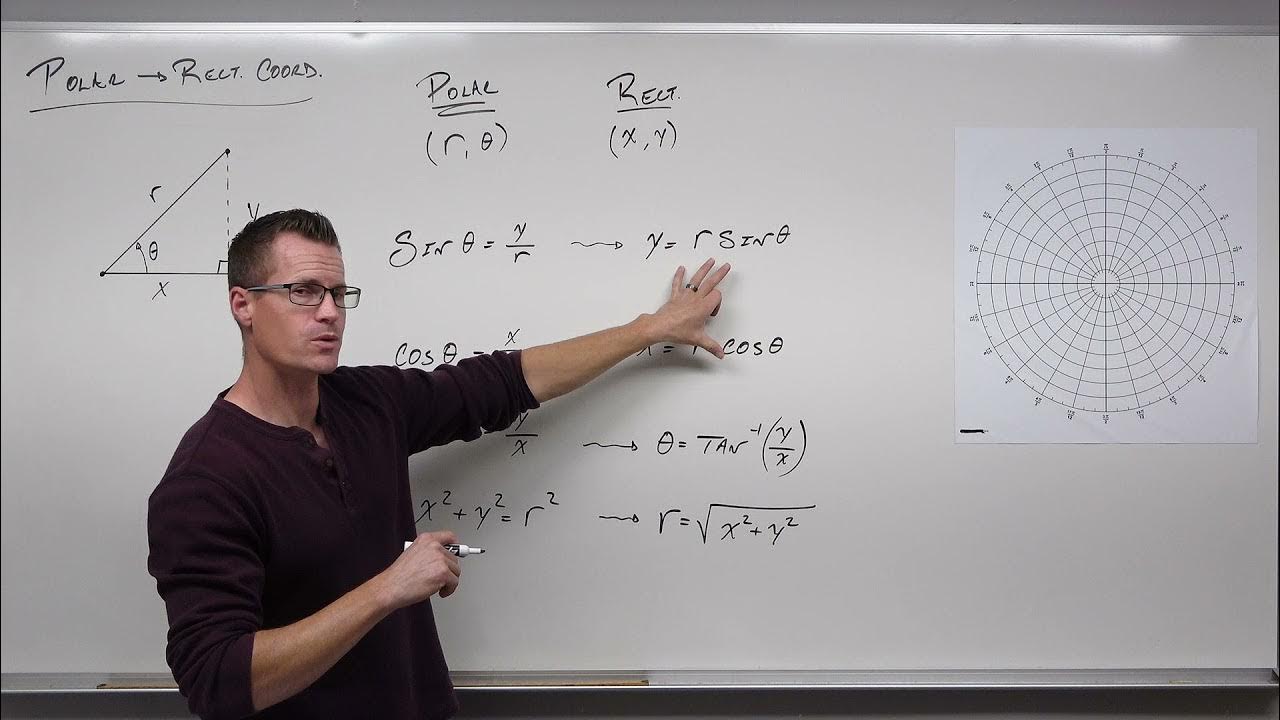
How to Convert From Polar Coordinates to Rectangular Coordinates (Precalculus - Trigonometry 37)

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

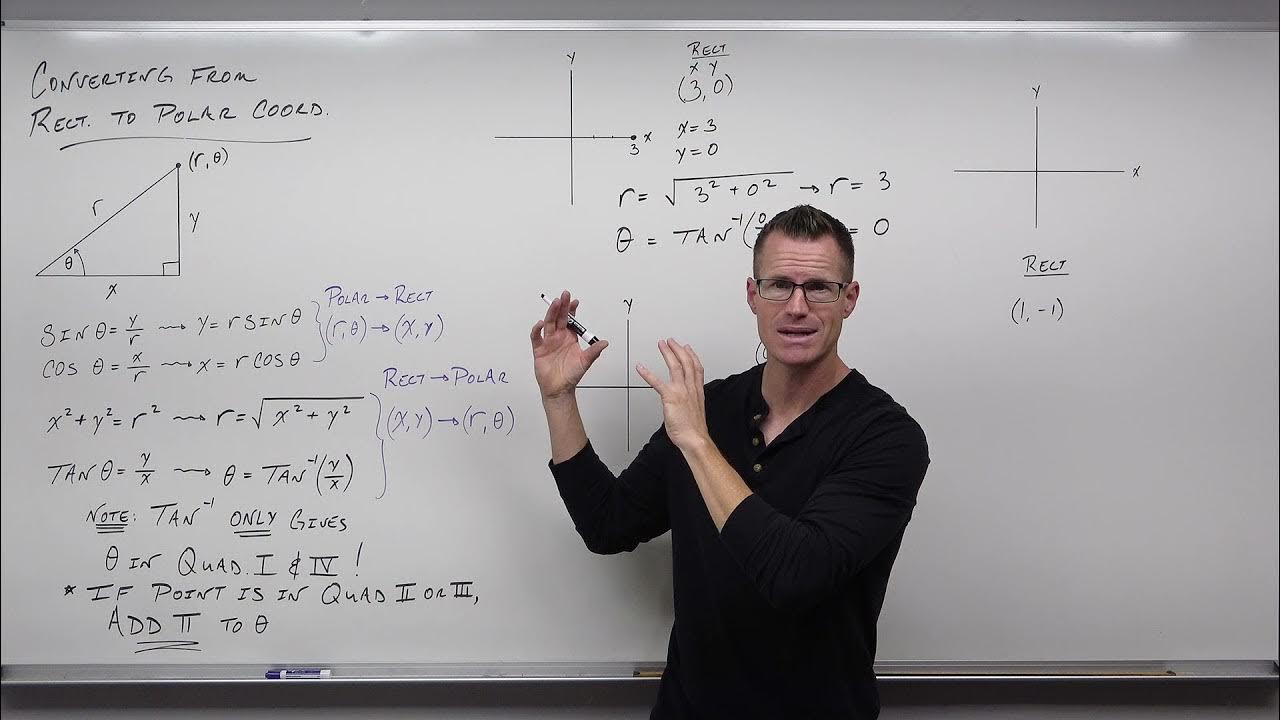
How to Convert From Rectangular Coordinates to Polar Coordinates (Precalculus - Trigonometry 38)

Polar Coordinates and Graphing Polar Equations

Takeaways

Q & A

What are the two coordinates used in polar coordinates?

Can the angle in polar coordinates be represented in degrees or radians?

How can the same point have different valid polar coordinates?

What information do we need to convert between polar and rectangular coordinates?

Why are polar coordinates useful for graphing circles?

How can we plot a polar equation by plugging in points?

What kinds of shapes can complex polar equations produce?

How can we check our work in converting between coordinate systems?

What is the advantage of using rectangular vs. polar coordinates?

What other contexts are polar coordinates used in besides graphing?