Lecture 09: Archimedes and the Tractrix
TLDRThis lecture explores the historical methods of calculating areas and volumes of geometric figures before the integral was defined. Cavalieri's method of indivisibles is discussed, which approximated the volume of a sphere by summing the volumes of countless tetrahedra. The video also delves into Archimedes' ingenious use of levers to deduce the volume of a sphere, balancing a sphere and a cone against a cylinder. Modern insights by Mama cone monatsukanian are highlighted, showing how to find areas under complex curves and between concentric circles by approximating shapes with polygons and sectors of circles. The Pythagorean theorem is also intriguingly derived from these area calculations. The lecture concludes with the application of these concepts to find the area under the tractrix curve, demonstrating the integral's philosophy of breaking down complex shapes into simpler components.
Takeaways
- 📚 The script discusses historical methods for calculating the areas and volumes of geometrical figures, predating the formal definition of the integral.
- 🎨 Cavalieri's method of indivisibles from the 17th century is highlighted, which involved breaking figures into small pieces to sum their volumes or areas.
- 🌐 Cavalieri deduced the volume of a sphere by imagining it composed of tetrahedra, leading to the formula \( \frac{4}{3} \pi r^3 \) for the volume.
- 🤔 Archimedes' method of levers from the 3rd century BC is presented as an innovative way to find the volume of a sphere using the balance of weights and distances.
- ⚖️ Archimedes balanced a sphere and a cone against a cylinder to derive the volume of the sphere, showcasing the relationship between geometry and algebra.
- 📉 The script transitions to modern techniques, introducing the work of Mama cone monatsukanian and Tom Apostle, who applied similar concepts to find areas under complex curves.
- 📐 Monox Canyon's method involves approximating shapes with polygons and sweeping sectors of circles to find areas, simplifying the process of calculating complex geometric properties.
- 🔶 The area between two concentric circles, or an annulus, can be found using Monox Canyon's strategy by considering the sectors as parts of a whole circle.
- 🛤️ The Pythagorean theorem is unexpectedly derived from Monox Canyon's analysis of the area of a ring between two circles, demonstrating the interconnectedness of mathematical concepts.
- 📈 The area under the tractrix curve, a complex figure, is found using a clever approximation of straight segments and circular sectors, resulting in a quarter-circle area.
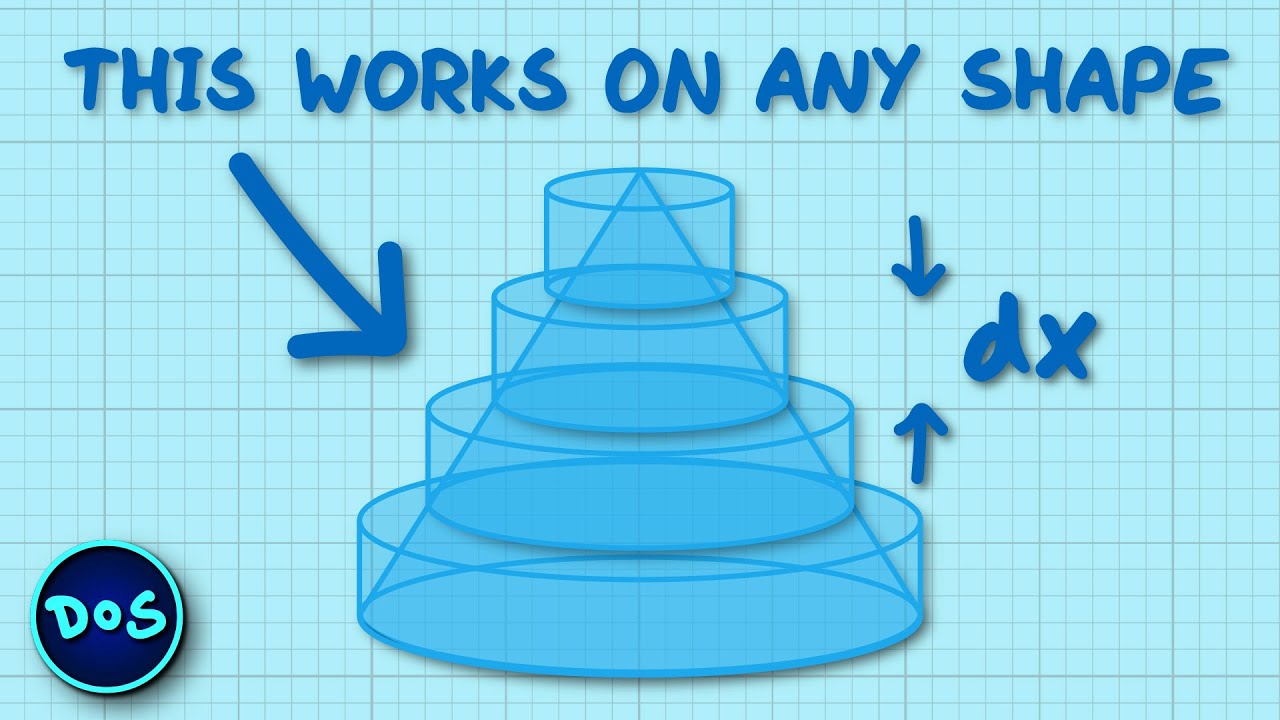
- 🧩 The overarching theme is the philosophy of integration, breaking down complex shapes into manageable pieces and recombining them to find total areas or volumes.
Q & A
What method did Cavalieri use to deduce the volume of a sphere?
-Cavalieri used the method of indivisibles, imagining the sphere as composed of a collection of tetrahedra with their bases on the surface of the sphere and cones down to the center. The volume of the sphere was then deduced to be one-third times the product of the sphere's surface area (4πr^2) and its radius (R).
How did Archimedes find the formula for the volume of a sphere?
-Archimedes used his method of levers, balancing the sphere with a cone and a cylinder of specific dimensions. By knowing the volume formulas for the cone and cylinder and using the principle of levers, he deduced the volume of the sphere to be four-thirds πR^3.
What is the significance of the Pythagorean theorem in the context of Archimedes' method for finding the volume of a sphere?
-The Pythagorean theorem is used to find the radius of the discs that are created when slicing the sphere, cone, and cylinder. This helps in balancing these shapes on a lever, which is crucial for deducing the volume of the sphere.
What is the relationship between the derivative of the volume of a sphere and its surface area?
-The derivative of the volume of a sphere with respect to its radius is equal to the surface area of the sphere. This is shown by taking the derivative of the volume formula (4/3 πR^3) and simplifying it to 4πR^2, which is the formula for the surface area of a sphere.
How does Mama cone monatsukanian's method relate to the concept of integration?
-Mama cone monatsukanian's method involves breaking up regions into small pieces and recombining them to find the total area, which is a fundamental concept of integration.
What is the area between two concentric circles called?
-The area between two concentric circles is called an annulus.
How does monatsukanian's strategy help in finding the area of a ring (annulus) between two concentric circles?
-Monatsukanian's strategy involves approximating the inner circle by a polygon with many sides and sweeping sectors of a circle around it. The sum of these sectors can be recombined to form a circle, whose area is equal to the area of the ring.
How does the analysis of the area of a ring lead to a proof of the Pythagorean theorem?
-By comparing the area of the ring found using monatsukanian's method (pi * a^2) with the area found by subtracting the areas of the circles (R^2 - r^2), we get the equation R^2 = a^2 + r^2, which is the Pythagorean theorem.
What is the tractrix and how can its area be found using monatsukanian's method?
-The tractrix is a curve obtained by dragging a point vertically above the origin along the x-axis. Monatsukanian's method can be used to approximate the area under the tractrix by considering it as a series of sectors of circles, which can be recombined to form a quarter of a circle, thus finding the area under the curve.
What insight does the script provide about the philosophy of integration?
-The script illustrates the philosophy of integration by showing how complex areas and volumes can be found by breaking them into small pieces and adding up their contributions, as demonstrated by the methods of Cavalieri, Archimedes, and monatsukanian.
Outlines
📚 Historical Methods for Calculating Volumes and Areas
This paragraph delves into historical mathematical methods used to calculate the areas and volumes of geometrical figures. It begins with Cavalieri's method of indivisibles from the 17th century, which involved breaking down shapes into small pieces to find their volumes. The example given is Cavalieri's calculation of the volume of a sphere by imagining it composed of tetrahedra. The paragraph then transitions to Archimedes' method from the 3rd century BC, which used the principle of levers to find the volume of a sphere. Archimedes' approach involved balancing the volumes of a sphere, a cone, and a cylinder on a lever to deduce the formula for the sphere's volume. The paragraph highlights the ingenuity of these ancient methods and their significance in the development of calculus.
🔍 Archimedes' Lever Method for Volume Calculation
This section focuses on Archimedes' innovative use of the lever principle to calculate the volume of a sphere. It describes a thought experiment where a sphere, a cone, and a cylinder are imagined to be placed on a lever to achieve balance. By slicing these shapes into thin discs and balancing them according to their weights and distances from the fulcrum, Archimedes was able to deduce the volume of the sphere. The paragraph explains the mathematical reasoning behind this balance, leading to the formula for the sphere's volume as four-thirds pi times the radius cubed. This method is celebrated for its elegance and the clever application of physical principles to a mathematical problem.
🎯 Modern Geometric Insights and the Pythagorean Theorem
The paragraph introduces modern geometric insights by Mama cone monatsukanian and Tom Apostle, which, while not predating the integral, may have been concepts considered by Archimedes himself. The discussion revolves around approximating complex shapes by breaking them into smaller, more manageable pieces. Monox Canyon's method involves sweeping sectors of a circle around polygons to eventually form a complete circle, demonstrating that the sum of these sectors equals the area of a circle with a radius equal to the distance of the sector's sweep. This insight is then applied to find the area between two concentric circles, leading to an unexpected proof of the Pythagorean theorem. The paragraph showcases how ancient geometric principles can still inspire novel mathematical discoveries.
🛠 Monox Canyon's Method for Area Calculations
This section explores Monox Canyon's method for calculating areas, particularly focusing on the area between two concentric circles. The method involves approximating the inner circle with a polygon and then sweeping sectors of a circle around it. As the polygon becomes more complex (e.g., a circle with a million sides), the sectors combine to form a ring that is indistinguishable from a circle with radius 'a', where 'a' is the distance from the inner circle's tangent to the outer circle. This approach simplifies the calculation of the area between two concentric circles and leads to the realization that two rings can have the same area if the distance from the inner circle's tangent to the outer circle is consistent. The paragraph also demonstrates how this method can be used to prove the Pythagorean theorem.
🌀 The Tractrix Curve and Its Area
The paragraph discusses the tractrix curve, a complex curve generated by dragging a point vertically above the origin along the x-axis. Traditional methods for finding the area under this curve involve calculus and can be quite challenging. However, Monox Canyon's method offers a clever alternative. By approximating the tractrix as a series of straight segments and circular sectors, the area under the curve can be visualized as a series of sectors that, when combined, form a quarter circle. As the number of segments increases, the approximation becomes more accurate, leading to the conclusion that the area under the tractrix curve is a quarter of a circle with radius 'a', where 'a' is the original height of the point above the origin. This approach simplifies the calculation and provides a geometric understanding of the area under the tractrix curve.
🧩 The Integral Philosophy and Its Applications
In the final paragraph, the video script wraps up by emphasizing the philosophy of integration, which involves breaking down complex shapes into smaller pieces and summing their areas to find the total. The tractrix curve example serves as a demonstration of this concept, showing how even intricate curves can have their areas determined through simple geometric constructions. The paragraph also expresses anticipation for future discussions, suggesting that these methods and insights will continue to be explored and applied to various mathematical problems. The integral philosophy is presented as a unifying theme that connects historical and modern approaches to calculating areas and volumes.
Mindmap
Keywords
💡Integral
💡Cavalieri's Principle
💡Archimedes
💡Method of Indivisibles
💡Tetrahedron
💡Surface Area
💡Volume
💡Pythagorean Theorem
💡Annulus
💡Tractrix
Highlights
Introduction of Cavalieri's method of indivisibles for calculating the volume of a sphere.
Cavalieri's deduction of the sphere's volume formula using tetrahedra.
Archimedes' method of levers to find the volume of a sphere.
Archimedes' balancing of geometric solids to deduce the volume of a sphere.
Derivation of the sphere's volume formula using Archimedes' method.
Connection between the derivative of the sphere's volume and its surface area.
Introduction of modern mathematician Mama cone monatsukanian's work.
Monatsukanian's strategy for approximating complex shapes with sectors of a circle.
Finding the area between two concentric circles using monotsikanian's method.
Proof of the Pythagorean theorem using the area of a ring.
Monatsukanian's method applied to find the area under a tractrix curve.
Approximation of the tractrix curve using sectors of a circle.
Calculation of the area under the tractrix curve as a quarter of a circle.
Philosophy of the integral and its application in breaking down complex shapes into smaller pieces.
Anticipating the next lecture with more insights into integral calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: