Lecture 20: Several Variables Volumes Galore

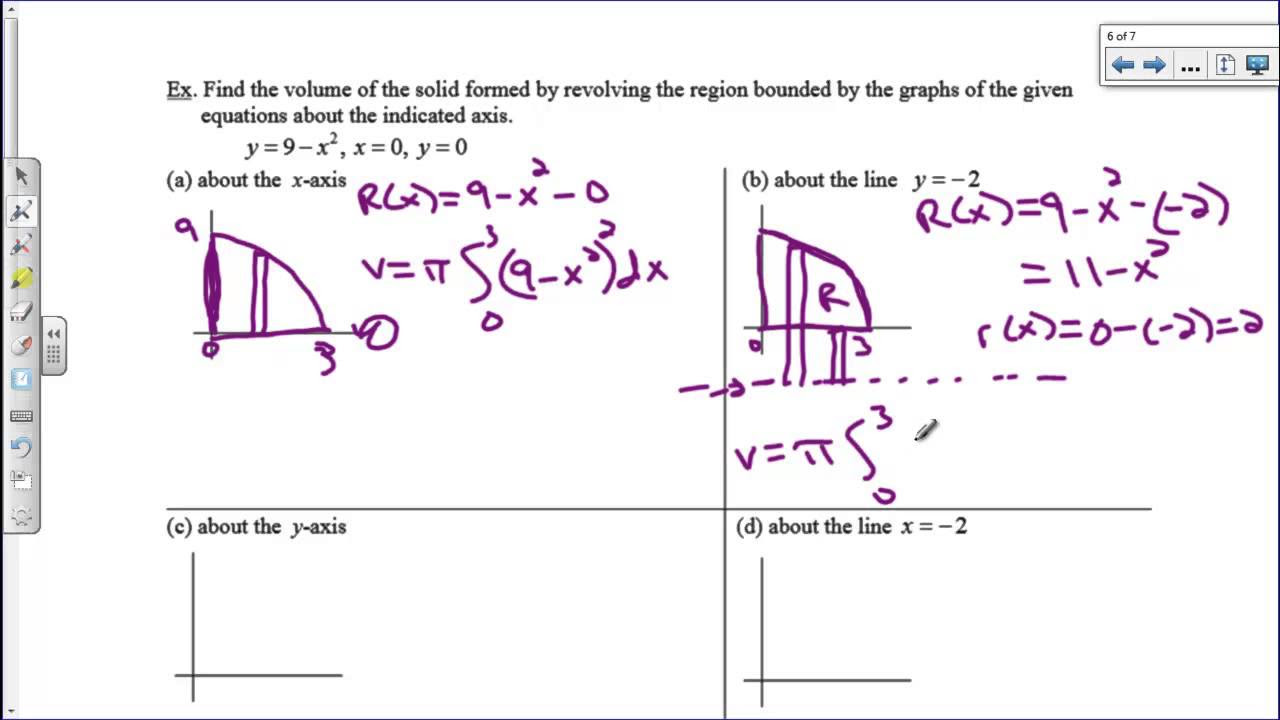
The lecture begins with a brief recap of the previous session, which focused on extending calculus concepts to functions of multiple variables, specifically discussing mountain slopes and functions of two variables. The main topic for the day is the analog of the integral for these functions. The professor introduces the concept of finding volumes of solids created by spinning an area around an axis, a common method that results in objects with rotational symmetry. Examples from everyday life are given to illustrate this concept. The lecture aims to teach how to calculate the volume of objects with rotational symmetry using integration, starting with a specific function, f(x) = x^2, revolving around the x-axis to form a solid. The process involves slicing the solid into discs and summing their volumes, which is the fundamental idea of an integral.

This paragraph delves into the method of calculating the volume of a solid of revolution by considering the function f(x) = x^2, which is revolved around the x-axis to form a solid. The process involves taking vertical slices of the solid at different x-values, creating discs with a radius equal to the function's value at that x. The area of each disc is calculated using the formula for the area of a circle, π times the radius squared, and then integrating over the interval [0, 2] to find the total volume. The integral is computed using the fundamental theorem of calculus, resulting in a volume of 32π/5 cubic units. The paragraph also explores an alternative method of calculating the volume by considering concentric cylinders instead of discs, leading to the same result.

The discussion shifts to a mathematical curiosity involving a solid of revolution that has finite volume but infinite surface area. The function considered is f(x) = 1/x, which is revolved around the x-axis from x=1 to infinity. The volume is calculated using the method of slicing into discs and integrating, resulting in a finite volume of π cubic units. However, when considering the surface area, it is shown that by comparing the actual shape to a series of cylinders with decreasing heights, the surface area of the solid approaches infinity. This paradoxical object has a finite volume that can be filled but an infinite surface area that cannot be fully painted, illustrating the intriguing properties of mathematical objects at infinity.

The lecture moves on to the concept of integrating functions of two variables, drawing an analogy with the area under a curve for functions of one variable. The goal is to find the volume under the graph of a function of two variables, akin to finding the volume of coal on a terrain. The strategy involves breaking the solid into slices parallel to one of the axes and integrating the volume of each slice to find the total volume. The process is demonstrated with a model surface representing a function of two variables, emphasizing the idea of slicing and integrating to find the volume under the surface.

The paragraph explains the strategy for calculating the volume under a surface defined by a function of two variables. The method involves visualizing the surface as a terrain and slicing the solid into pieces parallel to the x-axis or y-axis. Each slice is then treated as a function of one variable, and the volume of each slice is found by integrating over the domain of the other variable. The process is analogous to finding the area under a curve for a function of one variable but extended to two dimensions, resulting in a double integral that gives the total volume under the surface.

This paragraph provides a practical example of using double integrals to calculate the volume of a loaf of bread. The function considered is f(x, y) = x^2y, which represents the height of the surface at each point in the xy-plane. The goal is to find the volume under this surface over a rectangle defined by x ranging from 0 to 2 and y ranging from 0 to 3. The method involves slicing the solid in the y-direction, integrating the area of each slice (which is a parabola) with respect to x, and then integrating the resulting expression with respect to y. The double integral is computed, yielding a total volume of 12 cubic units. The paragraph concludes with a humorous remark that calculus is the best thing since sliced bread, highlighting the practical applications of calculus in everyday life.

The final paragraph presents an alternative method for calculating the volume under the surface defined by f(x, y) = x^2y, this time by slicing with respect to x first and then integrating with respect to y. The process involves fixing a value of x and integrating the function with y as the variable, resulting in an expression that can be integrated again with respect to x. The paragraph demonstrates that regardless of the order of integration (slicing), the final result remains the same, emphasizing the commutative property of double integrals. The lecture concludes with the idea that calculus can be applied to everyday objects, such as a loaf of bread, to understand their volume and other properties.