Lecture 15: The Best of All Possible World Optimization
TLDRThis educational video explores the practical applications of calculus, particularly derivatives, in optimization problems. It illustrates the process with examples like designing a cost-efficient box, maximizing the area enclosed by a given length of fencing, and minimizing the material used in soda can manufacturing. The video emphasizes a strategy involving derivatives to find optimal dimensions for various real-world scenarios, demonstrating how calculus can be used to solve complex problems efficiently.
Takeaways
- 📚 The video discusses practical applications of calculus, focusing on optimization problems involving derivatives.
- 📦 It presents a problem of designing a box to hold eight cubic feet with minimum material cost, introducing the concept of optimizing cost efficiency.
- 🔍 The strategy for solving optimization problems involves considering all possible designs and using derivatives to find the optimal dimensions.
- 📏 An example of optimizing a rectangular enclosure with a given amount of fencing is used to illustrate the process of maximizing an area.
- 🐫 The camel enclosure problem highlights the use of calculus to find the dimensions that maximize the enclosed area with a limited resource.
- 📈 The video explains that the maximum or minimum value in optimization problems often occurs where the derivative of the area function with respect to the variable is zero.
- 🌟 It demonstrates the process of taking the derivative of the area function, setting it to zero, and solving for the variable to find the optimal dimensions.
- 💨 Another example from biology explains how calculus can be used to determine the optimal constriction of the trachea for maximum expulsion of air when coughing.
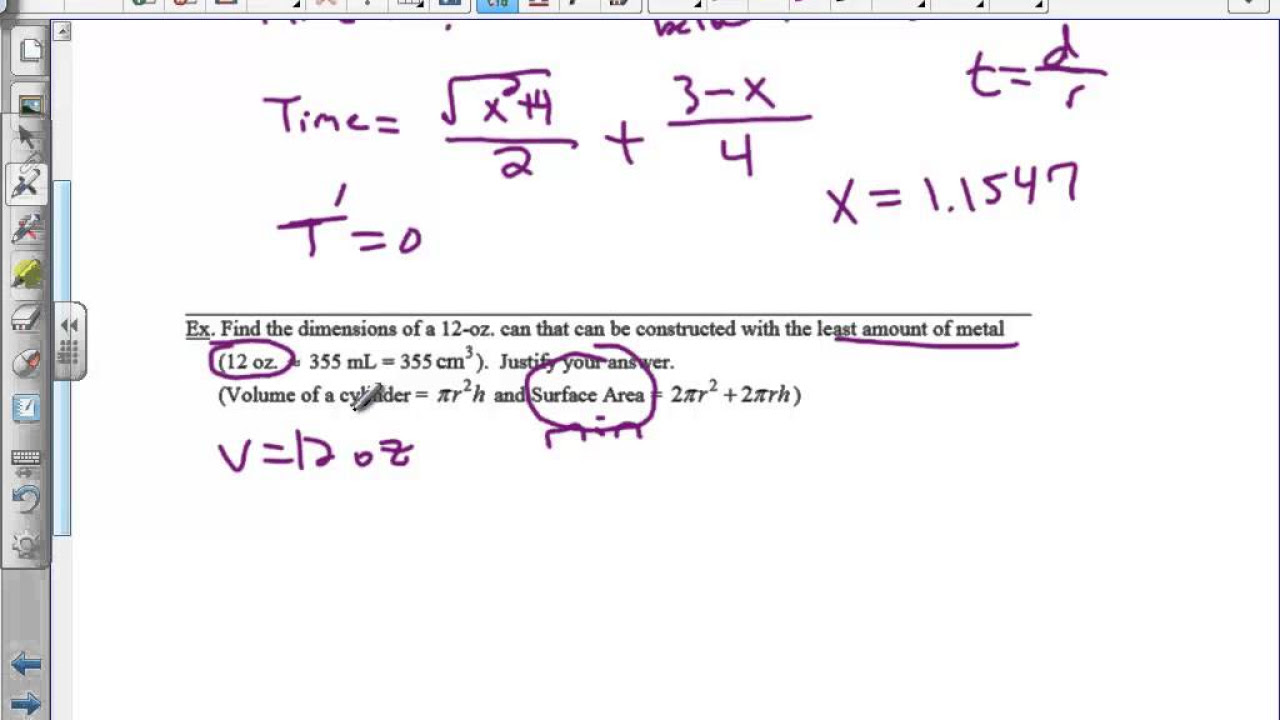
- 🥤 The video also covers the optimization of a soda can's design to contain a specific volume with the least amount of material, using calculus to find the ideal dimensions.
- 📊 The process involves expressing the volume and surface area in terms of a single variable, taking the derivative, and finding where it equals zero to minimize the surface area.
- 🛠 The script concludes by emphasizing the dynamic perspective that calculus offers for analyzing and optimizing real-world problems, with future lectures exploring applications in economics and architecture.
Q & A
What is the main application of calculus discussed in the video?
-The main application of calculus discussed in the video is optimization, specifically using derivatives to find the most efficient or cost-effective solutions to practical problems.
What is the problem presented in the video regarding building a box?
-The problem is to determine the shape of a box that can hold eight cubic feet of volume with the minimum cost of materials, given different costs per square foot for the bottom, sides, and top.
What is the strategy used in the video to find the optimal dimensions for enclosing the maximum area with a given amount of fencing?
-The strategy involves imagining all possible shapes for the enclosure, introducing a variable to represent one dimension, and using calculus to find where the derivative of the area function with respect to this variable is zero, indicating a maximum or minimum.
Why are word problems often challenging for students?
-Word problems are challenging for students because they require translating a verbal description into a mathematical model, which can be difficult when students are unsure of how to set up the problem.
What is the example involving fences, camels, and a river about?
-The example is about using 600 feet of fencing to create a rectangular enclosure next to a river to enclose the maximum area for a herd of camels, with the river forming one side of the enclosure.
How does calculus help in solving the enclosure problem with the camels?
-Calculus helps by allowing us to express the area enclosed by the fence as a function of one variable, then taking the derivative of this function and setting it to zero to find the optimal dimensions that maximize the area.
What is the significance of the derivative being zero in optimization problems?
-The derivative being zero signifies that the slope of the tangent line to the function at that point is horizontal, which corresponds to a maximum or minimum value of the function, depending on the context of the problem.
Can you provide an example of optimization in biology mentioned in the video?
-An example from biology is optimizing the constriction of the trachea to expel air at the fastest velocity, which is done by contracting the trachea to two-thirds of its resting radius.
What is the practical problem discussed in the video related to soda cans?
-The practical problem is designing a soda can that contains a certain volume, such as 12 ounces, using the least amount of material, specifically minimizing the surface area of the cylindrical can.
Why might soda cans not be designed in the optimal shape suggested by calculus?
-Soda cans might not be designed in the optimal shape suggested by calculus due to other considerations such as manufacturing processes, the need to cut the top from a rectangular sheet of aluminum, and the resulting wastage.
Outlines
📚 Introduction to Optimization with Calculus
The video script begins by introducing the concept of optimization using calculus, specifically derivatives. The host presents a practical problem involving the construction of a box with minimum material cost to hold a specific volume. This sets the stage for exploring optimization in various real-world applications, such as maximizing the area enclosed by a given length of fencing or minimizing the material used to create a soda can. The script emphasizes the importance of word problems and the strategy of using derivatives to find optimal solutions, which is the central theme of the lecture.
🔍 Optimizing the Area with a Given Amount of Fencing
The script delves into the problem of maximizing the area enclosed by 600 feet of fencing, with one side being a river. The strategy involves considering all possible shapes of the enclosure and using calculus to find the dimensions that yield the maximum area. The host explains that instead of examining the area for each configuration, the focus should be on how the area changes with each design choice. The process involves taking the derivative of the area function with respect to the variable representing the width of the enclosure, setting it to zero to find the optimal dimensions, and confirming that this indeed maximizes the area.
📉 Derivatives and the Strategy for Maximizing Area
The script explains the mathematical approach to finding the maximum area using derivatives. By introducing a variable 'w' for the width of the enclosure, the host formulates an equation for the area as a function of 'w'. The graph of this function is analyzed to identify the maximum point, where the derivative is zero. The process involves taking the derivative of the area function, setting it to zero, and solving for 'w'. The script confirms the maximum area by showing that any deviation from the optimal 'w' results in a decrease in area, thus validating the strategy of using calculus for optimization.
🌪️ Applying Optimization to Biological and Business Problems
The script extends the concept of optimization to a biological context, discussing how the velocity of air expelled from the mouth is maximized by constricting the trachea to two-thirds of its resting radius. This natural phenomenon is confirmed by X-ray photographs of people coughing. The host then transitions to a business-related problem, focusing on designing a soda can that contains a specific volume with the least amount of material. The script outlines the mathematical approach to finding the optimal dimensions of the can, emphasizing the practical applications of calculus in various fields.
🛍️ Designing the Soda Can with Minimal Material Usage
The script presents a detailed example of designing a soda can to contain 12 ounces of liquid using the least amount of material. The host provides the mathematical formulas for the volume and surface area of a cylindrical can and sets up an equation to solve for the optimal radius 'r' that minimizes the surface area. The process involves taking the derivative of the surface area function with respect to 'r', setting it to zero, and solving for the optimal radius. The script concludes with the optimal dimensions for the soda can and a humorous suggestion to produce such cans, highlighting the practicality of the optimization strategy.
🏭 Considerations for Real-World Application of Optimization
The script concludes by acknowledging that while the optimization strategy using calculus provides the theoretical minimum, real-world applications may involve additional considerations such as manufacturing processes and material wastage. The host explains that the top of a soda can must be cut from a rectangular sheet of aluminum, which affects the optimization. The script emphasizes that while calculus offers a powerful tool for finding optimal solutions, practical constraints can alter the final outcome.
Mindmap
Keywords
💡Optimization
💡Derivatives
💡Word Problems
💡Cost Efficiency
💡Fencing Problem
💡Cylinder
💡Surface Area
💡Volume
💡Trachea
💡Velocity
Highlights
Introduction to the practical applications of calculus, specifically the use of derivatives in optimization problems.
Exploring the problem of minimizing material costs for a box with a fixed volume, illustrating the real-world application of calculus.
The importance of word problems in calculus and their impact on students, often considered challenging but crucial for understanding.
Optimization strategy using derivatives to find the design that optimizes a feature, such as cost efficiency in the box example.
Examples of optimization problems, including maximizing the area enclosed by a given length of fencing and minimizing material use in soda can design.
The problem of fencing camels next to a river with a limited amount of fencing material, maximizing the enclosed area.
The strategy of imagining all possible enclosure shapes and using calculus to find the dimensions that maximize the area.
Using derivatives to understand how area changes with different design choices and finding the optimum through calculus.
The process of taking the derivative of the area function with respect to the width and setting it to zero to find the maximum area.
Confirmation of the maximum area solution by comparing areas with slightly altered dimensions.
Application of optimization in biology, specifically the velocity of air expelled from the mouth based on trachea constriction.
Finding the optimal constriction level of the trachea for maximum expulsion velocity using calculus.
Designing a soda can to contain a specific volume using the least amount of material, an example of optimization in business.
The mathematical formulation for the volume and surface area of a cylindrical soda can and the optimization process.
Deriving the optimal dimensions for the soda can that minimizes surface area for a given volume.
Practical considerations that affect the shape of soda cans, such as manufacturing and material wastage.
The dynamic perspective that calculus offers for optimization problems and its broad applications across different fields.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: