Conservation of Energy (Learn to solve any problem)
TLDRThis educational video script explores the concept of conservative forces and energy conservation. It explains that conservative forces, such as gravity and spring force, do work dependent only on initial and final positions, not the path taken. The script delves into kinetic and potential energy, highlighting their roles in the conservation of energy equation. Practical examples, including a rollercoaster problem and a spring scenario, illustrate how to apply these principles to solve physics problems, emphasizing the importance of understanding energy transformations in various mechanical systems.
Takeaways
- 🔄 A conservative force is one where the work done is independent of the path taken and depends only on the initial and final positions.
- 📦 Friction is an example of a non-conservative force as its work is path-dependent, unlike gravity or spring force which are conservative.
- 🏋️♂️ Kinetic energy represents the energy of motion, requiring work to accelerate a particle from rest to a certain velocity.
- ⏳ Potential energy is the stored energy of an object due to its position, measured by the work done by a conservative force to move it to a reference position (datum).
- 📈 Gravitational potential energy is calculated as the weight of an object times the vertical distance from the datum, with positive and negative values based on the object's position relative to the datum.
- 🔄 Elastic potential energy is the energy stored in a spring when it is compressed or stretched, calculated using Hooke's Law (K * x^2, where K is the spring constant and x is the displacement).
- 🌐 The conservation of energy principle states that the total mechanical energy (sum of kinetic and potential energies) of a closed system remains constant if non-conservative forces are negligible.
- 🎢 In the rollercoaster example, the minimum height of the initial hill and the normal forces at points B and C can be determined using the conservation of energy equation and considering the car's velocity and normal acceleration.
- 🤔 The minimum velocity required for a rollercoaster car to stay on the track at a loop is found by setting the normal force to zero and solving for velocity using the equation of motion.
- 🔍 To find the stiffness of a spring, one can use the conservation of energy equation, taking into account the initial and final positions of an object and the work done by gravity and the spring force.
- 🔄 In a pulley system, the conservation of energy can be applied to find the distance one block must descend for another to reach a certain speed, considering the system's initial and final kinetic and potential energies.
Q & A
What is a conservative force according to the script?
-A conservative force is one where the work done is independent of the path taken and depends only on the initial and final positions. Examples include gravitational force and spring force.
Why is friction considered a non-conservative force?
-Friction is a non-conservative force because the work done by friction depends on the length of the path traveled, not just the initial and final positions.
What is the definition of energy mentioned in the script?
-Energy is defined as the capacity for doing work. It can be kinetic, which is related to the motion of an object, or potential, which is stored energy based on an object's position or condition.
What is kinetic energy and how is it calculated?
-Kinetic energy is the energy an object possesses due to its motion. It is calculated as half times the mass of the object times the velocity squared (\( \frac{1}{2}mv^2 \) ).
Can you explain the concept of potential energy as described in the script?
-Potential energy is the stored energy of an object based on its position relative to other objects or forces. It can be gravitational potential energy, which is the energy due to an object's height above a reference point, calculated as mass times gravity times height above the datum.
What is the significance of the datum in potential energy calculations?
-The datum is a reference level from which potential energy is measured. It helps determine whether the potential energy is positive (above the datum) or negative (below the datum).
How is elastic potential energy related to the spring force?
-Elastic potential energy is related to the spring force by the equation \( PE = \frac{1}{2}Kx^2 \), where \( K \) is the spring's stiffness constant and \( x \) is the displacement from the equilibrium position.
What is the conservation of energy equation, and how is it used in the script?
-The conservation of energy equation states that the total mechanical energy (sum of kinetic and potential energy) of a closed system remains constant. In the script, it is used to solve problems involving changes in an object's energy state, such as in the rollercoaster problem.
How does the script use the conservation of energy to solve the rollercoaster problem?
-The script applies the conservation of energy to find the minimum height of the initial hill and the normal force at points B and C of the rollercoaster. It considers the initial and final kinetic and potential energies to determine these values.
What is the minimum velocity required for the car in the rollercoaster problem to stay on the track at point B?
-The minimum velocity required for the car to stay on the track at point B is calculated using the conservation of energy and the normal force being zero at the top of the loop.
How does the script determine the stiffness of the inner spring in the spring problem?
-The script uses the conservation of energy equation, considering the initial and final states of the system, including the gravitational potential energy and the elastic potential energy of both springs, to solve for the unknown stiffness of the inner spring.
In the pulley problem, how is the distance that Block B must descend related to the speed of Block A?
-The script relates the descent of Block B to the speed of Block A using the conservation of energy and the fixed length of the middle bar in the pulley system. It finds the distance Block B descends when Block A reaches a certain speed by considering the change in potential energy for both blocks.
Outlines
🔁 Understanding Conservative Forces and Energy Conservation
This paragraph introduces the concept of conservative forces, which are characterized by their work being path-independent, relying solely on initial and final positions. The paragraph provides examples such as friction, which is non-conservative due to its path-dependency, and gravity, which is conservative since the work done is independent of the path taken. It also explains the types of potential energy, including gravitational and elastic potential energy, and their relationship with conservative forces. The paragraph culminates in the introduction of the conservation of energy equation, which is fundamental for solving problems involving energy transformations between kinetic and potential forms.
🎢 Applying Energy Conservation to a Roller Coaster Problem
The second paragraph delves into a practical application of the conservation of energy principle using a roller coaster scenario. It describes the process of determining the minimum height required for a roller coaster to successfully navigate loops, considering the normal force and the minimum velocity needed at critical points. The paragraph outlines the steps to calculate these values, including the use of a free-body diagram and equations of motion for the normal axis. It also demonstrates how to apply the conservation of energy to find the velocities and heights necessary for the roller coaster to maintain contact with the track throughout its course.
🔧 Solving Spring and Pulley System Problems with Energy Conservation
The final paragraph extends the application of energy conservation to solve problems involving springs and pulley systems. It first discusses how to find the stiffness of a spring given a specific compression distance, using the conservation of energy equation. The paragraph then moves on to a pulley system problem, where the goal is to determine the descent distance of one block to achieve a certain velocity for another. The explanation includes setting up a datum, calculating displacements, and using the conservation of energy to relate the velocities and displacements of the blocks. The summary emphasizes the importance of considering both positive and negative potential energy changes in these mechanical systems.
Mindmap
Keywords
💡Conservative Force
💡Non-conservative Force
💡Kinetic Energy
💡Potential Energy
💡Conservation of Energy
💡Gravitational Potential Energy
💡Elastic Potential Energy
💡Normal Force
💡Datum
💡Equation of Motion
Highlights
Definition of a conservative force: A force is conservative if the work done is independent of the path taken and depends only on initial and final positions.
Friction as a non-conservative force example: Work done by friction increases with the length of the path.
Gravitational force as a conservative force: Work done by gravity is path-independent and depends on initial and final positions of a particle.
Spring force as a conservative force: Work done by a spring is dependent only on its elongation or compression.
Concept of energy as the capacity for doing work, including kinetic and potential energy.
Kinetic energy explained: The work needed to accelerate a particle from rest to a certain speed.
Gravitational potential energy defined: The energy possessed by a particle due to its position in a gravitational field.
Potential energy's relation to conservative forces: It measures the work done by a conservative force moving from a given position to a datum.
Significance of positive and negative potential energy based on the position of a particle relative to the datum.
Elastic potential energy formula introduced: The energy stored in a spring when it is compressed or stretched.
Conservation of energy equation: The sum of initial kinetic and potential energy equals the sum of final kinetic and potential energy.
Rollercoaster problem example: Using conservation of energy to find the minimum height of a hill and normal force at specific points.
Importance of considering normal acceleration in circular motion problems, especially in loops.
Equation of motion for normal axis in circular motion: Relates normal force, weight, and normal acceleration.
Minimum velocity calculation for a rollercoaster to stay on track at specific points using conservation of energy.
Verification of sufficient velocity for a rollercoaster to pass through loops using energy conservation.
Calculation of normal force on a rollercoaster car at specific points using motion equations.
Spring problem example: Determining the stiffness of a spring using conservation of energy principles.
Pulley system problem: Finding the distance one block must descend for another to reach a certain speed.
Use of position coordinates and conservation of energy in a pulley system to solve for block displacement.
Final advice on solving energy conservation problems by considering initial and final kinetic and potential energies.
Transcripts
Browse More Related Video
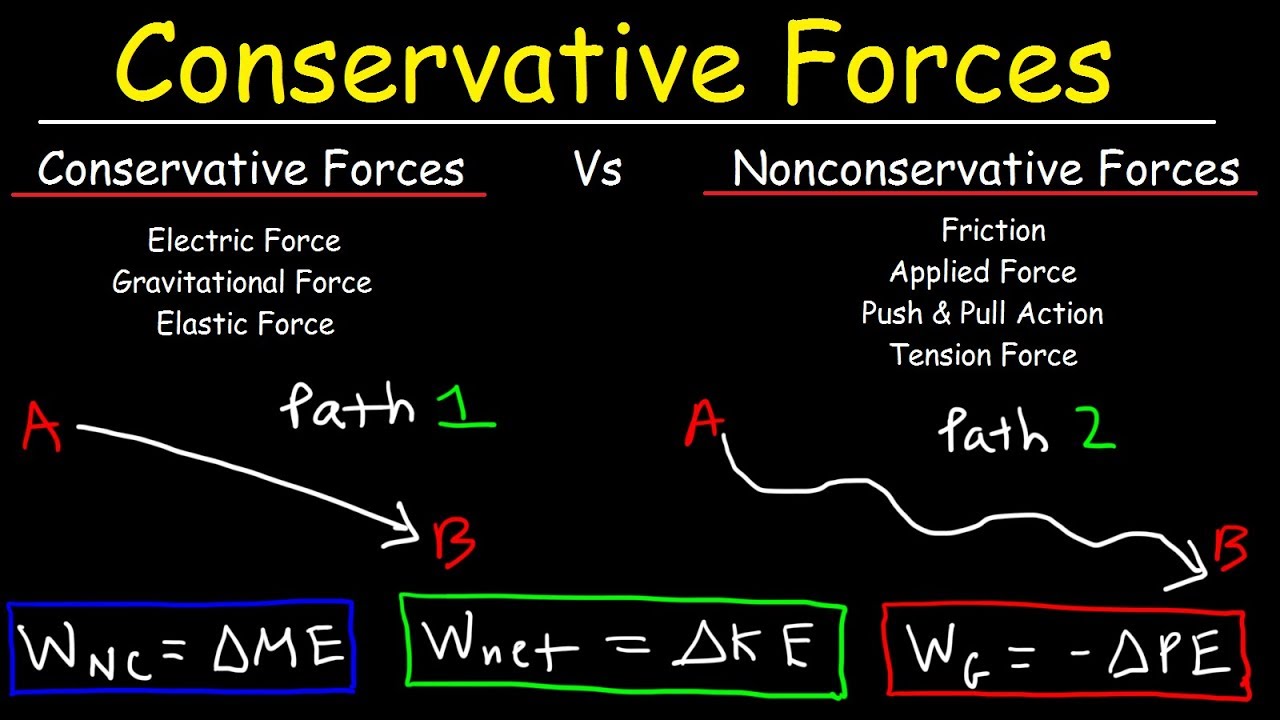
Conservative & Nonconservative Forces, Kinetic & Potential Energy, Mechanical Energy Conservation

Work and Energy

8.01x - Lect 11 - Work, Kinetic & Potential Energy, Gravitation, Conservative Forces
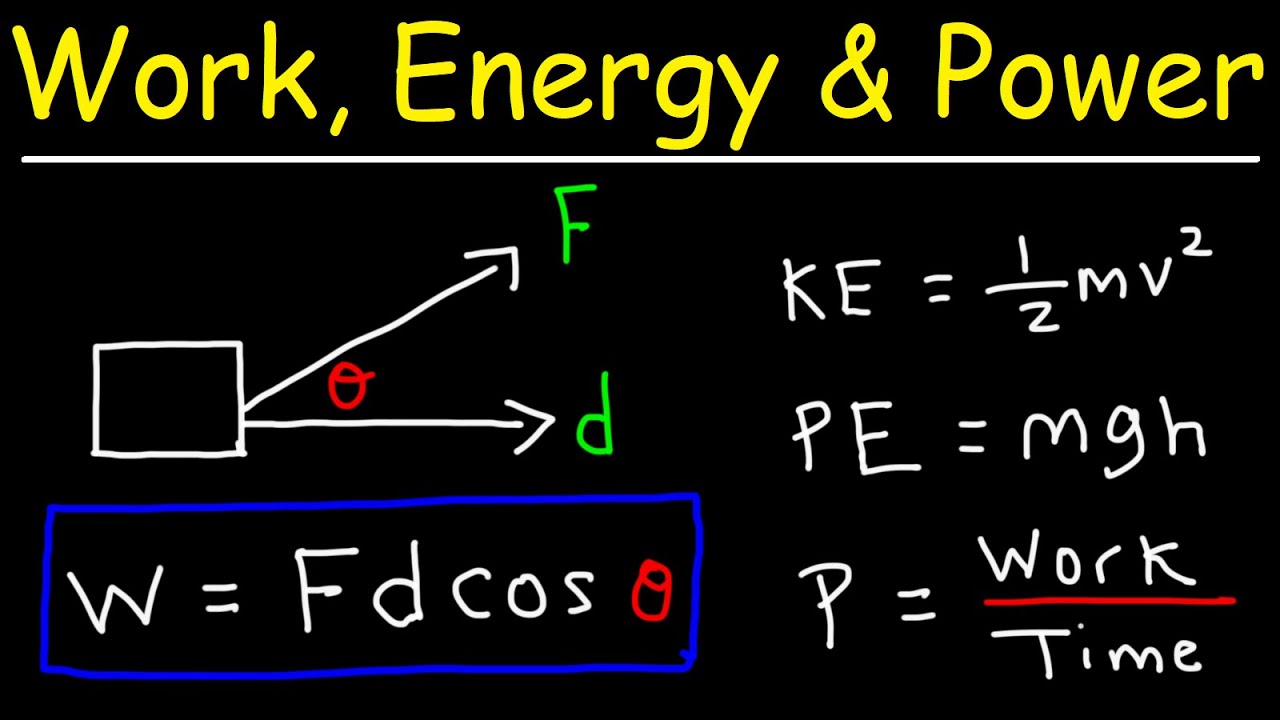
Work, Energy, and Power - Basic Introduction
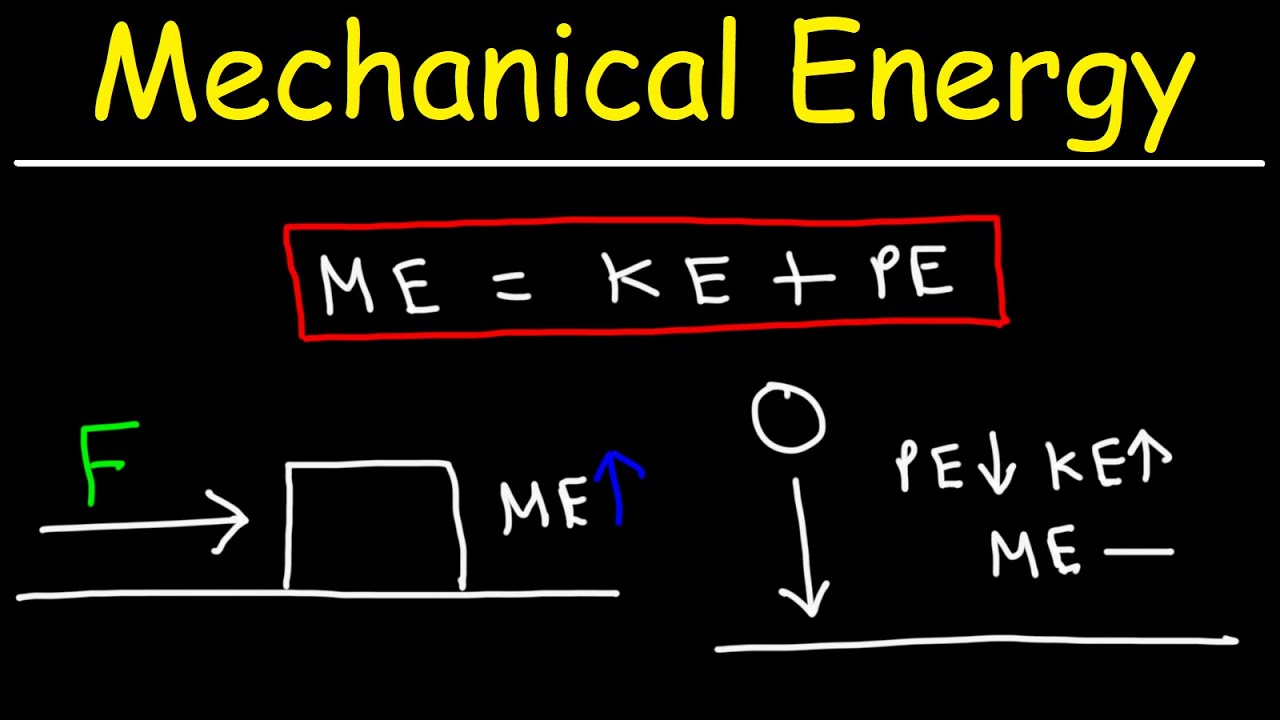
Mechanical Energy - Basic Overview

AP Physics C: Work, Energy, and Power Review (Mechanics)
5.0 / 5 (0 votes)
Thanks for rating: