Mechanical Energy - Basic Overview

The Organic Chemistry Tutor

29 Jul 202321:50

EducationalLearning

32 Likes 10 Comments

TLDRThis lesson delves into the concept of mechanical energy, explaining it as the sum of kinetic and potential energy. Kinetic energy is associated with motion, while potential energy is related to an object's position or condition. The script illustrates the conservation of mechanical energy when only conservative forces, such as gravity, are at play, and how mechanical energy can change when non-conservative forces are involved. It provides examples, including a block on a frictionless surface and a ball thrown from a cliff, to demonstrate calculations of mechanical energy, kinetic energy, and the work done by various forces.

Takeaways

📚 Mechanical energy is the sum of kinetic and potential energy.
🏃 Kinetic energy is the energy of motion, given by the formula KE = 0.5 * m * v^2, where m is mass and v is velocity.
📈 Gravitational potential energy is given by PE = m * g * h, where m is mass, g is the acceleration due to gravity, and h is height above a reference point.
🔄 In a system with only conservative forces, like gravity, the mechanical energy remains constant as potential energy converts to kinetic energy and vice versa.
🚫 Non-conservative forces, such as applied forces, can increase or decrease the mechanical energy of an object.
💥 When work is done on an object by a net force, it results in a change in kinetic energy.
🔧 The work done by conservative forces like gravity is equal to the negative change in potential energy.
📊 The mechanical energy of an object can be calculated by adding its kinetic and potential energies.
🎯 The work done by an applied force on an object is equal to the change in the object's mechanical energy.
🛠 To calculate the final speed and height of a falling object, one can use the equations of motion considering the initial conditions and gravitational acceleration.
📝 Practice problems help to understand the application of concepts related to mechanical energy, forces, and motion.

Q & A

What is mechanical energy?
-Mechanical energy is the sum of kinetic energy and potential energy. It is a measure of the total energy that an object possesses due to its motion and position.
What is kinetic energy?
-Kinetic energy is the energy of motion. An object that is moving possesses kinetic energy, which can be calculated using the formula KE = 0.5 * m * v^2, where m is the mass and v is the velocity of the object.
How is gravitational potential energy calculated?
-Gravitational potential energy is calculated using the formula PE = m * g * h, where m is the mass of the object, g is the acceleration due to gravity, and h is the height of the object above a reference point.
What happens to the mechanical energy of an object when only conservative forces are acting on it?
-When only conservative forces, such as gravity, act on an object, the mechanical energy of the object remains constant. This is because the potential energy and kinetic energy can convert into each other without any loss or gain in the total mechanical energy.
What is a non-conservative force?
-A non-conservative force is a force that does not conserve mechanical energy in a system. An example of a non-conservative force is friction or an applied force that does work on an object, leading to a change in the total mechanical energy of the system.
How does the work done by an applied force relate to the mechanical energy of an object?
-The work done by an applied force is equal to the change in the mechanical energy of an object. If an object was initially at rest and an applied force does work on it, the total mechanical energy of the object will increase by the amount of work done.
What is the relationship between work, kinetic energy, and potential energy?
-The work done by the net force on an object is equal to the change in the object's kinetic energy, while the work done by conservative forces like gravity is equal to the negative change in potential energy. The total work done in a system is related to the change in mechanical energy.
How can you calculate the final speed of a block that has been moved by an applied force?
-To calculate the final speed of a block moved by an applied force, you first need to determine the acceleration using Newton's second law (F_net = m * a). Then, use the formula v_final = v_initial + a * t, where v_initial is the initial speed, a is the acceleration, and t is the time the force is applied.
What is the formula to calculate the height an object is lifted by an upward force?
-The height an object is lifted can be calculated using the formula d = v_initial * t + 0.5 * a * t^2, where v_initial is the initial vertical speed, a is the acceleration in the vertical direction, and t is the time the force is applied.
How can you find the mechanical energy of a ball thrown downward from a height?
-To find the mechanical energy of a ball thrown downward from a height, you calculate the kinetic energy using the formula KE = 0.5 * m * v^2 and the potential energy using PE = m * g * h. Then, add the kinetic and potential energies to get the total mechanical energy of the ball.
What happens to the potential energy of an object when it falls?
-As an object falls, its height decreases, which leads to a decrease in gravitational potential energy. However, as the object gains speed, its kinetic energy increases. The potential energy is converted into kinetic energy, maintaining the constant total mechanical energy of the object if only conservative forces are acting on it.

Outlines

00:00

🌟 Understanding Mechanical Energy

This paragraph introduces the concept of mechanical energy, which is the sum of kinetic and potential energy. Kinetic energy is the energy of motion, calculated as 0.5 * m * v^2, where m is the mass and v is the velocity of the object. Potential energy, specifically gravitational potential energy, is given by m * g * h, where m is mass, g is the acceleration due to gravity, and h is the height above a reference point. The example of a ball released at rest illustrates the conservation of mechanical energy, where potential energy is converted into kinetic energy as the ball falls, maintaining a constant total mechanical energy due to the conservative force of gravity.

05:01

📈 Calculating Mechanical Energy with Non-Conservative Forces

This section discusses the impact of non-conservative forces on mechanical energy. An applied force, such as pushing a block across a frictionless surface, is an example of a non-conservative force. The mechanical energy of the block increases because the force does work on the block, leading to an increase in kinetic energy while potential energy remains constant. The work done by the applied force is calculated as the force times the displacement, resulting in 2000 Joules in this case. The final kinetic and mechanical energy of the block are also calculated, confirming the increase in energy due to the work done by the non-conservative force.

10:02

🚀 Mechanical Energy Calculation with Given Conditions

The paragraph presents a problem involving a 10 kg ball thrown downward from a 700-meter cliff. The mechanical energy of the ball is calculated by summing its kinetic and potential energies. Kinetic energy is derived from the ball's mass and velocity, while potential energy is based on its mass, gravitational acceleration, and height. The total mechanical energy is found to be 69,580 Joules, highlighting that the majority comes from potential energy in this scenario.

15:08

🔢 Analyzing Block's Motion and Energy with Tension Force

This part examines the motion and energy changes of a 15 kg block when an upward tension force of 500 N is applied for 5 seconds. The block's upward acceleration is calculated using the net force and mass. The vertical speed and height of the block after 5 seconds are then determined using kinematic equations. The total mechanical energy of the block is calculated by adding the kinetic energy, found using the vertical speed and mass, to the potential energy, which is determined by the height and gravitational force. The work done by the net force and the applied force is also discussed, showing that it equals the change in kinetic and mechanical energy, respectively.

20:08

🔄 Work-Energy Principles and their Implications

The final paragraph delves into the work-energy principles, explaining how the work done by conservative forces like gravity is equal to the negative change in potential energy, and how the work done by the net force is equal to the change in kinetic energy. The example provided shows the work done by gravity and the applied force, and how these relate to changes in potential and mechanical energy, reinforcing the concept that work is a measure of energy transfer.

Mindmap

Keywords

💡Mechanical Energy

Mechanical energy is defined as the sum of kinetic and potential energy present in a system. This concept is central to the lesson, which explains the dynamics of energy transformation within physical systems. For instance, the video describes how a ball thrown downwards or a block moved horizontally changes its mechanical energy due to the conversion between kinetic and potential energies, under the influence of conservative or non-conservative forces.

💡Kinetic Energy

Kinetic energy is the energy possessed by an object due to its motion. It is calculated with the formula one-half MV squared (1/2 mv^2), where 'm' is mass and 'v' is velocity. The lesson uses examples like a moving car or a block sliding down a hill to illustrate situations where kinetic energy is generated, emphasizing the role of motion in energy dynamics within systems.

💡Potential Energy

Potential energy is the energy stored in an object due to its position or configuration. For example, gravitational potential energy (MGH) involves height 'h', mass 'm', and the gravitational constant 'g'. The script explains how potential energy is intrinsic to situations like a ball held above the ground, highlighting its capacity to do work upon release, thereby converting potential energy into kinetic energy.

💡Conservative Forces

Conservative forces, such as gravity, are forces where the total mechanical energy (sum of kinetic and potential energy) of a system remains constant if only these forces are doing work. The lesson emphasizes that under conservative forces, energy transformations occur without a net loss or gain in mechanical energy, exemplified by gravity acting on a falling object.

💡Non-conservative Forces

Non-conservative forces are those that result in a change in mechanical energy of a system, such as friction or applied forces. The video uses the example of a block being pushed across a frictionless surface with an applied force to demonstrate how these forces can increase the system's mechanical energy, differentiating from conservative forces where mechanical energy is conserved.

💡Work

In physics, work is defined as the force applied over a distance. The video discusses how work done by non-conservative forces (like an applied force) on an object results in an increase in mechanical energy, illustrated by the calculation of work done on a block being moved horizontally which increases its kinetic energy.

💡Energy Transformation

Energy transformation refers to the process of energy changing from one form to another. In the video, this concept is highlighted during the discussion on how gravitational potential energy converts into kinetic energy as an object falls, maintaining constant mechanical energy in a closed system influenced only by conservative forces.

💡Displacement

Displacement in physics refers to the change in position of an object. It is crucial in calculating work done, as seen in the video where the displacement of a block (20 meters) is multiplied by the applied force (100 Newtons) to determine the work done, thereby affecting the mechanical energy of the block.

💡Acceleration

Acceleration is the rate of change of velocity of an object. It is a key factor in determining changes in kinetic energy. The script uses examples like calculating the acceleration of a block when a force is applied (100 Newtons divided by 5 kilograms) to show how it influences the final kinetic energy and mechanical energy of the system.

💡Gravitational Force

Gravitational force is a conservative force that acts on objects due to their mass and the mass of the Earth, drawing them towards each other. In the lesson, it is used to explain how the gravitational force acting on a falling object enables the conversion of potential energy into kinetic energy, maintaining the mechanical energy constant in the absence of non-conservative forces.

Highlights

Mechanical energy is the sum of kinetic and potential energy.

Kinetic energy is the energy of motion, calculated as (1/2)mv^2.

Gravitational potential energy is given by the formula mgh.

When only conservative forces like gravity act on an object, its mechanical energy remains constant.

Potential energy is converted to kinetic energy as an object falls under gravity, keeping mechanical energy constant.

Non-conservative forces like friction can cause mechanical energy to increase or decrease.

Applied force is a non-conservative force that increases an object's mechanical energy when work is done.

Work done on an object is equal to the change in its kinetic energy.

Work done by an applied force results in a change in the object's mechanical energy.

Work done by gravity, a conservative force, equals the negative change in potential energy.

The mechanical energy of an object can be calculated by adding its kinetic and potential energies.

Kinetic energy can be calculated using the final speed, acceleration, and displacement of an object.

The work-energy theorem states that the net work done on an object is equal to its change in kinetic energy.

Conservative forces like gravity convert potential energy to kinetic energy without changing the total mechanical energy.

In a system with only conservative forces, mechanical energy is conserved as potential energy is converted to kinetic energy.

When an object is at rest, the work done on it equals the final kinetic energy.

The work done by a net force is equal to the change in an object's kinetic energy, while the work done by an applied force results in a change in mechanical energy.

Transcripts

Browse More Related Video

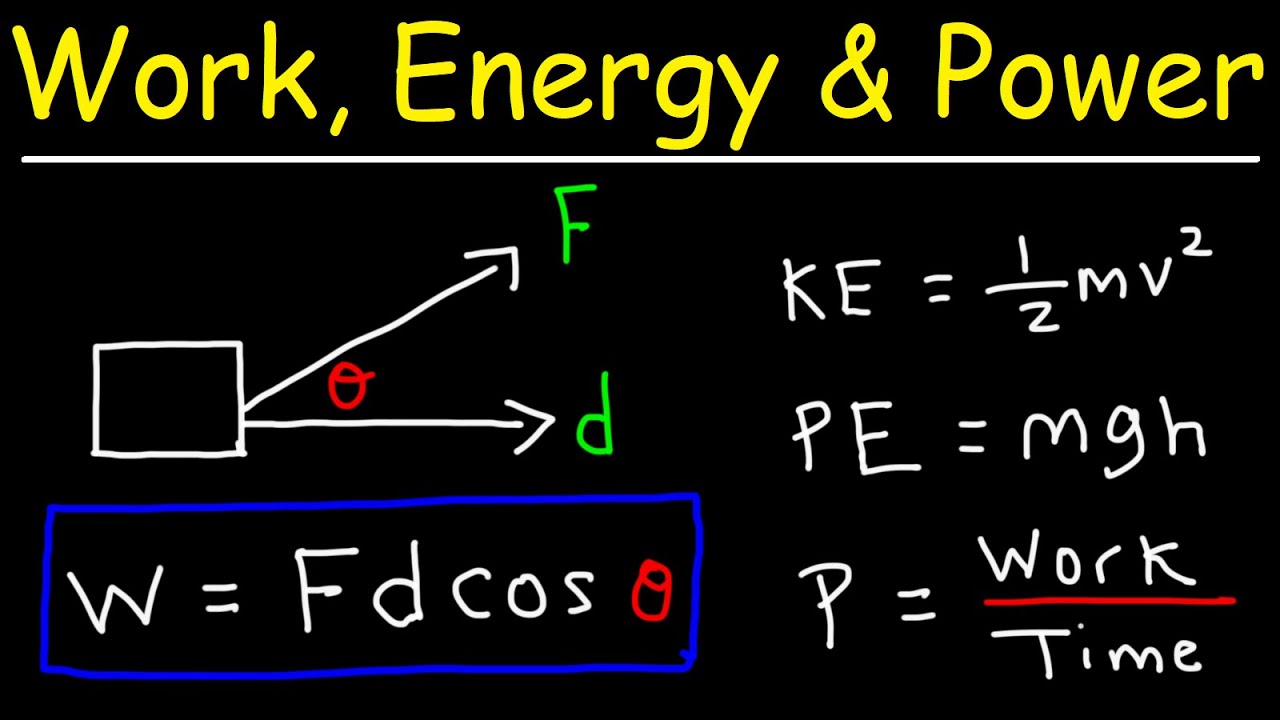
Work, Energy, and Power - Basic Introduction

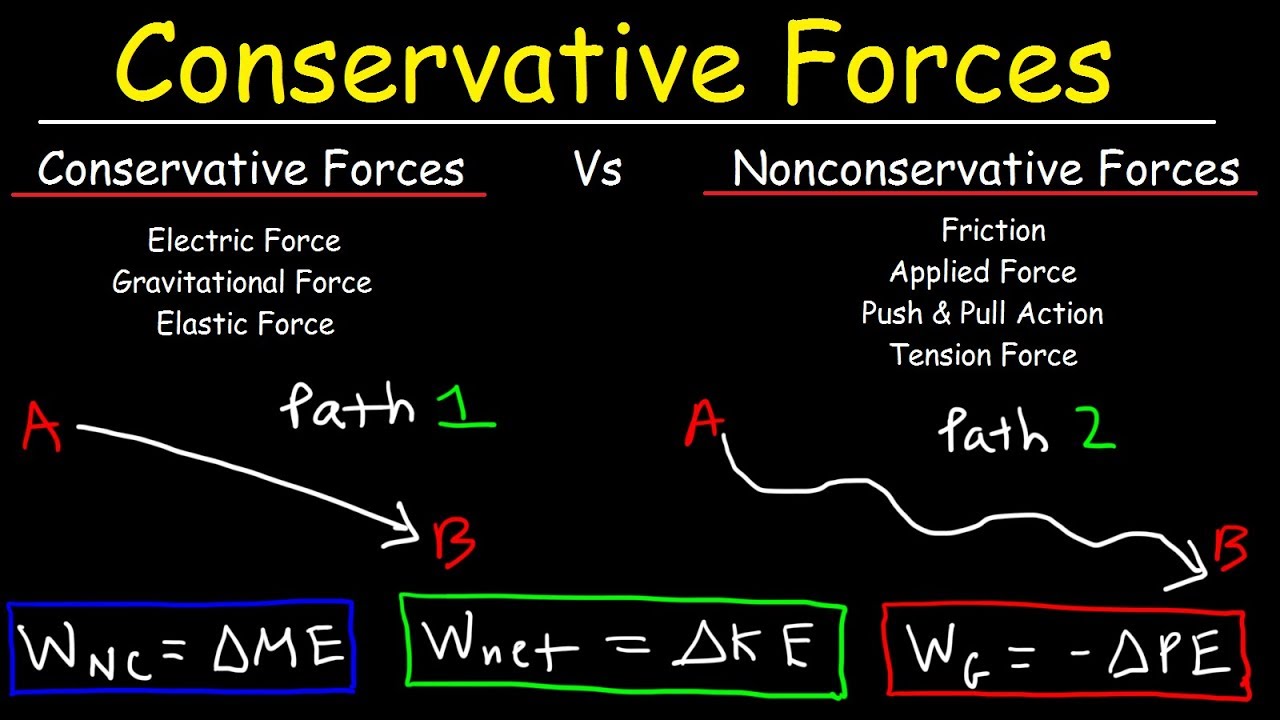
Conservative & Nonconservative Forces, Kinetic & Potential Energy, Mechanical Energy Conservation

Work and Energy

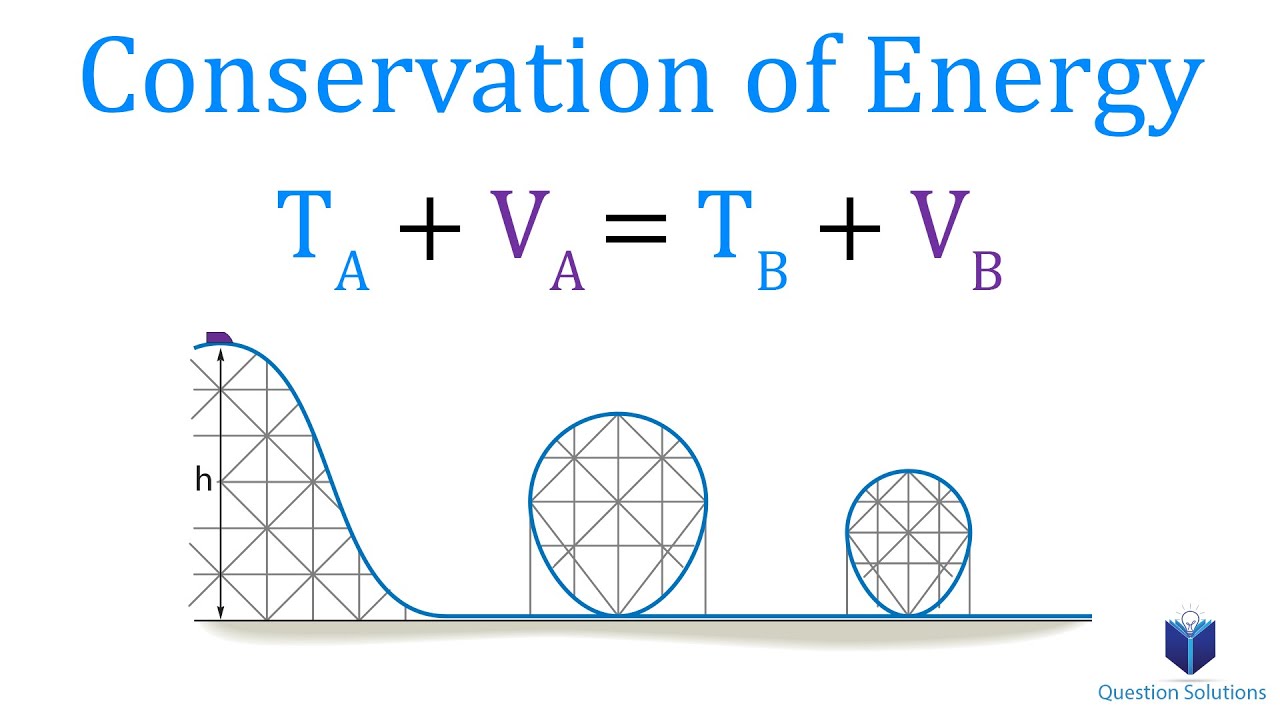
Conservation of Energy (Learn to solve any problem)

8.01x - Lect 11 - Work, Kinetic & Potential Energy, Gravitation, Conservative Forces

Open & Closed Systems in Energy - IB Physics

Related Tags

Mechanical Energy Kinetic Energy Potential Energy Conservation Laws Gravity Friction Physics Concepts Energy Transformation Applied Forces Work Calculation