Spacetime rotations, understanding Lorentz transformations
TLDRThis script from 'Science Click' explores the revolutionary concept of space-time as a four-dimensional framework, challenging traditional notions of space and time. It delves into the relativity of motion, the constancy of the speed of light, and the Lorentz transformations that warp space and time. The script introduces the Minkowski metric, which likens space-time to a map, and discusses how these concepts unify fields, momentum, and energy, leading to a deeper understanding of acceleration and gravity within the universe.
Takeaways
- 🌐 We live in a geometric space with three spatial dimensions and one temporal dimension, creating a four-dimensional space-time continuum.
- 📏 Frames of reference are unique perspectives from which we measure and observe the universe, with each observer potentially seeing different motion states.
- 🚗 The concept of relative motion is exemplified by a car moving on a street, where the car is stationary in the driver's frame but moving in the frame of a bystander.
- 🔄 Galilean transformation allows for a shift in perspective by sliding space slices to reposition the observer at the center of the universe.
- 💡 The constancy of the speed of light was a groundbreaking discovery, challenging the classical addition of velocities and introducing the absolute nature of light speed in all frames of reference.
- 🌀 The Lorentz transformation addresses the paradox of light speed by adjusting not only spatial but also temporal dimensions, leading to the warping of space-time.
- ⏱️ Time dilation and length contraction are consequences of special relativity, where moving clocks appear to tick slower and lengths contract in the direction of motion.
- 📊 Minkowski and Poincaré introduced the Minkowski metric, offering a new way to measure distances in space-time, akin to how a map represents the Earth's surface.
- 🔄 Hyperbolic rotations, or Lorentz transformations, are analogous to spatial rotations but act on the space-time fabric, distinguishing between time-like and space-like intervals.
- 🚀 The speed of light represents an infinite rapidity, the theoretical maximum speed attainable after infinite acceleration, highlighting the unattainable nature of light speed for objects with mass.
- 🔧 Space-time is not just an abstract concept but a physical entity with its own geometry, which has been instrumental in unifying concepts like electric and magnetic fields, as well as momentum and energy.
Q & A
What is a frame of reference and why is it important in understanding the universe?
-A frame of reference is a set of axes or coordinates that provide a basis for describing the motion and position of objects in the universe. It is important because it allows us to locate objects and measure distances and angles relative to a specific point of view, which is essential for understanding the dynamics of the universe.
How did the concept of time as a fourth dimension revolutionize our understanding of the universe?
-The recognition of time as a fourth dimension, along with the three spatial dimensions, led to the unification of space and time into a single continuum known as space-time. This concept is central to the theory of relativity, which has fundamentally changed our understanding of how motion, energy, and momentum are perceived differently by observers in different frames of reference.
What is a space-time diagram and how is it constructed?
-A space-time diagram is a graphical representation that combines time and space into a single framework. It is constructed by freezing each instant of time and stacking the images on top of one another to form a block, which represents the flow of time. Each slice of the block represents a moment in time, and the paths traced by objects within the diagram represent their world lines through space-time.
How does a Galilean transformation differ from a Lorentz transformation?
-A Galilean transformation involves shifting the slices of space to change the frame of reference, which is suitable for classical mechanics where velocities add linearly. In contrast, a Lorentz transformation not only shifts slices of space but also slices of time to account for the constancy of the speed of light in all frames of reference, which is a key feature of special relativity.
Why is the speed of light considered absolute in the context of special relativity?
-The speed of light is considered absolute because it is always measured to be the same value, 299,792,458 meters per second, in all inertial frames of reference. This constancy is a fundamental postulate of special relativity and has led to the understanding that time and space must be relative to accommodate this phenomenon.
What is the concept of time dilation in special relativity?
-Time dilation is a phenomenon in special relativity where the passage of time is perceived differently by observers in different frames of reference. Specifically, a clock moving relative to an observer will appear to tick slower than a clock at rest with respect to that observer.
What is length contraction and how does it relate to the motion of objects?
-Length contraction is the phenomenon where an object's length appears shorter when it is moving relative to an observer. It occurs due to the relative motion between the object and the observer and is another consequence of the Lorentz transformations in special relativity.
How do Minkowski and Poincaré contribute to our understanding of space-time?
-Minkowski and Poincaré introduced the concept of the Minkowski space-time, which is a mathematical model that combines space and time into a four-dimensional manifold. They proposed the Minkowski metric, which allows for a new way of measuring distances within the space-time diagram, making it possible to understand Lorentz transformations as a form of 'hyperbolic rotation'.
What is the Minkowski metric and how does it relate to hyperbolic rotations?
-The Minkowski metric is a method of measuring distances in space-time that preserves the geometry of space-time even when changing reference frames. It allows for the analogy of hyperbolic rotations, which are transformations that mix space and time in a way that preserves the speed of light and the geometry of space-time.
How does the theory of special relativity unify concepts like electric and magnetic fields?
-Special relativity shows that electric and magnetic fields are two aspects of the same electromagnetic force, and that these fields transform into each other under changes in the frame of reference. The electric field can be seen as the time component of a four-dimensional electromagnetic field, while the magnetic field is the space component.
What is the significance of the speed of light in the context of space-time and rapidity?
-The speed of light is significant because it represents an infinite rapidity, or the maximum theoretical speed that an object could reach if it were to accelerate indefinitely. It is the upper limit of velocity in the universe and is invariant in all frames of reference, which has profound implications for the geometry of space-time.
How does the concept of space-time relate to the phenomenon of gravity as described by general relativity?
-In general relativity, the presence of mass and energy distorts space-time, causing what we perceive as gravity. This is a direct consequence of the fact that space-time is not a flat, unchanging entity but a dynamic medium that can be warped by the distribution of mass and energy within it.
Outlines
🌌 Understanding Frames of Reference and the Fourth Dimension
This paragraph introduces the concept of frames of reference in the universe, where each person perceives the world from a unique vantage point. It explains how motion is relative and can be described differently depending on the observer. The paragraph then delves into the revolutionary idea that time is the fourth dimension, challenging the traditional three-dimensional perspective. The video aims to explain how this concept has led to the unification of space, time, and other physical phenomena like electric and magnetic fields, as well as momentum and energy.
🔄 The Paradox of Light Speed and Lorentz Transformations
The second paragraph explores the paradox of the speed of light, which remains constant in all reference frames, contrary to the classical addition of velocities. It describes the thought experiment involving two observers, A and B, where light emitted from both moves at the same speed, leading to the necessity of Lorentz transformations. These transformations adjust not only space but also time to maintain the constancy of light speed, introducing the relativity of time and space measurements. The paragraph also touches on the implications of this for the understanding of time dilation and length contraction.
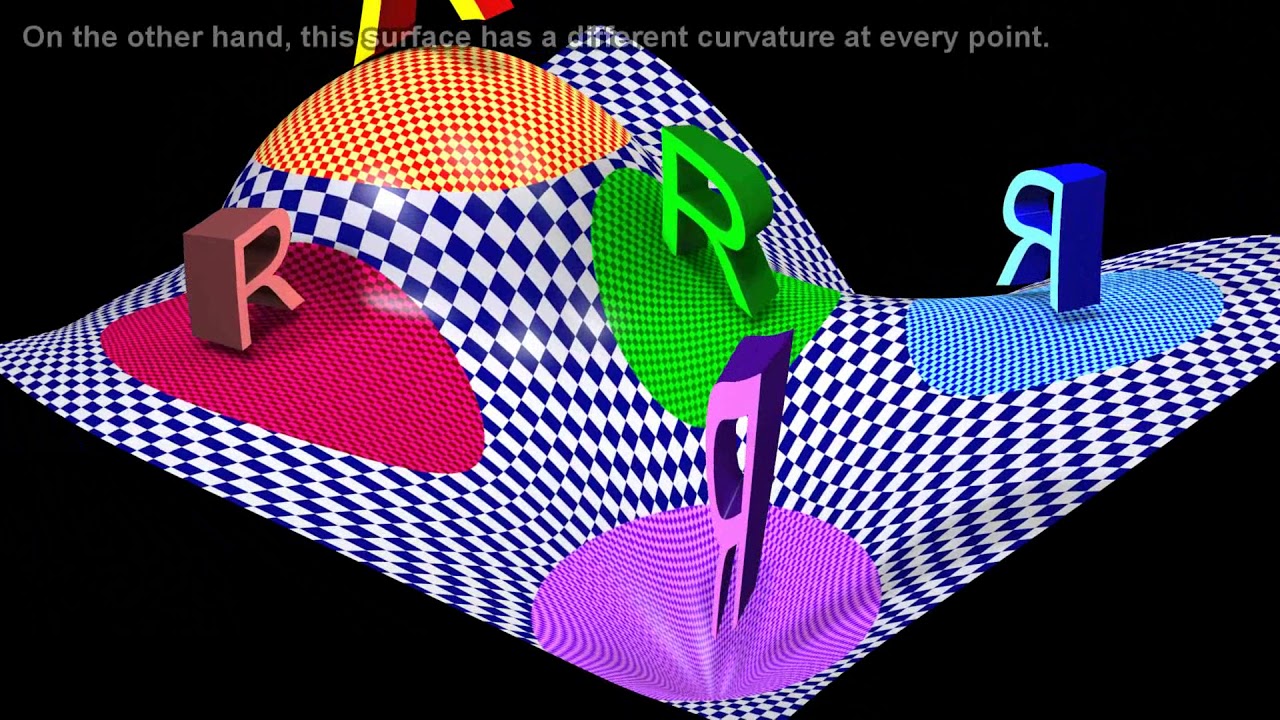
📏 The Geometry of Space-Time and Minkowski Metric
This paragraph discusses the geometric implications of special relativity, introducing the concept of space-time as a physical entity with its own geometry. It explains how the Minkowski metric allows for a new way of measuring distances within the space-time diagram, making the Lorentz transformations analogous to rotations, specifically hyperbolic rotations. The paragraph highlights how this new perspective helps to understand acceleration, the unification of electric and magnetic fields, and the relationship between momentum and energy within the framework of space-time.
🚀 The Implications of Special Relativity and the Future of Space-Time Understanding
The final paragraph of the script reflects on the broader implications of special relativity, including the unification of concepts like momentum and energy, and the understanding of acceleration within space-time. It also touches on the historical development of these ideas and hints at the ongoing quest to understand the fundamental nature of space-time, including theories about its granularity, additional dimensions, and quantum fluctuations. The paragraph concludes with a nod to the ongoing search for a deeper, unified understanding of the universe.
Mindmap
Keywords
💡Frame of Reference
💡Space-Time
💡Galilean Transformation
💡Speed of Light
💡Lorentz Transformation
💡Special Relativity
💡Minkowski Metric
💡Hyperbolic Rotation
💡Rapidity
💡General Relativity
💡Momentum and Energy
Highlights
We live in a geometric space with the ability to move, turn, measure lengths, and angles.
Frames of reference describe the universe from a given point of view, with three spatial axes and the observer at the center.
The 20th-century paradigm shift introduced time as a fourth dimension of the universe.
Space-time diagrams represent the flow of time by stacking images of motion.
Galilean transformation involves shifting space slices to change frames of reference.
The speed of light is constant in all reference frames, challenging the Galilean velocity addition.
Light's constant speed led to the understanding that space and time must be warped to accommodate this phenomenon.
Lorentz transformation allows for a change in reference frame while preserving the speed of light.
Time and space are relative, with durations and lengths changing depending on the observer.
Special relativity introduces the concept that moving clocks tick slower and lengths contract.
Space-time is an abstract representation similar to a map, with Lorentz transformations analogous to map rotations.
Minkowski and Ponkari introduced the Minkowski metric, a new way to measure distances within the space-time diagram.
Hyperbolic rotations, or Lorentz transformations, preserve the nature of time and space intervals.
The speed of light corresponds to an infinite rapidity, the maximum theoretical speed attainable after infinite acceleration.
Space-time is a physical entity with its own geometry, allowing for a better understanding of concepts like acceleration.
Electric and magnetic fields are unified within space-time, with electric fields being the time version of magnetic fields.
Momentum and energy are unified as different measurements of movement through space-time.
General relativity builds upon special relativity, introducing the concept of space-time distortion to explain gravity.
Modern theories explore the fundamental geometry of space-time, including the possibility of additional dimensions or quantum fluctuations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: