Mean Absolute Deviation - Statistics

The Organic Chemistry Tutor
14 Oct 202308:39
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains how to calculate the mean absolute deviation, a measure of the average distance between data points and the mean. It first walks through an example using a table to visualize taking the difference and absolute difference between each data point and the mean. It then shows the calculation using the formula, where you sum the absolute differences divided by n. It provides a second example solving directly with the formula. Finally, it visually depicts the distances on a number line to explain conceptually what mean absolute deviation indicates - the average distance of the data points from the mean.

Takeaways
  • 😀 The mean absolute deviation measures the average distance of all the values from the mean
  • 📊 To calculate it: Take the absolute difference between each data point and the mean, sum them up, and divide by n
  • 📈 First calculate the arithmetic mean by summing all values and dividing by n
  • ☑️ Subtract each data point from the mean and take the absolute values
  • ✅ Sum the absolute differences and divide by n to get the mean absolute deviation
  • 📋 Can use a table with columns for X values, mean, differences, and absolute differences
  • 🖥 Or directly apply the formula without a table
  • 👁‍🗨 Visualizing the deviations on a number line shows how far points are from the mean
  • 🎯 The mean absolute deviation tells us the average distance values are from the mean
  • 🤓 Useful for understanding spread and variation in a data set
Q & A

  • What does the mean absolute deviation measure?

    -The mean absolute deviation measures the average distance of all the values from the mean.

  • What are the steps to calculate mean absolute deviation using a table?

    -The steps are: 1) Calculate the arithmetic mean; 2) Subtract each data point from the mean; 3) Take the absolute value of the differences; 4) Sum the absolute differences; 5) Divide the sum by n.

  • How is the mean calculated?

    -The mean is calculated by taking the sum of all the data points and dividing by the total number of data points (n).

  • Why do we take the absolute value of the differences?

    -We take the absolute value so that the negative differences become positive. This allows us to sum the differences.

  • What is the formula to calculate mean absolute deviation without a table?

    -The formula is: Mean Absolute Deviation = (absolute value of (x1 - mean) + absolute value of (x2 - mean) + ... + absolute value of (xn - mean)) / n

  • What does the mean absolute deviation value signify?

    -The mean absolute deviation value signifies the average distance of the data points from the mean. It tells us how far, on average, the data points are from the mean.

  • Does the order of the data points matter when calculating mean absolute deviation?

    -No, the order of the data points does not matter. The mean absolute deviation depends only on the difference of each data point from the mean, not their order.

  • Can the mean absolute deviation be zero?

    -Yes, if all the data points have the same value, which is also the mean, then the mean absolute deviation will be zero.

  • Is a small or large mean absolute deviation better?

    -A small mean absolute deviation is better because it indicates the data points are clustered closely around the mean.

  • What are some real-world uses of mean absolute deviation?

    -Mean absolute deviation is used in statistics and finance to measure forecast error, assess risk, compare predictors, etc.

Outlines
00:00
📏 Calculating Mean Absolute Deviation

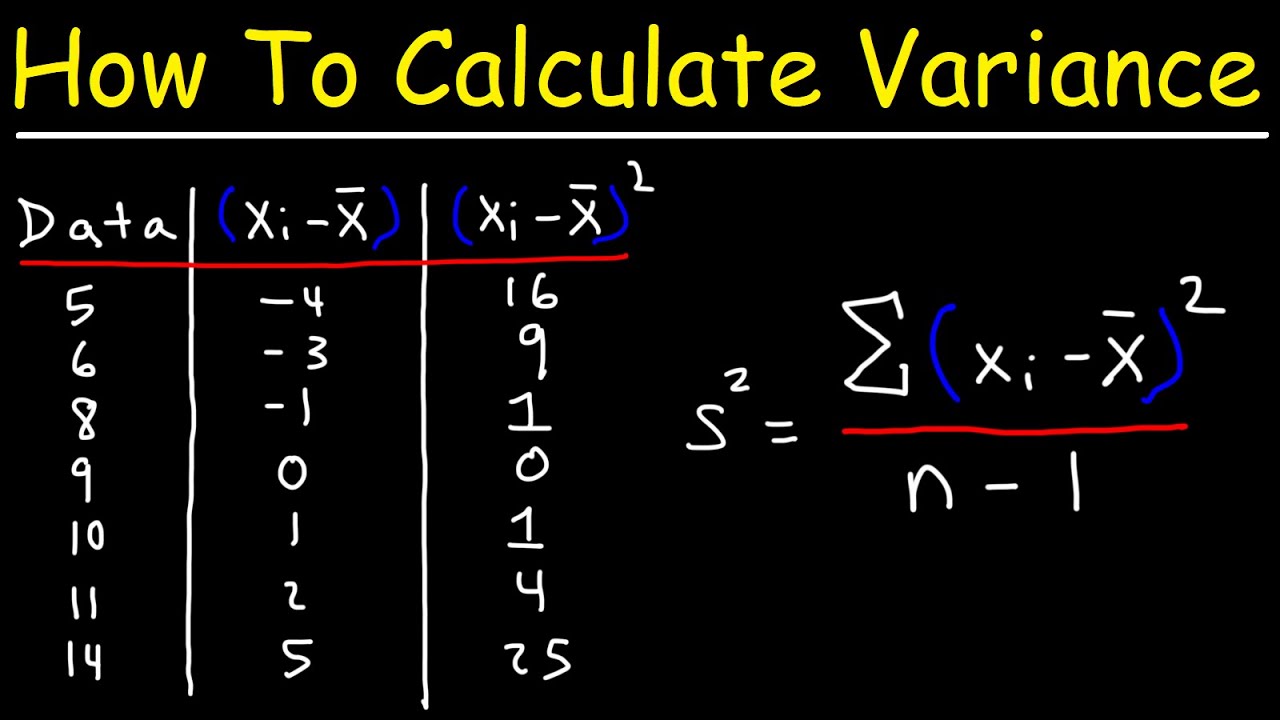
This paragraph explains how to calculate the mean absolute deviation. It provides an example using a table with values of 7, 11, 14, 19, 22, and 29. It steps through the calculation of the mean (17), difference of each data point from the mean, absolute value of the differences, sum of absolute differences (38), and the final mean absolute deviation (38/6 = 6.3 repeating).

05:03
😄 Visual Understanding of Mean Absolute Deviation

This paragraph provides a second example calculating mean absolute deviation for values 5, 9, 12, 16, 18. It finds the mean is 12, calculates the absolute deviations from 12, sums them (20), and divides by 5 data points to get the final mean absolute deviation of 4. It then explains this visually on a number line, showing that on average, the numbers are 4 units away from the mean of 12.

Mindmap
Keywords
💡Mean absolute deviation
The mean absolute deviation measures the average distance between each data point and the mean. It gives an idea of how spread out the data is from the mean value. The script shows the full calculation using a table with the data values, differences from the mean, absolute differences from the mean, summing those absolute differences, and dividing by the number of data points to find the average.
💡Arithmetic mean
Also called the average, the arithmetic mean is calculated by adding all the data values together and dividing by the total number of values. The script shows this calculation being done first before finding the absolute deviations from this mean.
💡Absolute difference
The absolute difference between a data point and the mean is the distance between them without regard to positive or negative. Taking the absolute value removes any negative signs. This transforms the raw differences into absolute differences that can be summed.
💡Number line
A number line graphically represents numerical values and differences between them. The script uses a number line centered on the mean value to visualize and explain the distances from the mean to the outlying data points. This ties back to the concept of absolute deviation.
💡Units
The distances along the number line are measured in units. The mean absolute deviation result of 4 units tells us that on average, the data values tend to be 4 units away from the mean.
💡Summation
Summation means adding together multiple quantities. The formula uses the Greek letter sigma ?? for summation showing that the absolute differences are all added up before dividing.
💡Formula
The script provides the formula for calculating mean absolute deviation: Summation of absolute differences divided by the count (n). The formula sums the distances and normalizes to the average distance.
💡Data points
The individual data values like 7, 11, 14 etc. are called data points. Mean absolute deviation measures distance of points from mean.
💡Dataset
A dataset is the full collection of related data points. Here there are two sample datasets analyzed to demonstrate calculating mean absolute deviation.
💡Spread
How dispersed or gathered the data is. Mean absolute deviation quantifies spread in terms of unit distances from the mean on average.
Highlights

The mean absolute deviation measures the average distance of all the values from the mean

To calculate it: 1) Calculate the arithmetic mean 2) Subtract each data point from the mean 3) Take the absolute value of the differences 4) Sum the absolute differences 5) Divide the sum by the number of data points

Illustrated the calculation using a table with columns for: the data points, the mean, the difference of each point from the mean, and the absolute difference of each point from the mean

The final formula: Mean absolute deviation = Sum of absolute differences / Number of data points

Worked through another example by directly applying the formula without using a table

Visualized the meaning of mean absolute deviation on a number line by showing the distance of each data point from the mean

On average each number is about four units away from the mean on average

The mean absolute deviation provides a measure of variability and how dispersed the data is from the mean value

Using a table breakdown makes the calculation steps clear but the formula can calculate it directly

Taking the absolute value before summing eliminates the cancellation of positive and negative differences

Dividing the sum of absolute differences by the number of data points gives an average distance from the mean

The number line visualization shows how each data point differs from the calculated mean value

Smaller mean absolute deviation indicates the data points are clustered closer to the mean

Larger mean absolute deviation indicates the data points are more spread out from the mean

Mean absolute deviation complements standard deviation as a statistic for variability

Transcripts

Browse More Related Video

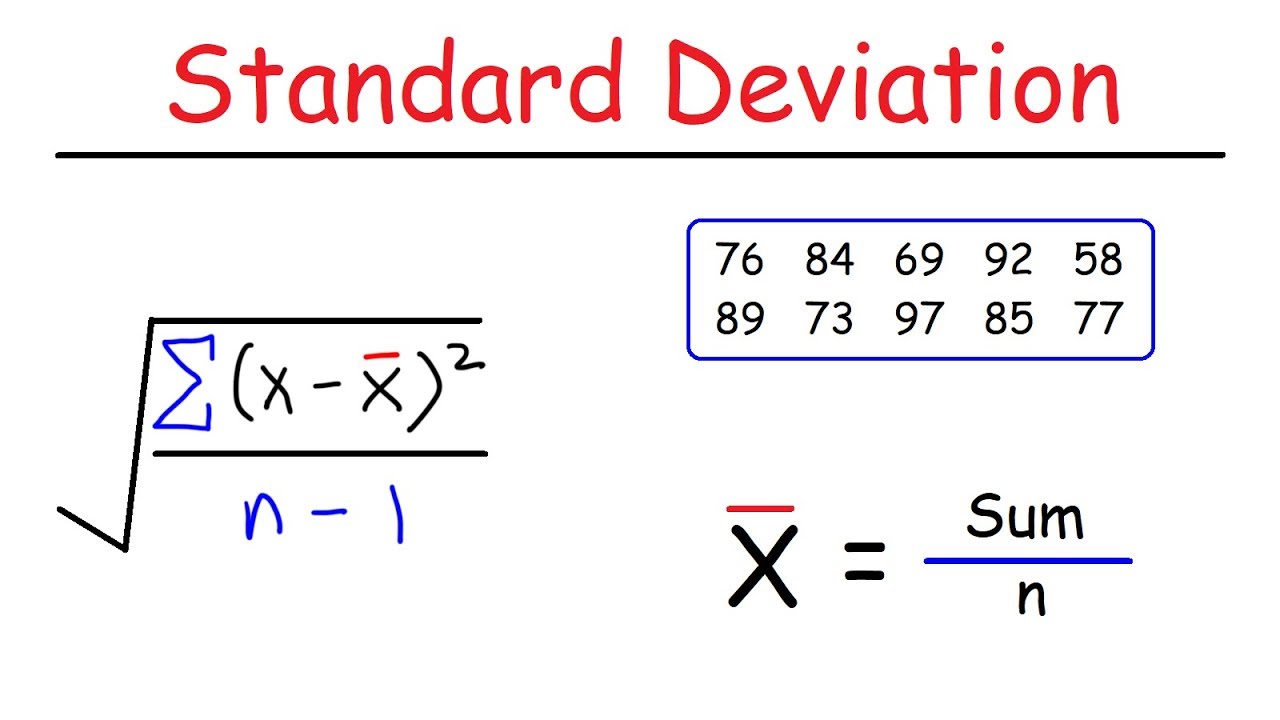
What is Standard Deviation and Mean Absolute Deviation | Math, Statistics for data science, ML

How To Calculate Variance

Standard Deviation & Mean Absolute Deviation Explained - 6-8-19]

How To Calculate The Standard Deviation

What is a "Standard Deviation?" and where does that formula come from

Mean Absolute Deviation

Rate This

5.0 / 5 (0 votes)

Thanks for rating: