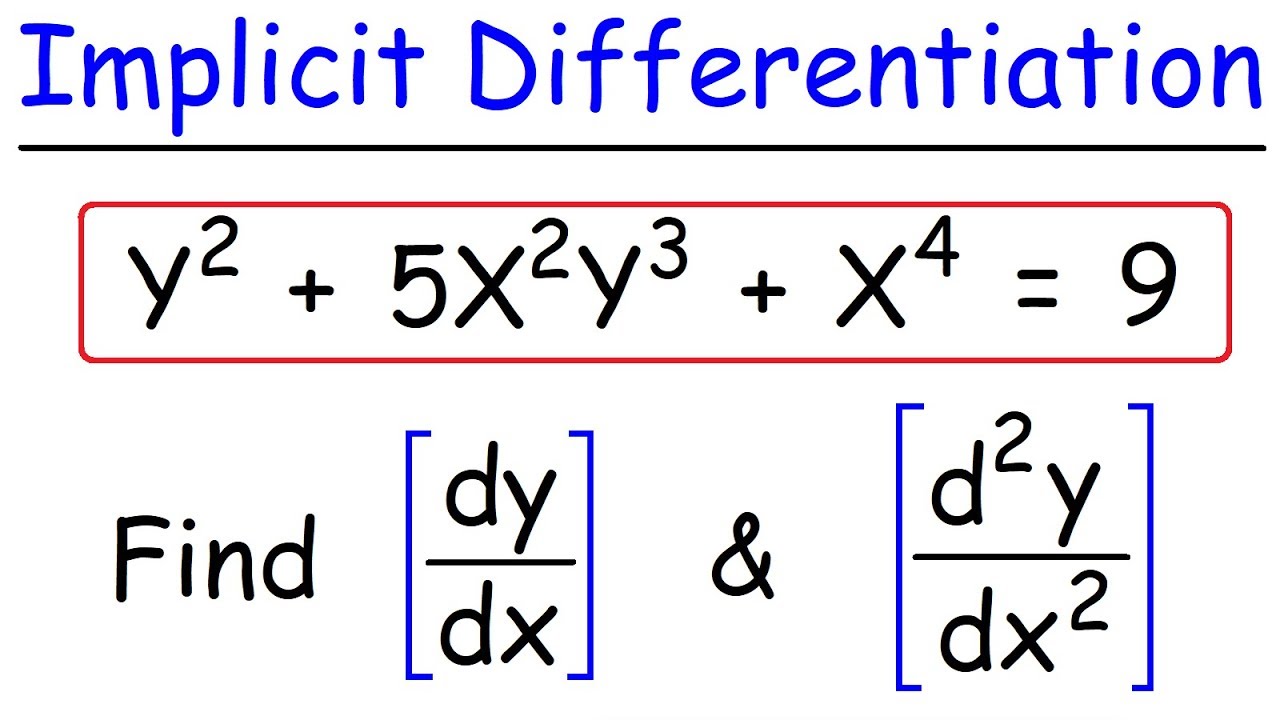
Implicit Differentiation Second Derivative Trig Functions & Examples- Calculus
TLDRThis video script provides a comprehensive guide to implicit differentiation, a mathematical technique used to find derivatives of equations where variables are not explicitly isolated. The script explains the process step by step, starting with basic differentiation rules for functions of 'y' with respect to 'x', emphasizing the inclusion of 'dy/dx' when differentiating 'y' terms. It then demonstrates how to handle constants and the cancellation of 'dx' terms. The video also covers finding the second derivative using the quotient rule, with a clear example provided. Further examples include differentiation involving trigonometric functions and the product rule, illustrating how to apply these concepts in various contexts. The script concludes with a problem involving square roots and trigonometric functions, showing how to simplify and solve for the first derivative. Throughout, the emphasis is on understanding the underlying principles of differentiation and applying them effectively to solve complex problems.
Takeaways
- 📚 When performing implicit differentiation, always include the term dy/dx when differentiating a y variable.
- 🔑 For differentiation with respect to x, dx/dx cancels out, so it's not necessary to write it explicitly next to terms involving x.
- 🔍 To isolate dy/dx, you may need to rearrange terms and factor out the greatest common factor (GCF) involving dy/dx.
- 📐 Use the quotient rule for finding second derivatives when dealing with fractions, which involves manipulating the expression f(x)g'(x) - g(x)f'(x) / [g(x)]^2.
- 🧮 Replace dy/dx with its expression from the first derivative when finding higher-order derivatives.
- 🔧 The product rule is essential when differentiating expressions involving a product of x and y, such as XY.
- 📐 When differentiating trigonometric functions, use the respective derivative formulas, such as sin(U) * U' for sin(U) and cos(U) * U' for cos(U).
- 🧹 Simplify expressions by canceling out common terms and reducing negative exponents to positive where possible.
- 🔗 In problems involving square roots, it may be helpful to rewrite the equation to avoid fractions and simplify differentiation.
- 📉 When isolating dy/dx, move all terms without dy/dx to one side of the equation and factor out dy/dx from the other side.
- 📈 For problems with trigonometric functions, apply the chain rule and product rule as necessary to find the derivatives.
Q & A
What is the process of implicit differentiation?
-Implicit differentiation is a method used to find the derivatives of equations where variables are not explicitly separated. It involves differentiating both sides of an equation with respect to 'x' and then isolating the derivative of 'y' with respect to 'x' (dy/dx).
How do you differentiate y^2 with respect to X using implicit differentiation?
-To differentiate y^2 with respect to X, you treat y as a function of X and apply the power rule, which results in 2y * (dy/dx).
What happens when you differentiate an equation with a constant term during implicit differentiation?
-When differentiating a constant term with respect to X, the derivative is zero because the constant does not change with respect to X.
How do you isolate dy/dx in an equation after applying implicit differentiation?
-To isolate dy/dx, you typically need to rearrange the terms so that all terms containing dy/dx are on one side of the equation and terms without dy/dx are on the other side. Then, you divide both sides by the coefficient of dy/dx to solve for dy/dx.
What is the quotient rule used for finding the second derivative in the context of implicit differentiation?
-The quotient rule is used when you need to differentiate a fraction. It states that the derivative of a quotient of two functions is the denominator function times the derivative of the numerator function minus the numerator function times the derivative of the denominator function, all divided by the square of the denominator function.
How do you find the second derivative of y with respect to x in an implicit differentiation problem?
-To find the second derivative, you first find the first derivative (dy/dx), then differentiate it again with respect to x. You may need to use the quotient rule if the second derivative involves a fraction. After finding the expression, you typically replace dy/dx with its expression from the first derivative to simplify the result.
What is the product rule used for in differentiation?
-The product rule is used to differentiate the product of two functions. It states that the derivative of the product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.
How do you handle the differentiation of trigonometric functions like cosine or sine in implicit differentiation?
-For trigonometric functions, you use the specific derivative rules for those functions. For example, the derivative of cosine of a function U (where U is some expression involving x and y) is -sine of U times the derivative of U. You then apply the product rule if U involves more than one variable.
What is the derivative of the square root of x with respect to x?
-The derivative of the square root of x (x^(1/2)) with respect to x is (1/2)x^(-1/2), which simplifies to 1/(2*sqrt(x)).
How do you simplify the expression for the derivative of y with respect to x in an equation like x^3 - xy + y^2 = 4?
-After differentiating both sides with respect to x and rearranging terms, you isolate dy/dx on one side of the equation. You then simplify the expression by factoring out dy/dx and dividing both sides by the resulting expression to solve for dy/dx.
What is the general approach to solving for the first derivative in an implicit differentiation problem?
-The general approach involves differentiating both sides of the given equation with respect to x, treating y as a function of x, and then isolating the term containing dy/dx. This often requires applying algebraic manipulation and possibly the product or quotient rule for differentiation.
Outlines
📚 Introduction to Implicit Differentiation
This paragraph introduces the concept of implicit differentiation, a method used to find derivatives of equations that are not explicitly expressed as functions of y = f(x). It explains the process of differentiating an equation with respect to x, emphasizing the need to include dy/dx when differentiating y variables. The paragraph provides examples of differentiating various expressions involving y and x, and demonstrates how to isolate dy/dx to find the first derivative. It also touches on the use of the quotient rule for finding the second derivative and how to simplify the expression by canceling out terms.
🔍 Isolating and Simplifying Derivatives
The second paragraph delves into the process of isolating dy/dx in an equation and simplifying the expression. It covers the steps to find the first derivative of a given equation, including moving terms without dy/dx to one side and factoring out the greatest common factor (GCF). The paragraph also explains how to find the second derivative using the quotient rule, substituting dy/dx with the expression obtained from the first derivative, and simplifying the final expression.
🧮 Applying Product and Quotient Rules
This paragraph focuses on the application of the product rule and quotient rule in implicit differentiation. It provides an example where the product rule is used to differentiate XY, followed by the quotient rule to find the second derivative. The paragraph emphasizes the importance of distributing terms, factoring out dy/dx, and simplifying the expression to find the final form of the derivatives.
🔢 Dealing with Square Roots and Fractions
The fourth paragraph discusses the differentiation of equations involving square roots and fractions. It demonstrates how to rewrite equations to simplify the differentiation process and how to handle terms with square roots by moving them from the numerator to the denominator to make negative exponents positive. The paragraph also shows how to simplify expressions by canceling out terms and combining like terms.
📐 Trigonometric Functions in Implicit Differentiation
This paragraph explores the differentiation of equations involving trigonometric functions. It explains the process of differentiating the product of x and y within a cosine function using the product rule. The paragraph also covers how to handle the derivative of sine and cosine functions and how to isolate dy/dx by factoring and simplifying the equation.
🤔 Advanced Implicit Differentiation Techniques
The sixth paragraph presents more complex examples of implicit differentiation, including equations with cosine functions and sums of variables. It illustrates the process of differentiating the angle of a cosine function and isolating dy/dx by moving terms and factoring out common factors. The paragraph concludes with a final expression for dy/dx, demonstrating the application of implicit differentiation in various scenarios.
Mindmap
Keywords
💡Implicit Differentiation
💡Derivative
💡Product Rule
💡Quotient Rule
💡Chain Rule
💡Trigonometric Functions
💡
💡Slope
💡First Derivative
💡Second Derivative
💡Greatest Common Factor (GCF)
💡Constant
Highlights
Implicit differentiation involves differentiating an equation with respect to x while treating y as a function of x.
When differentiating y^2 with respect to x, the result is 2y * dy/dx.
For the derivative of r^3 with respect to x, it is 3r^2 * dr/dx.
Differentiating x to the power of 4 with respect to x yields 4x^3 * dx/dx, which simplifies to 4x^3.
In implicit differentiation, dy/dx is added whenever differentiating a y variable.
The derivative of a constant with respect to x is zero.
To find the second derivative, the quotient rule is applied, treating the numerator and denominator as separate functions.
The formula for the quotient rule is (G * F' - F * G') / G^2, where F and G are functions of x and y.
When finding the second derivative, it's important to replace dy/dx with its expression from the first derivative.
The second derivative of x^3 - xy + y^2 = 4 is found by applying the quotient rule to the first derivative expression.
For the equation XY = 4, the first derivative dy/dx is found using the product rule, resulting in -y/x.
The second derivative of the equation XY = 4 is simplified by substituting dy/dx with its expression from the first derivative.
When dealing with square roots in the equation, the power rule is applied, and fractions are simplified by multiplying by a common factor.
Trigonometric functions within an equation require the use of product rule for differentiation, treating the trigonometric function as a composition of functions.
The derivative of cosine(XY) is found by applying the chain rule, resulting in -sin(XY) * (y + x * dy/dx).
Isolating dy/dx in trigonometric equations involves factoring out the common factor and dividing through by it.
The first derivative of the equation sin(x + y) = 2x - 2y is found by differentiating both sides and isolating dy/dx.
The final answer for dy/dx in various problems is simplified by canceling out common terms and expressing the result in its simplest form.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: