Math 11 - Section 3.8
TLDRThe video script offers an in-depth exploration of implicit differentiation, a mathematical technique used to find the derivative of a function when it's not expressed solely in terms of y. The presenter begins by contrasting implicit differentiation with explicit differentiation, demonstrating how the latter is straightforward when y can be isolated. However, the script's focus is on the more complex scenarios where y cannot be explicitly solved, necessitating implicit differentiation. The process involves differentiating both sides of an equation with respect to x, applying the product and chain rules where necessary, and then solving for dy/dx. The presenter illustrates the method with several examples, including those with y terms in multiple places within the equation, emphasizing the importance of moving all dy/dx terms to one side, factoring them out, and then isolating dy/dx. The script concludes with an encouragement to practice the technique and seek help if needed, highlighting the utility of implicit differentiation in finding the slope of tangent lines and curves without explicitly solving for y.
Takeaways
- 📚 Implicit differentiation is a technique used to find the derivative of y with respect to x when we cannot explicitly solve for y in terms of x.
- 🔑 When differentiating y implicitly, treat y as a function of x, and use the chain rule to account for dy/dx when differentiating terms involving y.
- 🔍 To differentiate an equation implicitly, differentiate every term with respect to x, applying the sum and product rules as necessary.
- 🧮 If an equation contains y terms raised to a power, use the power rule and multiply by dy/dx for each instance of y.
- 📉 When you have dy/dx on both sides of the equation, isolate it on one side and factor it out to solve for dy/dx.
- 🔗 The derivative dy/dx represents the slope of the tangent line to the curve at any given point (x, y).
- ⚖️ To find the slope of the tangent line at a specific point, substitute the x and y values of the point into the derived dy/dx expression.
- 🤝 The product rule is essential when differentiating terms that are the product of x and y, such as 2xy, where both the derivative of x times y and y times the derivative of x are needed.
- 🔢 When presented with an equation that includes x and y in the same term, like x^2 + 2xy = 3y^2, use implicit differentiation and carefully apply the product rule and chain rule.
- 📌 If the equation involves more than one instance of dy/dx, move all terms without dy/dx to one side and factor out dy/dx to solve for it.
- 📐 For problems that include x and y in the same term, such as x^4 - x^2y^3 = 12, use implicit differentiation and the product rule to find the derivative dy/dx, then simplify and factor as needed.
Q & A
What is implicit differentiation?
-Implicit differentiation is a method used to find the derivative of y with respect to x when it is not possible to explicitly solve for y in terms of x.
Why is implicit differentiation necessary?
-Implicit differentiation is necessary when the equation relating y and x cannot be easily solved for y alone, allowing us to find the derivative dy/dx without isolating y.
How do you differentiate the sum or difference of terms with respect to x?
-When differentiating the sum or difference of terms with respect to x, you can differentiate each term individually and then add or subtract the results as appropriate.
What is the product rule used for?
-The product rule is used when differentiating a product of two functions, such as 2xy, where you multiply the first function by the derivative of the second and add it to the second function times the derivative of the first.
How do you handle the derivative of a constant?
-The derivative of a constant with respect to any variable is zero, as constants do not change with respect to the variable.
What is the chain rule?
-The chain rule is a fundamental rule in calculus used to compute the derivative of a composite function. It states that the derivative of a composition of functions is the product of the derivative of the outer function and the derivative of the inner function.
What is the process to find the slope of the tangent line at a given point using implicit differentiation?
-To find the slope of the tangent line at a given point, you first differentiate the equation implicitly with respect to x, then solve for dy/dx, and finally plug in the given x and y values to find the slope at that point.
How do you solve for dy/dx when there are multiple instances of dy/dx in the equation?
-When there are multiple instances of dy/dx, you first move all terms without dy/dx to one side of the equation and factor out dy/dx on the other side. Then, you can solve for dy/dx by dividing by the remaining factor.
What is the role of dy/dx in implicit differentiation?
-In implicit differentiation, dy/dx represents the derivative of y with respect to x. It is used to account for the changes in y as x changes, especially when y cannot be expressed explicitly in terms of x.
Why is it sometimes preferable to factor out a negative sign when expressing the final answer?
-Factoring out a negative sign can simplify the expression and make it more readable. It is often preferred to avoid leading with a negative sign and to have a positive factor where possible.
How do you apply implicit differentiation to an equation with y terms raised to powers?
-When applying implicit differentiation to an equation with y terms raised to powers, you use the power rule for the derivative of y terms, and then multiply by dy/dx for each y term to account for the chain rule.
What is the final step after finding dy/dx in implicit differentiation?
-The final step after finding dy/dx is to simplify the expression, if possible, and then you can either leave the result in terms of dy/dx or evaluate it further if specific values for x and y are provided.
Outlines
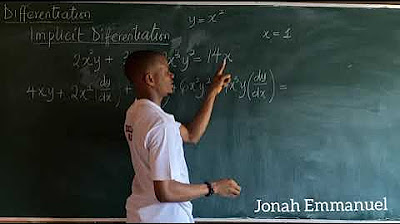
📚 Introduction to Implicit Differentiation
This paragraph introduces the concept of implicit differentiation, a method used to find the derivative of y with respect to x when y cannot be isolated. The speaker illustrates the process by first solving a simple equation for y explicitly and then re-approaching the problem using implicit differentiation. The key takeaway is that when differentiating with respect to x and y is involved, the chain rule applies, leading to dy/dx being a part of the derivative expression.
🔍 Implicit Differentiation: Application and Necessity
The speaker delves into the application of implicit differentiation with examples, emphasizing its necessity when explicit solutions for y are not feasible. The process involves differentiating both sides of an equation with respect to x, handling the differentiation of y as a function, and isolating dy/dx to find the derivative. The examples given include a circle equation and a problem where the derivative is evaluated at a specific point, demonstrating the concept's utility.
🧮 Chain Rule and Product Rule in Implicit Differentiation
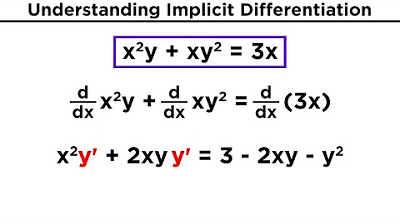
This section focuses on the use of the chain rule and product rule within the context of implicit differentiation. The speaker clarifies that when differentiating a product of x and y, the product rule is essential. The paragraph walks through a step-by-step process of differentiating an equation involving the product of x and y, highlighting the need to distribute and simplify the resulting expression to solve for dy/dx.
🔢 Solving for dy/dx with Complex Equations
The speaker tackles more complex equations where x and y are intertwined, necessitating the use of implicit differentiation. The process involves taking derivatives with respect to x, applying the product rule when necessary, and strategically moving terms to isolate dy/dx. The speaker also demonstrates how to factor and simplify the equation to make it easier to solve for dy/dx, showcasing the method's adaptability in various scenarios.
📉 Evaluating dy/dx at Specific Points
This paragraph discusses the evaluation of the derivative dy/dx at specific points provided by the problem. The speaker shows how to plug in given x and y values into the derived expression for dy/dx to find the slope of the tangent line or the curve at that point. The process is demonstrated with a detailed example, emphasizing the practical application of implicit differentiation in finding slopes at particular points on a curve.
🔁 Handling Multiple dy/dx Terms
The speaker addresses the challenge of having more than one term with dy/dx in an equation. The strategy involves moving all terms without dy/dx to one side of the equation and factoring out dy/dx to solve for it explicitly. The paragraph provides a detailed example of this process, emphasizing the importance of combining like terms and factoring to simplify the equation before dividing by the common factor to isolate dy/dx.
📝 Final Thoughts and Practice Advice
In the concluding paragraph, the speaker summarizes the key points of implicit differentiation, emphasizing the importance of differentiating with respect to x normally but applying the chain rule when differentiating with respect to y. The speaker advises practicing the method, especially when dealing with multiple dy/dx terms, and encourages asking questions for further clarification. The goal is to master the technique for solving derivatives in complex equations where y cannot be explicitly isolated.
Mindmap
Keywords
💡Implicit Differentiation
💡Derivative
💡Chain Rule
💡Product Rule
💡Slope of the Tangent Line
💡Function
💡Explicit Differentiation
💡Differentiation with Respect to X
💡Equation
💡dy/dx
💡Plug-in Values
Highlights
Introduction to implicit differentiation, a method for finding the derivative of y when it cannot be explicitly solved for.
Demonstration of explicit differentiation by solving for y and then differentiating.
Illustration of implicit differentiation by differentiating an equation involving y without solving for y explicitly.
Derivation of dy/dx for the equation y + 3x^2 - 5 = 0 using implicit differentiation.
Explanation of the necessity of implicit differentiation when y is a function and not a variable.
Use of the chain rule when differentiating a function of y with respect to x.
Differentiation of x^2 + y^2 = 25 using implicit differentiation, resulting in dy/dx = -2x/y.
Process of solving for dy/dx by isolating it on one side of the equation.
Differentiation of x^3 + 2y^3 = 6 and finding the slope of the tangent line at a given point (2, -1).
Application of the product rule in implicit differentiation when x and y are multiplied together.
Differentiation of 2xy + 3 = 0 using the product rule and solving for dy/dx.
Handling of equations with multiple occurrences of y by combining dy/dx terms and solving for dy/dx.
Differentiation of x^4 - x^2y^3 = 12, applying the product rule, and evaluating dy/dx at a specific point.
Strategy for dealing with negative signs by factoring out common terms to simplify the derivative.
Differentiation of x^2 + 2xy = 3y^2 and solving for dy/dx by combining and factoring dy/dx terms.
Differentiation of xy + y^2 - 2x = 0, applying the product and chain rules, and evaluating dy/dx at the point (1, -2).
Emphasis on the importance of recognizing when to apply the chain rule and how to manage multiple dy/dx terms.
Encouragement to practice implicit differentiation and to ask questions for further understanding.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: