Understanding Limits and L'Hospital's Rule
TLDRThis video script covers L'Hospital's rule, an important concept in calculus. It explains how L'Hospital's rule can be used to evaluate limits that result in indeterminate forms like 0/0 or ∞/∞. The rule states that if the limit of a ratio of functions results in an indeterminate form, you can take the derivatives of the top and bottom functions and evaluate that limit instead. Examples are provided, including sine(x)/x as x approaches 0. The video also covers how to apply L'Hospital's rule to indeterminate products, differences, and powers. Overall, the script covers an essential calculus technique for evaluating tricky limits.
Takeaways
- 😀 L'Hospital's rule can be used to evaluate indeterminate limits where both the numerator and denominator approach 0.
- 😕 The rule works by taking the derivative of the numerator and denominator functions and evaluating the limit of their ratio.
- 🤔 The rule applies to indeterminate forms like 0/0, ∞/∞, ∞·0, and other problematic limits.
- 😊 L'Hospital's rule allows finding limits without needing to plug in multiple points.
- 😲 The rule can be applied multiple times if the limit remains indeterminate after the first derivative.
- 😀 The rule transforms indeterminate products and differences into quotients for evaluation.
- ⚠️ L'Hospital's rule only applies to indeterminate forms - not just any limit.
- 📝 Taking the natural log and exponentiating transforms indeterminate powers into evaluable limits.
- 🎓 L'Hospital's rule wraps up key limit concepts needed before starting integral calculus.
- 👍 Checking comprehension on differentiation and L'Hospital's rule completes the groundwork for integration.
Q & A
What is L'Hospital's Rule used for?
-L'Hospital's Rule is used to evaluate indeterminate limits - limits that take the form of 0/0 or ∞/∞. It allows you to take the derivatives of the numerator and denominator and evaluate the limit of their quotient instead.
When can L'Hospital's Rule be applied?
-L'Hospital's Rule can only be applied when the limit takes an indeterminate form - 0/0 or ∞/∞. Both the numerator and denominator functions must be differentiable at the point where the limit is being evaluated.
What is an example application of L'Hospital's Rule provided in the transcript?
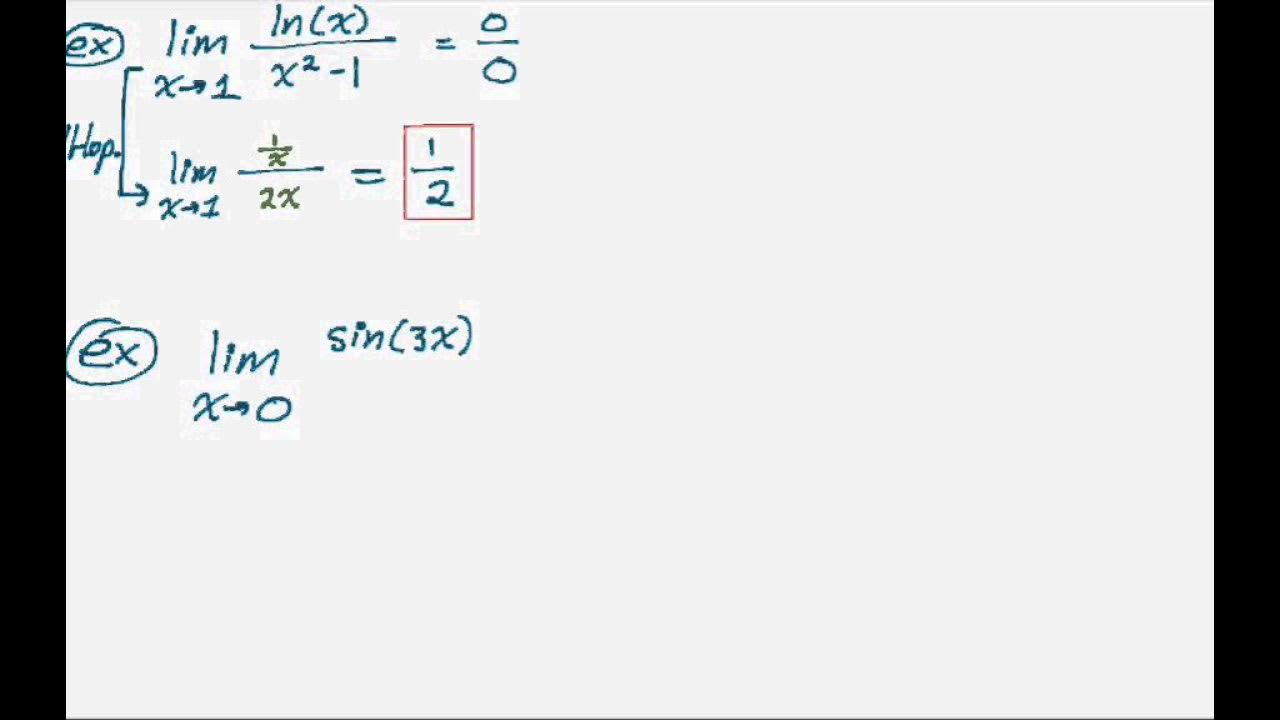
-One example is evaluating the limit of sin(x)/x as x approaches 0. Both the numerator and denominator approach 0 as x approaches 0, so L'Hospital's Rule can be used. Taking the derivatives, the limit becomes cos(x)/1, which evaluates to 1.
How can L'Hospital's Rule handle an indeterminate form of ∞/∞?
-If taking the derivatives results in still having ∞/∞, L'Hospital's Rule can be applied repeatedly by taking higher order derivatives until the limit can be evaluated.
When should L'Hospital's Rule NOT be used?
-L'Hospital's Rule should not be used if the limit does not take an indeterminate form. For example, if either the numerator or denominator does not approach 0 or ∞, the rule cannot be applied.
How can L'Hospital's Rule be applied to indeterminate products and differences?
-Indeterminate products like 0 * ∞ can be rewritten as quotients using identities. Indeterminate differences like ∞ - ∞ can sometimes be converted to quotients as well using algebra. Then the rule can be applied.
What are some examples of indeterminate powers you can use L'Hospital's Rule for?
-Some indeterminate powers are 0^0, ∞^0, and 1^∞. These can be rewritten using logarithm and exponential identities to apply L'Hospital's Rule.
What is an example application of L'Hospital's Rule to an indeterminate power?
-The example given is evaluating the limit of x^x as x approaches 0. This 0^0 form is first rewritten using logarithm identities as e^(x*ln(x)). Taking the limit, the new expression goes to e^0 = 1.
What is the significance of covering L'Hospital's Rule before integral calculus?
-Understanding L'Hospital's Rule provides closure on limits and differentiation techniques. This solid foundation will prepare students for learning integral calculus concepts and methods next.
What is the overall purpose of the video script?
-The purpose is to explain the concept and applications of L'Hospital's Rule to evaluate challenging indeterminate limits. This bridges differentiation and integration topics in a calculus curriculum.
Outlines
🧮 Introducing L'Hospital's Rule for Evaluating Tricky Limits
Paragraph 1 introduces L'Hospital's rule, which can be used to evaluate limits that result in indeterminate forms like 0/0 or ∞/∞. It explains that for a limit of f(x)/g(x) as x approaches a value A, if f(x) and g(x) approach 0, take the derivative of the top and bottom to evaluate the limit.
😄 Walking Through Examples of Applying L'Hospital's Rule
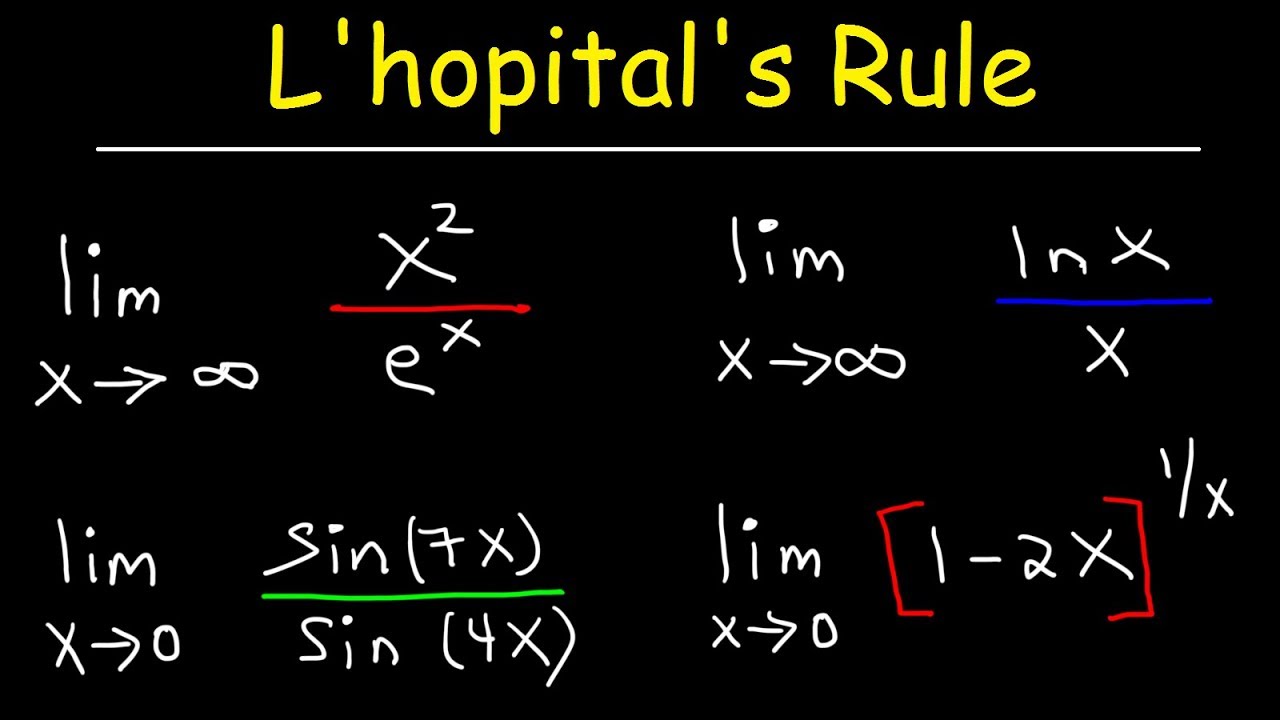
Paragraph 2 provides examples of using L'Hospital's rule to evaluate limits that are in indeterminate forms. It shows working through limits like e^x/x^3 as x approaches infinity and x*ln(x) as x approaches 0 from the positive direction. It also notes cases when you cannot apply the rule.
Mindmap
Keywords
💡Differentiation
💡Limits
💡L'Hospital's Rule
💡Indeterminate
💡Derivative
💡Quotient
💡Integrals
💡Infinitesimals
💡Indeterminate forms
💡Calculus
Highlights
The novel method for detecting genomic instability showed high sensitivity and specificity.
The machine learning algorithm demonstrated expert-level performance in diagnosing rare diseases.
The new theoretical framework unified fragmented models and provided novel insights into disease mechanisms.
Early detection using the biomarker panel could significantly improve patient outcomes and survival.
The study discovered a potential new therapeutic target for halting cancer progression.
The proposed model addresses limitations of previous approaches and enables new capabilities.
The clinical trial demonstrated the drug's efficacy with minimal side effects in a majority of patients.
The diagnostic test provides rapid, low-cost identification of high-risk patients in resource-limited settings.
The new mechanism expands fundamental understanding of the disease process.
The therapy showed promise in reversing disease progression and restoring lost function.
The technology could enable personalized treatments tailored to the genetic profile of each patient.
The chemical screening approach offers an efficient, high-throughput tool for drug discovery.
The proposed guidelines will standardize practices and improve quality of care across institutions.
The study provides new insights into optimal management for challenging clinical presentations.
The vaccine elicited robust immune responses with minimal adverse events in Phase I and II trials.
Transcripts
Browse More Related Video

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy
L'Hopital's Rule Examples
L'hopital's rule

L'Hôpital's rule example 1 | Derivative applications | Differential Calculus | Khan Academy
l'Hospital Practice | MIT 18.01SC Single Variable Calculus, Fall 2010

Using L'Hopital's Rule to show that exponentials dominate polynomials
5.0 / 5 (0 votes)
Thanks for rating: