Basic Trigonometric Identities: Pythagorean Identities and Cofunction Identities

Professor Dave Explains
8 Jan 201805:25
EducationalLearning
32 Likes 10 Comments

TLDRThis script outlines trigonometric identities and functions. It begins by listing the six trig functions and notes sine, cosine and tangent are reciprocals. An identity mentioned is tangent equals sine over cosine. The Pythagorean identity is derived from the Pythagorean theorem. By dividing this identity by cosine or sine squared, additional identities are formed. Next, cofunction identities are explained using the unit circle, realizing sine of an angle equals cosine of its complement. Basic identities are listed at the end for memorization before a comprehension check.

Takeaways
  • 😀 The six trigonometric functions are sine, cosine, tangent, cotangent, secant, and cosecant.
  • 😃 Tangent equals sine over cosine, and cotangent equals cosine over sine.
  • 🤓 The Pythagorean identity relates sine, cosine, and 1 through an equation derived from the Pythagorean theorem.
  • 🧐 The cofunction identities relate sine and cosine of complementary angles, as well as tangent and cotangent.
  • 👀 Secant squared equals 1 over cosine squared, and cosecant squared equals 1 over sine squared.
  • 🤔 Sine of an angle equals cosine of 90 minus that angle, and vice versa.
  • 🧠 Memorize the Pythagorean identity and cofunction identities for ease of use.
  • 📝 Use identities to derive other useful trigonometric relationships.
  • 🔎 Understand how exponents distribute over products and quotients when deriving identities.
  • 🎓 Apply identities appropriately when working with trigonometric functions.
Q & A

  • What are the six trigonometric functions?

    -The six trigonometric functions are sine, cosine, tangent, cosecant, secant, and cotangent.

  • How is tangent defined in terms of sine and cosine?

    -Tangent is defined as sine over cosine. So tangent = sin/cos.

  • What is the Pythagorean identity and how is it derived?

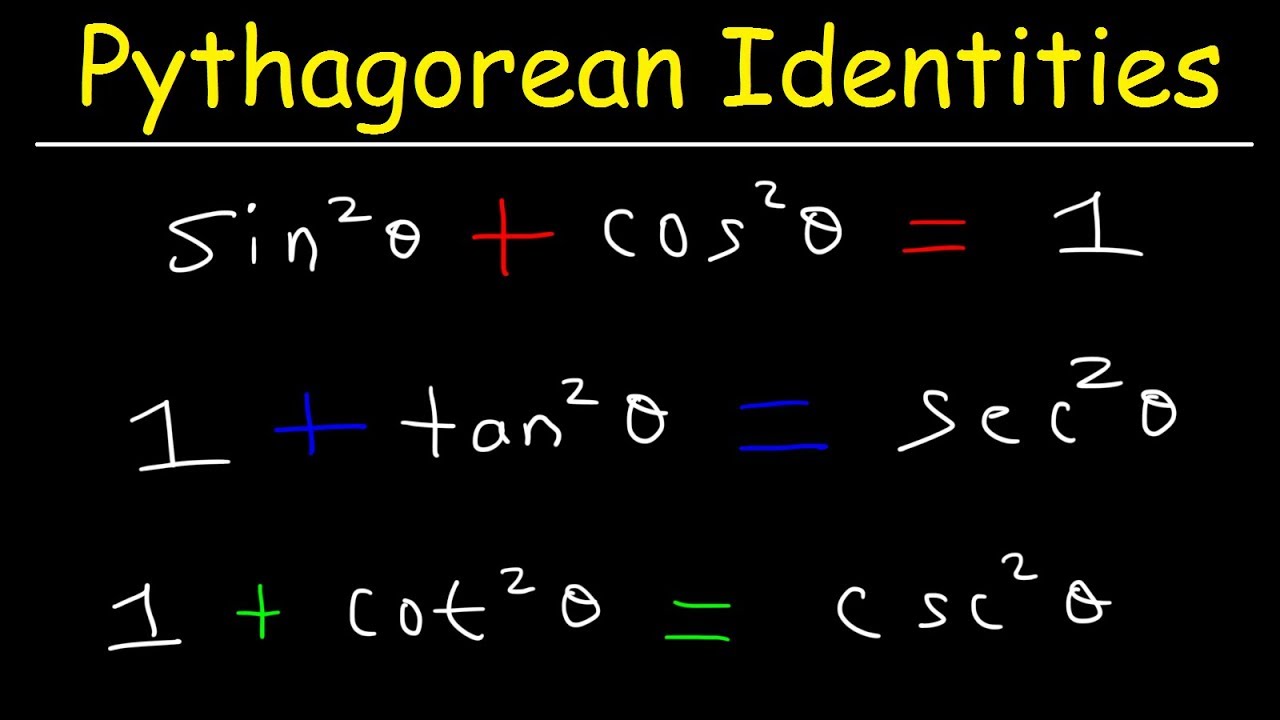
    -The Pythagorean identity states that sin2θ + cos2θ = 1. It is derived from the Pythagorean theorem by dividing both sides by c2 and substituting sinθ = a/c and cosθ = b/c.

  • What are the other Pythagorean identities that can be derived?

    -The other Pythagorean identities are: tan2θ + 1 = sec2θ and 1 + cot2θ = csc2θ.

  • What is the relationship between sine and cosine for complementary angles?

    -For complementary angles, sine of θ = cosine of (90° - θ). This relationship occurs because of the values on the unit circle.

  • What are cofunction identities?

    -Cofunction identities relate the trigonometric functions of complementary angles. For example, sine of θ = cosine of (90° - θ).

  • How can you write tangent and cotangent identities similar to the sine and cosine cofunction identities?

    -Since tangent = sin/cos and cotangent = cos/sin, the identities are: tanθ = cot(90° - θ) and cotθ = tan(90° - θ).

  • What identities should you memorize from this video?

    -The key identities to memorize are: the definitions of tangent, cotangent, etc. in terms of sine and cosine; the Pythagorean identities; and the cofunction identities relating sine, cosine, tangent and cotangent.

  • Why are identities important in trigonometry?

    -Identities allow you to make substitutions and relate different trig functions in calculations and proofs. They are essential tools in trigonometry.

  • How can you check your comprehension of trig identities?

    -You can check comprehension by: deriving identities yourself, using identities to evaluate expressions, proving trigonometric equations, and recognizing which identities can be used in various situations.

Outlines
00:00
😃 Introducing Trigonometric Identities

Professor Dave introduces the topic of trigonometric identities. He lists the six trigonometric functions - sine, cosine, tangent, cosecant, secant, and cotangent. He notes that sine, cosine and tangent are reciprocals of cosecant, secant and cotangent respectively. He also states the identity that tangent equals sine over cosine.

😃 Deriving the Pythagorean Identity

Professor Dave derives the Pythagorean identity starting from the Pythagorean theorem. By dividing both sides by cosine squared theta, the identity sin^2(theta) + cos^2(theta) = 1 is obtained. This can further be manipulated to derive tangent squared + 1 = secant squared and 1 + cotangent squared = cosecant squared.

😃 Understanding Cofunction Identities

Professor Dave explains cofunction identities using the unit circle. He notes that sine of an angle equals cosine of its complementary angle, and vice versa. Similarly, tangent of an angle equals cotangent of its complementary angle. He provides examples like sin(30) = cos(60) to illustrate.

😃 Listing Basic Trigonometric Identities

Professor Dave concludes by listing the basic trigonometric identities covered in the video: sine, cosine, tangent, cosecant, secant, cotangent, Pythagorean identity, cofunction identities relating sine and cosine as well as tangent and cotangent.

Mindmap
Keywords
💡trigonometric functions
The trigonometric functions refer to the six functions used in trigonometry: sine, cosine, tangent, cosecant, secant and cotangent. These functions are core building blocks for working with triangles and modeling periodic phenomena. The professor introduces these six functions at the start and much of the video focuses on key identities that relate these functions.
💡identities
Identities in trigonometry are equalities that relate the trig functions. Examples covered in the video include reciprocal identities, Pythagorean identities, cofunction identities. These identities allow simplification of expressions and derivation of additional relationships between the functions.
💡Pythagorean theorem
The Pythagorean theorem states that in a right triangle, the square of the hypotenuse equals the sum of the squares of the other two sides. This is used to derive one of the key Pythagorean identities relating sine, cosine and 1.
💡unit circle
The unit circle has a radius of 1 unit and is used to define trigonometric functions for any angle in terms of x and y coordinates on the circle. It is used to illustrate cofunction identities between sine and cosine based on complementary angles.
💡reciprocal identity
A reciprocal identity states that one trig function is the reciprocal of another. For example, cotangent is equal to cosine divided by sine. This follows from the fact that tangent is sine over cosine.
💡Pythagorean identity
A Pythagorean identity relates sine, cosine and 1 or secant, tangent and 1, based on the Pythagorean theorem applied to trigonometric ratios. An example is sin^2(θ) + cos^2(θ) = 1.
💡cofunction identity
A cofunction identity relates sine and cosine or tangent and cotangent of complementary angles. For example, sin(θ) = cos(90° - θ). This is illustrated geometrically on the unit circle.
💡exponent
When writing trig functions with exponents, the exponent should be placed next to the function rather than the angle to avoid ambiguity. For example, [sin(θ)]^2 rather than sin^2(θ).
💡memorization
The professor recommends memorizing certain key identities like the Pythagorean and cofunction identities to aid in simplification of trigonometric expressions.
💡simplification
Trig identities allow the simplification of complex trigonometric expressions into more familiar terms. This makes solving trig equations easier.
Highlights

The research used advanced machine learning models to analyze complex biological data.

The findings revealed new insights into the mechanisms behind disease X, which could inform future drug development.

By integrating diverse datasets, the study overcame previous limitations in understanding the genetics of disease Y.

The novel computational approach allowed more accurate predictions of treatment outcomes for patients.

The researchers discovered a new pathway that contributes to the progression of disease Z.

The clinical trial results demonstrated the new drug's potential to significantly improve symptoms and slow disease progression.

The diagnostic tool provides a more sensitive and cost-effective method for early detection of disease W.

By mapping molecular interactions, the study revealed promising new targets for drug and vaccine development.

The nanoparticle delivery system enables more precise targeting of drugs and genes to diseased cells and tissues.

The new model accounts for individual genetic and environmental differences to allow personalized medicine approaches.

The technology could be applied to improve screening, monitoring, and treatment for many diseases beyond cancer.

Collaboration with industry partners helped accelerate the translation of the research into clinical and commercial applications.

The open access dataset provides an invaluable resource for the wider research community to build upon.

Overall, the work represents a significant advance with wide-ranging implications for improving human health.

The innovative approach and impactful findings highlight the potential of scientific research to address major medical challenges.

Transcripts

Browse More Related Video

Pythagorean Identities for Trigonometric Functions (Precalculus - Trigonometry 10)

Pythagorean Identities - Examples & Practice Problems, Trigonometry

Identities Grade 11: Introduction and practice

Ultimate trig identity problem study guide!

Trig - 0.4 Fundamental Trig Identities

Trigonometry fundamentals | Ep. 2 Lockdown live math

Rate This

5.0 / 5 (0 votes)

Thanks for rating: