Evaluating Indefinite Integrals

Professor Dave Explains
30 Apr 201810:44
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains indefinite integrals, which are integrals with no limits of integration. Indefinite integrals represent families of functions known as antiderivatives. While definite integrals evaluate to numbers, indefinite integrals evaluate to functions that contain an arbitrary constant C. The properties of indefinite integrals mirror those of definite integrals. Examples demonstrate how to find antiderivatives of various functions, including special cases like e^x and 1/x. The summary highlights key differences between definite and indefinite integrals.

Takeaways
  • 😀 Indefinite integrals have no limits of integration, so they represent functions or families of functions rather than numbers.
  • 😲 The main difference between definite and indefinite integrals is that definite integrals evaluate to numbers while indefinite integrals evaluate to functions.
  • 📝 When finding indefinite integrals, we must include the constant C at the end to account for all possible antiderivatives.
  • 👍 Properties of definite integrals like the sum rule and power rule still apply for indefinite integrals.
  • 🔍 Indefinite integrals require finding antiderivatives, just like evaluating definite integrals.
  • 🧠 You must memorize that the integral of 1/x is ln(x), not x^-1, which is an exception to the power rule.
  • ⚠️ Watch out for cases where you need to manipulate the integrand before integrating, like multiplying out roots or flipping exponents.
  • 🤯 The integral of e^x is e^x, because e^x is the only function that is unchanged by differentiation.
  • ✏️ Practicing polynomial indefinite integrals helps get a feel for the process.
  • 📺 Definite integrals give areas under curves over specific intervals, indefinite integrals give functions to generate those areas.
Q & A

  • What is the main difference between a definite integral and an indefinite integral?

    -A definite integral evaluates the antiderivative over a specific interval and results in a number, while an indefinite integral has no limits of integration specified and results in a function or family of functions.

  • Why do we have to include + C when writing indefinite integrals?

    -We include + C because there are infinitely many antiderivatives, differing only by a constant. The constant C represents any possible constant that could be added.

  • What is the rule for integrating e^x?

    -The integral of e^x is e^x. It is the only function that does not change when differentiated or integrated.

  • What is the integral of 1/x?

    -The integral of 1/x is ln|x|. This is an exception to the general rule for integrals of negative exponents.

  • Can the properties of definite integrals, like the constant rule, be applied to indefinite integrals?

    -Yes, the properties of indefinite integrals are precisely the same as those for definite integrals.

  • How do you integrate a multiplication of functions?

    -For a multiplication of functions, you cannot directly apply the product rule. Instead, you must distribute or expand the terms first.

  • What are some types of functions besides polynomials that we may need to integrate?

    -We may need to integrate exponential, logarithmic, trigonometric, and other special functions. We have to memorize key derivatives and integrals.

  • Why is the process of integration referred to as going up the ladder?

    -Integration is the opposite of differentiation - finding the antiderivative. We can visualize this process as reversing down the ladder of derivatives back up to the original function.

  • What is the fundamental theorem of calculus in relation to integrals?

    -The fundamental theorem of calculus states that the derivative of the integral of a function is equal to the original function. Integration and differentiation are inverse processes.

  • What are some strategies to make integrating complex functions easier?

    -Strategies include: breaking integrals of sums/differences into multiple integrals, bringing constants outside the integral, changing forms of functions into more integrable forms, and identifying special functions with known integrals.

Outlines
00:00
📝 Defining Indefinite Integrals

This paragraph explains the difference between definite and indefinite integrals. Definite integrals evaluate to a number by integrating a function over a specified interval. Indefinite integrals have no limits, so they represent a family of functions or the antiderivative itself. The main takeaway is that indefinite integrals are functions, while definite integrals give numerical values.

05:05
😀 Finding Indefinite Integrals

This paragraph provides examples of finding indefinite integrals. Key points are that constants can be pulled out, sums/differences can be split up, and special rules apply for exponentials and 1/x. Must remember to include the constant C at the end to account for all possible antiderivatives. Practice problems integrate polynomials and rational functions.

Mindmap
Keywords
💡Antiderivative
An antiderivative is a function whose derivative is the original function being integrated. When evaluating definite integrals, we are finding the antiderivative of the function over a specific interval. Antiderivatives allow us to calculate areas under curves. The professor explains that indefinite integrals involve finding the antiderivative of a function, just like with definite integrals.
💡Indefinite integral
An indefinite integral has no limits of integration specified. Therefore, indefinite integrals represent entire families of functions, not specific numerical values. The professor contrasts indefinite integrals with definite integrals, which evaluate to numbers over a fixed interval. He explains indefinite integrals using the example of integrating f(x) to find F(x).
💡Constant of integration
When finding an indefinite integral, we must include an arbitrary constant C at the end. This is because there are infinitely many antiderivatives, differing only by a constant. The professor emphasizes that this constant is relevant for indefinite integrals but disappears when evaluating definite integrals over a fixed interval.
💡Properties of integration
The professor reviews properties like the constant rule, sum rule, and power rule that apply to both definite and indefinite integration. He notes these properties will be useful for evaluating more complex integrals.
💡Logarithmic functions
When integrating 1/x, the antiderivative is ln(x), not x^(-1). The professor notes this is an exception to remember. It relates to the fact that the derivative of ln(x) is 1/x.
💡Exponential functions
The professor notes that e^x is unique in that its derivative is itself. Therefore, the integral of e^x is also e^x. This contrasts with other functions changing form between differentiation and integration.
💡Fundamental theorem of calculus
This theorem connects differentiation and integration, stating that the derivative of an integral (antiderivative) gives back the original function. The professor relates this to how integration undoes differentiation.
💡Area under a curve
The professor reviews how the original motivation for integrals was to find areas under curves. Definite integrals evaluate to numerical area values. Indefinite integrals represent functions whose areas we can calculate.
💡Integration techniques
The professor notes there are many types of functions requiring different integration techniques. After covering basic polynomials, he previews more complex functions like logarithms and exponentials that will need special methods.
💡Antiderivative
An antiderivative is a function whose derivative is the original function being integrated. When evaluating definite integrals, we are finding the antiderivative of the function over a specific interval. Antiderivatives allow us to calculate areas under curves. The professor explains that indefinite integrals involve finding the antiderivative of a function, just like with definite integrals.
Highlights

The use of next-generation sequencing has revealed new insights into the genetic basis of autism spectrum disorder.

Whole-exome sequencing has identified de novo mutations and novel candidate genes that may contribute to autism risk.

Studies of autism-related copy number variants have shown recurrent deletions and duplications that impact neurodevelopment.

Analysis of autism brain tissue has revealed abnormalities in neuronal organization, dendritic spine density, and gene expression patterns.

The gut microbiome differs in composition between children with and without autism, suggesting a potential role of the microbiome.

Prenatal exposure to certain medications, infections, and air pollution have been associated with increased autism risk.

Early behavioral interventions can improve social communication and language outcomes for many children with autism.

Brain imaging reveals atypical neural connectivity and activity in autism, especially in social brain networks.

Autism has a strong genetic component, but environment and gene-environment interactions also contribute to risk.

The heterogenous manifestations of autism likely arise from various combinations of genetic, environmental, and neurobiological factors.

Animal models have provided insights into the neural and molecular mechanisms underlying autism-related behaviors.

There are promising advances in biomarkers to enable earlier autism diagnosis and personalized treatment approaches.

Understanding autism as a spectrum disorder with multiple etiologies will be key for developing targeted interventions.

Further research into gene-environment interactions can elucidate critical windows of vulnerability and reversibility.

A holistic, integrative approach is needed to understand the complex interplay between the many factors involved in autism.

Transcripts

Browse More Related Video

What's the difference between a DEFINITE integral and an INDEFINITE integral? | CALCULUS

Calculus 1: Indefinite Integrals and the Net Change Theorem (Section 5.4) | Math with Professor V

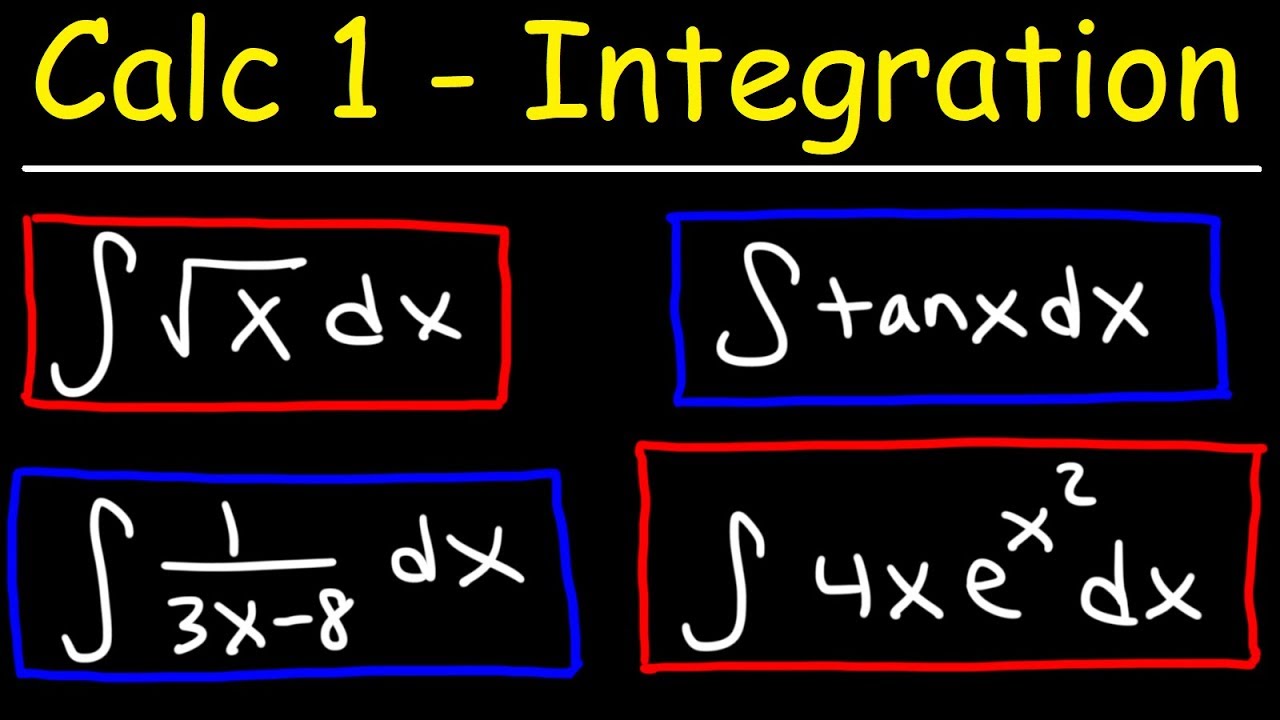
Calculus 1 - Integration & Antiderivatives

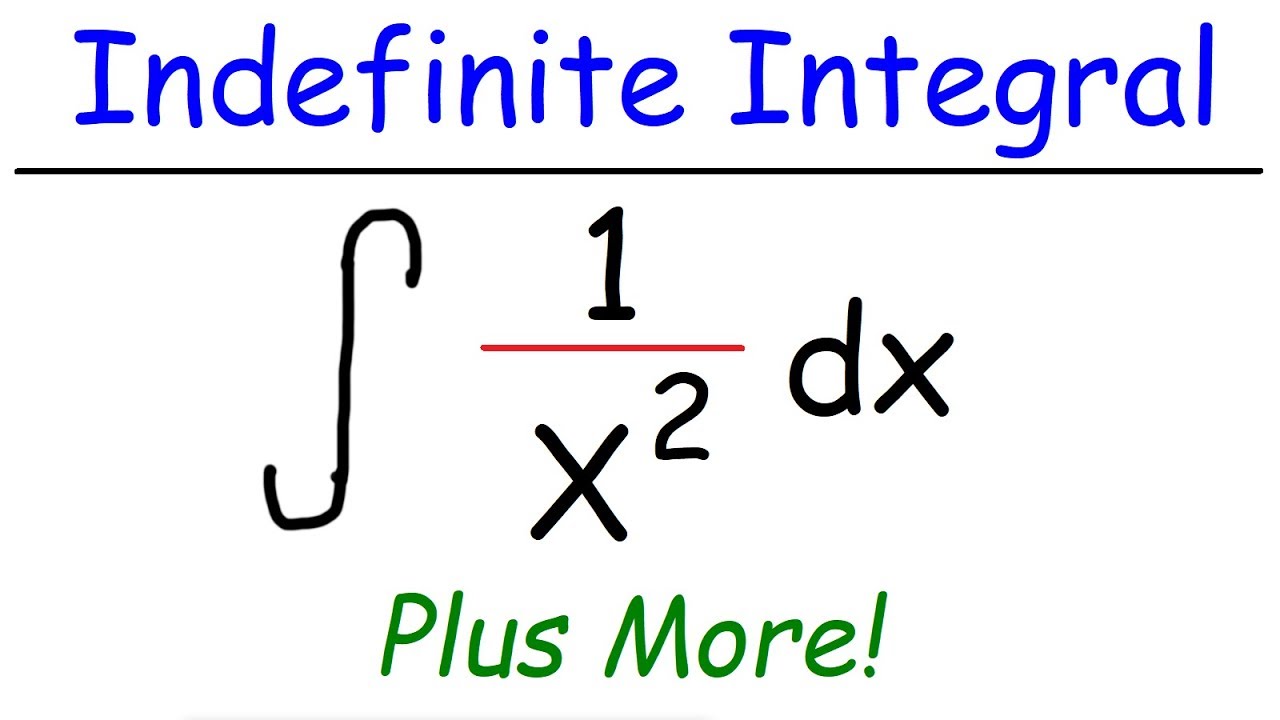
Indefinite Integral of 1/x^2

AP Calculus AB: Lesson 6.6 Fundamental Theorem of Calculus, Part 2

Definite Integrals — Topic 88 of Machine Learning Foundations

Rate This

5.0 / 5 (0 votes)

Thanks for rating: