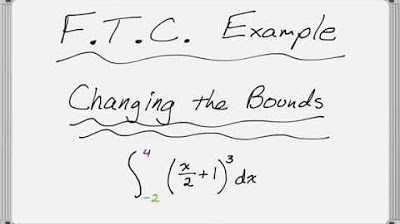Integration Using The Substitution Rule

Professor Dave Explains
7 May 201810:40
EducationalLearning
32 Likes 10 Comments

TLDRThe substitution rule is introduced as a technique to simplify complicated integrals by representing portions of the integral as a variable. For the rule to apply, the integral must be in the form f(g(x)) * g'(x)dx. To apply: replace g(x) with u so g'(x)dx becomes du; integrate f(u)du which is now simpler; then substitute u back to original form. Examples show applying substitution rule to simplify integrals with trig functions or roots inside. Substitution rule works like chain rule in reverse to ignore inner pieces during integration, though it doesn't always apply like chain rule does. Recognizing when substitution can simplify is key.

Takeaways
  • 📚 The substitution rule is a technique used in integration to simplify complex integrands by replacing a portion of them with a single variable, typically denoted as 'u'.
  • 📈 Integrating simple polynomials is straightforward, akin to taking derivatives, but not all integrations follow a simple, rigid algorithm.
  • 🔧 The substitution rule is useful when direct integration methods are not applicable due to the complexity of the integrand.
  • 📖 By substituting a part of the integrand (e.g., 'x squared plus one') with 'u', the integration process becomes simpler, as 'cosine of u' can be integrated more easily than 'cosine of (x squared plus one)'.
  • 📃 To apply the substitution rule, it's necessary to express the differential 'dx' in terms of 'du', aligning with the substitution made in the integrand.
  • 📊 The derivative of the function inside the substitution (e.g., 'x squared plus one') is used to find 'du', which replaces 'dx' in the integral.
  • 📝 Integrating with the substitution rule might involve algebraic manipulation, such as adjusting coefficients to match the differential 'du' with the integrand.
  • 📉 The substitution rule can be thought of as the reverse of the chain rule, symbolizing a portion of the function with a single variable to simplify integration.
  • 📱 This technique does not always work; it's effective when the integrand is in the form 'f of g of x times g prime of x', allowing for the substitution to simplify integration.
  • 🔬 Examples in the script illustrate how to apply the substitution rule in different scenarios, including integrating functions with trigonometric expressions and polynomials.
Q & A

  • What is the substitution rule in integration?

    -The substitution rule allows us to take a complicated integrand and simplify it by representing some portion of it with the variable u.

  • When can we apply the substitution rule?

    -We can apply the substitution rule when the integrand is in the form f(g(x)) * g'(x) dx. In this case we can let u = g(x) and du = g'(x) dx.

  • How does the substitution rule relate to the chain rule?

    -The substitution rule can be thought of as the reverse of the chain rule. With the chain rule we break down a composite function into inner and outer functions. With substitution we symbolize part of the function to simplify integration.

  • Why do we sometimes need to manipulate dx algebraically when applying substitution?

    -We may need to manipulate dx to change it to du so that the differential agrees with the new integrand in terms of u. We will justify this manipulation later in the series.

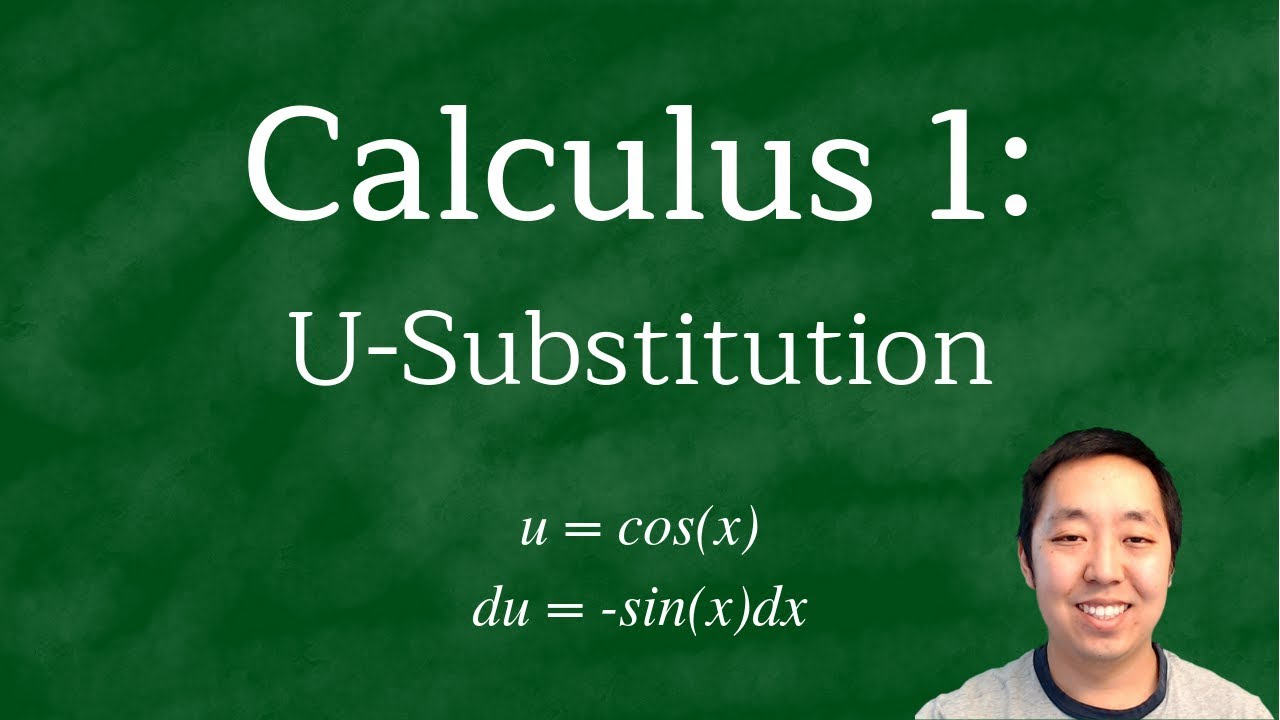
  • When integrating tangent x dx, why did we choose cosine x as our u?

    -Tangent is sine over cosine. Knowing the derivative of cosine is negative sine, substituting u = cosine x and du = -sine x dx allowed us to simplify the integrand into a form we could integrate.

  • Why do we sometimes need absolute value brackets with ln(u)?

    -The natural log can only take positive values. If u represents a trig function like cosine, it may sometimes be negative. The absolute value brackets ensure we take the ln of the positive value.

  • What are some cases where the substitution rule would not apply?

    -The substitution rule only works when the integrand contains the derivative of the substituted function multiplied by dx. If this structure is not present, substitution will not simplify the integral.

  • If an integral contains multiple terms, can I apply substitution to only part of it?

    -Yes, you can apply substitution to simplify only part of an integral if the structure matches the necessary form. The remaining portions can then be integrated normally.

  • Does the substitution variable u need to represent the entire function?

    -No, u can represent any part of the function as long as you can determine du/dx and substitute it into the integrand appropriately.

  • What should I do if I don't see how substitution could simplify an integral?

    -If the integrand does not contain the derivative of a suitable function, substitution will not help. Move on to trying other integration techniques like integration by parts.

Outlines
00:00
📘 Introduction to the Substitution Rule

This segment introduces the substitution rule as a fundamental technique for solving complex integrals that do not adhere to straightforward algorithms. Professor Dave explains the concept by taking the example of integrating a function involving cosine and a polynomial. The substitution rule simplifies the integrand by replacing a portion of it with a variable 'u', thereby transforming the integral into a more manageable form. This method hinges on identifying an appropriate substitution that simplifies the integrand and its differential, illustrating the process with the integral of 2x times cosine (x squared plus one). The explanation covers the algebraic manipulation of differentials and the integration process, concluding with a verification step through differentiation. This approach is likened to the reverse application of the chain rule, emphasizing the conditional applicability of the substitution rule based on the form of the integrand.

05:07
📐 Applying the Substitution Rule with Examples

The second paragraph delves into further applications of the substitution rule, showcasing its versatility across different types of integrals. Starting with an example involving a square root function, Professor Dave demonstrates the process of identifying an appropriate substitution, handling differentials, and simplifying the integral for easier computation. The narrative then transitions to examples involving trigonometric functions, illustrating the rule's effectiveness in simplifying integrals of sine to the sixth power times cosine and the integral of tangent. These examples highlight the strategic use of substitution to manipulate the integral into a form where direct integration is possible, culminating in simplified solutions. The segment emphasizes the importance of recognizing when and how the substitution rule can be applied, serving as a critical tool in the integration toolkit.

Mindmap
Keywords
💡substitution rule
The substitution rule is the main topic of the video. It is a method to simplify and evaluate integrals by substituting part of the integrand with a new variable. For example, replacing x^2 + 1 with u. This can transform complicated integrals into easier forms that can be integrated.
💡integrate
Integration is calculating the indefinite integral or antiderivative of a function. The video focuses on using substitution as a technique to integrate functions that may not be integrable using basic rules.
💡integrand
The integrand is the function that appears inside the integral sign that we need to integrate. The goal of substitution is to simplify the form of the integrand to make integration easier.
💡derivative
Taking the derivative of the function we substituted with the new variable gives us the differential for the new variable. For example, the derivative of x^2 + 1 is 2x, so du = 2x dx.
💡differential
The differential allows us to substitute dx in the integrand with du after making the variable substitution. This completes setting up the integral with the new substitution.
💡chain rule
The lecturer relates substitution as the reverse of the chain rule. Just as the chain rule breaks down differentiation into simple steps, substitution breaks down integration into simpler parts.
💡evaluate
After using substitution and integration, the final step is to evaluate by substituting the new variable back to the original complex function. For example, replace u with x^2 + 1.
💡simplify
The main purpose of using substitution for integration is to simplify complicated integrals into easier forms. This allows us to evaluate integrals that we couldn't otherwise using basic rules.
💡trigonometric functions
The substitution method can be applied to integrate trigonometric functions, like sine, cosine and tangent. The lecturer provides examples of substituting sine(x) and cosine(x) to integrate related integrals.
💡indefinite integral
We are calculating indefinite integrals using substitution, meaning the integrals have a constant of integration rather than numerical bounds. This gives the most general form of the antiderivative function.
Highlights

The study found evidence that cognitive behavior therapy can be effective for treating anxiety disorders.

Researchers developed a new theoretical model to explain the role of rumination in depression.

Statistical analysis revealed a significant correlation between social media use and symptoms of depression.

MRI scans showed reduced activity in the prefrontal cortex of patients with obsessive compulsive disorder.

The review highlighted the need for more research on the long-term efficacy of pharmacotherapy for ADHD.

Interviews with patients provided insights into the subjective experience of living with schizophrenia.

Genome-wide association studies found new genetic variants linked to increased risk of bipolar disorder.

Mindfulness meditation was shown to reduce symptoms of anxiety and depression in cancer patients.

The drug trial demonstrated improved memory and cognition in patients with Alzheimer's disease.

Researchers proposed changes to diagnostic criteria for personality disorders in the DSM-5.

Data revealed concerning increases in cases of adolescent depression and suicidal ideation.

Experts emphasized the importance of early intervention and accessible mental health resources.

The study found no evidence to support the use of antidepressants in children and teens.

Researchers called for more rigorous evaluation of popular mobile apps for mental health.

Experts discussed strategies to reduce stigma and improve mental health literacy globally.

Transcripts

Browse More Related Video

The Chain Rule

Integration by Substitution

Fundamental Theorem of Calculus: Changing the Bounds with u-Substitution

Find The Definite Integral Of x3 Into 3 Plus x Power 4 All Power -2 [Evaluate The Definite Integral]

Calculus 1: U-Substitution Examples

Email Answer about When To Use U-Sub

Rate This

5.0 / 5 (0 votes)

Thanks for rating: