Chapter 2 - Motion Along a Straight Line

MU Physics and Astronomy

24 Aug 201337:24

EducationalLearning

32 Likes 10 Comments

TLDRThe transcript discusses the fundamentals of motion along a straight line in the context of mechanics, focusing on kinematics and dynamics. It introduces key concepts such as position, velocity, and acceleration, explaining their definitions, units, and how they relate to one another. The distinction between average speed and velocity is clarified, and the mathematical treatment of velocity and acceleration using calculus is explored. The script also delves into the concept of constant acceleration motion, specifically freefall, and the terminal velocity achieved when air resistance balances gravitational acceleration.

Takeaways

📚 Mechanics is the study of how things work, particularly focusing on motion.
🏃 Kinematics is a subfield of mechanics that deals with motion without considering the causes.
📈 Position (X), velocity (V), and acceleration (A) are the three key quantities of interest in kinematics.
📊 The reference frame is essential for describing position, often using a coordinate system with X and Y axes.
🚀 Average speed is the total distance traveled divided by the total time taken, without considering direction.
🌟 Velocity is a vector quantity that describes both the rate of change of position and the direction of motion.
📉 Instantaneous velocity can be found graphically by calculating the slope of the tangent to the position-time graph at a specific point.
🧮 Acceleration is the rate of change of velocity over time and can be calculated using calculus or by applying the kinematic equations.
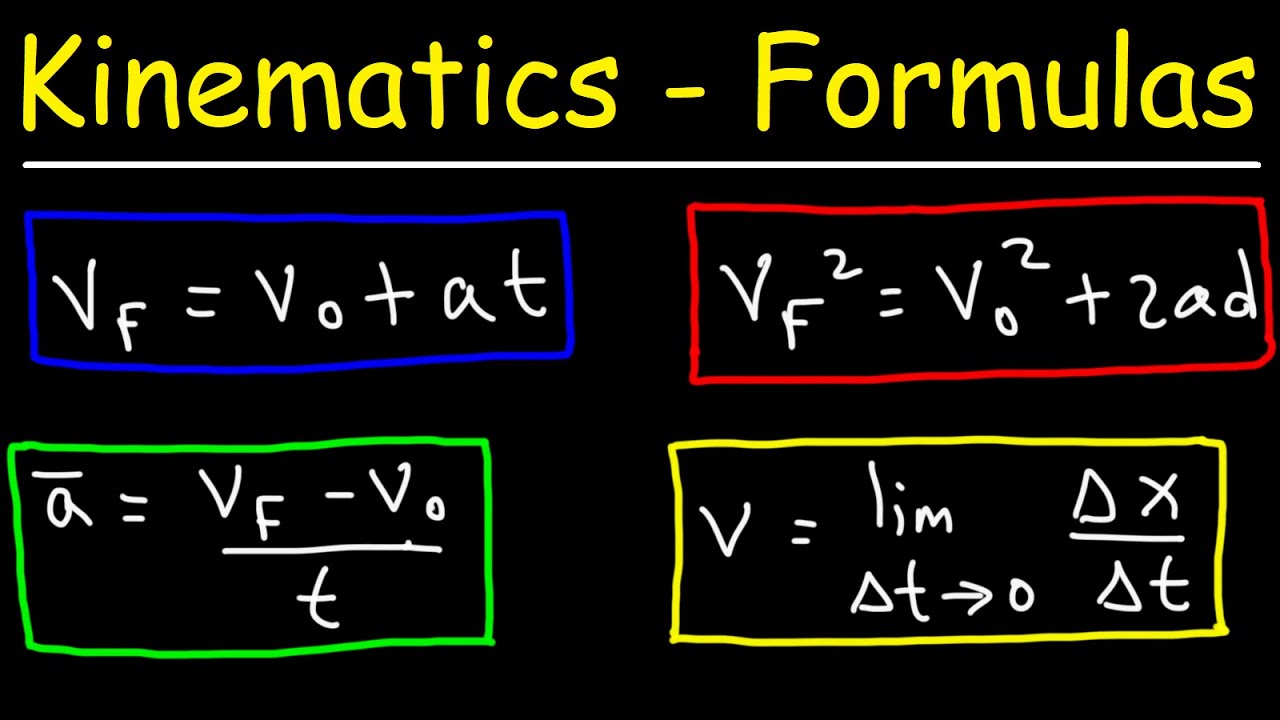
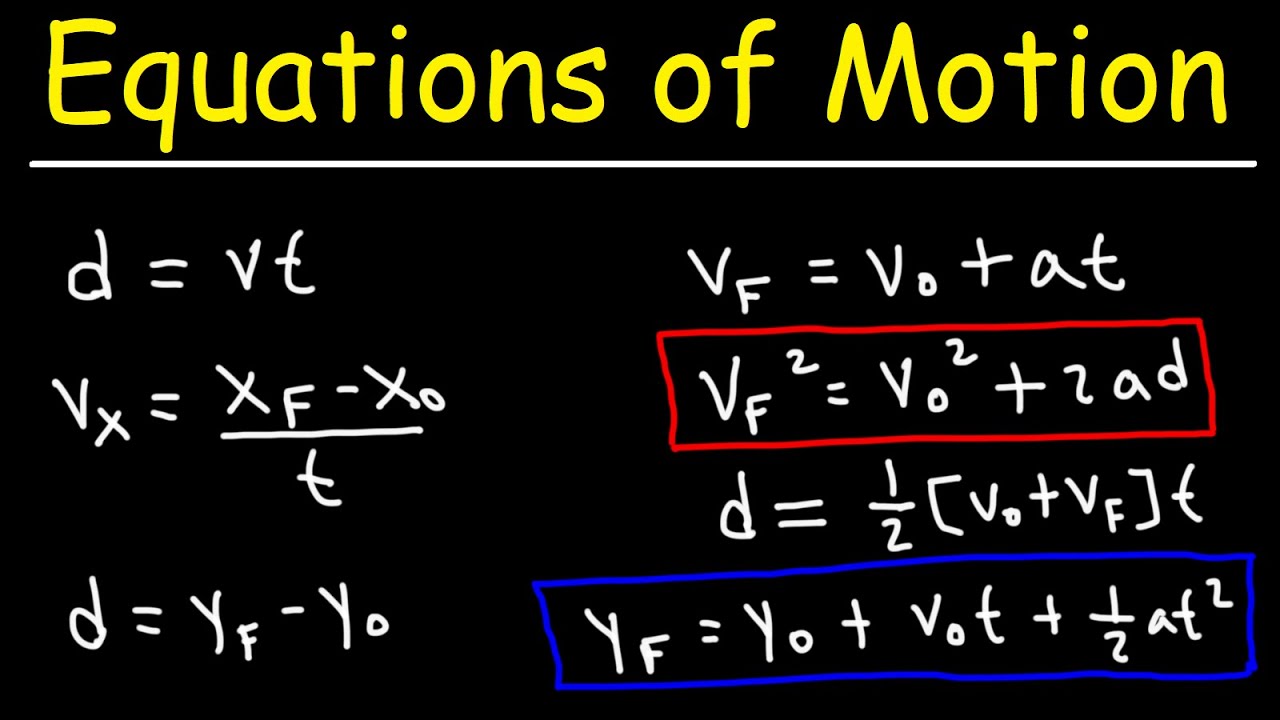
🔄 Constant acceleration motion problems can be solved using a set of five kinematic equations that relate position, velocity, and time.
💫 Freefall is a special case of constant acceleration motion where the only force acting is gravity.
🪂 Terminal velocity occurs when air resistance balances the force of gravity, resulting in constant velocity with no further acceleration.

Q & A

What is the main topic of Chapter 2?
-The main topic of Chapter 2 is motion along a straight line, focusing on the concepts of mechanics and kinematics.
What is the difference between kinematics and dynamics?
-Kinematics studies the motion of objects without considering the forces causing the motion, while dynamics investigates the forces, such as gravity, that cause changes in motion.
What are the three quantities of interest in kinematics?
-The three quantities of interest in kinematics are position (X), velocity (V), and acceleration (A).
How is position represented in kinematics?
-Position is represented by the coordinate X, which describes the location of an object in a reference frame, typically using a coordinate system with an X and Y axis.
What is the definition of average speed?
-Average speed is the total distance traveled divided by the total time taken to travel that distance, without considering any stops or changes in direction during the trip.
How is velocity different from speed?
-Velocity is a vector quantity that describes both the rate of motion (speed) and the direction of movement, while speed is a scalar quantity that only describes the rate of motion without direction.
How can you find the average velocity between two points graphically?
-You can find the average velocity between two points graphically by calculating the slope of the line connecting those two points on a position versus time graph. The slope represents the change in position (ΔX) divided by the change in time (ΔT).
What is the definition of instantaneous velocity?
-Instantaneous velocity is the velocity of an object at a very specific point in time. It can be found graphically by looking at the slope of the tangent to the curve at that point or mathematically using calculus to find the limit as the time interval approaches zero.
How is acceleration defined in the context of constant acceleration motion?
-In the context of constant acceleration motion, acceleration is defined as the change in velocity (ΔV) divided by the change in time (ΔT) or as the second derivative of position with respect to time (d²x/dt²).
What are the four kinematic equations used for constant acceleration motion?
-The four kinematic equations for constant acceleration motion are: 1) v = v₀ + at, 2) x = x₀ + v₀t + 1/2at², 3) x - x₀ = v₀t + 1/2at², and 4) v² - v₀² = 2ax.
What is the concept of terminal velocity?
-Terminal velocity is the constant velocity reached when air resistance or friction balances the acceleration due to gravity, resulting in no further increase in speed as an object falls.

Outlines

00:00

📚 Introduction to Mechanics and Kinematics

This paragraph introduces the concept of mechanics, focusing on the study of motion and the forces that cause it, particularly within the subfield of kinematics. It explains the importance of understanding position (denoted as X) in a reference frame, typically using a coordinate system with X and Y axes. The paragraph also introduces velocity (labeled as V) and acceleration (labeled as A) as key quantities of interest in kinematics, setting the stage for further discussion on these topics.

05:03

📈 Understanding Velocity through Graphs and Equations

The paragraph delves into the concept of velocity, differentiating between average speed and average velocity. It explains how velocity is calculated using the change in position (ΔX) over time (ΔT) and introduces the units of velocity as meters per second (m/s). The explanation includes a graphical approach to finding velocity by calculating the slope of a position versus time graph. The paragraph also touches on the mathematical representation of velocity and the significance of positive and negative values in indicating direction.

10:04

🔢 Calculating Instantaneous Velocity and Acceleration

This section discusses the concept of instantaneous velocity, which is the velocity at a specific instant in time. It explains how to find this graphically by looking at the slope between two points that are very close together on a position-time graph. The paragraph then transitions to a mathematical approach using calculus to find the limit as ΔT approaches zero, which defines instantaneous velocity as the derivative of position with respect to time. The concept of acceleration is also introduced as the rate of change of velocity over time, with both average and instantaneous definitions provided.

15:05

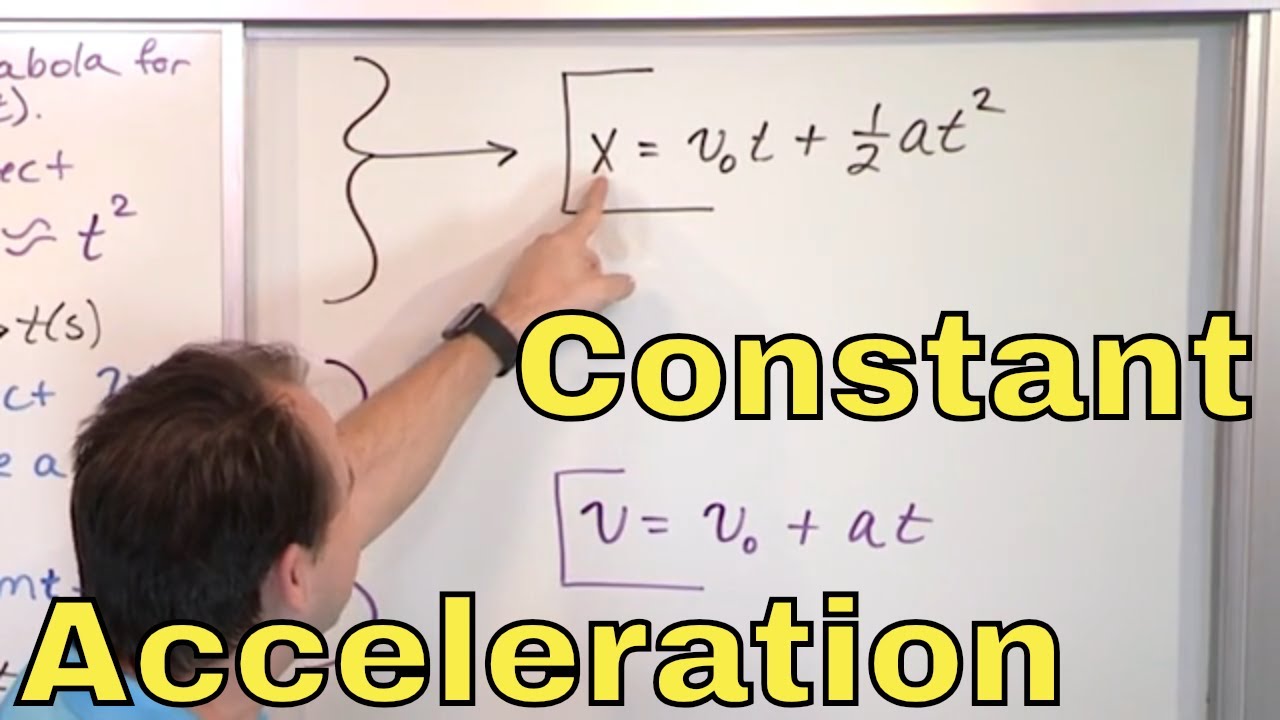
📚 Deriving Kinematic Equations using Calculus

The paragraph focuses on deriving kinematic equations using calculus. It starts by defining velocity as the first derivative of position with respect to time and then moves on to find the velocity function for a given position function. The paragraph also explains how to find acceleration by taking the derivative of velocity with respect to time. The section concludes with a self-consistency check by deriving one of the kinematic equations from the derived velocity function and confirming it matches the expected result.

20:07

🌐 Freefall: A Special Case of Constant Acceleration

This paragraph discusses freefall as a special case of constant acceleration, where the only force acting is gravity. It explains the concept of gravitational acceleration (denoted as 'g') and its direction relative to the chosen coordinate system. The paragraph modifies the standard kinematic equations for motion in the vertical (Y) direction, accounting for the negative value of 'g' to represent downward acceleration. The concept of terminal velocity is introduced, where air resistance balances the gravitational pull, resulting in constant velocity without further acceleration.

Mindmap

Keywords

💡Mechanics

Mechanics is a branch of physics that deals with the motion of objects and the forces that cause these motions. In the context of the video, it is used to introduce the study of how objects move, particularly in relation to the concepts of kinematics and dynamics. The video explains that while kinematics focuses on the motion without considering the causes, dynamics will later be introduced to explore the forces behind the motion.

💡Kinematics

Kinematics is the branch of classical mechanics that describes the motion of points, bodies (objects), and systems of bodies without consideration of the forces that cause the motion. In the video, kinematics is introduced as the study of motion along a straight line, focusing on quantities such as position, velocity, and acceleration without considering the causes of these motions, which will be covered in dynamics.

💡Position (X)

In the context of the video, position (denoted as X) refers to the location of an object in a reference frame, typically along a horizontal axis. It is a fundamental quantity in kinematics, describing where an object is at a particular moment in time. The reference frame is essential for defining position, as it provides the coordinate system within which the object's location is measured.

💡Velocity (V)

Velocity (denoted as V) is a measure of the rate of change of position with respect to time. It describes both the speed (how fast an object is moving) and the direction of the object's motion. In the video, average velocity is calculated as the change in position (ΔX) divided by the change in time (ΔT), providing a measure of how quickly an object's position changes over a certain time interval.

💡Acceleration (A)

Acceleration (denoted as A) is the rate of change of velocity with respect to time. It quantifies how quickly the velocity of an object changes. In the video, acceleration is defined using calculus concepts, as the limit of the change in velocity (ΔV) over the change in time (ΔT) as ΔT approaches zero, or equivalently, as the derivative of velocity with respect to time.

💡Reference Frame

A reference frame is a set of criteria or a coordinate system that is used to measure and observe the motion of objects. In the video, the reference frame is established by the X and Y axes, which are used to define the position (X) of an object moving along a straight line. The choice of reference frame is crucial for accurately describing the motion of an object.

💡Average Speed

Average speed is the total distance traveled divided by the total time taken to travel that distance. Unlike average velocity, average speed does not take direction into account and only considers the magnitude of the distance covered. In the video, average speed is introduced as a concept to understand the basic idea of how fast an object is moving over a period of time, without considering the path taken.

💡Instantaneous Velocity

Instantaneous velocity is the velocity of an object at a specific moment in time. It is a more precise measure than average velocity, which considers the overall motion over a time interval. Instantaneous velocity can be determined graphically by finding the slope of the tangent to the position-time graph at a particular point, or mathematically by taking the derivative of the position function with respect to time.

💡Freefall

Freefall is the motion of an object falling under the sole influence of gravity, without any other forces acting on it (such as air resistance). In the video, freefall is used as an example of constant acceleration motion, where the acceleration due to gravity (denoted as 'g') causes the object to accelerate towards the Earth. The direction of freefall is typically considered negative when the upward direction is defined as positive.

💡Terminal Velocity

Terminal velocity is the constant speed that an object reaches when the air resistance (or drag force) acting on it balances the gravitational force pulling it downward. At terminal velocity, the object no longer accelerates and continues to fall at a constant rate. In the video, terminal velocity is introduced as the point when air friction balances the acceleration due to gravity, resulting in a skydiver falling at a constant velocity.

Highlights

Introduction to mechanics and the concept of motion along a straight line.

Definition of kinematics and its distinction from dynamics.

Explanation of the three quantities of interest in kinematics: position, velocity, and acceleration.

Description of position as a vector quantity with reference to a frame of reference.

Discussion on average speed versus average velocity, highlighting the importance of direction in velocity.

Introduction to the concept of instantaneous velocity and its practical applications.

Explanation of how to graphically determine velocity from a position-time graph.

Derivation of the kinematic equations for constant acceleration motion.

Illustration of how to find velocity and acceleration using calculus.

Discussion on the direction of acceleration and its relation to speeding up or slowing down.

Introduction to the concept of freefall and the acceleration due to gravity (G).

Explanation of how the direction of G is negative in freefall problems due to the chosen coordinate system.

Description of terminal velocity as the point where air friction balances gravitational acceleration.

Example of a skydiver to illustrate the concept of terminal velocity in real-life scenarios.

Discussion on the importance of understanding the signs of velocity and acceleration in determining direction.

Explanation of how to calculate the slope of a position-time graph to find velocity.

Clarification on the difference between scalar quantities like speed and vector quantities like velocity.

Use of calculus to find the derivative of position with respect to time to determine velocity.

Transcripts

Browse More Related Video

Kinematics in one dimension

Kinematics Physics Formulas

Equations of Motion

Kinematics Part 1: Horizontal Motion

8.01x - Lect 2 - 1D Kinematics - Speed, Velocity, Acceleration

01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

Related Tags

PhysicsEducation Mechanics101 Kinematics Velocity Acceleration FreeFall TerminalVelocity ConceptExplanation MathApplications ScienceLecture EducationalContent