iit foundation class 8 maths Linear equations in one #maths
TLDRThe script discusses the concept of linear equations in one variable, defining them as equations expressible in the form of a + b = 0. It introduces the process of solving such equations using methods like transposition and cross-multiplication. The importance of understanding the sign conventions when transposing terms from one side of the equation to the other is emphasized. The script also explains the cross-multiplication method, demonstrating how to solve equations through step-by-step examples and highlighting the need for balancing constants and variables on each side of the equation.
Takeaways
- 📚 The script discusses linear equations in one variable, defined as equations expressible in the form a + b = 0.
- 🔢 The variable in a linear equation in one variable is represented by 'x', and the constant term is represented by 'b'.
- 💡 A linear equation in one variable will have a single solution, as the variable 'x' is isolated on one side of the equation.
- 🌟 The process of solving a linear equation in one variable can be achieved through various methods, including transposition, cross-multiplication, and balancing.
- ✏️ Transposition involves moving terms from one side of the equation to the other, which changes the sign of the term being moved.
- 🔄 Cross-multiplication is a technique used to eliminate variables by multiplying both sides of the equation by a common factor.
- ⚖️ Balancing refers to the process of adjusting the equation so that both sides are equal, which may involve combining like terms and ensuring the equation is simplified.
- 📌 The script emphasizes the importance of understanding the concepts of 'coefficients' (denoted as 'a') and 'constant terms' (denoted as 'b') in solving equations.
- 📈 The process of solving equations is demonstrated through an example, showing how to isolate 'x' and find its value by simplifying the equation.
- 🔍 The script highlights the need for clear understanding and application of mathematical rules, such as sign conventions when transposing terms.
- 🚦 The script mentions that solving linear equations in one variable is foundational and necessary for understanding more complex mathematical concepts.
- 📝 The script serves as a tutorial, guiding the reader through the steps of solving linear equations and emphasizing the importance of practice and repetition for mastery.
Q & A
What is the definition of a linear equation in one variable?
-A linear equation in one variable is an equation that can be expressed in the form of a + b = 0, where 'a' and 'b' are terms involving the variable, and the highest power of the variable is one.
What is the significance of the term 'coefficient' in the context of a linear equation?
-The coefficient in a linear equation refers to the numerical factor that multiplies the variable. It determines the slope of the line in the linear equation's graphical representation.
What is the role of the constant term in a linear equation?
-The constant term in a linear equation is the term that does not involve the variable. It shifts the line represented by the equation vertically on the graph and is crucial in determining the y-intercept of the line.
What happens to the solution of a linear equation when you transpose terms?
-When you transpose terms in a linear equation, the sign of the term changes if it is moved from the left side to the right side or vice versa. This process is essential for solving the equation and isolating the variable.
What is the elimination method in the context of solving linear equations?
-The elimination method is a technique used to solve systems of linear equations. It involves adding or subtracting equations to eliminate one variable, making it easier to find the solution for the remaining variable.
What is the cross-multiplication method and when is it used?
-The cross-multiplication method is a technique used to solve proportions or to reduce fractions. It involves multiplying terms diagonally across the equation and then adding the products to one side to simplify and find the solution.
How does the balancing rule affect the process of solving linear equations?
-The balancing rule is crucial in ensuring that both sides of a linear equation remain equal after operations like transposing or combining like terms. It helps maintain the integrity of the equation and leads to the correct solution.
What is the purpose of the 'transposition' process in solving linear equations?
-The purpose of the 'transposition' process is to rearrange the terms of the equation to isolate the variable on one side. This is typically done by moving variable terms to one side of the equation and constant terms to the other, which simplifies the process of solving for the variable.
What is the expected outcome when a linear equation in one variable is solved correctly?
-When a linear equation in one variable is solved correctly, the outcome is a value for the variable that makes both sides of the equation equal. This value is known as the solution to the equation.
Why is it important to maintain the balance of an equation during the solving process?
-Maintaining the balance of an equation is crucial because it ensures that the solution remains valid. Any operation performed on one side of the equation must be performed on the other side as well to preserve equality, leading to an accurate solution.
What does it mean when there is only one solution for a linear equation in one variable?
-When there is only one solution for a linear equation in one variable, it means that there is a unique value for the variable that satisfies the equation. This is typical for linear equations, as they represent straight lines, which intersect the y-axis at a single point.
Outlines
📚 Introduction to Linear Equations in One Variable
This paragraph introduces the concept of linear equations in one variable. It explains that a linear equation is an equation that can be expressed in the form a*x + b = 0, where 'a' and 'b' are constants, and 'x' is the variable. The speaker emphasizes that there will be only one solution to such an equation, and the process of solving it involves isolating the variable 'x'. The paragraph also touches upon the idea of 'equilibrium' in equations and the importance of understanding the basic form of linear equations before proceeding to solve them.
🔄 The Transposition Method for Solving Equations
The second paragraph delves into the process of solving linear equations using the transposition method. It describes how to shift terms from one side of the equation to the other, changing the sign of the terms in the process. The speaker explains the concept of 'transposition' in detail, including how to handle constant terms and variable terms when shifting them. The paragraph also introduces the idea of 'non-adjacent' terms and how their transposition affects the equation. The speaker reassures the listener that understanding the transposition method will help in solving equations effectively.
🤹 Balancing Equations: The Cross Multiplication Method
This paragraph introduces the cross multiplication method as a technique to solve linear equations. The speaker explains that this method involves multiplying terms across the equals sign and then balancing the equation by performing similar operations on both sides. The paragraph emphasizes the universal nature of this method and its application when dealing with equations that have variable terms. The speaker also provides a step-by-step guide on how to apply cross multiplication, including how to handle the coefficients and constant terms to arrive at the solution.
📈 Simplification and Solving: The Final Steps
The final paragraph focuses on the final steps of solving linear equations after applying the cross multiplication method. It discusses the process of simplifying the equation by reducing coefficients and handling constant terms. The speaker explains how to isolate the variable to find its value and emphasizes the importance of following the correct order of operations. The paragraph concludes with a reminder that understanding the balancing rules is crucial for solving equations and that the listener will learn more about balancing in the upcoming sections.
Mindmap
Keywords
💡Linear Equation
💡Variable
💡Coefficient
💡Constant Term
💡Transposition Method
💡Cross Multiplication Method
💡Balancing
💡Solving Equations
💡Sign Convention
💡Solution
💡Variables and Constants
Highlights
Linear equation in one variable is defined as an equation expressed in the form a + b = 0.
The variable in a linear equation in one variable is the variable that will have a single solution.
The constant term in the equation is the term without a variable.
The process of solving a linear equation in one variable involves methods such as transposition, cross-multiplication, and balancing.
Transposition involves shifting terms from one side of the equation to the other, which changes the sign of the term being moved.
Cross-multiplication is a method used to solve equations by multiplying terms across an equality sign.
Balancing is the third method mentioned, which involves adhering to four rules to maintain the equality during the solving process.
The concept of 'coefficient of x' is introduced, which is represented by the variable a in the equation a + b = 0.
The constant term in the equation is represented by b, which does not have a variable associated with it.
The process of solving a linear equation is likened to a journey, where understanding the methods is compared to having a clear map.
The impact of shifting terms from the left to the right side of the equation is explained, emphasizing the change in sign.
The transcript provides a step-by-step guide on how to apply transposition and cross-multiplication methods to solve equations.
The concept of 'equility' is discussed, which refers to the balance achieved when both sides of the equation are equal.
The transcript emphasizes the importance of understanding the concepts and methods to avoid problems when solving equations.
An example equation (2x + 4 = 10) is used to demonstrate the application of transposition and simplification methods.
The solution to the example equation is provided, showing that x equals 2, which is the only solution to the linear equation.
The transcript reassures the reader that with a clear understanding of the methods, solving the equations will not pose any difficulties.
The concept of 'cross-multiplication method' is introduced as a universal approach to solving equations.
The transcript explains the cross-multiplication process, emphasizing the importance of multiplying terms across the equality sign.
The process of simplifying equations is highlighted, showing how to reduce complex terms to simpler forms.
The transcript concludes with a discussion on balancing, which is the final step in solving linear equations in one variable.
Transcripts
Browse More Related Video
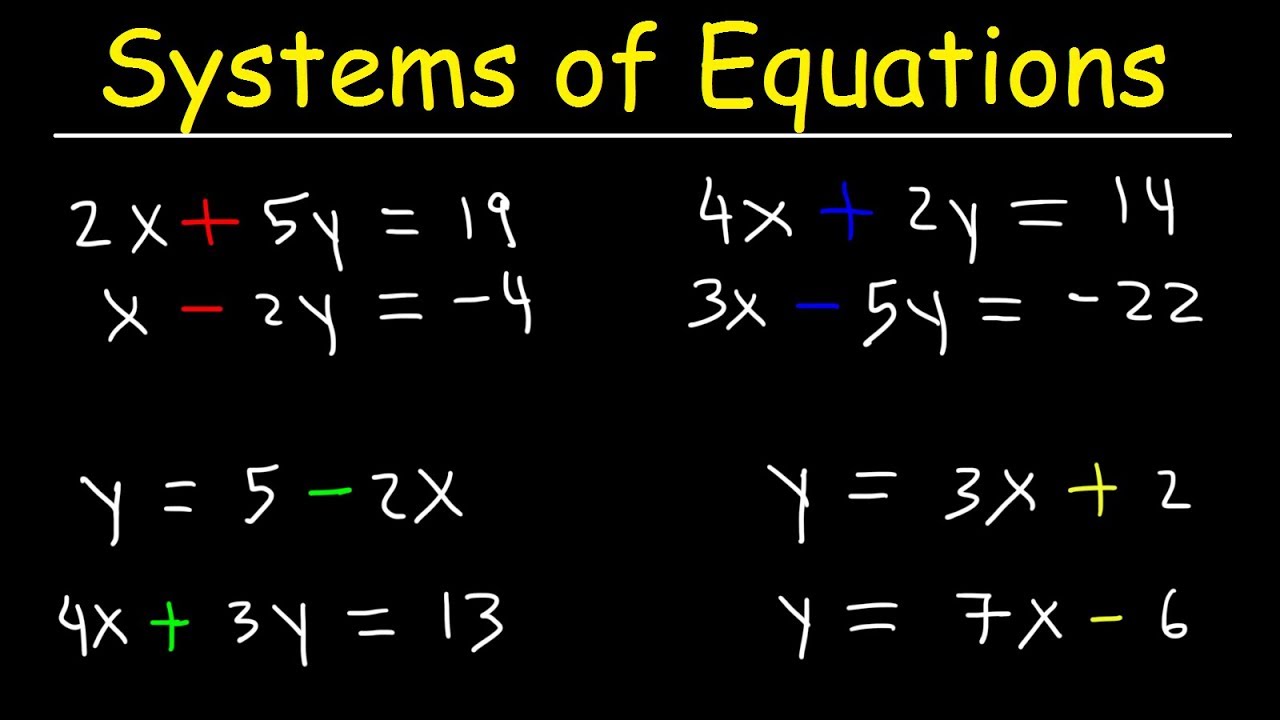
Solving Systems of Equations By Elimination & Substitution With 2 Variables
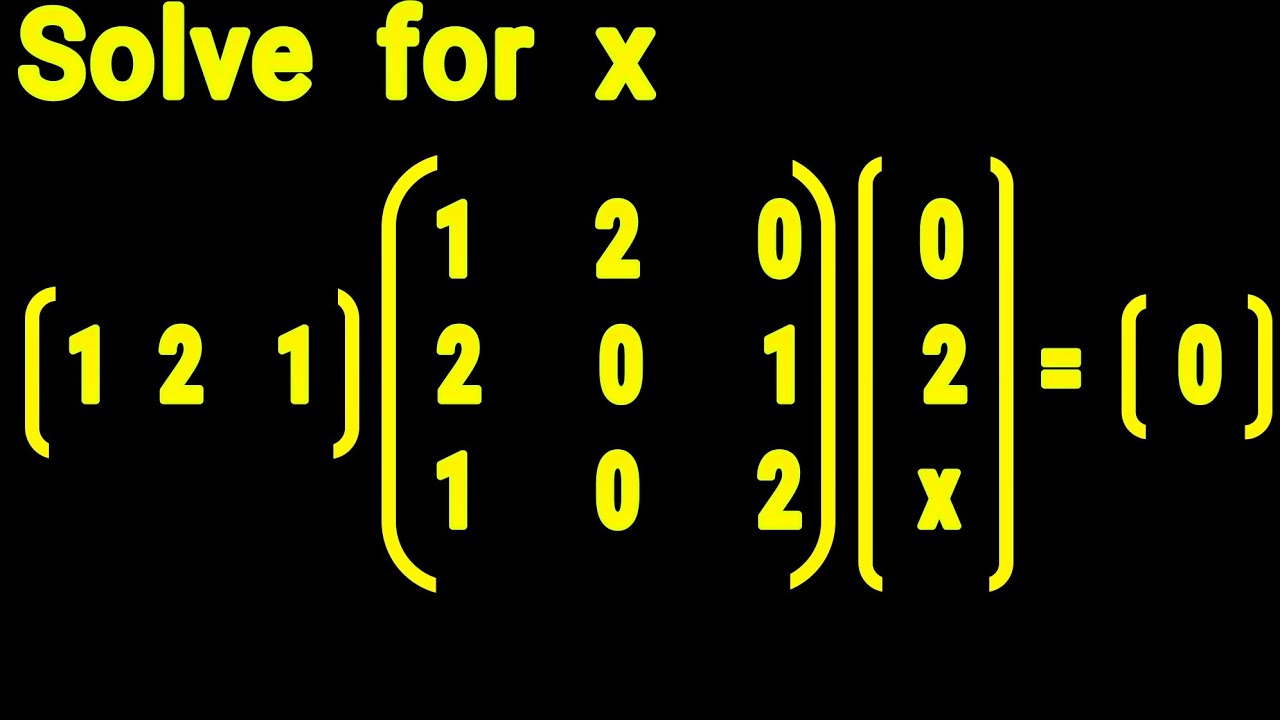
Solve for x | Matrix Multiplication
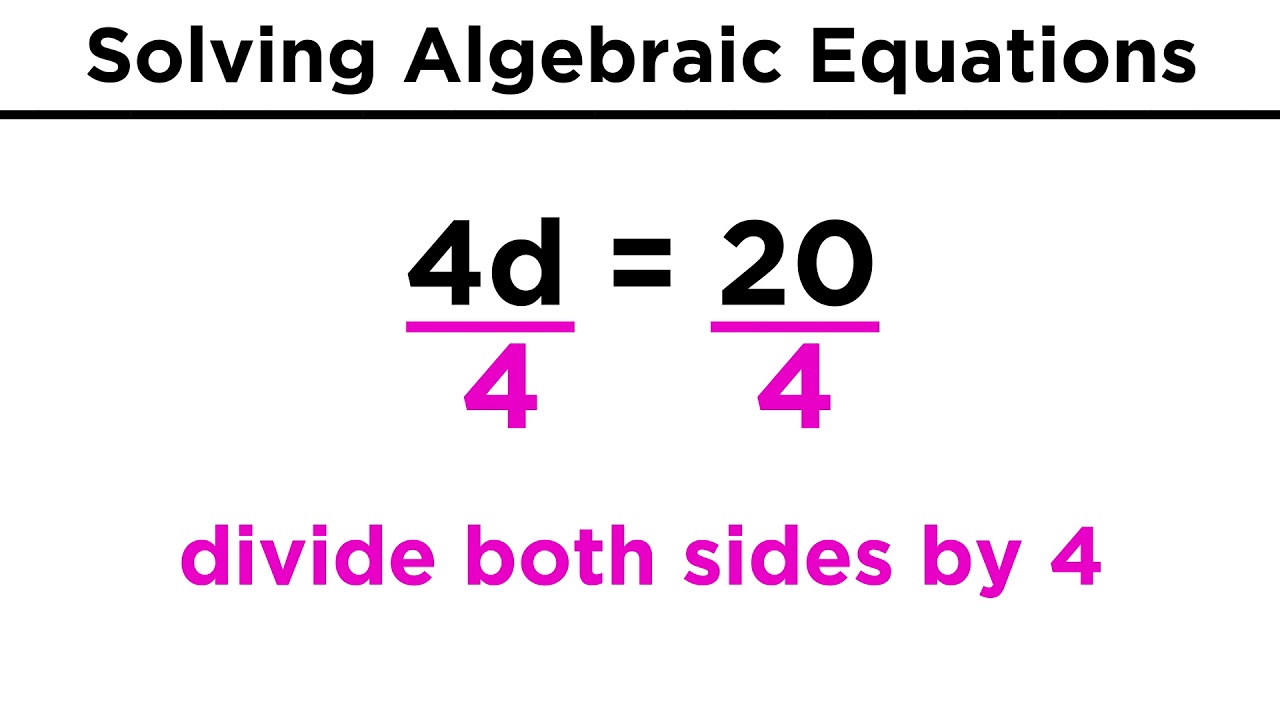
Algebraic Equations and Their Solutions
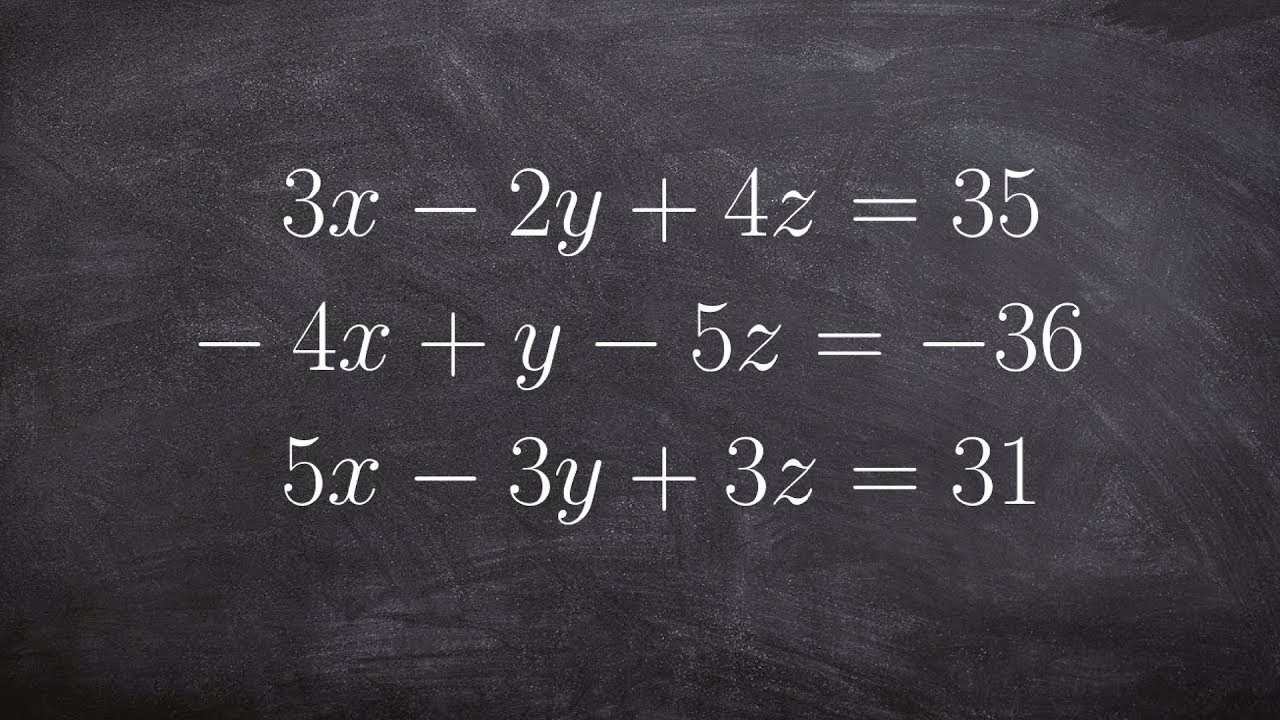
Solve a system with three variables
Solving Polynomial and Rational Equations
Solving Logarithmic Equations with Exponentials (Precalculus - College Algebra 63)
5.0 / 5 (0 votes)
Thanks for rating: