"Mutually Exclusive" and "Independent" Events (...are VERY different things!)
TLDRIn this informative video, Justin from zedstatistics.com clarifies the common misconception between mutually exclusive and independent events. Using the example of high school students' sports preferences, he explains that mutually exclusive events, like playing basketball and cricket in the summer, cannot occur simultaneously, hence their intersection probability is zero. In contrast, independent events, such as playing basketball and studying modern history, do not influence each other's probability. Justin further illustrates this with another example involving basketball and height, showing that dependent events have a different probability when conditions are applied. He emphasizes that mutually exclusive events are a form of dependence, not independence, and concludes by encouraging viewers to visit zedstatistics.com for more content and to consider donating to an education charity via the Super Thanks button on YouTube.
Takeaways
- 🏀 **Mutually Exclusive Events**: Two events are mutually exclusive if they cannot occur at the same time. In the example, a student cannot play basketball and cricket simultaneously during the summer season.
- 🤔 **Defining Mutually Exclusive**: The probability of both events A and B happening at the same time is zero, which is the defining characteristic of mutually exclusive events.
- 📊 **Calculating Probabilities**: For mutually exclusive events, the probability of each event can be found by dividing the number of students participating in the event by the total number of students.
- 🎓 **Independence in Events**: Two events are independent if the occurrence of one does not affect the probability of the other. In the script, playing basketball and studying modern history are independent events.
- ⚽ **Intersection in Independent Events**: There must be an intersection (students who play basketball and study modern history) for two events to be considered independent.
- 📉 **Assessing Independence**: To assess if events are independent, you must look at the numbers and see if the probability of one event remains the same regardless of the condition of the other event.
- 🚫 **Dependence in Events**: If the probability of one event changes when conditioned on the other, the events are dependent. For instance, the probability of playing basketball changes when considering only students taller than 175 cm.
- 🔄 **Conditional Probability**: The conditional probability of an event A given that event B has occurred can indicate dependence. If the conditional probability is different from the non-conditional probability, the events are dependent.
- 🤓 **Confusion Between Concepts**: People often confuse mutually exclusive events with independent events, but they are distinct concepts. Mutually exclusive events are a form of complete dependence, not independence.
- 💰 **Charitable Initiative**: The speaker, Justin, mentions that funds raised through the Super Thanks button on YouTube are donated to an education charity, which he does not keep for himself.
- 📚 **Resource for More Information**: For more content like this, viewers are directed to zedstatistics.com, where Justin uploads his videos on statistical concepts.
Q & A
What is the main difference between mutually exclusive and independent events?
-Mutually exclusive events cannot occur at the same time, meaning the probability of both events happening simultaneously is zero. Independent events, on the other hand, can occur simultaneously, and the occurrence of one event does not affect the probability of the other.
How many students out of 100 were surveyed in the example given in the video?
-A total of 100 high school students were surveyed.
In the example, what is the probability of a student playing basketball?
-The probability of a student playing basketball is 40 out of 100, or 0.4.
What is the definition of mutually exclusive events?
-Mutually exclusive events are events that cannot happen at the same time. The intersection of these events is zero, meaning there is no possibility of both events occurring together.
What is the probability of a student playing cricket in the example provided?
-The probability of a student playing cricket is 25 out of 100, or 0.25.
What is the key to determining whether two events are independent?
-The key to determining independence is to look at the numbers and calculate the probabilities. Two events are independent if the probability of one event is the same regardless of whether the other event occurs.
How many students in the example both play basketball and study modern history?
-Eight students out of the 100 both play basketball and study modern history.
What is the probability of a student playing basketball given that they study modern history?
-The probability of a student playing basketball given that they study modern history is 8 out of 20, or 0.4.
What is the condition for two events to be considered independent?
-Two events are considered independent if the probability of one event (event A) is equal to the probability of that event given that the other event (event B) has occurred.
In the video, what is the example given to illustrate dependent events?
-The example given for dependent events is a student playing basketball and being taller than 175 centimeters. The probability of playing basketball given the condition of being taller than 175 centimeters is 0.7, which is different from the overall probability of playing basketball, indicating dependence.
Why are mutually exclusive events considered the most extreme form of dependence?
-Mutually exclusive events are the most extreme form of dependence because the occurrence of one event completely excludes the occurrence of the other event. There is no intersection between the two events, which means they cannot happen at the same time.
What does Justin do with the funds raised through the Super Thanks button on YouTube?
-Justin donates all the funds raised through the Super Thanks button on YouTube to an education charity of his choice.
Outlines
🏀 Understanding Mutually Exclusive Events
In this paragraph, Justin introduces the concept of mutually exclusive events using the example of high school students choosing between playing basketball or cricket. Since both sports are played in the summer, students cannot participate in both simultaneously, making them mutually exclusive. The probability of each event is calculated, with 40 out of 100 students playing basketball and 25 out of 100 playing cricket. The key takeaway is that the intersection of these two events is zero, meaning no student can be part of both events at the same time, which is the defining characteristic of mutually exclusive events.
📚 Examining Independence in Events
The second paragraph delves into the concept of independent events, contrasting it with the previously discussed mutually exclusive events. Justin uses the same group of students but changes the second event to studying modern history, which is assumed not to affect the likelihood of playing basketball. The intersection of playing basketball and studying modern history is found to be eight students, which is crucial for assessing independence. The paragraph explains that independence is determined by whether the probability of one event remains the same regardless of the occurrence of the other event. In this case, the probability of playing basketball (40 out of 100) is not affected by the condition of studying modern history, confirming their independence. The paragraph concludes with an example of dependent events, where a student's height (taller than 175 centimeters) affects the likelihood of playing basketball, illustrating the difference between independence and dependence.
Mindmap
Keywords
💡Mutually Exclusive Events
💡Independent Events
💡Probability
💡Intersection
💡Conditional Probability
💡Dependence
💡Summer Sports
💡High School Students
💡ZedStatistics.com
💡Education Charity
💡Statistical Analysis
Highlights
Mutually exclusive events cannot occur at the same time, while independent events can.
Mutually exclusive events are the most extreme form of dependence, not independence.
Example: 40 out of 100 high school students play basketball, 25 play cricket, and 35 play neither - mutually exclusive.
Probability of mutually exclusive events occurring together is zero.
For independent events, the probability of one event does not change given the occurrence of the other.
Example: 8 out of 100 students play basketball and study modern history - independent events.
To assess independence, you need to look at the numbers and see if the intersection exists.
Applying a condition (e.g. studying modern history) should not affect the probability of the other event (playing basketball).
Dependent events have an overlap and the probability changes when a condition is applied.
Example: Students taller than 175 cm are more likely to play basketball - dependent events.
Conditional probability is different from non-conditional probability for dependent events.
Mutually exclusive events have zero conditional probability, indicating maximum dependence.
To determine independence, check if the probability of one event remains the same given the other event.
Intersection is necessary for events to be possibly independent.
If there is no intersection, the events are mutually exclusive and dependent.
The video provides clear examples and explanations to differentiate between mutually exclusive and independent events.
The speaker, Justin, hosts the video and provides additional information on his website zedstatistics.com.
Funds raised through the Super Thanks button on YouTube are donated to an education charity.
Transcripts
Browse More Related Video
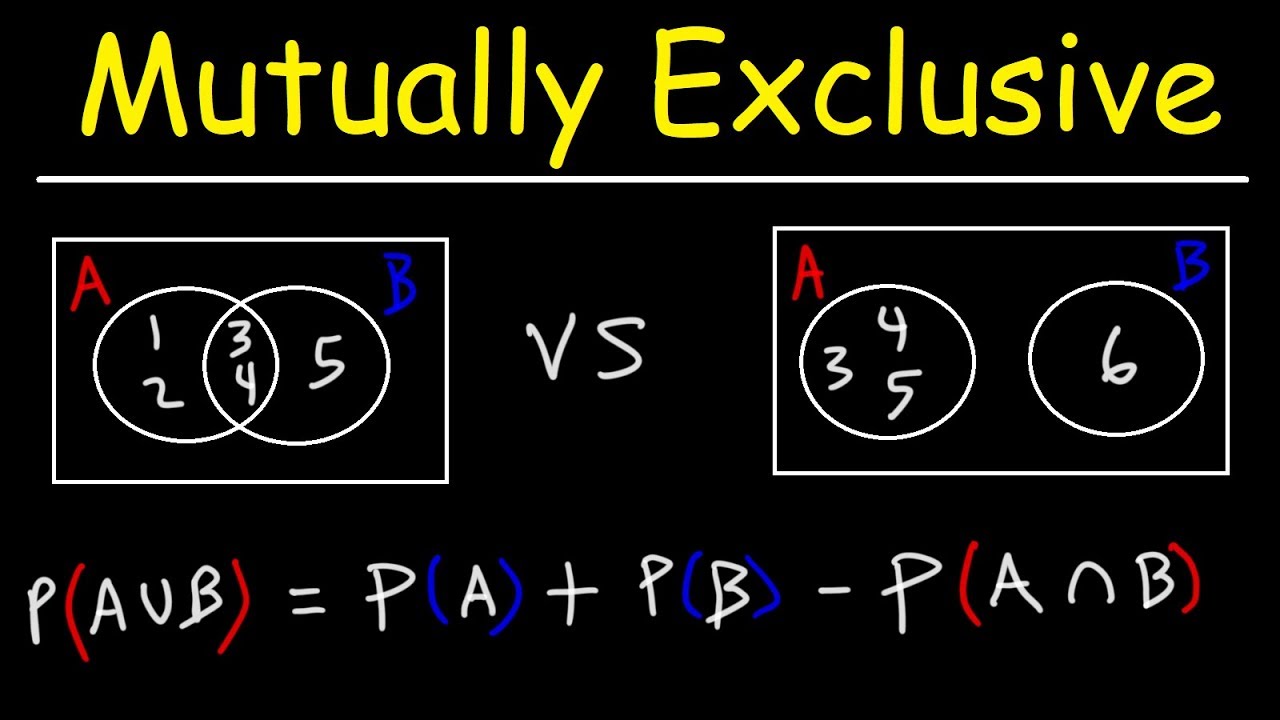
Probability of Mutually Exclusive Events With Venn Diagrams
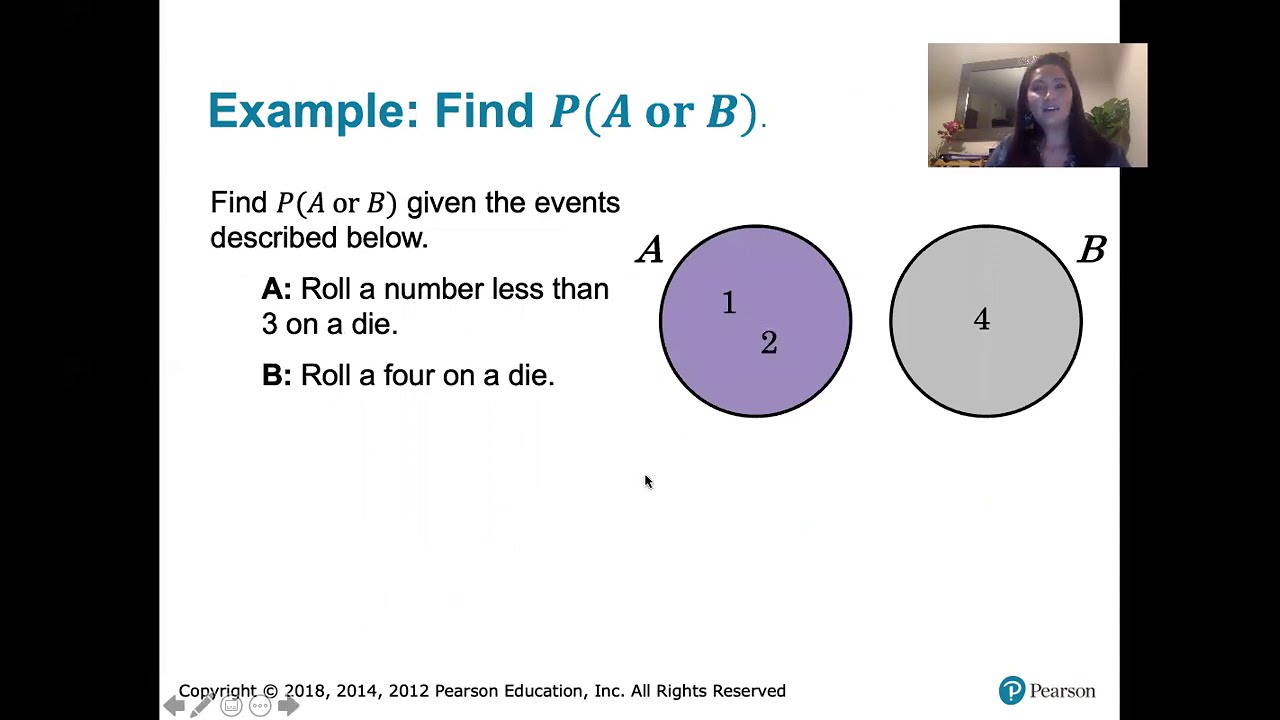
4.2.3 Addition and Multiplication Rules - Disjoint Events and Implications for Probability

AP Stats Test Quick Review: Probability

Chapter 4 Probability Part 2

Elementary Stats Lesson #8
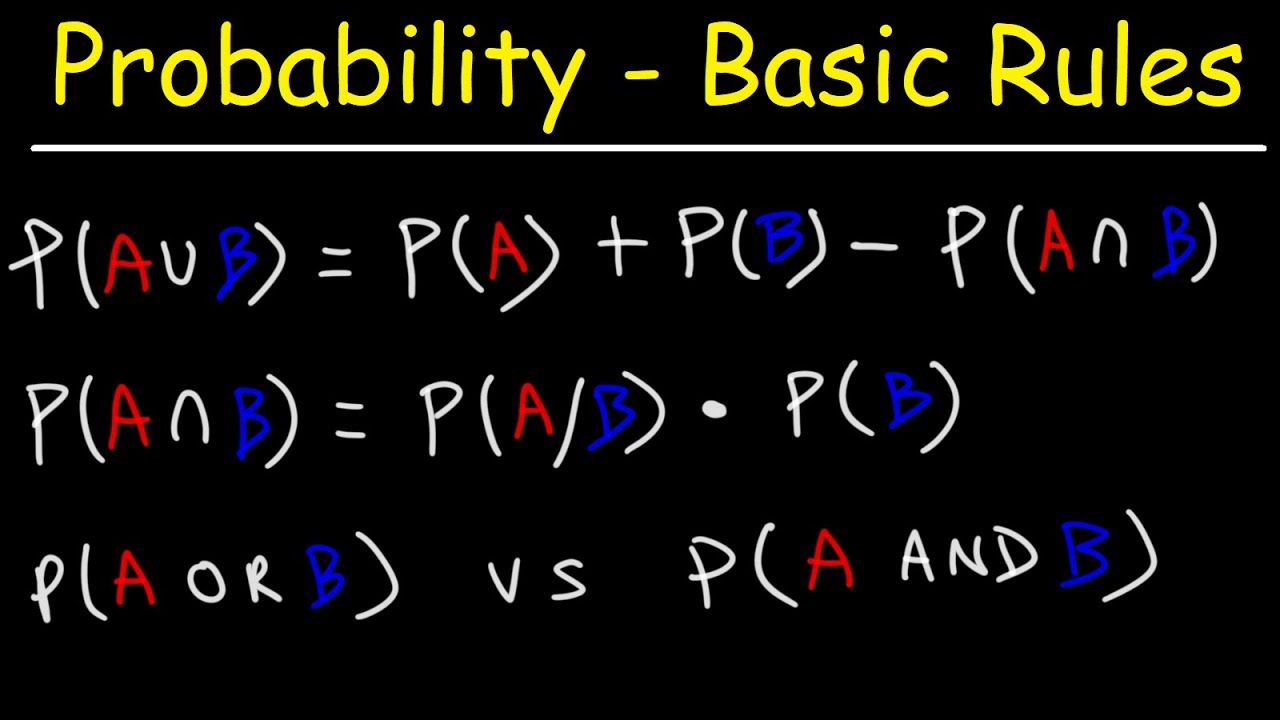
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events
5.0 / 5 (0 votes)
Thanks for rating: