Scalars, Vectors, and Vector Operations
TLDRProfessor Dave introduces the concepts of scalars and vectors, essential tools in understanding physics. Scalars are quantities with magnitude, like mass or temperature, while vectors include both magnitude and direction, crucial for analyzing forces. The video covers basic vector operations, such as addition, subtraction, and breaking down vectors into components, using examples like boat motion in a river. Trigonometric functions are introduced for finding angles and components, with a focus on their application in physics problems.
Takeaways
- 📚 Physics requires understanding of language and conventions, including units of measurement, scientific notation, and dimensional analysis.
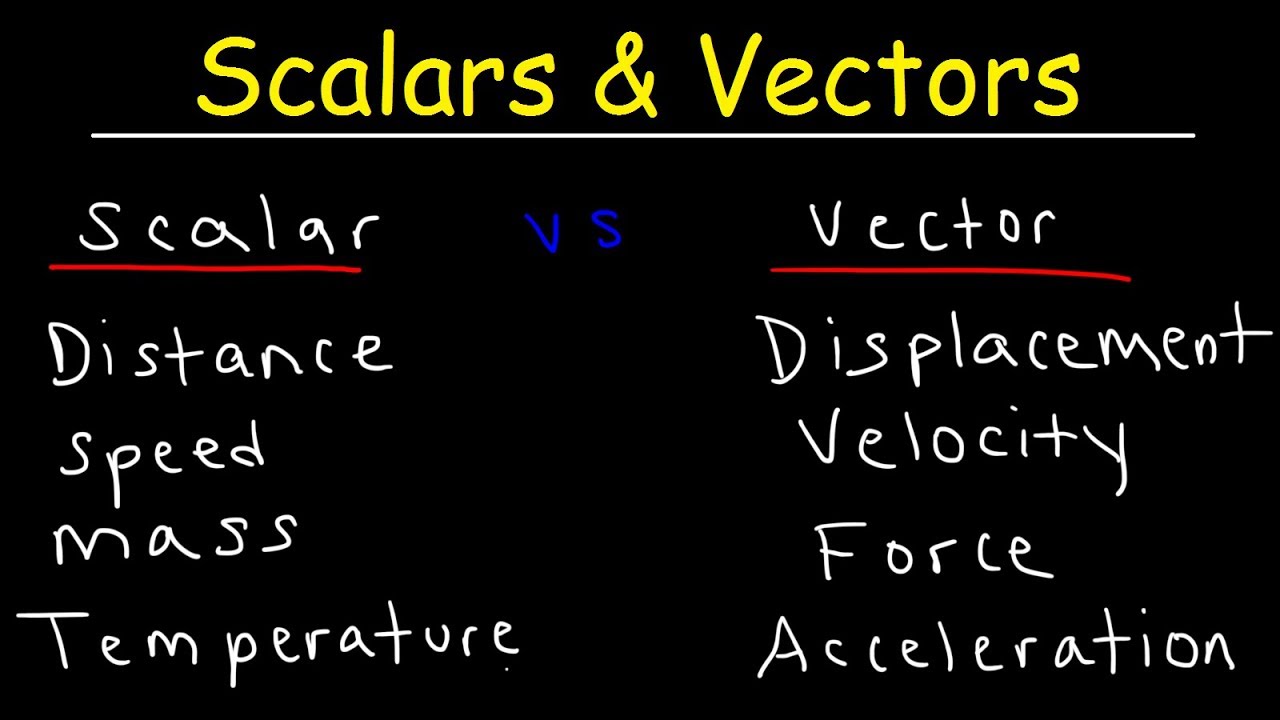
- 📈 Scalars are quantities with only magnitude, such as mass, time, or temperature, and are represented by numerical values with units.
- 🛤️ Vectors have both magnitude and direction, like forces, and are crucial in physics for representing quantities with directional significance.
- 🡆 Vectors are denoted using bold letters with an arrow, indicating their direction and magnitude.
- 🔜 Vector addition involves lining up vectors and creating a resultant vector that goes from the start of the first to the end of the second, retaining direction.
- 📐 For vectors in the same direction, their sum is a vector with the combined magnitude.
- 🔻 Perpendicular vectors' resultant can be found using the Pythagorean theorem, not by simply adding magnitudes.
- 📐 Trigonometric functions like sine, cosine, and tangent relate the angles and sides of a right triangle and are essential for physics calculations.
- 🤔 The mnemonic SOHCAHTOA helps remember the trigonometric relationships: sine theta = opposite/hypotenuse, cosine theta = adjacent/hypotenuse, and tangent theta = opposite/adjacent.
- 🠔 Vector subtraction is similar to addition but involves inverting the direction of the second vector, equivalent to multiplying by -1.
- 🔄 Breaking a vector into X and Y components is useful for analyzing motion in two dimensions, like projectile motion.
Q & A
What are the fundamental concepts in physics that need to be understood before diving into more complex topics?
-The fundamental concepts include units of measurement, scientific notation, dimensional analysis, and the understanding of scalars and vectors.
What is a scalar quantity and provide an example?
-A scalar quantity is a quantity that communicates a particular size or magnitude without direction. For example, the mass of a book, the amount of time spent watching a video, or the temperature outside.
How is a vector different from a scalar?
-A vector is different from a scalar because, in addition to having magnitude, it also has direction. This means a vector answers both 'How much?' and 'Which way?'.
How are vectors typically represented?
-Vectors are typically represented using letters in bold font with an arrow on top, indicating their direction.
What is the process of adding two vectors together?
-To add two vectors, you align them head to tail, and then draw a new resultant vector that goes from the start of the first to the end of the second vector, retaining their original directions.
How is the magnitude of the resultant vector calculated when two vectors point in the same direction?
-When two vectors point in the same direction, the magnitude of their sum is simply the sum of the magnitudes of the original vectors.
What is the Pythagorean theorem and how is it used in vector addition?
-The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (A squared) plus the square of one leg (B squared) equals the square of the other leg (C squared). It is used to find the magnitude of the resultant vector when two vectors form a right triangle.
Explain the use of trigonometric functions in determining the components of a vector.
-Trigonometric functions like sine, cosine, and tangent are used to find the X and Y components of a vector when the vector's magnitude and its angle from the horizontal are known. These functions relate the angles of a right triangle to the lengths of its sides.
What is vector subtraction and how does it differ from vector addition?
-Vector subtraction is similar to vector addition, but instead of adding the second vector normally, its direction is inverted (multiplied by -1), and then the two vectors are added head to tail. The resultant vector is found in the same manner as with vector addition.
How can a vector be broken into X and Y components?
-A vector can be broken into X and Y components by knowing the vector's magnitude and its angle from the horizontal. Using trigonometry, the sine and cosine of the angle can be applied to the magnitude to find the lengths of the X and Y components.
What is the example given in the script to illustrate vector addition and how it relates to real-world physics?
-The example given is a boat traveling across a river with a current. The boat's velocity in still water and the current's velocity are represented as vectors. By adding these vectors, we can determine the boat's resultant speed and direction, illustrating how vector addition applies to understanding motion in physics.
Outlines
📚 Introduction to Scalars and Vectors
Professor Dave introduces the concepts of scalars and vectors, emphasizing their importance in physics. Scalars are quantities with magnitude, such as mass or temperature, while vectors include both magnitude and direction, like force. The video explains how vectors are represented and the basic operations involving them, such as addition and the importance of direction in vector operations. It also introduces the concept of using trigonometric functions to resolve vectors into components and solve for angles in the context of right triangles.
📐 Trigonometric Functions and Vector Operations
This paragraph delves deeper into the use of trigonometric functions in relation to right triangles and vectors. It explains how to find unknown angles and sides using sine, cosine, and tangent, with a mnemonic device (SOHCAHTOA) to remember the relationships. The video also covers vector subtraction, the breakdown of vectors into X and Y components, and scalar multiplication. An example is provided to illustrate how to calculate the resultant vector for a boat moving in a river with a current, highlighting the practical application of vector addition and trigonometry in physics problems.
🚀 Conclusion and Call to Action
Professor Dave concludes the lesson on scalars and vectors by encouraging viewers to apply the concepts learned to understand motion in various situations. He emphasizes the abstract nature of the approach but assures its utility in studying physics. The video ends with a call to action, inviting viewers to subscribe to the channel for more tutorials, support the content creation on Patreon, and reach out via email for further questions or clarifications.
Mindmap
Keywords
💡Scalars
💡Vectors
💡Units of Measurement
💡Scientific Notation
💡Dimensional Analysis
💡Vector Addition
💡Pythagorean Theorem
💡Trigonometric Functions
💡Vector Subtraction
💡Vector Components
💡Scalar Multiplication
Highlights
Professor Dave introduces the concepts of scalars and vectors in physics.
Scalars are quantities with only magnitude, like mass and temperature.
Vectors have both magnitude and direction, essential for describing forces in physics.
Vector addition involves lining up vectors and summing their magnitudes and directions.
When vectors are in the same direction, their sum is a longer vector in the same direction.
Perpendicular vectors' sum is found using the Pythagorean theorem.
Trigonometric functions are used to find the components of vectors in right triangles.
The mnemonic SOHCAHTOA is introduced for remembering trigonometric relationships.
Vector subtraction is similar to addition but involves reversing the direction of one vector.
A vector can be broken into X and Y components, useful for analyzing projectile motion.
Vectors can be multiplied by scalars, changing their magnitude while keeping direction.
An example is given of a boat moving in a river with a current, illustrating vector addition.
The boat's speed and direction are calculated using vector addition and trigonometry.
Understanding vectors is crucial for analyzing motion in various physical situations.
The video encourages viewers to subscribe for more tutorials and support the content creation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: