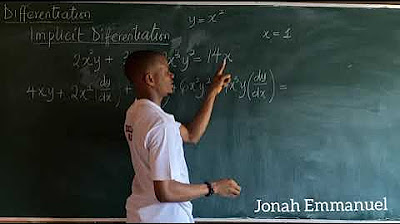
Implicit Differentiation for Calculus - More Examples #2
TLDRThe video script provides a detailed walkthrough of using implicit differentiation to find the derivative of a given function. The function in question is \( \frac{1}{x} + \ln y + x^3y = 4x \). The presenter begins by simplifying \( \frac{1}{x} \) to \( x^{-1} \) and proceeds to apply the rules of differentiation, including the chain rule and product rule, to each term. Emphasis is placed on correctly handling the derivative of terms involving \( y \), which requires multiplying by \( \frac{dy}{dx} \). The process involves moving all terms without \( \frac{dy}{dx} \) to one side and factoring out \( \frac{dy}{dx} \) to solve for it. The presenter demonstrates the steps to isolate \( \frac{dy}{dx} \) and presents a final expression for the derivative. The summary underscores the importance of careful application of differentiation rules and the systematic approach to solving such problems, making it an excellent educational resource for understanding implicit differentiation.
Takeaways
- 📚 Start by recognizing that the given equation involves implicit differentiation.
- ✍️ Rewrite the equation with \( x^{-1} \) as \( x^1 \) to simplify the differentiation process.
- 🔍 Differentiate each term of the equation with respect to \( x \), applying the chain rule and product rule where necessary.
- 📌 Remember to include the \( \frac{dy}{dx} \) term when differentiating parts of the equation that involve \( y \).
- 🔢 After differentiating, collect terms without \( \frac{dy}{dx} \) on one side of the equation and those with \( \frac{dy}{dx} \) on the other.
- 🔄 Factor out \( \frac{dy}{dx} \) from the terms on the left side to isolate it.
- 🧩 Simplify the equation by distributing and combining like terms.
- ➗ Divide both sides of the equation by the term that does not contain \( \frac{dy}{dx} \) to solve for \( \frac{dy}{dx} \).
- 📉 Move terms without \( \frac{dy}{dx} \) to the right side of the equation to prepare for solving.
- 📈 Recognize that the process involves moving all terms with \( \frac{dy}{dx} \) to one side and factoring out \( \frac{dy}{dx} \) to find its value.
- 📝 The final solution is an expression for \( \frac{dy}{dx} \) in terms of \( x \) and \( y \), which can be further simplified if desired.
Q & A
What is the given equation in the transcript that we are differentiating?
-The given equation is 1/x + ln(y) + x*y^3 = 4x.
How does the speaker rewrite the term 1/x in the equation?
-The speaker rewrites the term 1/x as x to the power of -1, but chooses not to rewrite the whole equation for simplicity.
What is the derivative of 1/x with respect to x?
-The derivative of 1/x with respect to x is -1x^(-2) or -1/x^2.
What is the derivative of ln(y) with respect to x, and why is it necessary to include dy/dx?
-The derivative of ln(y) with respect to x is 1/y * dy/dx. It is necessary to include dy/dx because we are differentiating with respect to x, not y, and y is considered a function of x.
How does the speaker apply the chain rule and product rule when differentiating x*y^3?
-The speaker applies the chain rule by taking the derivative of y^3 and multiplying it by the derivative of x, which gives 3*x*y^2. Then, the product rule is applied to x and y^3, resulting in 3*x*y^2 + 3*x*y^2 * dy/dx.
What is the final expression for dy/dx after moving all terms without dy/dx to the right side?
-The final expression for dy/dx is (1/y) * dy/dx + 3*x*y^2 * dy/dx = 4 - 1/x^2.
How does the speaker factor out dy/dx from the left side of the equation?
-The speaker factors out dy/dx by recognizing that every term on the left side of the equation now contains dy/dx, and then divides the entire equation by the term without dy/dx on the right side.
What is the simplified form of the equation after factoring out dy/dx?
-The simplified form of the equation after factoring out dy/dx is dy/dx * (x^3 + y^3) = 4x^2 - 3x^2*y^3.
What is the final step to solve for dy/dx?
-The final step to solve for dy/dx is to divide both sides of the equation by the term that does not contain dy/dx, which is (x^3 + y^3).
What is the final expression for dy/dx given in the transcript?
-The final expression for dy/dx is (4x^2 - 3x^2*y^3) / (x^3 + y^3).
What is the importance of distributing terms during the differentiation process?
-Distributing terms is important to ensure that each part of the expression is correctly differentiated and to simplify the equation, making it easier to solve for dy/dx.
What is the general approach to solving implicit differentiation problems as described in the transcript?
-The general approach includes taking the derivative of the given equation, moving terms involving dy/dx to one side and other terms to the other, factoring out dy/dx, and then dividing by the remaining terms to isolate dy/dx.
Outlines
🧮 Implicit Differentiation Example
This paragraph explains the process of using implicit differentiation to find the derivative of a given function. The function in question is (1/x) + ln(y) + x*y^3 = 4x. The explanation begins by simplifying (1/x) to x^-1 and then differentiating each term, applying the chain rule and product rule where necessary. The derivative of (1/x) is -x^-2, and for ln(y) it's (1/y) multiplied by dy/dx. The term x*y^3 requires both the product rule and the chain rule, resulting in 3x*y^2 plus 3x*y^2 * dy/dx. The goal is to solve for dy/dx, which is done by moving terms without dy/dx to the right side and factoring out dy/dx from the left side. The final step is to isolate dy/dx by dividing by the remaining terms, resulting in dy/dx = (4 + x^2 - 3x^2*y^3)/(y + 3x^2*y^2). The paragraph emphasizes the importance of careful application of differentiation rules and the steps to isolate dy/dx.
📝 Tips for Solving Implicit Differentiation Problems
The second paragraph offers advice on solving problems involving implicit differentiation. It emphasizes that when differentiating terms involving y, the term dy/dx must be included. The paragraph also suggests strategies for simplifying the equation by removing brackets and parentheses, grouping terms with dy/dx on one side, and then factoring out dy/dx to solve for it. The final step is to divide by the remaining terms to isolate dy/dx. The paragraph concludes by stating that this is a typical process for such problems and encourages checking the solution against the answer provided.
Mindmap
Keywords
💡Implicit Differentiation
💡Derivative
💡Natural Logarithm (Ln)
💡Chain Rule
💡Product Rule
💡
💡Dy/Dx
💡Rewriting Equations
💡Distributing
💡Simplifying Expressions
💡Factoring
💡Dividing by Terms
Highlights
Introduction to using implicit differentiation to find the derivative of a given equation involving x and y
Rewriting 1/x as x^(-1) to simplify the differentiation process
Derivative of x^(-1) results in -1x^(-2), illustrating the power rule
Differentiating ln(y) yields 1/y, introducing the concept of treating y as a function of x
Applying the chain rule and product rule to differentiate XY^3, emphasizing the importance of understanding the order of operations
The constant term 3 is factored out during the differentiation of XY^3
Careful application of the product rule for the inner function of XY^3
The goal of solving for dy/dx is established, providing a clear objective for the differentiation process
Elimination of brackets to facilitate the addition and subtraction of terms involving dy/dx
Distributing terms to combine like terms involving dy/dx for easier manipulation
Isolating terms without dy/dx on one side of the equation
Subtracting terms to isolate variables and constants on the opposite side of the equation
Factoring out dy/dx from all terms on the left side of the equation
Simplifying the equation by squaring terms and factoring out common variables
Dividing by the remaining terms to solve for dy/dx, the final step in finding the derivative
Resulting expression for dy/dx is obtained, showcasing the final solution
Emphasis on the typical process of differentiating equations involving y as a function of x
Advice on cleaning up the final expression for clarity and ease of understanding
Summary of the key steps in implicit differentiation: taking derivatives, combining like terms, factoring, and solving for dy/dx
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: