05 - Sine and Cosine - Definition & Meaning - Part 1 - What is Sin(x) & Cos(x) ?
TLDRThis script offers an in-depth exploration of the sine and cosine functions, fundamental to trigonometry. It emphasizes the practical application of these functions in breaking down forces or movements into horizontal (x-axis) and vertical (y-axis) components. The lesson uses the concept of a force being applied at an angle to a box to illustrate how sine and cosine work. The sine function is defined as the ratio of the opposite side to the hypotenuse of a right triangle, representing the vertical component of the force when projected onto the y-axis. Conversely, the cosine function is the ratio of the adjacent side to the hypotenuse, representing the horizontal component projected onto the x-axis. The script creatively employs the term 'chopping function' to describe how sine and cosine scale the hypotenuse to find the lengths of the sides of the triangle, which correspond to the components of the force in their respective directions. This approach simplifies the understanding of how these trigonometric functions are used to analyze and calculate motion and force in various real-world scenarios, such as physics problems involving vectors.
Takeaways
- 📐 The sine and cosine functions are fundamental to trigonometry and are used to break down forces or movements into horizontal (x-axis) and vertical (y-axis) components.
- 🔍 Sine is defined as the ratio of the opposite side to the hypotenuse of a right triangle, and it represents the vertical component of a force when applied at an angle.
- 📏 Cosine is defined as the ratio of the adjacent side to the hypotenuse of a right triangle, and it represents the horizontal component of a force when applied at an angle.
- 🤔 Understanding the practical meaning of sine and cosine is crucial before moving on to more advanced topics, as they form the basis for solving problems involving vectors and angles.
- 🧮 The values of sine and cosine for an angle will always be between -1 and 1, representing a ratio of the sides of a right triangle.
- 📈 The sine of an angle can be thought of as a 'chopping function' for the y-axis, while the cosine is the 'chopping function' for the x-axis, assuming the length of the hypotenuse is 1.
- 🌟 The sine and cosine functions are essential for analyzing motion or forces in two dimensions, such as when throwing a ball or calculating the trajectory of a space probe.
- 🔄 To find the horizontal and vertical components of a force, multiply the sine or cosine of the angle by the length of the hypotenuse.
- 📉 For small angles, the cosine value will be close to 1 (indicating most of the force is horizontal), and the sine value will be close to 0 (indicating little force is vertical).
- 🔗 The Pythagorean theorem (a² + b² = c²) must always hold true when calculating the sides of a right triangle using sine and cosine.
- 🔃 The process of calculating the components of a force or analyzing motion in different directions is a key application of sine and cosine in physics and engineering.
Q & A
What is the main purpose of studying sine and cosine functions?
-The main purpose of studying sine and cosine functions is to understand and break down angled quantities, such as forces, velocities, or accelerations, into their horizontal and vertical components, which simplifies the analysis of problems involving vectors.
What is a 'chopping function' in the context of sine and cosine?
-A 'chopping function' is a term used in the script to describe how sine and cosine functions reduce the hypotenuse of a triangle to its corresponding side (opposite for sine and adjacent for cosine) by multiplying the hypotenuse with the sine or cosine value of the angle in question.
How does the sine function relate to the vertical component of a force acting at an angle?
-The sine function gives the ratio of the opposite side to the hypotenuse in a right triangle. When applied to a force acting at an angle, it represents the fraction of that force which is acting in the vertical direction.
How does the cosine function relate to the horizontal component of a force acting at an angle?
-The cosine function gives the ratio of the adjacent side to the hypotenuse in a right triangle. When applied to a force acting at an angle, it represents the fraction of that force which is acting in the horizontal direction.
What is the significance of the 3-4-5 triangle in understanding sine and cosine functions?
-The 3-4-5 triangle is a special right triangle where the sides are in whole numbers, which makes it easy to understand the concepts of sine and cosine. It helps in visualizing how the sine and cosine values for an angle (36.87 degrees in this case) can be derived and what they represent in terms of the force components.
Why is the Pythagorean theorem important when dealing with sine and cosine functions?
-The Pythagorean theorem (a^2 + b^2 = c^2) is important because it ensures that the calculated sides of a triangle using sine and cosine values actually form a right triangle, validating the use of these trigonometric functions in breaking down forces or vectors into their components.
What does it mean when the script says that sine and cosine are 'projections'?
-When the script refers to sine and cosine as 'projections', it means that they represent the lengths of the shadows or projections that would be cast in the vertical (sine) and horizontal (cosine) directions if a light were shone on the hypotenuse of the triangle.
How does the size of the triangle affect the sine and cosine values of its angles?
-The size of the triangle does not affect the sine and cosine values of its angles. These values are determined solely by the angle's measure and are independent of the triangle's side lengths.
Why are sine and cosine ratios always less than one?
-Sine and cosine ratios are always less than one because they are derived from the ratio of two sides of a right triangle, with the denominator being the hypotenuse, which is the longest side of the triangle.
What is the practical application of understanding sine and cosine functions in physics problems?
-In physics, understanding sine and cosine functions allows one to break down complex problems involving vectors, such as forces, velocities, and accelerations, into simpler, more manageable components along the horizontal and vertical axes.
How can one remember the association between sine, cosine, and their respective directions (horizontal and vertical)?
-A mnemonic used in the script is to remember that 'sine goes with y' and 'cosine goes with x', associating sine with the vertical direction and cosine with the horizontal direction.
Outlines
🔍 Introduction to Sine and Cosine Functions
This introductory lesson emphasizes the fundamental importance of understanding sine and cosine functions in trigonometry, extending from algebra through calculus. The presenter aims to teach these concepts in a practical way, different from typical textbook methods, by explaining their practical applications early on rather than after several chapters of theory. The lesson involves a scenario where a box is pushed at an angle to illustrate how forces can be decomposed into horizontal and vertical components using sine and cosine, thereby setting the stage for deeper exploration into these trigonometric functions.
📐 Application of Right Triangle Ratios
The lesson proceeds to explain the application of trigonometric functions specifically to right triangles, using a 3-4-5 triangle example to demonstrate the use of the Pythagorean theorem. It details how trigonometric functions such as sine and cosine can dissect a force vector into its horizontal and vertical components, illustrating with practical examples how these functions are essential for understanding forces and angles in physics. The significance of these trig functions in breaking down vectors into manageable components is highlighted as a fundamental concept in trigonometry and physics.
🧮 Defining Sine and Cosine
This section delves into the mathematical definitions of sine and cosine functions, explaining them as ratios of the sides of a right triangle relative to an angle. The sine function is described as the ratio of the opposite side to the hypotenuse, while the cosine function is the ratio of the adjacent side to the hypotenuse. These definitions provide a foundation for understanding how trigonometric functions project forces into vertical (sine) and horizontal (cosine) components, effectively simplifying complex physical scenarios into easier calculations.
🔢 Practical Meaning of Sine and Cosine
The lesson further clarifies the practical applications of sine and cosine, emphasizing their role in determining the proportion of a force in the vertical and horizontal directions respectively. It introduces the concept of these functions as 'chopping functions', which reduce a force into its directional components based on an angle. This part of the lesson aims to solidify the learner's understanding of how trigonometric functions are used to analyze and solve real-world problems involving angles and forces.
🛠️ Sine and Cosine in Force Decomposition
This segment demonstrates using sine and cosine to decompose a force vector into its y (vertical) and x (horizontal) components. The presenter calculates the components of different forces using the sine and cosine functions, explaining the concept of 'chopping' the force according to the triangle's hypotenuse and the angle's sine or cosine. The calculations help visualize how trigonometry simplifies the analysis of forces, facilitating easier problem-solving in physics and engineering.
📏 Extending the Concept to Larger Forces
The discussion extends to manipulating larger forces using the same angle but increasing the force magnitude. This part illustrates how the sine and cosine functions scale with changes in the force, maintaining consistent proportions for the components regardless of the total force. This consistency underlies many practical applications of trigonometry, such as in construction and mechanical engineering, where forces often vary in magnitude but not necessarily in direction.
🔎 Verifying Trigonometric Calculations
This final segment involves verifying the calculations of force components for a larger triangle to ensure they align with the principles of trigonometry, particularly the Pythagorean theorem. The presenter revisits the concept of sine and cosine as functions dependent solely on the angle, demonstrating their reliability and consistency across different scenarios and force magnitudes. This reinforces the idea that trigonometric functions provide a dependable method for dissecting and analyzing forces in various fields of science and engineering.
Mindmap
Keywords
💡Trigonometry
💡Sine Function
💡Cosine Function
💡Right Triangle
💡Hypotenuse
💡Force
💡Pythagorean Theorem
💡Projection
💡Angle
💡Vector
💡Chopping Function
Highlights
The lesson focuses on the foundational understanding of sine and cosine, essential for various levels of trigonometry.
Sine and cosine are introduced through a practical scenario involving force, providing a conceptual understanding rather than just a mathematical definition.
The concept of force is used to explain how sine and cosine functions work with angled forces, emphasizing their role in breaking down forces into horizontal and vertical components.
A hypothetical box is used as a prop to visualize the application of force at an angle, leading to the introduction of right triangles in relation to sine and cosine.
The lesson clarifies that sine and cosine only apply to right triangles, highlighting the importance of the 90-degree angle in trigonometric functions.
The Pythagorean theorem is used to demonstrate how the sides of a right triangle relate to the sine and cosine of an angle.
The sine function is defined as the ratio of the opposite side to the hypotenuse of a right triangle, providing a method to calculate the vertical component of a force.
Cosine is defined as the ratio of the adjacent side to the hypotenuse, used to determine the horizontal component of a force.
The lesson emphasizes that sine and cosine are ratios, inherently less than one, due to the hypotenuse being the longest side of the triangle.
The terms 'chopping function' and 'projection' are introduced to describe how sine and cosine work to break down forces in the y and x directions respectively.
The lesson uses the example of a 3-4-5 triangle to illustrate the calculation of sine and cosine for an angle of 36.87 degrees.
The concept of scaling the triangle while maintaining the same angle is explored to show how sine and cosine values remain constant for a given angle.
The self-consistency of sine and cosine is demonstrated by recalculating these values using the sides of the triangle formed by the initial sine and cosine calculations.
The lesson concludes with the practical application of sine and cosine in analyzing forces in different directions, setting the stage for more advanced topics.
The importance of understanding the foundational concepts of sine and cosine is stressed for their application in physics and other mathematical fields.
The lesson encourages students to internalize the concepts by watching and practicing the calculations multiple times for deeper understanding.
Transcripts
Browse More Related Video

Which are the Three Functions in Trigonometry? | Don't Memorise

Trig - 0.6 Trig Functions of Acute Angles
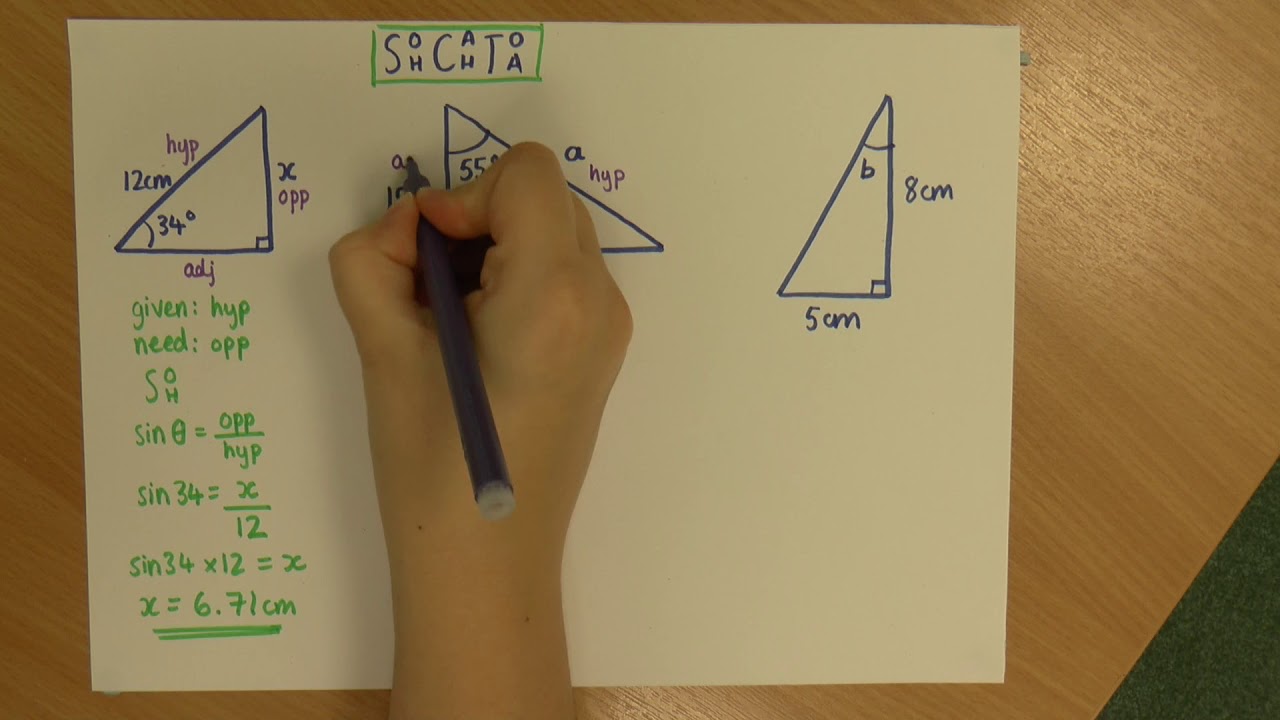
Trigonometry: Finding missing sides and angles
When Do I use Sin, Cos or Tan?
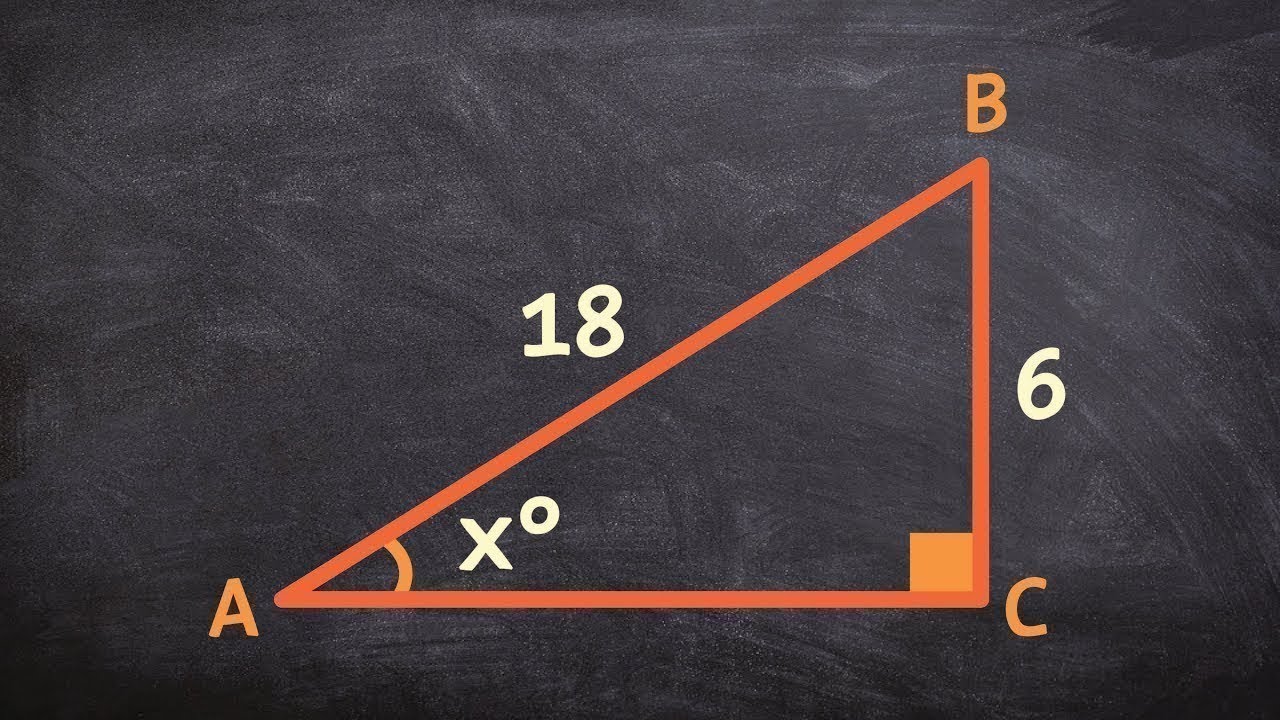
Learn to find the missing angles for a triangle using inverse trig functions

Vector components from magnitude and direction
5.0 / 5 (0 votes)
Thanks for rating: