Calculus 2 Lecture 10.5: Calculus of Polar Equations
TLDRIn this transcript, the speaker delves into the concept of polar equations, focusing on how to calculate the area bounded by polar curves. The explanation begins with a discussion on the definition of polar curves and their relationship with angles and radii. The speaker then introduces the formula for finding the area, emphasizing the importance of integrating over a range of angles. The concept is further illustrated with examples, including the calculation of areas for specific polar equations. The segment also touches on finding the area between two polar curves and the intersection points of different polar equations, providing a comprehensive understanding of the topic.
Takeaways
- 📌 Understanding polar coordinates and how polar curves are defined by an angle (theta) that dictates the radius (R) is fundamental for calculating areas and lengths in polar coordinates.
- 📈 To find the area bounded by a polar curve, one must integrate the area of infinitesimally small sectors (similar to Riemann sums in rectangular coordinates) over the given interval of angles.
- 🔍 The formula for the area of a sector in polar coordinates is (1/2) * R^2 * theta, which is used to calculate the area of the infinitesimally small sectors that make up the total area bounded by the polar curve.
- 🌀 When dealing with polar equations, it's crucial to recognize the symmetry of the curves, which can simplify the process of finding areas and lengths by allowing us to calculate only half of the shape and then double the result.
- 🧩 To find the area between two polar curves, the strategy is to subtract the area under the smaller curve from the area under the larger curve, using the same angular limits for integration.
- 🤔 When locating intersections between two polar curves, setting the equations equal to each other and solving for theta can yield the angular values where the curves intersect.
- 📊 The arc length of a polar curve is found by integrating a function that involves the square root of the derivative of R with respect to theta, similar to the arc length formula in Cartesian coordinates.
- 🌎 The surface area of a shape obtained by revolving a polar curve around an axis is calculated by integrating the product of the circumference of the revolving circle (2πR) and the length of the curve along the axis.
- 🤗 The process of finding the area bounded by polar curves can be illustrated with examples, such as the area between a circle (R=3) and a cardioid (R=2+2cosθ), which requires finding the intersection angles and integrating over that range.
- 🛠 When solving for intersections in polar coordinates, it's essential to consider the quadrants and the direction of angle rotation to ensure the correct solutions are found.
- 🌐 The intersection points of polar curves can be at the same location but at different angles, indicating that the order in which they pass through a point can vary, which is a unique aspect of polar coordinate geometry.
Q & A
How do you define a polar curve?
-A polar curve is defined by an equation that relates the radius (R) to the angle (theta). It is a type of curve plotted in polar coordinates, where each point on the curve is determined by a distance (R) from a central point (the origin) and an angle (theta) from a reference direction (usually the polar axis).
What is the significance of the area bounded by a polar curve?
-The area bounded by a polar curve is important in various mathematical and real-world applications, such as calculating the surface area of revolution objects, determining the size of regions in polar coordinate systems, and solving problems in physics and engineering that involve polar coordinates.
How do you find the area between two polar curves?
-To find the area between two polar curves, you integrate the difference of the squared functions of the two curves over the interval of interest. Specifically, if you have two polar curves R1(theta) and R2(theta), the area A is given by the integral from alpha to beta of (1/2 * (R1(theta)^2 - R2(theta)^2)) * dtheta.
What is the formula for the area of a sector of a polar curve?
-The area of a sector of a polar curve is given by the formula (1/2) * R^2 * theta, where R is the radius of the sector and theta is the central angle in radians.
How do you determine the intersection points of two polar curves?
-To determine the intersection points of two polar curves, you set the equations of the curves equal to each other and solve for the independent variable (theta). The solutions will give you the angles where the curves intersect. To find the corresponding radial distances (R values), you substitute these angles back into either of the original polar curve equations.
What is the significance of symmetry in polar coordinates?
-Symmetry in polar coordinates is significant because it can simplify calculations, such as finding the area bounded by a polar curve. If a curve is symmetrical about the polar axis or the theta=π/2 axis, you can calculate the area for half of the curve and then double the result to get the full area, taking advantage of the symmetry to reduce the computational effort.
How do you calculate the length of a polar curve?
-The length of a polar curve is calculated using the integral of the square root of the derivative of R with respect to theta, over the interval of interest. Specifically, the arc length L is given by the integral from alpha to beta of the square root of (R'(theta)^2 + R^2) * dtheta, where R'(theta) is the derivative of R with respect to theta.
What is the formula for the surface area of revolution about the polar axis?
-The surface area of revolution about the polar axis (x-axis in polar coordinates) is given by the integral of (2πR) * sqrt(R'(theta)^2 + R^2) * dtheta, where R is the function of theta, and R'(theta) is its derivative. This formula represents the sum of the areas of infinitesimally thin circular disks as the curve is revolved around the polar axis.
What is the difference between finding the area bounded by a polar curve and the surface area of revolution?
-The area bounded by a polar curve is the two-dimensional region enclosed by the curve and is calculated using integral calculus in polar coordinates. On the other hand, the surface area of revolution is the three-dimensional area that would be formed if the curve were revolved around an axis, such as the polar axis, and is also calculated using integral calculus but considering the circumference of the curve at each point and the length of the curve.
How do you use trigonometric identities to simplify polar equations?
-Trigonometric identities, such as the double angle formula, can be used to simplify polar equations by reducing the complexity of the expressions involved. For example, the double angle formula cos(2θ) = 2cos²(θ) - 1 can be used to convert terms involving cos(2θ) into a form that is easier to manipulate and integrate when working with polar curves.
Outlines
📚 Introduction to Polar Curves and Areas
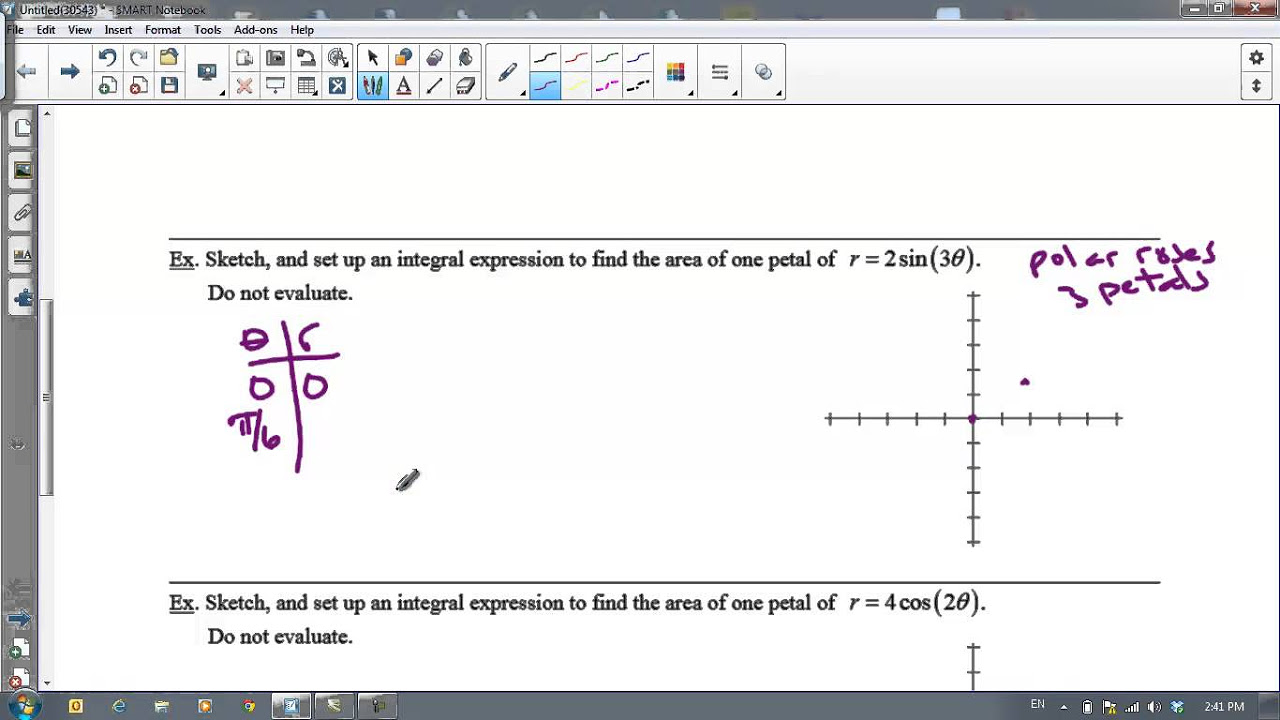
The paragraph introduces the concept of polar curves and how to find the area bounded by a polar curve. It explains that polar curves are defined by an angle and a function of that angle, and the area is found by integrating the area of infinitesimally small sectors defined by the curve and the angle change. The explanation includes an example of a polar curve and how to visualize the area it encloses.
📈 Calculating Areas using Integrals
This section delves into the mathematical process of calculating the area under a polar curve using integrals. It explains the concept of Riemann sums and how they apply to polar coordinates, using the formula for the area of a sector to illustrate the process. The paragraph also provides an example of finding the area bounded by a specific polar equation and discusses the symmetry properties of the curve to simplify the calculation.
🌀 Examples of Finding Polar Curve Areas
The paragraph presents examples of finding the area bounded by polar curves, using specific polar equations. It explains the process of setting up the integral, using substitution for integration, and evaluating the integral to find the area. The examples illustrate how to handle different types of polar equations and their corresponding areas, providing insights into the mathematical techniques involved.
🤔 Understanding Polar Curve Intersections
This section discusses the concept of finding the intersection points of two polar curves. It explains the algebraic process of setting the equations of two curves equal to each other and solving for the intersection angles. The paragraph emphasizes the importance of considering the quadrants and the direction of angle change in polar coordinates to correctly identify the intersection points.
📊 Area Between Two Polar Curves
The paragraph explains how to find the area between two polar curves. It introduces the idea of subtracting the area under the smaller curve from the area under the larger curve to find the area between them. The explanation includes the mathematical formula for this process and an example of how to set up and solve the integral for such a scenario.
🌟 Symmetry and Intersections in Polar Coordinates
This section explores the symmetry properties of polar curves and how they can be used to simplify the process of finding areas and intersections. It discusses the concept of coterminal angles and how they are used to determine the correct interval for integration. The paragraph also provides an example of finding the area between two curves using their symmetry and the intersection points to define the limits of integration.
🧮 Solving Polar Equations for Intersections
The paragraph focuses on the process of solving polar equations to find their intersections. It explains how to use trigonometric identities and algebraic manipulation to solve for the angles where two polar curves intersect. The section also highlights the importance of considering the signs of the trigonometric functions in different quadrants and how they affect the solution.
🔄 Intersections at the Origin
This section discusses the interesting case where polar curves intersect at the origin. It explains that even though two curves can pass through the origin at different times, they can still have intersections at the same point but at different angles. The paragraph provides an example of this phenomenon and emphasizes the unique aspects of polar coordinates that allow for such intersections.
Mindmap
Keywords
💡Polar Coordinates
💡Polar Curves
💡Area Calculation
💡Integral Calculus
💡Symmetry
💡Trigonometric Functions
💡Riemann Sums
💡Derivatives
💡Arc Length
💡Surface Area of Revolution
Highlights
Exploring the concept of finding the area bounded by polar curves through integration.
Defining polar curves by an angle that dictates the radius R as a function of theta.
Starting at an angle alpha and ending at angle beta to find the area enclosed by the polar curve.
Using the formula for the area of a sector to break down the polar curve into infinitesimally small sectors for integration.
Integrating with respect to theta to find the area, summing up an infinite number of small sectors from alpha to beta.
Understanding the polar curve as a comb-like shape and the area being calculated as a series of these shapes.
Applying calculus concepts to polar equations, specifically using integrals to find areas and lengths.
Demonstrating the process of finding the area bounded by a polar curve with a practical example of R squared equals four cosine two theta.
Using symmetry of the polar curve to simplify the calculation of the area by multiplying the area from 0 to PI/4 by 4.
Explaining the integral of trigonometric functions in the context of finding the area bounded by polar curves.
Discussing the concept of finding the area bounded by two polar curves by integrating the difference between the upper and lower curves.
Integrating the formula for the area between two polar curves, R1 squared minus R2 squared, to find the enclosed area.
Describing the process of finding the intersection points of two polar curves without graphing by setting them equal to each other.
Using trigonometric identities to simplify the process of finding intersections of polar curves, such as cosine squared theta.
Exploring the unique behavior of polar curves intersecting at the same point but at different angles, as seen when they pass through the origin at different times.
Providing a comprehensive understanding of the mathematical concepts and methods involved in calculating areas and lengths of polar curves.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: