AP Calculus BC Lesson 9.9
TLDRIn this lesson, the focus is on calculating the area of regions bounded by multiple polar curves. The process involves identifying the inner and outer curves and setting up integrals with appropriate bounds and functions. Examples are worked through, demonstrating how to find intersection points and use symmetry to simplify calculations. The lesson builds understanding of polar equations and their application in finding areas of complex shapes in polar coordinates.
Takeaways
- 📌 The area of a region bounded by a single polar curve is calculated using the formula A = 1/2 ∫ from α to β of r^2 dθ.
- 🔄 To find the area between two polar curves, integrate the outer curve's r^2 and subtract the inner curve's r^2 over the given interval of θ.
- 🔢 For the example with R = 1 + 2cos(θ) and R = 3 + cos(θ), determine which curve is the outer and inner by plugging in θ = 0.
- 📈 The area is found by setting up a single integral with the difference of the outer and inner curve's r^2, ∫ from 0 to π/2 of (3 + cos(θ))^2 - (1 + 2cos(θ))^2.
- 🤔 When the roles of the curves change (inner/outer), use two separate integrals with different α and β values to find the total area.
- 📍 Intersection points of polar curves are found by setting the equations of the two curves equal to each other and solving for θ.
- 🌀 For complex regions, sketching a unit circle and identifying the quadrants involved can help determine the correct bounds for integration.
- 🔄 Use symmetry in polar curves to simplify area calculations by finding half or a quarter of the area and multiplying by the appropriate factor.
- 📊 When using a calculator, remember that even though θ is used, it can be treated as x in the calculator's radian mode for integration.
- 🎯 Always verify the bounds of integration and the number of times a curve completes a circle to ensure accurate area calculations.
- 📝 For non-calculator problems, simplify the integral expressions as much as possible before attempting to find the area.
Q & A
What is the formula used to find the area of a region bounded by a single polar curve?
-The formula to find the area of a region bounded by a single polar curve is A = (1/2) * ∫ from α to β of r^2 dθ, where α is the starting angle, β is the ending angle, and r is the equation for the radius.
How can the area between two polar curves be found?
-The area between two polar curves can be found by applying a similar process to finding the area between curves in Cartesian coordinates, but using polar equations. You calculate the area for the outer curve and subtract the area for the inner curve over the same interval of θ.
What is the first example given in the script for finding the area between two polar curves?
-The first example given is to find the area of the shaded region bounded by r = 1 + 2cos(θ) and r = 3 + cos(θ) on the interval from 0 to π/2.
How do you determine which curve is the outer curve and which is the inner curve in a given problem?
-To determine which curve is the outer or inner curve, you can plug in the endpoints of the interval (usually 0 and π/2) into both curves and see which one produces a larger value of r at the endpoints. The curve with the larger r value at the endpoints is the outer curve.
What is the method used to find the area when the inside and outside curves are changing?
-When the inside and outside curves are changing, you need to design two separate integrals with different α and β values to find the area. You also need to find the points of intersection by setting the two r's equal to each other and solving for θ.
How can symmetry be used to simplify the process of finding the area of shaded regions in polar curves?
-Symmetry can be used to simplify the process by finding the area of one symmetrical part of the shaded region and then multiplying that by the number of identical parts. For example, if a figure is symmetrical about an axis and you find the area of one quarter, you can multiply by 4 to find the total area.
What is the significance of finding the intersection points of two polar curves?
-Finding the intersection points of two polar curves is significant because it helps determine the bounds of the integrals used to calculate the area of the shaded regions. These points are where the two curves meet, and they define the limits within which the area is being calculated.
How can you verify that a polar curve traces its way around the circle the correct number of times?
-You can verify this by setting the polar radius equation equal to a constant value and solving for θ. The number of solutions within the given interval (usually 0 to 2π) will indicate how many times the curve traces around the circle.
What is the integral expression for finding the area of the region between R = 3 and R = 3 - 2sin(2θ) on the closed interval from 0 to π?
-The integral expression for the area is A = (1/2) * ∫ from 0 to π of (3 - 2sin(2θ))^2 dθ for the interval from 0 to π/2, plus (1/2) * ∫ from π/2 to π of 3^2 dθ for the interval from π/2 to π.
In the example with R = 4 and R = 3 + 2cos(θ) intersecting at θ = π/3 and θ = 5π/3, how do you set up the integral to find the area of the shaded region?
-You set up the integral from π/3 to 5π/3 using the outer curve R = 4 and the inner curve R = 3 + 2cos(θ). The integral expression is A = (1/2) * ∫ from π/3 to 5π/3 of (4^2 - (3 + 2cos(θ))^2) dθ.
What is the area of the shaded region between R = 3 and R = 3 - 2sin(2θ) on the closed interval from 0 to π?
-The area of the shaded region is approximately 9.708, obtained by evaluating the integral expression from the previous answer.
Outlines
📚 Introduction to Finding Area Between Polar Curves
This paragraph introduces the concept of calculating the area between multiple polar curves. It explains that the method previously learned for finding the area between a single polar curve can be extended to more complex scenarios involving multiple curves. The paragraph provides an example of finding the area between two specific polar curves, R = 1 + 2cos(θ) and R = 3 + cos(θ), over the interval from 0 to π/2. It emphasizes the importance of identifying the outer and inner curves and the process of setting up and simplifying the integral expressions to calculate the area.
🔍 Determining Curves and Intersection Points
The paragraph delves into the process of determining which of the given polar curves is the outer and which is the inner curve. It explains how to find the intersection points by setting the equations of the curves equal to each other and solving for θ. The example provided involves the curves R = 2 and R = 3 + 2cos(θ), and the method for finding the intersection points at θ = 2π/3 and θ = 4π/3 is discussed. The paragraph also touches on the importance of understanding the orientation of the curves and how to approach calculating the area when the roles of the curves change.
📊 Area Calculation with Changing Inner Curves
This section focuses on calculating the area when the inner curve changes throughout the interval. The example given involves the curves R = 2 and R = 3 + 2cos(θ), and the area is calculated in two separate regions due to the changing roles of the inner curve. The process of setting up the integrals for each region and adding them together to find the total area is explained in detail. The paragraph also provides tips for solving such problems, including the use of symmetry and the importance of accurate intersection point determination.
🤔 Multiple Choice Questions and Verification
The paragraph presents multiple choice questions related to finding the area between polar curves, emphasizing the need for verification of certain properties of the curves, such as the number of radians it takes for a curve to complete a full trace. The questions involve identifying the correct curves and calculating the sum of areas for shaded regions based on the given polar equations. The importance of verifying that the curve traces exactly the right amount of radians is highlighted to ensure accurate area calculations.
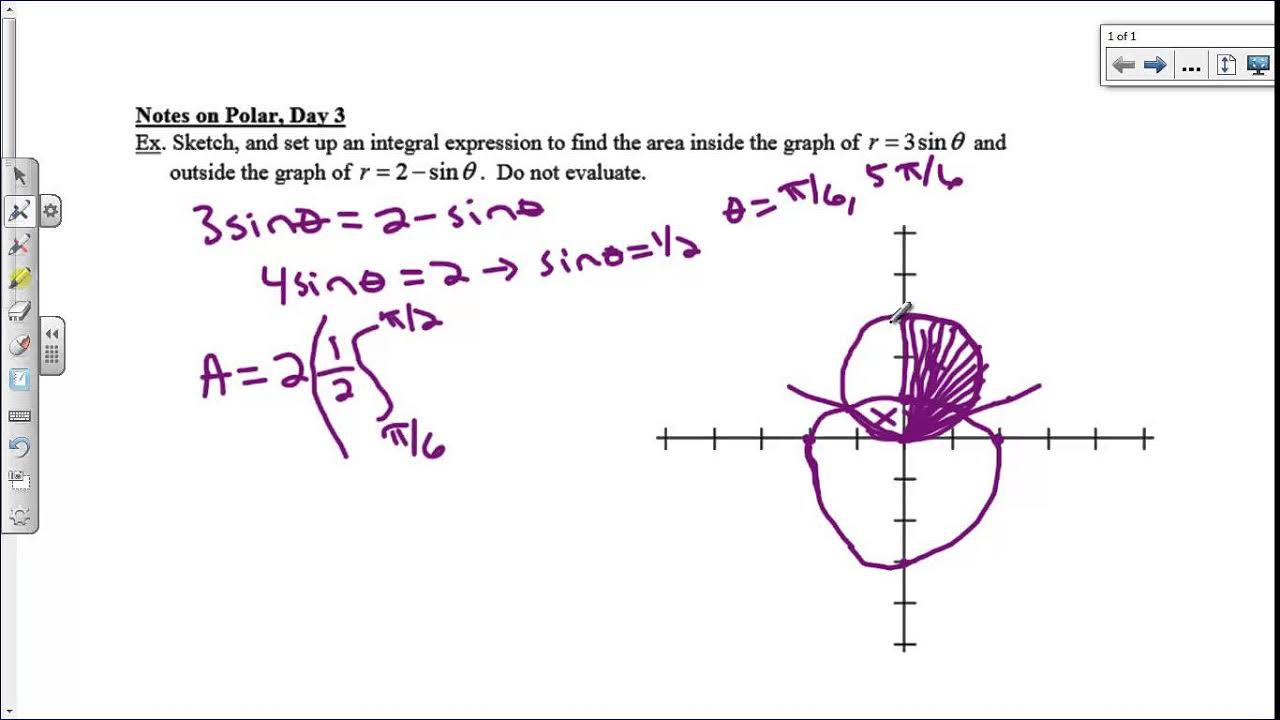
📐 Non-Calculator Free Response Questions
This section discusses non-calculator free response questions involving polar curves. The paragraph outlines the process of setting up integral expressions to find the area of shaded regions based on the given polar equations. It provides examples of how to identify the outer and inner curves, determine the correct intervals for integration, and calculate the area using integral expressions. The paragraph emphasizes the use of symmetry and the importance of accurate bounds for integration in these types of problems.
📝 Calculator-Free Response Question
The final paragraph presents a calculator-free response question involving the polar curves R = 3 and R = 3 - 2sin(2θ). The task is to find the area of the shaded region on the closed interval from 0 to π. The paragraph explains the process of setting up two separate integrals with different integrands due to the changing inner curve throughout the interval. The method for identifying the correct bounds and integrands for each part of the interval is described, and the steps for calculating the total area are outlined.
Mindmap
Keywords
💡Polar Curves
💡Area Calculation
💡Integration
💡Theta (θ)
💡Radius (r)
💡Inner and Outer Curves
💡Intersection Points
💡Polar Coordinates
💡Symmetry
💡Bounds
Highlights
Discussion of finding the area of regions bounded by multiple polar curves.
Explanation of the formula for the area bounded by a single polar curve, where area equals one-half the integral from alpha to beta of r squared d theta.
Process for determining the outer and inner curves when dealing with two polar curves.
Example of finding the area of the shaded region bounded by R=1+2cos(theta) and R=3+cos(theta) on the interval from 0 to pi/2.
Method for identifying the outer and inner curves by plugging in values to the polar curve equations.
Explanation of how to set up and simplify the integral expression for the area of the shaded region.
Calculation of the area using the simplified integral expression and the result of 6.105.
Discussion on the complexity of finding areas with multiple polar curves when the inside and outside curves change.
Illustration of how to find the intersection points of two polar curves by setting the radii equal to each other.
Explanation of using separate integrals with different alpha and beta values to find the area of a region.
Demonstration of how to find the area of a region when the inside curve changes, using two separate integrals.
Verification of the total area enclosed by a polar curve by checking the radians it takes to trace the curve.
Strategy for finding the area with two polar curves by setting r equal to R and solving for theta.
Use of symmetry to simplify the process of finding the area of regions bounded by polar curves.
Explanation of how to determine which curve is the inner and which is the outer by plugging in theta values.
Process of finding the area of a region with changing inside curves by splitting it into two separate regions.
Method for finding the polar zeros and using symmetry to help in determining the area of regions bounded by polar curves.
Example of calculating the area of a region with changing inside curves and the resulting total area of 10.370.
Tips for finding the area with two polar curves, including determining the intersection points and checking the radians for curve tracing.
Multiple choice question involving identifying the correct polar curve and calculating the sum of the areas of the shaded regions.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: