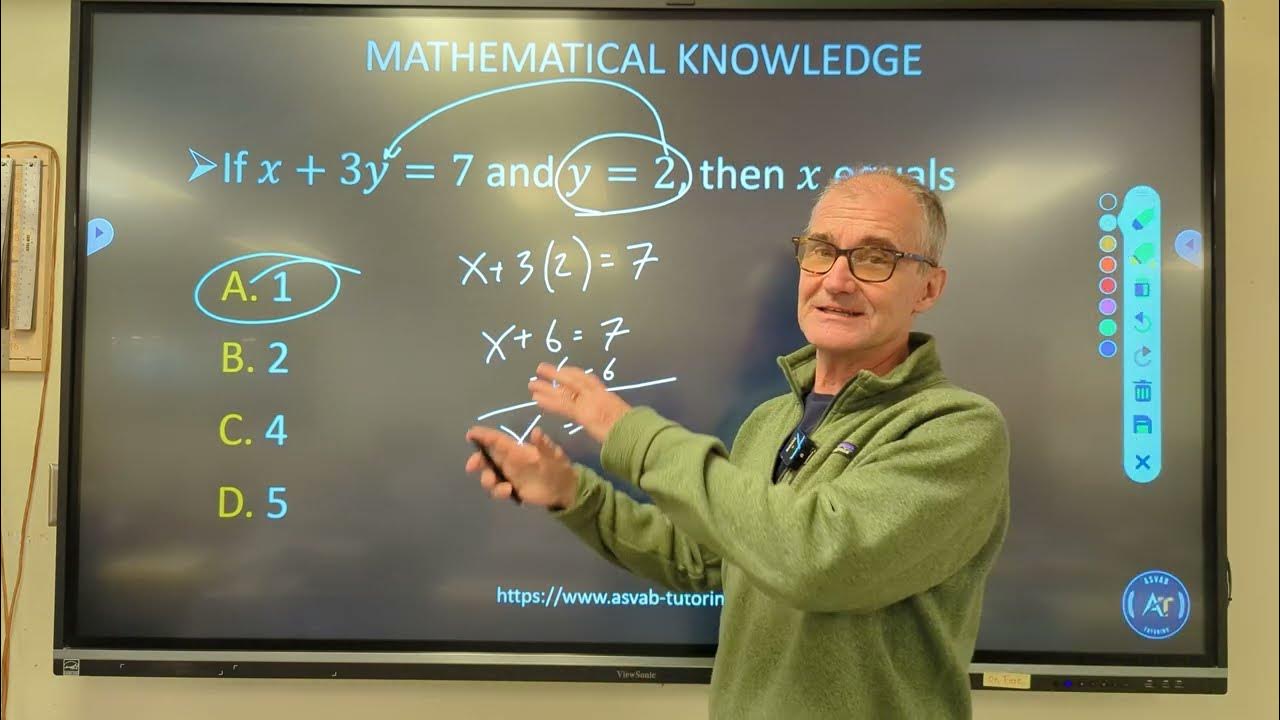Pre-Algebra Final Exam Review
TLDRThis video script is a comprehensive guide for students preparing for their pre-algebra final exam. It covers a variety of topics, including order of operations, solving equations, understanding geometric concepts, and calculating probabilities. The instructor uses step-by-step explanations and real-life examples to clarify complex concepts, making the material accessible and engaging. The video also encourages students to practice problems on their own and provides resources for further learning, ensuring a well-rounded review for the exam.
Takeaways
- 📝 The video is a pre-algebra final exam review providing a multiple-choice practice test for students.
- 📌 Familiarity with the order of operations (PEMDAS) is essential for solving mathematical expressions correctly.
- 🔢 Understanding the concept of exponents and their priority in calculations is crucial, such as 4 squared meaning 4 times 4.
- 🔄 When solving equations, it's important to isolate the variable (e.g., getting rid of addition by subtracting, or eliminating multiplication by dividing).
- 🤔 To add or subtract fractions with different denominators, find a common denominator and then perform the operation on the numerators.
- 📐 Pythagorean theorem (a^2 + b^2 = c^2) is used to find the missing side length of a right triangle.
- 🌐 For monomials, the greatest common factor (GCF) is determined by identifying common factors in both terms.
- 📈 To factor a binomial, find the GCF and then express the expression as the GCF times the difference of the terms.
- 📊 In geometry, understanding the relationships between angles (vertical, alternate interior/exterior, corresponding, and supplementary) is important.
- 🔢 The script provides methods for calculating the volume and surface area of geometric shapes like cones and rectangular prisms.
- 💡 The video encourages pausing to attempt problems independently before checking answers against the provided solutions.
Q & A
What is the order of operations referred to as in the transcript?
-The order of operations referred to in the transcript is PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right).
How does one determine the value of x in the equation 3x + 7 = 19?
-To determine the value of x in the equation 3x + 7 = 19, you first subtract 7 from both sides of the equation to isolate the term with x. This results in 3x = 12. Then, you divide both sides by 3 to solve for x, which gives you x = 4.
What is the Pythagorean theorem mentioned in the transcript?
-The Pythagorean theorem mentioned in the transcript is a fundamental principle in geometry, stating that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It is expressed as a^2 + b^2 = c^2, where c represents the hypotenuse and a and b represent the other two sides.
How does one add two fractions with different denominators?
-To add two fractions with different denominators, you first need to find a common denominator, usually by multiplying the two denominators together. Then, you convert each fraction to an equivalent fraction with the common denominator as the new bottom number (by multiplying both the numerator and the denominator by the necessary factor). After that, you can add the numerators of the two fractions and keep the common denominator, resulting in a single fraction.
What is the greatest common factor (GCF) of the monomials 35a^2b^3 and 42ab^3?
-The greatest common factor (GCF) of the monomials 35a^2b^3 and 42ab^3 is 7a^2b^3. This is found by identifying the common factors of the coefficients (7 in this case) and the lowest powers of the variables present in both monomials (a^2 and b^3).
How does one solve the inequality Y ≥ 3?
-To solve the inequality Y ≥ 3, you would graph the number line and mark the point 3 on it. Since the inequality includes equality (the 'greater than or equal to' part), you would use a closed circle to mark the 3 on the number line. Then, you would shade the portion of the number line that is to the right of the 3, indicating all values of Y that are either equal to or greater than 3 satisfy the inequality.
What is the least common multiple (LCM) of the monomials a^2 and a^3?
-The least common multiple (LCM) of the monomials a^2 and a^3 is a^3. When finding the LCM of monomials with the same base, the LCM is the monomial with the highest exponent.
How does the speaker calculate the area and perimeter of a rectangle with given dimensions?
-The speaker calculates the area of the rectangle by multiplying the length by the width (8 feet times 5 feet equals 40 square feet). The perimeter is calculated by adding the lengths of all four sides, which is 2 times the length plus 2 times the width (2 times 8 feet plus 2 times 5 feet equals 26 feet).
What is the volume of a cylinder with a radius of 8 feet and a height of 12 feet?
-The volume of a cylinder is calculated by multiplying the area of the base (which is πr^2, where r is the radius) by the height (h). With a radius of 8 feet and a height of 12 feet, the volume is π * (8^2) * 12, which equals approximately 2,412.7 cubic feet.
How does the speaker solve the complex fraction 28/36 divided by 35/81?
-The speaker applies the rule for dividing fractions, which is to change the division to multiplication and then flip the second fraction. So, 28/36 divided by 35/81 becomes 28/36 * 81/35. The speaker then simplifies the fractions by canceling out common factors (7 and 9 and 4), which results in the final answer of 9/5.
What is the midpoint between the points (2, 3) and (-8, 8)?
-The midpoint between two points (x1, y1) and (x2, y2) is calculated as ((x1 + x2)/2, (y1 + y2)/2). For the points (2, 3) and (-8, 8), the midpoint is ((2 - 8)/2, (3 + 8)/2), which simplifies to (-6/2, 11/2) or (-3, 5.5).
Outlines
📝 Pre-Algebra Final Exam Review
This paragraph introduces a video designed as a review for the pre-algebra final exam. It emphasizes the importance of understanding the order of operations (PEMDAS), and provides a step-by-step guide to solving multiple-choice questions. The focus is on practicing problems involving order of operations, exponents, and basic algebraic manipulations.
🧩 Solving Equations and Inequalities
The second paragraph delves into solving equations and inequalities. It explains how to isolate variables, apply operations, and handle complex fractions. The paragraph also covers finding the least common multiple (LCM) of monomials and solving inequalities graphically on a number line.
📊 Geometry Problems: Triangles and Circles
This paragraph focuses on geometry problems involving triangles and circles. It covers the application of Pythagorean theorem to find missing sides of triangles, calculating the circumference and area of a circle, and determining the volume and surface area of a cylinder.
🤔 Algebraic Expressions and Monomials
The fourth paragraph discusses the manipulation of algebraic expressions and monomials. It explains how to factor binomials, calculate the greatest common factor (GCF), and work with monomials involving exponents. The paragraph also touches on the multiplication and division of monomials with the same base.
🛒 Financial Math and Percentages
The fifth paragraph applies mathematical concepts to real-world financial scenarios. It covers calculating percentages, understanding discounts and tax implications on purchases, and determining the total cost of items. The paragraph also explains how to calculate interest on annuities and compare prices to find the best value.
📐 Coordinate Geometry and Transformations
The final paragraph focuses on coordinate geometry and graphical transformations. It discusses how to find the new coordinates of a point after transformations such as reflections over the x-axis, y-axis, and the origin, as well as rotations and translations. The paragraph emphasizes the importance of understanding how these transformations affect the coordinates of points on a graph.
Mindmap
Keywords
💡Pre-Algebra
💡Order of Operations (PEMDAS)
💡Variables
💡Solving Equations
💡Pythagorean Theorem
💡Fractions and Common Denominators
💡Exponents
💡Absolute Value
💡Greatest Common Factor (GCF)
💡Factoring
💡Geometry
Highlights
The video serves as a comprehensive review for pre-algebra final exam, providing multiple-choice questions and detailed explanations.
The concept of order of operations (PEMDAS) is introduced and used to solve the first problem, emphasizing the importance of understanding mathematical priorities.
The video explains how to substitute variables in expressions and solve for their values, as demonstrated in problem two involving powers and absolute values.
Problem three illustrates the process of solving linear equations and isolating variables, using subtraction and division to find the value of x.
The fourth problem shows how to add fractions with different denominators by finding a common denominator and adding numerators.
Pythagorean theorem is utilized in problem five to find the missing side length of a triangle, demonstrating its application in geometry.
The video covers solving equations with fractions, using the concept of opposite operations to isolate variables, as shown in problem six.
Problem seven explains how to find the greatest common factor (GCF) of monomials, which is crucial for simplifying and comparing algebraic expressions.
The process of multiplying monomials and combining like terms is detailed in problem eight, which is essential for expanding and simplifying algebraic expressions.
Problem nine involves dividing monomials and handling negative exponents, showcasing techniques for simplifying complex algebraic expressions.
The video teaches how to factor binomials by determining the GCF and using the distributive property, as demonstrated in problem ten.
The diameter and radius of a circle are discussed in problem eleven, along with calculating the circle's circumference and area using formulas.
Problem twelve covers calculating the area and perimeter of a rectangle, providing a straightforward method for determining these properties.
The volume and surface area of a cylinder are calculated in problem thirteen, using formulas and demonstrating how to handle units of measurement.
Complex fractions are simplified in problem fourteen using the method of keeping, changing, flipping, and simplifying large numbers.
The video explains how to find the value of a variable in an equation involving fractions, using the reciprocal property, as shown in problem fifteen.
Problem sixteen demonstrates how to find the midpoint between two points, which is crucial for understanding coordinates and distances in geometry.
The least common multiple (LCM) of monomials is calculated in problem seventeen, highlighting the importance of identifying the highest exponents for each variable.
Inequalities are solved and graphed on a number line in problem eighteen, teaching essential skills for understanding and visualizing mathematical relationships.
Problem nineteen involves finding the value of a variable in a figure, emphasizing the importance of adding angles in a quadrilateral and using their sum to solve the problem.
Triangles similarity is used in problem twenty to find the value of an unknown side, demonstrating the use of proportions and equivalent ratios in geometry.
The video concludes with problem twenty-one, where it explains how to calculate the remaining amount of a substance after usage, in this case, flour for making cakes.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: