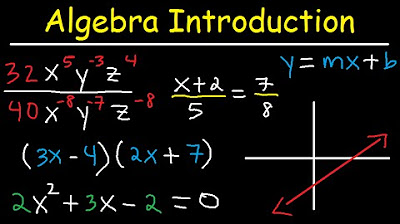
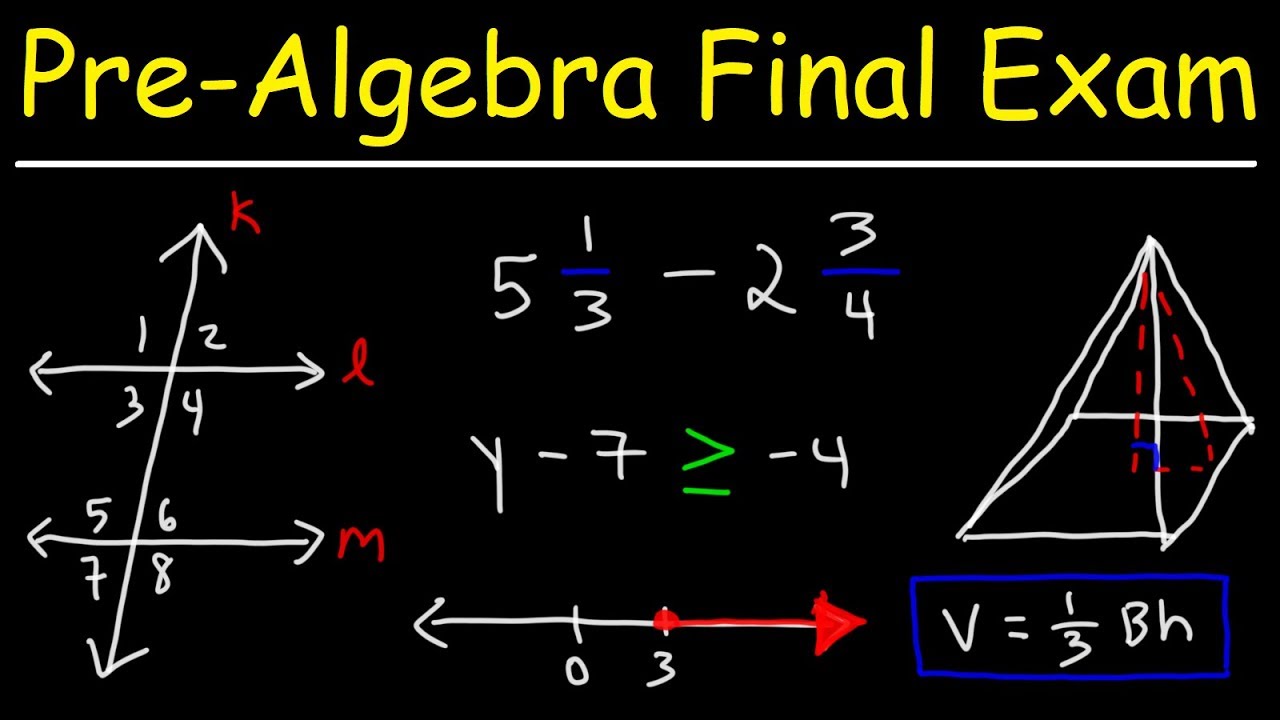
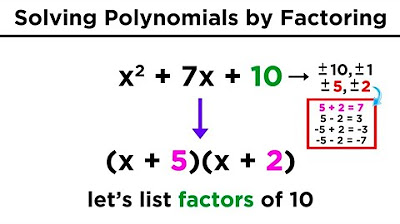
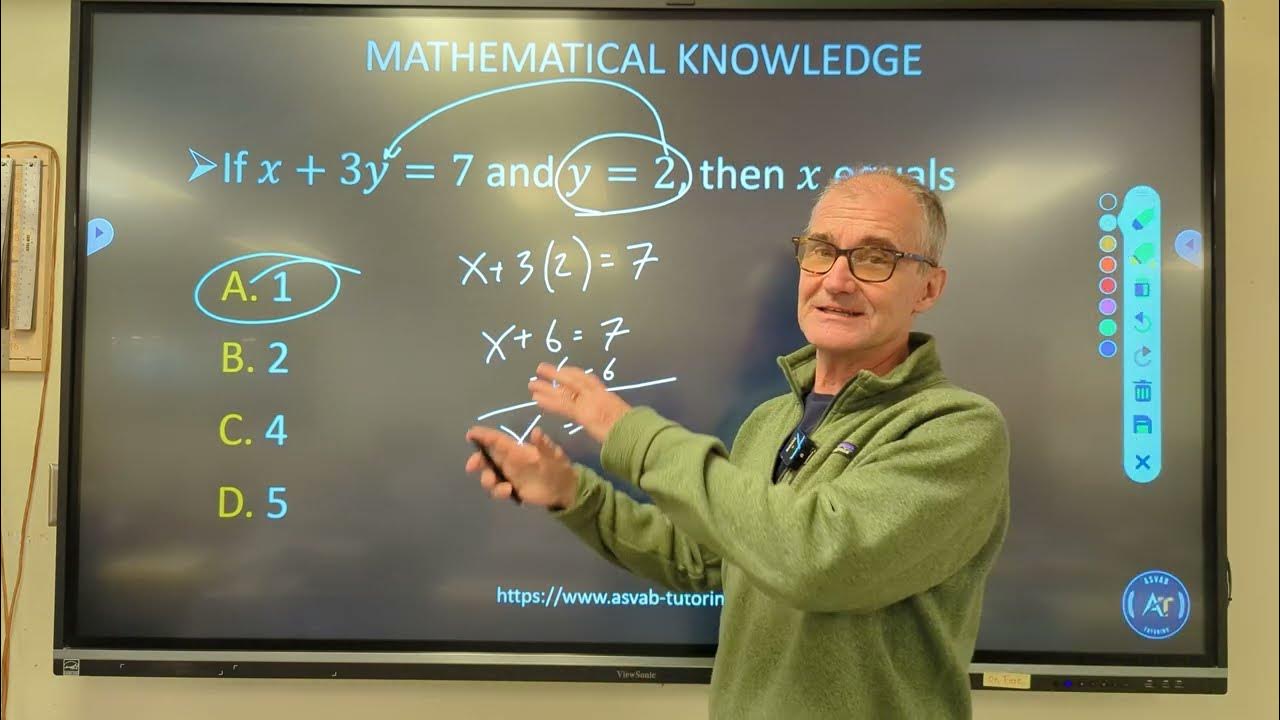Algebra Final Exam Review
TLDRThe video script is an educational guide for students preparing for their algebra final exam. It covers various algebraic concepts and problem-solving techniques, including the FOIL method for multiplying binomials, distributing and combining like terms, calculating slopes of lines, applying the order of operations (PEMDAS), and factoring expressions using difference of squares and cubes. The script also explains how to solve linear equations and systems of equations, and graph linear equations. The instructor encourages students to practice problems independently before checking their answers against the video's solutions.
Takeaways
- 📚 The video is a study guide for an algebra final exam, covering various algebraic concepts and problem-solving techniques.
- 📈 The FOIL method is introduced for multiplying binomials, demonstrated through an example involving 3x and 4x.
- 📊 When dealing with polynomials, the video explains how to distribute and combine like terms to simplify expressions.
- 📐 The concept of slope is discussed, with a formula provided to calculate the slope of a line given two points.
- 🔢 The order of operations (PEMDAS) is emphasized for solving expressions involving parentheses and various operations.
- 📶 Factoring expressions is explained using the difference of squares and perfect cubes formulas, with examples provided for clarity.
- 🔍 The video provides a method for solving linear equations, including distributing and simplifying to isolate variables.
- 📊 The process of graphing linear equations is detailed, with steps for finding intercepts and plotting points.
- 📝 The video script includes a problem involving a rectangle's area and perimeter, with a step-by-step solution provided.
- 📑 The transcript concludes with additional resources for further algebra practice, directing viewers to a YouTube channel for more examples.
- 🎯 The video aims to reinforce algebraic concepts and build problem-solving skills in preparation for a final exam.
Q & A
What is the main purpose of the video?
-The main purpose of the video is to help students prepare for their algebra final exam by going over various algebra problems and providing step-by-step solutions.
What method is used to multiply two binomials together?
-The FOIL method is used to multiply two binomials together.
How is the difference of two polynomials calculated?
-The difference of two polynomials is calculated by distributing the negative sign through the parentheses and then combining like terms.
What formula is used to find the slope of a line passing through two points?
-The formula used to find the slope of a line passing through two points is (y2 - y1) / (x2 - x1).
What does PEMDAS stand for in the context of the order of operations?
-In the context of the order of operations, PEMDAS stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right).
How can the difference of squares formula be applied in factoring?
-The difference of squares formula, a^2 - b^2, can be applied in factoring by taking the square root of each term, adding or subtracting the square roots based on the sign between the terms, and then factoring as needed.
What is the process of factoring by grouping?
-Factoring by grouping involves taking out the greatest common factor (GCF) from two or more terms, then factoring the expression by grouping terms that have a common factor.
What is the difference of perfect cubes formula?
-The difference of perfect cubes formula is a^3 - b^3, which can be factored into (a - b)(a^2 + ab + b^2).
How is the solution found for a linear equation with fractions?
-To find the solution for a linear equation with fractions, you cross-multiply to eliminate the fractions, then simplify and isolate the variable to find the value.
How can the quadratic formula be used to solve a quadratic equation?
-The quadratic formula, x = (-b ± √(b^2 - 4ac)) / (2a), can be used to solve a quadratic equation by plugging in the values of a, b, and c from the equation ax^2 + bx + c = 0, and then solving for x.
What are the steps to graph a linear equation in slope-intercept form?
-To graph a linear equation in slope-intercept form (y = mx + b), plot the y-intercept (b), use the slope (m) to find another point by moving up m units and right 1 unit, and then draw a straight line through the two points.
Outlines
📚 Algebra Study Guide: FOIL Method and Polynomials
This paragraph introduces a video aimed at helping students prepare for their algebra final exam. It discusses the use of the FOIL method for multiplying binomials and provides a step-by-step solution to a problem involving the multiplication of two binomials. The paragraph also emphasizes the importance of practicing problems independently before checking answers. Additionally, it covers simplifying polynomial expressions, including distributing negative signs and combining like terms, with a focus on solving for equivalent expressions and differences of polynomials.
🧠 Understanding Pemdas and Order of Operations
This section delves into the concept of Pemdas (Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction) and its application in solving algebraic expressions. It explains the process of solving problems by first addressing parentheses and then moving on to exponents, followed by multiplication and division, and finally addition and subtraction. The paragraph provides a detailed example of applying Pemdas to a complex expression, resulting in the correct answer choice. It also touches on factoring expressions completely, using the difference of squares method for a specific problem and explaining the steps involved in the process.
🔢 Factoring Techniques and Solving Quadratic Equations
The paragraph focuses on various factoring techniques, including the difference of squares and factoring by grouping. It explains how to find two numbers that multiply to a specific product and add up to a given coefficient. The process of factoring a trinomial with a leading coefficient of one is detailed, as well as a method for factoring a trinomial with a leading coefficient that is not one. Additionally, the paragraph covers solving quadratic equations by factoring and using the quadratic formula, providing examples and the steps to arrive at the correct solutions.
📈 Graphing Linear Equations and Systems
This part of the script discusses the process of graphing linear equations, including both slope-intercept and standard form equations. It explains how to find intercepts and use them to plot equations on a graph. The paragraph also covers solving systems of linear equations using the elimination method, providing a step-by-step guide to finding the solution. The explanation includes how to manipulate equations to cancel out variables and arrive at the values of x and y, ultimately resulting in the correct ordered pair solution.
🤔 Problem Solving: Quadratic Equations and Geometric Shapes
The paragraph presents a problem-solving approach to quadratic equations and geometric shapes. It explains how to use the quadratic formula to solve equations and provides a detailed calculation of the solution. Additionally, it tackles a real-world problem involving the area and perimeter of a rectangle, demonstrating how to set up and solve equations based on given conditions. The paragraph concludes with a practical application of geometry, showing how to calculate the perimeter of a rectangle using the derived length and width.
📏 Graphing Linear Equations: Intercepts and Slope-Intercept Form
This section focuses on the methods for graphing linear equations, emphasizing the use of intercepts and the slope-intercept form. It explains how to graph equations with single variables, such as x equals a number and y equals a number, using vertical and horizontal lines. The paragraph then describes how to graph linear equations in standard form by finding x and y intercepts and connecting them with a line. It also covers graphing linear equations in slope-intercept form, starting with the y-intercept and using the slope to find additional points on the graph.
Mindmap
Keywords
💡Algebra
💡FOIL Method
💡Polynomials
💡Slope-Intercept Form
💡Graphing
💡Factoring
💡Solving Equations
💡Quadratic Equations
💡Systems of Linear Equations
💡Difference of Squares
💡Order of Operations (PEMDAS)
Highlights
The video is aimed at students preparing for their final exam in algebra.
The FOIL method is used for multiplying two binomials.
When distributing a negative sign without a term in front, the parentheses can be removed without changing the signs.
The slope-intercept form of a line is y = mx + b, where m is the slope and b is the y-intercept.
The formula for the difference of squares is (a + b)(a - b) = a^2 - b^2.
Factoring a trinomial with a leading coefficient of one involves finding two numbers that multiply to the product of the leading coefficient and the constant term, and add up to the middle coefficient.
The difference of perfect cubes formula is a^3 - b^3 = (a - b)(a^2 + ab + b^2).
To solve an equation, isolate the variable on one side of the equation.
For a quadratic equation, the quadratic formula is x = (-b ± √(b^2 - 4ac)) / (2a).
In solving a system of linear equations, both substitution and elimination methods can be used.
To find the perimeter of a rectangle, sum all four sides: P = 2l + 2w.
For graphing a linear equation in standard form, find the x and y intercepts and connect them with a line.
In slope-intercept form, y = mx + b, the y-intercept is b and the slope is m.
To graph a vertical line, set x equal to a constant value.
To graph a horizontal line, set y equal to a constant value.
When factoring, if the leading coefficient is not one, multiply the leading coefficient by the constant term to find the product needed for factoring.
For a linear equation in slope-intercept form, use the slope to find additional points on the line after plotting the y-intercept.
When solving a quadratic equation by factoring, set each factor equal to zero to find the solutions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: