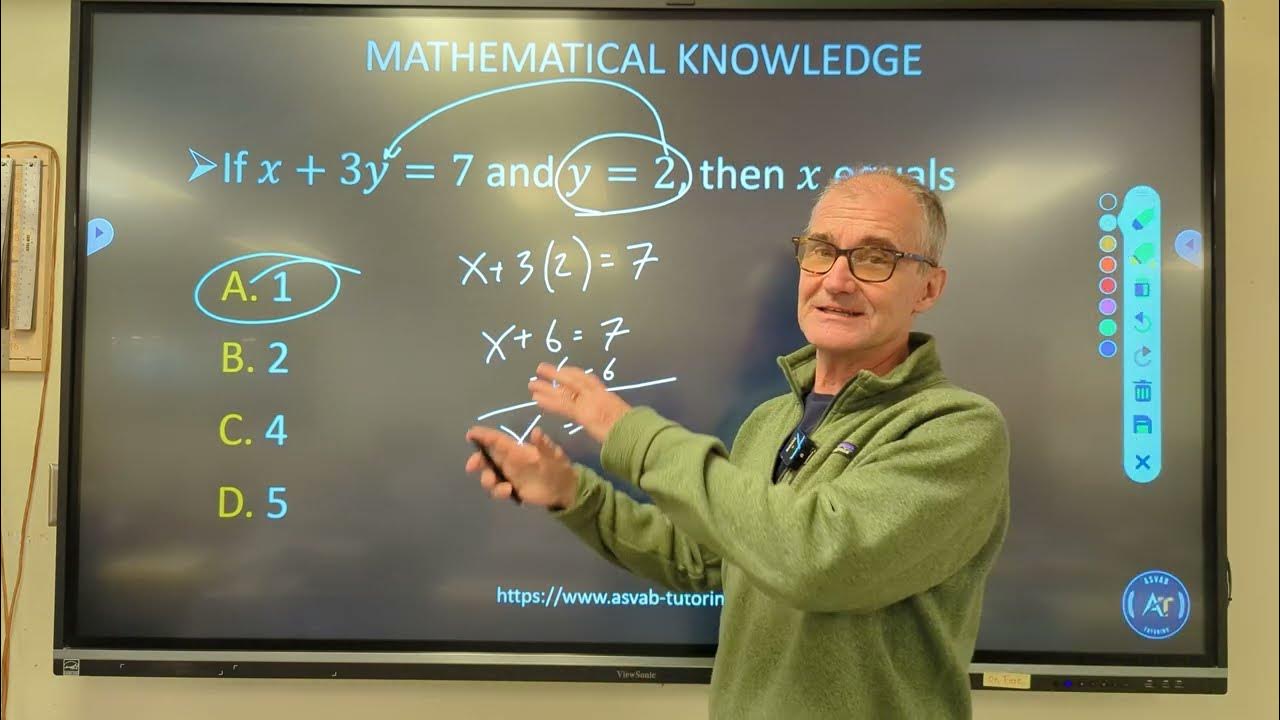ATI TEAS Test Math Review - Study Guide

The Organic Chemistry Tutor

22 May 202157:28

EducationalLearning

32 Likes 10 Comments

TLDRThis instructional video guide offers strategies for tackling the math section of the TEAS exam. It encourages viewers to practice by pausing after each question to solve it independently before checking the solution. The script covers various topics including order of operations, solving equations, converting decimals to fractions, and understanding percentages. It also addresses more complex concepts like absolute value equations and calculating averages, with a focus on step-by-step problem-solving techniques.

Takeaways

📚 The video is designed to assist individuals preparing for the math section of the TEAS exam by encouraging active participation in solving problems alongside the video.
🔢 The importance of understanding the order of operations (PEMDAS) is highlighted for correctly solving mathematical expressions, including handling parentheses, exponents, multiplication, division, addition, and subtraction in the right sequence.
📉 The script demonstrates how to solve equations by isolating the variable, using methods such as subtracting terms from both sides and using opposite operations to isolate the variable.
🔃 A step-by-step guide on converting decimals to fractions is provided, including multiplying by a power of 10 to eliminate the decimal and simplifying the resulting fraction.
🔄 The process of converting fractions to decimals is explained using long division, providing a method to verify the equivalence of fractions and decimals.
💊 The video covers the conversion of percentages to decimals by dividing by 100, moving the decimal point two places to the left, and provides examples of such conversions.
📈 The concept of percentage increase is introduced with an example of increasing medication dosage, illustrating how to calculate the new dosage based on a percentage increase from the original amount.
🤑 The script explains how to calculate take-home pay after taxes, including calculating overtime pay and subtracting federal income taxes, social security, and medicare taxes.
📐 The process of solving absolute value equations is demonstrated, showing how to handle two possible scenarios resulting from the absolute value.
📈 The video provides a method for calculating the average of a set of numbers by summing them and dividing by the count of numbers.
📊 The script discusses how to find the sum of mean, median, mode, and range for a set of numbers, including arranging numbers in ascending order and performing the necessary calculations.

Q & A

What is the recommended method for learning math problems from the video?
-The recommended method is to pause the video for each question, try solving the problem yourself, and then unpause to check your solution against the provided one.
What does the acronym PEMDAS stand for in the context of mathematical operations?
-PEMDAS stands for Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right), indicating the order of operations to be followed in mathematical expressions.
How do you solve the equation to find the value of x when the equation is 5x + 12 = 20?
-First, subtract 3x from both sides to isolate x terms on one side. Then, subtract 12 from both sides to move constants to the other side. Finally, divide by 2 to solve for x, which gives x = 4.
How can you convert a decimal to a fraction?
-Convert the decimal to a fraction by placing the decimal number over 1 and then multiplying both the numerator and denominator by a factor that will eliminate the decimal point, resulting in a simplified fraction.
What is the fraction equivalent to the decimal 0.35?
-The decimal 0.35 is equivalent to the fraction 35/100, which simplifies to 7/20 after canceling common factors.
How do you convert a percentage to a decimal?
-To convert a percentage to a decimal, divide the percentage by 100, which involves moving the decimal point two places to the left.
What is the process of multiplying binomials (FOIL method)?
-The FOIL method involves multiplying the First terms, Outer terms, Inner terms, and Last terms of the binomials and then combining like terms to simplify the result.
How much should be administered to John if he is currently receiving 300 mg of medication and the dosage needs to be increased by 25%?
-To increase the dosage by 25%, calculate 25% of 300 mg, which is 75 mg, and then add this to the original 300 mg, resulting in a total of 375 mg to be administered.
What is the process to calculate Megan's take-home pay after working 60 hours, including overtime and taxes?
-Calculate her base pay for the first 40 hours at $28 per hour, then calculate overtime pay for the additional 20 hours at 1.5 times her base hourly rate. Sum these amounts to get total earnings, then subtract 30% for taxes to find her take-home pay.
How do you solve an equation to isolate x when the equation is 3x/4 = 13 - 7?
-First, subtract 7 from both sides to simplify the right side. Then, multiply both sides by 4 to eliminate the fraction, and finally divide by 3 to solve for x, which gives x = 8.
What is the sum of the mean, median, mode, and range of a set of numbers?
-Calculate the mean by summing all numbers and dividing by the count. Identify the median as the middle number when arranged in order. The mode is the most frequent number. The range is the difference between the highest and lowest numbers. Sum these values to get the final result.
How do you convert pounds to kilograms?
-Use the conversion factor where 1 kilogram equals 2.2 pounds. Divide the weight in pounds by 2.2 to get the weight in kilograms.
What is the process to find the area of a composite figure?
-Break the composite figure into smaller rectangles or shapes. Calculate the area of each rectangle by multiplying the length by the width. Sum these areas to get the total area of the composite figure.
How much change should Jason receive if he buys items totaling $2.45 and pays with a $5 bill?
-Subtract the total cost of the items from $5 to find the change. $5 - $2.45 equals $2.55 in change.
What is the percent increase in the number of patients admitted to Hospital XYZ from 2017 to 2018?
-Calculate the difference between the new and original values, divide by the original value, and multiply by 100 to get the percent increase, which is 12.5% in this case.
How do you express a fraction as a percentage?
-Convert the fraction to a decimal by performing the division, and then multiply the decimal by 100 to get the percentage.
What is the result of multiplying 4.5 by 2.36 without using a calculator?
-Multiply the numbers digit by digit, remembering to carry over where necessary. The result of 4.5 times 2.36 is 10.62.
How many employees in a hospital of 800 are neither doctors nor nurses if 5% are doctors and one-fourth are nurses?
-Calculate 5% of 800 for doctors and one-fourth of 800 for nurses. Subtract the sum of these two numbers from the total to find the number of employees who are neither, which is 560.
What is the perimeter of a rectangle that is 5 feet wide and 8 feet long?
-The perimeter is the sum of all sides. For a rectangle, this is twice the width plus twice the length. So, 5 + 5 + 8 + 8 equals 26 feet.

Outlines

00:00

📚 Math Study Tips for TEAS Exam

This paragraph introduces a video aimed at helping viewers with the math section of the TEAS exam. The speaker suggests pausing the video to attempt each problem before revealing the solution. The first example involves evaluating an expression using the order of operations (PEMDAS), which results in the answer 21. The second example demonstrates solving an equation by isolating the variable, resulting in x = 4. The paragraph emphasizes the importance of practice and understanding the mathematical concepts presented.

05:01

🔢 Converting Decimals and Percentages

The speaker discusses methods for converting decimals to fractions and percentages to decimals. For decimals, the process involves dividing by 1 and then multiplying by a power of 10 to eliminate the decimal point, simplifying the fraction. To convert percentages to decimals, one divides by 100, moving the decimal two places to the left. Examples are provided, such as converting 0.35 to a fraction (7/20) and 23.8% to a decimal (0.238). The paragraph also covers converting fractions to decimals using long division.

10:02

📝 Algebraic Expressions and Problem Solving

This section focuses on algebraic expressions, starting with simplifying 'three x minus two times two x plus five' to 6x squared plus 11x minus 10. The speaker then provides an example of how to calculate an increased dosage of medication by applying a percentage increase to a base amount, resulting in 375 milligrams. The paragraph also covers Megan's earnings as a nurse, including overtime pay and tax deductions, concluding with her take-home pay calculation.

15:04

🧮 Advanced Math Concepts and Equations

The paragraph delves into more complex math problems, such as solving for x in an equation with fractions and multiples, resulting in x = 8. It also explains how to add mixed fractions by converting them to improper fractions, finding a common denominator, and then converting back to mixed numbers if necessary. Examples include adding 2 1/3 + 3 1/2 and multiplying mixed fractions, with simplification techniques to avoid dealing with large numbers.

20:05

📉 Arranging Numbers and Solving Absolute Values

The speaker instructs how to arrange a set of numbers from least to greatest, starting with negative numbers and then positive ones, converting fractions and percentages to decimals for comparison. The method for solving absolute value equations is presented, resulting in two possible values for x, with the specific answer provided being x = -2. The paragraph also explores Roman numerals, explaining their values and how to interpret the expression MDCLXV as 1667.

25:06

📊 Statistics and Unit Conversions

This paragraph covers calculating averages, such as Sally's average test score, and the sum of mean, median, mode, and range for a set of numbers. It also explains unit conversions, such as pounds to kilograms and milligrams to grams, using conversion factors. Additionally, it describes how to calculate the driving distance in miles from a map's scale and the area of a composite figure by breaking it down into smaller rectangles.

30:08

🛒 Shopping and Percentage Increase Calculation

The speaker provides a real-world example of calculating change from a purchase, detailing the cost of items and the resulting change from a five-dollar bill. It also explains how to calculate the percent increase in the number of patients admitted to a hospital over two years, resulting in a 12.5% increase. The paragraph concludes with expressing a fraction as a percentage by converting it to a decimal and then multiplying by 100.

35:10

🏥 Hospital Staff Composition and Rectangle Geometry

This section calculates the number of hospital employees who are neither doctors nor nurses, given percentages for doctors and a quarter for nurses, resulting in 560 employees. It also explains how to find the perimeter of a rectangle given its width and length, demonstrating the formula and arriving at a perimeter of 26 feet.

Mindmap

Keywords

💡TEAS Exam

The TEAS (Test of Essential Academic Skills) Exam is a standardized test used for admission to many nursing programs. In the video, it is the focus for the math section, indicating that the content is geared towards helping students prepare for this specific exam. The script provides various math problems and solutions that one might encounter in the TEAS math section.

💡Order of Operations

The order of operations, often remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), is a fundamental concept in mathematics that dictates the sequence in which operations should be performed. The video script emphasizes the importance of this concept in solving the first math problem, where the expression is evaluated correctly by following the order.

💡Equation

An equation is a mathematical statement that asserts the equality of two expressions. In the context of the video, solving equations is a key theme, as the script walks through the process of isolating variables to find their values, which is a common task in the TEAS math section.

💡Decimal to Fraction

Converting a decimal to a fraction is a process that involves expressing a non-whole number as a ratio of two integers. The script provides a method to do this by multiplying the decimal by a power of 10 to move the decimal point to the right and create a whole number, which can then be simplified to its lowest terms, as demonstrated in the video with the conversion of 0.35 to 7/20.

💡Percentage

A percentage is a way of expressing a number as a fraction of 100. It is used in the video to illustrate how to convert percentages into decimals, which is a common mathematical operation. The script explains that dividing a percentage by 100 or moving the decimal point two places to the left gives the equivalent decimal form.

💡FOIL Method

The FOIL method is a mnemonic for multiplying two binomials, which stands for First, Outer, Inner, Last. The video script uses this method to expand the product of (3x - 2)(x + 5) into a quadratic expression, demonstrating how to combine like terms to reach the final simplified form.

💡Percent Increase

A percent increase indicates the rate at which a quantity has grown. In the video, the concept is applied to a medical dosage scenario where the script explains how to calculate the new dosage after a 25% increase, demonstrating the process of multiplying the original amount by 1 plus the percentage increase expressed as a decimal.

💡Overtime Pay

Overtime pay refers to the additional compensation earned for hours worked beyond the standard workweek. The script discusses how Megan, a nurse, earns 1.5 times her base pay for every hour worked above 40 hours in a week, illustrating the calculation of her total earnings including overtime.

💡Absolute Value

Absolute value is a mathematical operation that returns the non-negative value of a number, regardless of its sign. The script explains how to solve an equation involving absolute value by creating two separate equations, one assuming the expression inside the absolute value is positive and another assuming it is negative, as shown in the equation 2x - 5 = 9 or 2x - 5 = -9.

💡Mixed Fractions

A mixed fraction is a combination of a whole number and a proper fraction. The video script covers how to add and convert mixed fractions into improper fractions and vice versa, providing examples of converting two and one-third and two and three-eighths into improper fractions and then combining them.

💡Roman Numerals

Roman numerals are a numeral system that originated in ancient Rome and use letters from the Latin alphabet to represent values. The script explains the basic symbols and their values, such as I for 1, V for 5, X for 10, L for 50, C for 100, D for 500, and M for 1000, and provides an example of converting the Roman numeral MDCLXV to its decimal equivalent, 1666.

💡Average

The average, or mean, is a measure of central tendency that represents the sum of a set of numbers divided by the count of numbers in the set. The video script provides an example of calculating the average test score from a set of scores, which involves adding all the scores together and then dividing by the number of scores to find the mean value.

💡Unit Conversion

Unit conversion is the process of changing a physical quantity from one unit to another. The script includes examples of converting pounds to kilograms and milligrams to grams, which are essential skills for understanding measurements in different systems, such as the metric system used in healthcare.

💡Composite Figure

A composite figure is a shape made up of two or more individual shapes. The script describes how to calculate the area of a composite figure by breaking it down into smaller rectangles, calculating the area of each, and then summing these areas to find the total area of the composite figure.

💡Percent Increase

The script explains how to calculate the percent increase in the number of patients admitted to a hospital from one year to the next. This involves finding the difference between the new and original values, dividing by the original value, and then multiplying by 100 to get the percentage change, which in this case is an increase.

💡Percentage as a Decimal

The script demonstrates how to express a fraction as a percentage by first converting the fraction to a decimal using long division and then multiplying the decimal by 100 to get the percentage. This is shown with the fraction 5/8, which is converted to a decimal and then to a percentage, resulting in 62.5%.

💡Perimeter

The perimeter of a shape is the total distance around the shape, calculated by adding the lengths of all its sides. In the context of the video, the script explains how to calculate the perimeter of a rectangle by adding the lengths of all four sides, providing an example with a rectangle that is 5 feet wide and 8 feet long, resulting in a perimeter of 26 feet.

Highlights

The importance of understanding the order of operations (PEMDAS) for solving math problems.

Step-by-step explanation of evaluating expressions using parentheses and exponents.

Solving for a variable by isolating it on one side of the equation through subtraction and division.

Converting decimals to fractions by multiplying the numerator and denominator by powers of ten.

Explanation of converting percentages to decimals by dividing by 100.

Foiling binomials to simplify expressions involving multiplication of two binomials.

Calculating percentage increases and decreases in various scenarios.

Converting between different units of measurement, such as milligrams to grams and pounds to kilograms.

Understanding Roman numerals and their modern equivalents for interpreting historical documents.

Calculating averages by summing up all values and dividing by the number of values.

Finding the mean, median, mode, and range of a data set for basic statistical analysis.

Step-by-step process for solving absolute value equations by considering both positive and negative cases.

Using the keep-change-flip method to divide fractions.

Simplifying complex fractions and converting improper fractions to mixed numbers.

Calculating the area and perimeter of composite figures by breaking them into smaller shapes.

Transcripts

Browse More Related Video

ATI TEAS Version 7 Mathematics Numbers and Algebra (How to Get the Perfect Score)

Algebra Final Exam Review

ASVAB 2024 Mathematical Knowledge Practice Test

Basic Math Review

Pre-Algebra Final Exam Review

The GED Calculator How to Use it

Related Tags

Math Help TEAS Exam Problem Solving Educational Video Study Guide Percentage Conversion Fractions to Decimals Algebra Solutions Healthcare Math Calculation Skills Exam Preparation