2021 Live Review 8 | AP Calculus AB | Reviewing Multiple-Choice & Free-Response Questions
TLDRThe video script is from an educational session, likely part of a series, focusing on calculus. The instructor, Virgil, is energetic and welcoming, indicating it's the last session of the series. The session includes a review of exam details, with the paper exam scheduled for May 4th and a digital exam on June 9th. The format of the exams is discussed, highlighting the structure and rules, such as the use of calculators and the types of questions to expect. The session also involves solving a variety of calculus problems, including integrals, derivatives, and differential equations, with an emphasis on understanding the concepts and making mistakes to learn from them. The instructor encourages students to practice and provides resources for further study. The summary also touches on the importance of courage and continuous learning, reminding students that their worth is not measured by exams but by their growth and effort. The session concludes with well wishes and encouragement for future success.
Takeaways
- 📚 The last session of the AP Calculus course emphasized wrapping up the material and preparing for the exam.
- 🔗 A mini mock exam is available on Google Drive, featuring problems from the two-week journey to help students practice.
- 📅 The exam format includes a paper and pencil test on May 4th and a digital exam on June 9th, with specific timings for multiple-choice and free-response sections.
- ✅ Students are encouraged to pause the video and work through problems independently to solidify their understanding.
- 🚫 The digital exam differs from the paper exam and from last year's digital exam, with no ability to go back and forth between problems.
- 📈 The instructor highlighted the importance of practicing traditional exam formats and using available resources like an app for the digital exam practice.
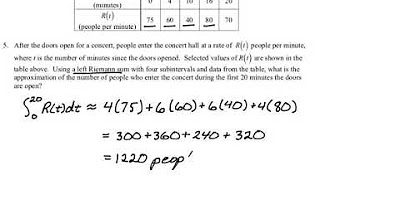
- 🧮 A common mistake when integrating the sum of functions is to forget to multiply the constant by the integration bounds.
- 📉 For a function with a positive first derivative and a negative second derivative, the value increases but at a decreasing rate, which is characteristic of a concave-down function.
- 🤔 The concept of critical points and inflection points was discussed, noting that the presence of these points does not necessarily imply differentiability or continuity.
- 📈 The process of finding a particular solution to a differential equation was demonstrated, including using the initial condition to determine the constant of integration.
- 📝 The instructor shared a personal motivational quote about courage and emphasized that taking challenges and making mistakes are part of the learning process.
Q & A
What is the main purpose of the session described in the transcript?
-The main purpose of the session is to provide a final review or wrap-up of the material covered over a two-week period, preparing students for an upcoming exam by discussing exam format, answering questions, and solving practice problems.
What types of exam formats are mentioned in the transcript?
-The transcript mentions two exam formats: a traditional paper and pencil exam, which includes multiple choice and free response sections, and a digital exam which is different from the traditional format.
How is the paper and pencil exam structured according to the transcript?
-The paper and pencil exam consists of 30 multiple choice questions for 60 minutes without a calculator, followed by 15 multiple choice questions for 45 minutes where a calculator is needed, and finally two free response sections. Between sections, there are short breaks.
What is unique about the digital exam mentioned in the transcript?
-The digital exam differs from the traditional exam and the previous year's digital exam. It does not allow students to move back and forth between questions, and calculators are permitted on all sections.
What is discussed about the practice materials and mock exams?
-The transcript mentions that practice materials and a mini mock exam, which includes problems picked across the two weeks journey, are available. The mock exam is accessible via a link provided under the video, and students are encouraged to print and practice.
How does the transcript address student questions about the exam?
-The transcript addresses questions about the exam format by explaining the structure of both the traditional and digital exams, and by providing guidance on how practice materials can be used effectively to prepare.
What type of mathematical problems are discussed or solved in the session?
-The session includes solving calculus problems such as determining the integral of a function and discussing the properties of differential equations. Specific examples include calculating slopes, handling piecewise functions, and evaluating integrals with respect to x.
What advice is given for students to prepare for the exam?
-The transcript suggests that making mistakes during practice is beneficial as it helps in remembering and correcting them in the actual exam. It also encourages students to keep practicing with the problems provided in the mock exams and practice materials.
What is the significance of the last day of the session as mentioned in the transcript?
-The last day of the session is significant as it marks the conclusion of a series of preparatory sessions designed to equip students with the necessary knowledge and skills to succeed in their upcoming exam. It serves as a final review and motivational boost.
What motivational advice do the presenters give to students?
-The presenters encourage students to stay positive, ignore the critics, and remember that their worth is not determined by the exam. They emphasize the importance of courage, continuous learning, and the personal growth that comes from academic challenges.
Outlines
📝 Final Session Overview and Exam Details
The speaker, Mark, expresses enthusiasm for the final session of a course and welcomes everyone. He outlines the day's plan, which includes discussing the exam format, providing a mini mock exam, and addressing feedback from participants. The exam details are shared, including dates, format (paper and pencil or digital), and the structure of the test, which consists of multiple-choice and free-response questions. Mark also emphasizes the importance of practicing traditional exam formats and using the provided resources, such as a Dropbox link and QR code.
🧮 Solving an AP Calculus Warm-up Problem
Mark presents a calculus warm-up problem involving the integration of continuous functions g and h. He guides the audience through solving the problem step by step, emphasizing the importance of understanding the bounds of integration and the concept of integrating a constant. The correct answer is identified as option B, and Mark cautions against common mistakes such as not accounting for the bounds correctly. He also touches on the difference between integrating sums/differences and products/quotients.
📚 Mini Mock Exam and Exam Preparation Tips
The session continues with a mini mock exam that is not in the full format but covers various topics. Mark encourages students to pause the video and work through the problems, which include a mix of odd and even numbered questions. He mentions that full annotated solutions will be available and discusses the importance of reviewing previous videos for a comprehensive understanding. The focus is on practicing and understanding calculus problems, with an emphasis on the learning journey rather than just the exam.
🔍 Analyzing a Piecewise Function and Its Derivatives
Mark works through a problem involving a piecewise function f, which is defined differently for x ≤ -1 and x > -1. He discusses the conditions for the function to be continuous and differentiable, which leads to solving for the parameters m and k. The problem involves finding the slope of the tangent line at a specific point, which requires taking the derivative of the function and applying the given conditions.
📉 Evaluating Function Behavior and Critical Points
The paragraph deals with evaluating the behavior of a function h with given select values and critical points. Mark discusses the implications of critical points and inflection points on the function's graph. He eliminates certain answer choices based on the information provided and the properties of the function. The discussion involves understanding the relationship between the function's values, its critical points, and the overall trend of the function within a given interval.
🧵 Differential Equations and Slope Fields
Mark explores the concept of slope fields for differential equations. He examines a given differential equation and identifies the slope field that corresponds to it. The process involves analyzing the equation's zeros and the behavior of the slope function. Mark also discusses how to confirm the correct slope field by checking the behavior of the function, such as its increasing and decreasing intervals.
📈 Deriving the Graph of a Function from Its Derivative
In this part, Mark is given the graph of the derivative of a function g and must determine which of the provided graphs could represent the function g itself. He analyzes the behavior of g' to infer the behavior of g, considering aspects such as increasing and decreasing intervals, as well as concavity. Mark uses the information to eliminate impossible options and identify the correct graph for g.
🟡 The Yellow Sheet: A Custom Formula Sheet for Study
Mark introduces the concept of the 'yellow sheet,' a personalized formula sheet for studying. He emphasizes the importance of creating one's own sheet to review necessary formulas for the AP Calculus exam. Although the yellow sheet cannot be used during the exam, it serves as a valuable study tool. Mark also humorously mentions that the sheet could be used for constant review, even in unconventional places like the bathroom mirror.
🔢 Solving a Differential Equation and Estimating with Tangent Lines
Mark tackles a differential equation problem, providing a step-by-step solution. He starts by finding the equation of the tangent line to a function f at x = 2 and then uses it to approximate the value of f at x = 1.9. The process involves understanding the concept of derivatives as slopes and applying the tangent line equation. Mark also discusses the implications of the second derivative on the accuracy of the approximation.
📉 Determining Overestimates and Underestimates Using Concavity
The paragraph deals with the analysis of overestimates and underestimates in the context of calculus problems. Mark calculates the second derivative of a function and uses its sign to determine whether an approximation is an overestimate or an underestimate. He explains the concept of concavity and how it relates to the shape of the function's graph, providing a clear understanding of why the approximation is either too high or too low.
🧮 Completing the Differential Equation and Final Thoughts
Mark completes the solution to the given differential equation, applying algebraic techniques to isolate y and express it as a function of x. He emphasizes the importance of simplifying the equation according to the problem's requirements. Mark also reflects on the progress students have made in their calculus journey, highlighting their improved algebraic skills and the challenges they've overcome. He encourages students to continue challenging themselves and helping others.
🎓 Final Encouragement and Closing Remarks
In the final paragraph, Mark and his co-host share words of encouragement and advice for students preparing for exams. They stress the importance of viewing tests as a measure of knowledge on a specific day, rather than a measure of self-worth. The hosts provide takeaways that emphasize the students' growth as mathematicians and the value of making mistakes as part of the learning process. They also mention the availability of answer keys and additional practice circuits for continued study and improvement.
Mindmap
Keywords
💡Integral
💡Derivative
💡Differential Equation
💡Tangent Line
💡Concavity
💡Critical Points
💡Multiple Choice
💡Free Response
💡Exam Format
💡Practice Problems
💡Conceptual Understanding
Highlights
The last session begins with an energized and welcoming tone, setting the stage for the final day of the course.
A mini mock exam is introduced, which includes a variety of problems selected from the two-week course.
The exam format is discussed in detail, with specific dates and types of exams (paper and pencil, digital) outlined.
The presenter emphasizes the importance of understanding the exam structure, including the timing and use of calculators.
A common mistake is highlighted regarding the addition of constants in integral calculus, providing a valuable learning opportunity.
The concept of concavity in functions is explained, with an example used to illustrate how to determine the shape of a function from its derivatives.
The presenter provides a step-by-step solution to a differential equation, emphasizing the importance of following instructions and understanding the problem.
The use of a tangent line to approximate function values is demonstrated, showcasing the application of derivatives in function analysis.
The impact of the second derivative on approximations is discussed, with the presenter showing how to determine if an approximation is an over- or underestimation.
A motivational message is shared, encouraging students to embrace challenges and to view tests as opportunities for growth rather than measures of self-worth.
The presenter shares a personal quote about courage, emphasizing its importance alongside honor as a mental quality.
The importance of making mistakes and learning from them is stressed, as it is part of the process of getting better at calculus.
The presenter advises students to keep their pencil moving and stay engaged with the material to avoid getting stuck.
A reminder is given about the availability of answer keys and additional practice circuits for further study and preparation.
The session concludes with well wishes for the students' exams and future academic endeavors, highlighting the supportive and encouraging nature of the course.
The presenter encourages students to provide feedback, showing the interactive and adaptive nature of the course to student needs.
A final reminder is given about the resources available through Google Drive, ensuring students have access to study materials post-session.
Transcripts
Browse More Related Video

2022 Live Review 1 | AP Calculus AB | Practicing with Derivatives & Chain Rule
AP Calc AB & BC Practice MC Review Problems #1

Zoom session Last Minute AP Calculus Exam Review (May 11, 2020)
AP Calculus Practice Exam Part 1 (MC #1-10)

AP Calculus: EVERYTHING YOU NEED TO KNOW

2021 Live Review 8 | AP Physics C: Mechanics | Understanding Resistive Forces
5.0 / 5 (0 votes)
Thanks for rating: