12 - What are Vectors and Scalars?
TLDRThis lesson introduces the fundamental concepts of scalars and vectors in physics. Scalars are quantities with only magnitude, such as temperature and mass, without direction. Vectors, on the other hand, have both magnitude and direction, like velocity and force. The distinction between scalars and vectors is crucial for understanding physical phenomena, as vectors account for directional influence, which scalars do not. The lesson also explains how to represent these quantities visually with arrows and how they will be transitioned into equations for problem-solving in physics.
Takeaways
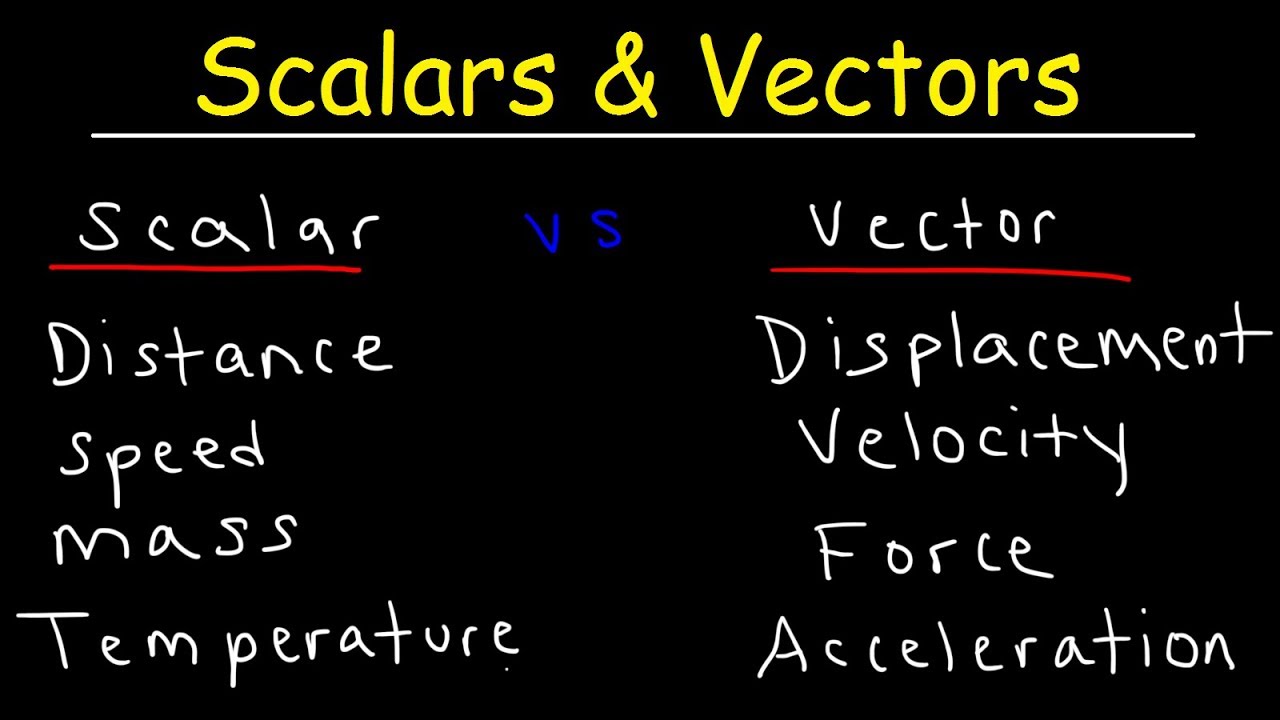
- Scalars are quantities with only magnitude and no direction, such as temperature, pressure, and mass.
- Vectors have both magnitude and direction, like velocity, force, and acceleration.
- Understanding the difference between scalars and vectors is crucial in physics, as they represent different types of physical quantities.
- Examples of scalars include temperature (27°C), pressure (34 N/m²), and mass (0.05 kg).
- Examples of vectors include velocity (34 m/s), force (negative 37 N), and acceleration (positive or negative indicating speeding up or slowing down).
- In textbooks, scalars are often represented with regular variables (e.g., T for temperature, P for pressure), while vectors have an arrow or other notation above them (e.g., ⃗V for velocity).
- Graphical representation of vectors uses arrows, where the length indicates magnitude and the direction indicates the direction of the vector.
- In physics, understanding and working with both scalars and vectors is essential for problem-solving and comprehension of physical concepts.
- Electric and magnetic fields are examples of vector fields, with strength and direction specified at every point in space.
- The transition from graphical representations to equations is important in physics education, as it helps move from visualization to practical problem-solving.
Q & A
What is the main topic of the lesson?
-The main topic of the lesson is understanding the difference between scalars and vectors, and why they are both important in physics.
What is a scalar quantity?
-A scalar quantity is a measurement that only has magnitude and no direction information. Examples of scalars include temperature, pressure, time, volume, speed, and mass.
How are scalars represented in physics?
-Scalars are represented using regular variables without any special symbols. For example, temperature might be denoted as T, pressure as P, and mass as M.
What is a vector quantity?
-A vector quantity is a quantity that has both magnitude and direction. Examples of vectors include velocity, force, and acceleration.
How are vectors represented in physics?
-Vectors are represented with an arrow above the variable symbol to indicate that they have both magnitude and direction. The length of the arrow represents the magnitude, and the direction of the arrow indicates the direction of the vector.
What is the difference between speed and velocity?
-Speed is a scalar quantity that only describes how fast an object is moving, while velocity is a vector quantity that describes both how fast an object is moving and the direction of its motion.
What are some examples of vector fields?
-Examples of vector fields include electric fields, which emanate from charged objects like protons, and magnetic fields, which are associated with magnets and other magnetic materials.
How do we visually represent the magnitude of vectors?
-The magnitude of vectors is visually represented by the length of the arrow. A longer arrow indicates a greater magnitude, while a shorter arrow indicates a lesser magnitude.
Why is it important to understand the difference between scalars and vectors?
-Understanding the difference between scalars and vectors is important because they represent different types of physical quantities. Scalars only have magnitude, while vectors have both magnitude and direction. This distinction is crucial for accurately describing and solving physical problems.
How will the lesson proceed in teaching about vectors?
-The lesson will start by discussing scalars and their properties, then move on to vectors, their definitions, and examples. It will also cover how to graphically represent vectors and eventually transition to working with vectors through equations without the need for graphical representations.
What is the significance of the direction component in vector quantities?
-The direction component in vector quantities is significant because it provides information about the orientation of the physical quantity in space. This is essential for accurately describing motion, force, and other physical phenomena that involve directionality.
Outlines
📚 Introduction to Scalars and Vectors
This paragraph introduces the fundamental concepts of scalars and vectors in physics. It explains that these are essential topics for students new to physics and highlights the difference between the two. Scalars are described as quantities with only magnitude, such as temperature, pressure, and mass, without any directional component. The explanation emphasizes that scalars are familiar concepts, even though students may not have used the term before. The paragraph sets the stage for a deeper exploration of vectors and scalars in the following sections.
🔍 Understanding Scalars in Detail
This paragraph delves deeper into the concept of scalars, emphasizing their nature as quantities with magnitude but no direction. Examples of scalars such as temperature, pressure, time, volume, and mass are provided to illustrate the concept. The explanation clarifies that scalars are not associated with any particular direction and can be represented by regular variables. The paragraph also discusses how scalars are represented in textbooks, usually without any special notation, to distinguish them from vector quantities.
🚀 Exploring the Concept of Vectors
This paragraph introduces vectors as quantities that have both magnitude and direction, contrasting them with scalars. It provides examples of vector quantities, such as velocity, force, and acceleration, and explains how their directional component is crucial. The paragraph also touches on other vector quantities like magnetic and electric fields, which are important in advanced physics. The explanation includes a discussion on how vectors are visually represented with arrows to indicate both magnitude and direction, setting the foundation for further exploration of vector operations in physics.
📈 Visual Representation and Equations of Vectors
This paragraph focuses on the visual representation of vectors using arrows and how they are written in equations. It explains the convention of using an arrow above a variable to denote a vector quantity and how the length of the arrow represents magnitude while the direction of the arrow indicates direction. The paragraph also addresses the variations in notation found in different textbooks, such as the use of a bar or bold text to represent vectors. It concludes by noting that while graphical representations are useful for visualization, the actual problem-solving in physics involves writing and manipulating equations without the need for drawing arrows.
Mindmap
Keywords
💡Scalars
💡Vectors
💡Magnitude
💡Direction
💡Temperature
💡Pressure
💡Volume
💡Speed
💡Mass
💡Acceleration
💡Force
Highlights
Scalars and vectors are fundamental concepts in physics, distinguishing between quantities based on whether they possess only magnitude or both magnitude and direction.
Scalars are quantities with only magnitude, such as temperature, pressure, time, volume, speed, and mass, lacking any directional component.
Vectors, in contrast to scalars, include both magnitude and direction, such as velocity, force, and acceleration, making them more complex and essential in describing physical phenomena.
The concept of scalars and vectors is not intuitively understood without formal education in physics, making it a crucial topic for beginners to grasp.
Understanding scalars and vectors is vital for comprehending further advanced topics in physics, as they form the basis for many physical descriptions and equations.
Examples of scalars include temperature, which is a measure of heat, and mass, which quantifies the amount of matter in an object, both without directional attributes.
Examples of vectors include velocity, which describes the speed and direction of an object's motion, and force, which is characterized by its push or pull along a specific direction.
In physics, scalars are represented by regular variables without any special notation, whereas vectors are denoted with an arrow above the variable to indicate their directional nature.
The representation of vectors graphically as arrows helps in visualizing their magnitude and direction, which is crucial for understanding their physical implications.
While graphical representation of vectors is useful for visualization, the actual problem-solving in physics often involves writing equations without the graphical component.
The transition from using graphical representations to purely algebraic equations is similar to learning mathematical concepts, where initial visualization tools are gradually phased out as understanding deepens.
The lecture introduces the concept of electric and magnetic fields as vector quantities, which are invisible but crucial for understanding interactions between charged particles and magnets.
The electric field around a positive charge, like a proton, is represented by vector field lines emanating from the charge, indicating both the strength and direction of the field.
Magnetic fields, similar to electric fields, are vector quantities, with the direction of the field lines indicating the direction in which the magnetic force would act.
The lecture emphasizes the importance of distinguishing between scalar and vector quantities, as this understanding is essential for solving problems and comprehending the physical world.
The concept of scalars and vectors is not only applicable in physics but also has practical applications in various fields of science, engineering, and technology.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: