What Are Vectors and Scalars? | Physics in Motion
TLDRIn this engaging segment of 'Physics in Motion,' the Marietta High School marching band illustrates the fundamental concepts of vectors and scalars in physics. Scalars, quantities defined solely by magnitude like speed, temperature, and weight, are introduced first. The band then demonstrates vectors, which include both magnitude and direction, using their movement to show how velocity is a vector quantity. The video continues by explaining how to add vectors using diagrams, resulting in a resultant vector that encapsulates the combined effect of multiple movements. The marching band's coordinated movements serve as a practical example of vector addition, emphasizing the importance of both magnitude and direction in describing motion. The segment concludes with a reminder of the significance of these concepts in understanding physical phenomena and the assurance that comfort with vectors and scalars is crucial for further study in physics.
Takeaways
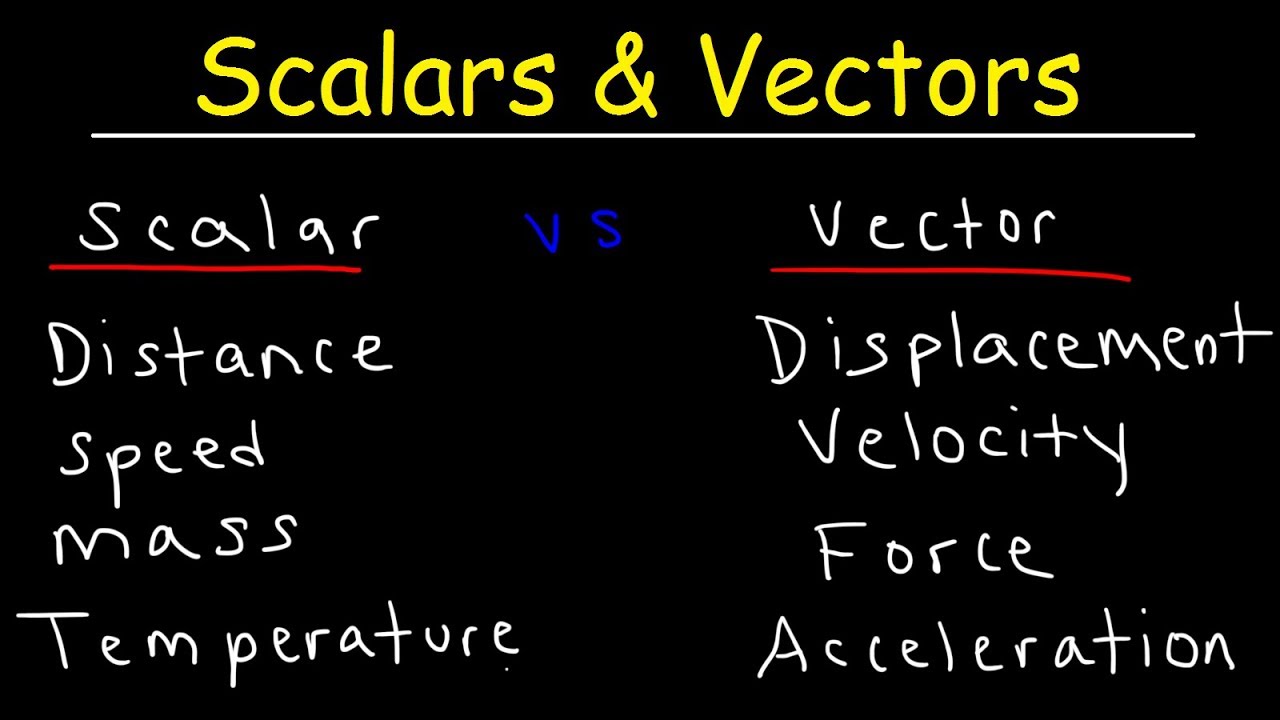
- 🎓 **Scalars and Vectors**: Scalars are quantities described by magnitude alone, like speed or temperature. Vectors include both magnitude and direction, like velocity.
- 📏 **Magnitude**: In scalars, magnitude refers to the quantity's size, such as distance, strength, or amount.
- 🕰️ **Examples of Scalars**: Speed of the marching band (1.5 m/s), temperature (85 degrees), and the weight of a tuba (12 kg) are all scalar quantities.
- 🧭 **Direction in Vectors**: Vectors describe motion with both speed and direction, such as the band's velocity heading west at 1.5 m/s.
- 📍 **Importance of Direction**: Understanding direction is crucial in physics for describing movement, as seen with the use of maps and navigation.
- 🔍 **Vector Diagrams**: Adding vectors is visualized through diagrams, where the resultant vector is drawn from the tail of the first vector to the tip of the last.
- 🔗 **Adding Vectors**: The sum of vectors (A + B) is found by placing the tail of vector B at the tip of vector A, resulting in a single vector representing the combined effect.
- 📐 **Pythagorean Theorem**: Used to calculate the magnitude of the resultant when adding vectors at right angles, like north and east movements.
- ↔️ **Order of Vector Addition**: The order in which vectors are added does not affect the final resultant; the outcome is the same regardless of the sequence.
- 🔄 **Vector Translation**: Vectors can be moved parallel to their original position without changing their magnitude or direction, which aids in calculations with multiple vectors.
- 📊 **Coordinate Plane**: Demonstrated using a graph with X and Y axes, where groups move to form vectors that, when combined, yield the same resultant regardless of the path taken.
- 🔎 **Applications of Vectors**: Vectors are used to represent various physical quantities, including force, indicating both strength and direction.
Q & A
What are the two important terms in physics that describe motion discussed in the script?
-The two important terms in physics that describe motion are vectors and scalars.
What is a scalar quantity, and how does it differ from a vector quantity?
-A scalar quantity is described by magnitude alone, such as speed, temperature, or mass. It differs from a vector quantity, which includes both magnitude and direction, providing a more comprehensive description of motion.
What is the speed of the marching band as mentioned in the script?
-The marching band is moving at a speed of approximately 1.5 meters per second.
How does the direction factor into the description of motion when using vector quantities?
-Direction is a critical component of vector quantities. It tells us not only how fast something is moving but also the path or orientation of the motion.
What is the significance of adding vectors together in physics?
-Adding vectors together allows us to determine the resultant or net effect of multiple forces or motions acting on an object. This is essential for understanding complex movements and the combined effects of different influences.
How can you visually represent the addition of vectors?
-Vectors can be visually represented and added using a diagram where each vector is a line with an arrow indicating direction. To add them, place the tail of the second vector at the tip of the first, and the resultant vector is drawn from the tail of the first to the tip of the second vector.
What is the Pythagorean Theorem's role in calculating the resultant of added vectors?
-The Pythagorean Theorem is used to calculate the magnitude of the resultant vector when vectors are added together, especially in two-dimensional space. It helps find the length of the diagonal in a right-angled triangle formed by the vector addition.
Why is it important to understand that the order of vector addition does not affect the resultant?
-The order of vector addition does not affect the resultant because vector operations are commutative. This means that regardless of the sequence in which vectors are added, the final position or state of the object remains the same.
How can the concept of vectors be applied to real-world scenarios like navigation?
-Vectors are crucial in navigation as they provide both the distance to be traveled and the direction in which to travel. For example, a mapping application would use vector information to guide a user, combining both magnitude (how far) and direction (which way).
What is the term used to describe the final vector after adding multiple vectors together?
-The term used to describe the final vector after adding multiple vectors is the 'resultant vector'. It represents the net displacement or effect of all the individual vectors.
How does the concept of vectors relate to the strength and direction of a force?
-Vectors can represent force by indicating both the strength (magnitude) of the force and the direction in which it acts. This allows for a more detailed analysis of how forces interact with objects, including their effects on motion.
What additional resources are available for further learning about the concepts discussed in the script?
-For more practice problems, lab activities, and note-taking guides, one can refer to the 'Physics in Motion' Toolkit mentioned in the script.
Outlines
🎵 Understanding Scalars and Vectors in Physics
This paragraph introduces the concepts of scalar and vector quantities in physics. Scalars are described by magnitude alone, such as speed (1.5 meters per second), temperature (85 degrees), mass (12 kilograms), time (ten seconds or one hour), and volume (a cup or a quart). Vectors, on the other hand, provide information about both magnitude and direction. An example given is the velocity of the marching band, which is moving at 1.5 meters per second towards the west. The importance of vectors is highlighted by their necessity in describing more complex motions, such as when direction is involved. The concept of adding vectors is introduced, explaining that the sum of two vectors, A and B, is represented by a line from the tail of A to the tip of B, resulting in a resultant vector. The Pythagorean Theorem is used to calculate the magnitude of the resultant when adding vectors in orthogonal directions, such as north and east, resulting in a displacement of 15.6 kilometers northeast. The paragraph concludes with a demonstration of how the resultant vector remains the same regardless of the order in which vectors are added.
🎶 Demonstrating Vector Addition and Resultants
The second paragraph demonstrates vector addition with a practical example involving the marching band. Two groups from the band are instructed to move in different directions at the same speed, creating two separate vectors. The first group moves 20 meters along the X-axis, while the second moves 30 meters along the Y-axis, both at a speed of 1.5 meters per second. Afterward, the groups reverse their movements, with the second group moving along the X-axis and the first along the Y-axis. The resultant of both movements is shown to be the same, illustrating that vector addition is commutative. The paragraph emphasizes the utility of vectors in depicting displacement, force, and direction. It concludes with an encouragement to become comfortable with these concepts as they are fundamental to the study of physics. The 'Physics in Motion' Toolkit is promoted as a resource for further practice and understanding.
Mindmap
Keywords
💡Vectors
💡Scalars
💡Magnitude
💡Direction
💡Velocity
💡Resultant
💡Pythagorean Theorem
💡Displacement
💡Coordinate Plane
💡Addition of Vectors
💡Physics in Motion
Highlights
Scalars and vectors are two important terms in physics that describe motion.
Scalars are quantities described by magnitude alone, like speed, temperature, mass, time, and volume.
Vectors express both magnitude and direction, providing more information than scalars.
Velocity is an example of a vector quantity, describing both speed and direction of motion.
Many concepts in physics, like north, south, left, right, up, down, have both a direction and a magnitude.
Vector information is crucial for navigation, as it tells us both the distance to travel and the direction to turn.
To add vectors, place the tail of the second vector at the tip of the first to find the resultant vector.
The Pythagorean Theorem can be used to calculate the magnitude of the resultant when adding vectors.
The order in which vectors are added does not affect the final resultant vector.
Vectors can be moved parallel to their original position without changing their magnitude and direction.
A coordinate plane with X and Y axes can be used to visualize and add vectors.
Vectors can represent other physical quantities like force, showing both strength and direction.
Understanding vectors and scalars is essential for many concepts in physics.
The length of a vector on a diagram represents the displacement of an object.
The marching band demonstration visually shows how vectors are added to find the resultant vector.
Vectors provide valuable information to understand an object's speed, direction, and overall motion.
The Physics in Motion Toolkit offers additional resources for practicing vector and scalar concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: