Fall 2023 MNC: Take it to the limit with numerical, graphical, algebraic representations
TLDRIn this engaging calculus session, Curtis Brown and his guests explore various limit problems, emphasizing the importance of understanding the behavior of functions as they approach certain values. They delve into numerical, graphical, and algebraic approaches to estimating limits, highlighting the significance of one-sided limits and the application of limit properties. The session also incorporates technology, specifically the TI-84 calculator, to illustrate and analyze functions and limits, providing a comprehensive understanding of the concepts discussed.
Takeaways
- 📅 The event is a Monday Night Calculus themed session, hosted by Curtis Brown, with Steve Kokoska and Tom Dick as guests.
- 🎥 The session focuses on exploring limits using numerical, graphical, and algebraic approaches, emphasizing interaction and problem-solving.
- 📊 Importance of tables and graphs in estimating limits is discussed, with the presenter highlighting that these are not proofs but helpful for understanding limit behavior.
- 🤔 The session encourages participants to ask questions and engage with the material, with the chat being a tool for posing questions and receiving help.
- 📚 The educators, Steve and Tom, use technology, specifically Mathematica and TI-84, to illustrate and analyze various calculus problems related to limits.
- 📝 Curtis Brown presents a problem involving the limit of a product of functions, highlighting the need to check if the individual limits exist before multiplying them.
- 🔢 Steve Kokoska discusses a limit involving the composition of functions, emphasizing the importance of one-sided limits when the inner function's limit does not exist.
- 📈 Tom Dick demonstrates how to use the TI-84 calculator to graph functions and analyze limits, showcasing the benefits of technology in visualizing mathematical concepts.
- 💡 The session concludes with a series of overtime problems, which are additional challenging questions related to limits, encouraging further exploration and learning.
- 🎓 The presenters remind viewers about the availability of teacher professional development hours and the option to request a certificate for participation.
- 🔗 Curtis provides his email for teachers to request additional resources and solutions, promoting continued learning and engagement with the material.
Q & A
What is the main topic of the transcript?
-The main topic of the transcript is a discussion and analysis of various calculus problems related to limits, focusing on numerical, graphical, and algebraic approaches.
Who are the speakers in the transcript?
-The speakers in the transcript are Curtis Brown, Steve Kokoska, and Tom Dick.
What is the significance of the consistent schedule mentioned in the transcript?
-The consistent schedule, with all events happening on Monday nights, allows for a more predictable and streamlined approach to the calculus events and helps participants better plan their participation.
How does Curtis Brown approach the problem involving the limit of x squared times the log of x as x approaches zero from the right?
-Curtis Brown approaches the problem by considering the behavior of the individual functions (x squared and log of x) as x approaches zero from the right, then uses a table of values to estimate the limit and graphs to visually support his analysis.
What technology is mentioned in the transcript for solving calculus problems?
-The technology mentioned in the transcript for solving calculus problems is Mathematica, as well as the TI-84 calculator.
What is the main takeaway from the discussion about the limit of the composition function involving the log of the sine of x?
-The main takeaway is that the limit can be estimated by looking at a table of values and analyzing the graph of the function, which suggests that the limit goes to negative infinity as x approaches zero from the right.
How does the speaker suggest students engage with the material?
-The speaker suggests that students should actively participate by asking questions, calling out their work, and interacting with the material to better understand the concepts being discussed.
What is the significance of the vertical asymptote in the function G discussed in the overtime problems?
-The vertical asymptotes in the function G are significant because they indicate values of x where the function is not defined and the graph of the function has a sharp break or 'jump' in the graph. These points are important for understanding the domain and behavior of the function.
What is the main challenge with the limit problem involving 1 over x plus the log of x as x approaches zero from the right?
-The main challenge with this limit problem is determining whether the two competing infinities (positive infinity from 1 over x and negative infinity from the log of x) cancel each other out or if one dominates, resulting in the limit either being zero or positive infinity.
How does the speaker suggest using one-sided limits to solve certain problems?
-The speaker suggests using one-sided limits to solve problems when the intuitive property of limits does not apply, such as when one of the limits in a product or a composition does not exist. By examining the left and right one-sided limits, one can gain insight into the behavior of the function at the point of interest.
What is the main purpose of the overtime problems discussed in the transcript?
-The main purpose of the overtime problems is to provide additional, challenging practice for students and teachers, encouraging deeper exploration of calculus concepts and problem-solving strategies beyond the core content of the session.
Outlines
📚 Introduction to Monday Night Calculus
The video begins with Curtis Brown enthusiastically welcoming viewers to another season of Monday Night Calculus. He is excited about the consistent schedule this season, with all events happening on Monday nights. Curtis introduces his colleagues, Steve Kokoska and Tom Dick, and encourages students to ask questions and seek extra credit opportunities. The session aims to be interactive, with a focus on limits using numerical, graphical, and algebraic approaches. Curtis highlights the importance of the chat for questions and acknowledges that the upcoming discussions are not proofs but rather estimations of limits.
📈 Analyzing Limits with Tables and Graphs
Curtis and his team delve into the specifics of estimating limits using tables of values and graphical representations. They discuss the behavior of functions as x approaches zero, using the example of x squared times the log of x. Curtis shares his thought process as he examines the function, considering the competition between x squared approaching zero and the log function approaching negative infinity. The use of technology, specifically Mathematica, is introduced to illustrate the function's graph and how it crosses the x-axis. The conversation transitions to a new technology platform, with some initial technical difficulties acknowledged.
🔢 Investigating Limits of Trigonometric Functions
The discussion shifts to the limit of the composition function, log of sine of x, as x approaches zero from the right. Curtis uses trigonometry to predict the behavior of the sine function and expects the composition to approach negative infinity. This expectation is confirmed through a table of values and a graph. Open questions are posed for further exploration, such as the function's behavior as x increases without bound and the possibility of vertical asymptotes. Curtis then attempts another limit problem, involving a difference of functions, and shares his reasoning process before looking at the table and graph.
💻 Utilizing Technology for Limit Analysis
Tom takes over to demonstrate the use of a TI-84 calculator to analyze the limits discussed by Steve. He walks through the process of entering functions, graphing, and using the table feature to investigate the behavior of function values as x approaches zero. Tom emphasizes that these are not proofs but rather estimations. He also addresses a chat question about changing a subtraction sign to a plus sign and how to approach that on a calculator. The demonstration includes adjusting the table settings for a better understanding of limit behavior and using the ask function for more precise inputs.
📊 Deep Dive into Function Behavior near Zero
Tom continues to explore the behavior of functions near zero using the calculator. He examines the function x squared times the natural log of x and uses the table feature to find function values as x approaches zero from the right. The values indicate that the function is approaching zero. Tom also discusses the limitations of the table feature and how to work around them by manually inputting values. He then moves on to another function, the natural log of the sine of x, and examines its behavior near zero, noting the slow decrease towards zero and the potential for a vertical asymptote.
🤔 Challenging Limit Problems and Solutions
Curtis and Tom present a series of challenging limit problems, encouraging viewers to think critically about the properties of limits. They discuss the existence of limits for piecewise functions and the conditions under which limit properties can be applied. Curtis presents a problem involving the product of two functions as x approaches one and emphasizes the need to examine one-sided limits when the intuitive approach fails. The problem is solved by considering the left and right limits separately, leading to the conclusion that the overall limit exists and is zero. Tom then explores another limit involving a composition of functions, highlighting the importance of function continuity.
🧠 Solving Limits Algebraically and Graphically
Curtis and Tom tackle a series of limit problems using both algebraic and graphical methods. They discuss the importance of notational fluency and carrying the limit expression through the solution process. Curtis solves a limit problem algebraically by rationalizing the denominator and finds that the limit is two-thirds. Tom then uses the TI-84 to graph the function and verify the solution. They also discuss the domain of the function and the presence of a vertical asymptote at x equals minus one. Tom demonstrates how to enter piecewise functions into the calculator and explores the combination of two functions to analyze their product and limit behavior as x approaches one.
🎓 Closing Remarks and Future Learning Opportunities
Curtis concludes the session by thanking everyone for their participation and expressing his gratitude for the engagement with the overtime problems. He encourages teachers to request professional development certificates via email and reminds them to check for the student document and solutions to be posted in the coming weeks. Curtis also mentions that the next session will be held on October 2nd and encourages students and teachers to join for further learning and discussion.
Mindmap
Keywords
💡Calculus
💡Limits
💡Functions
💡Graphs
💡Logarithm
💡Technology
💡Algebraic
💡Numerical
💡One-sided limits
💡Vertical asymptote
Highlights
Introduction to Monday Night Calculus, a themed calculus event
Curtis Brown and Steve Kokoska discussing consistent scheduling for the event
Engagement with the audience through chat for questions and interactions
Use of technology, specifically Mathematica, to visualize and solve calculus problems
Exploration of the limit as X approaches zero using a table of values and graphing
Discussion on the limit of the product of two functions and their competing behaviors
Investigation of the limit of a composition function, log of sine of X as X approaches zero
Use of the TI-84 calculator to graph and analyze functions
Explaining how the domain of a function affects the location of vertical asymptotes
Demonstration of piecewise functions on a calculator
Analysis of a limit involving a rational function multiplied by a logarithmic function
Discussion on the limit properties and their application in solving complex problems
Investigation of a limit involving the subtraction of two functions
Exploration of the behavior of functions as they approach undefined points
Discussion on the importance of notation fluency and carrying the limit expression through
Introduction to overtime problems and their significance in understanding limit properties
Analysis of a limit involving a sum of two functions
Closing remarks on the session, including the provision of teacher professional development certificates
Transcripts
Browse More Related Video

Monday Night Calculus: Limits at Infinity and Infinite Limits
Fall 2023 MNC: Trick or treat - Some important theorems in differential calculus

Fall 2023 MNC: Slice thin and add - Riemann sums and definite integrals
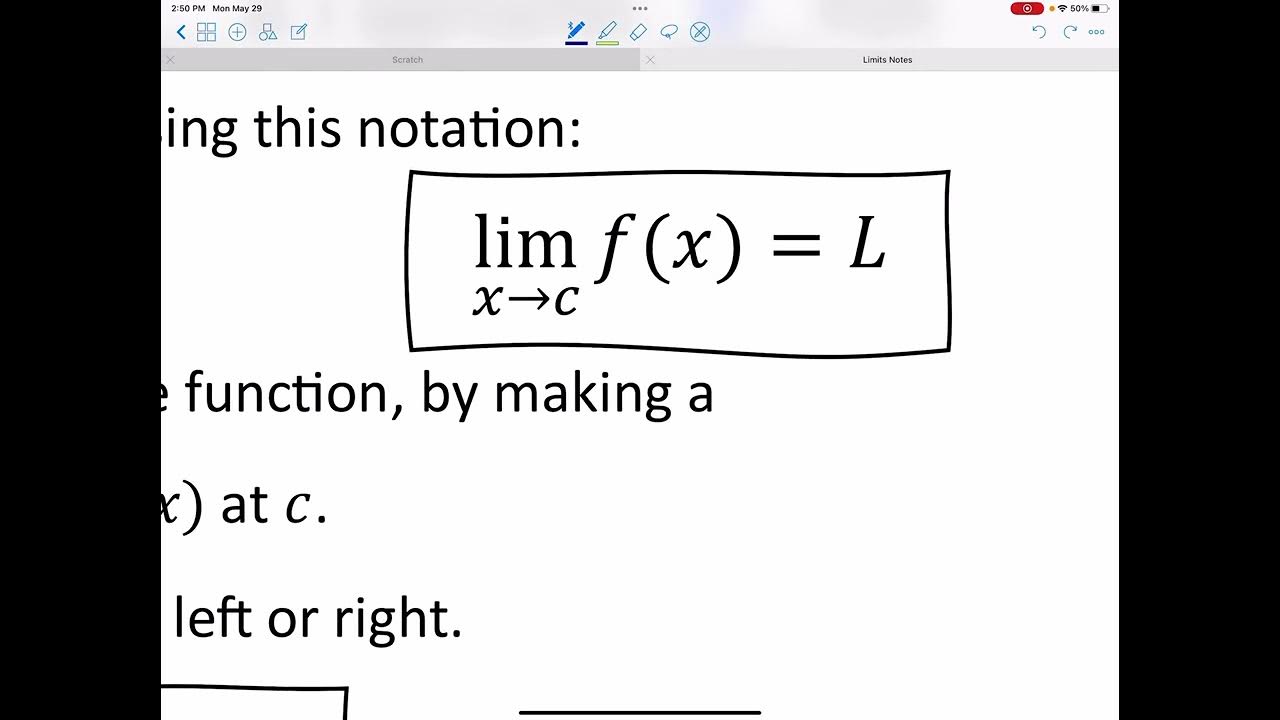
Limits

Limits via Graphs!

2021 Live Review 3 | AP Calculus AB | How to Find Limits & Continuity
5.0 / 5 (0 votes)
Thanks for rating: