Monday Night Calculus: Limits at Infinity and Infinite Limits
TLDRIn this engaging calculus session, Steve and Tom explore the concepts of infinite limits and limits at infinity. They delve into the behavior of functions as x approaches specific values, illustrating the ideas with graphs and numerical examples. The session also covers vertical asymptotes, horizontal asymptotes, and the impact of function composition on limits. The presenters provide insightful explanations, address audience questions, and offer technology tips for examining limits, rounding off with challenging problems to ponder.
Takeaways
- 📚 The session focused on understanding and working with calculus problems, particularly limits at infinity and infinite limits.
- 🌟 Curtis, Steve, and Tom hosted the session, aiming to provide a comprehensive understanding of the topics through problem sets and technology tips.
- 📈 The importance of checking both one-sided limits was emphasized, as the behavior from the left and right can differ, leading to different outcomes.
- 🔢 The session discussed how to handle cases where a function's limit does not exist by using the concept of infinity as a descriptive tool rather than a numerical value.
- 📊 The use of graphs and tables was highlighted as a valuable tool for visually understanding the behavior of functions as they approach certain values, including infinity.
- 🤔 The concept of vertical asymptotes was explored, with examples provided to illustrate how to identify them and understand their role in the function's behavior.
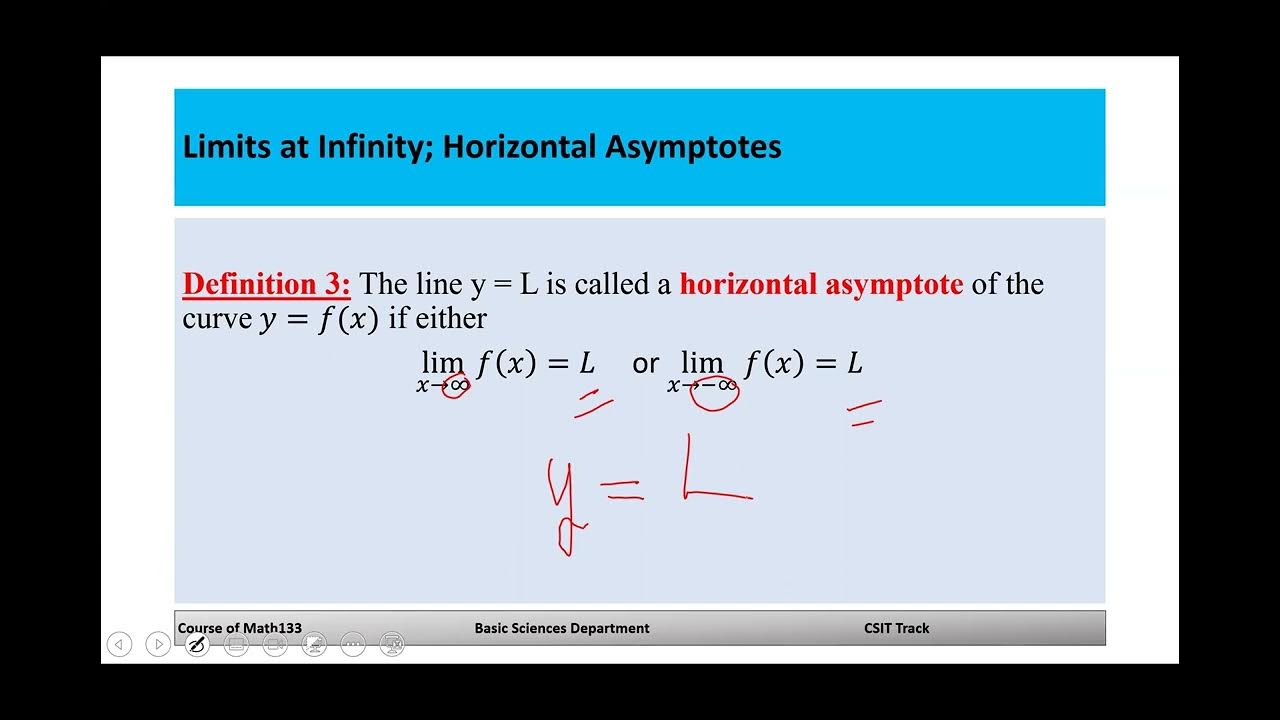
- 📐 The role of horizontal asymptotes in analyzing the long-term behavior of functions was discussed, including how to determine their existence and their impact on the function's graph.
- 💡 The session touched on the use of technology, such as graphing calculators and software like Mathematica, to aid in visualizing and solving calculus problems.
- 📝 The importance of epsilon-delta arguments and formal definitions in understanding the precise nature of limits was mentioned, particularly for advanced calculus students.
- 🚫 The session reminded viewers to be cautious with compositions of functions, as the domain and continuity of the individual functions can significantly affect the limit of the composite function.
- 🔄 The session concluded with a discussion on the different possible outcomes for the limit of composite functions based on the choice of functions and their continuity.
Q & A
What is the main topic of the calculus session presented in the transcript?
-The main topic of the calculus session is 'Infinite Limits and Limits at Infinity', focusing on understanding the behavior of certain functions as the variable approaches specific values or infinity.
How does the host, Curtis Brown, introduce the session and the instructors, Steve Kakoska and Tom Dick?
-Curtis Brown introduces the session by expressing excitement that the audience has chosen to join the Monday night calculus session. He introduces Steve Kakoska and Tom Dick as the instructors who have prepared problem sets and technology tips for the session.
What is the significance of the function 1/(x - 2)^2 in the discussion about limits?
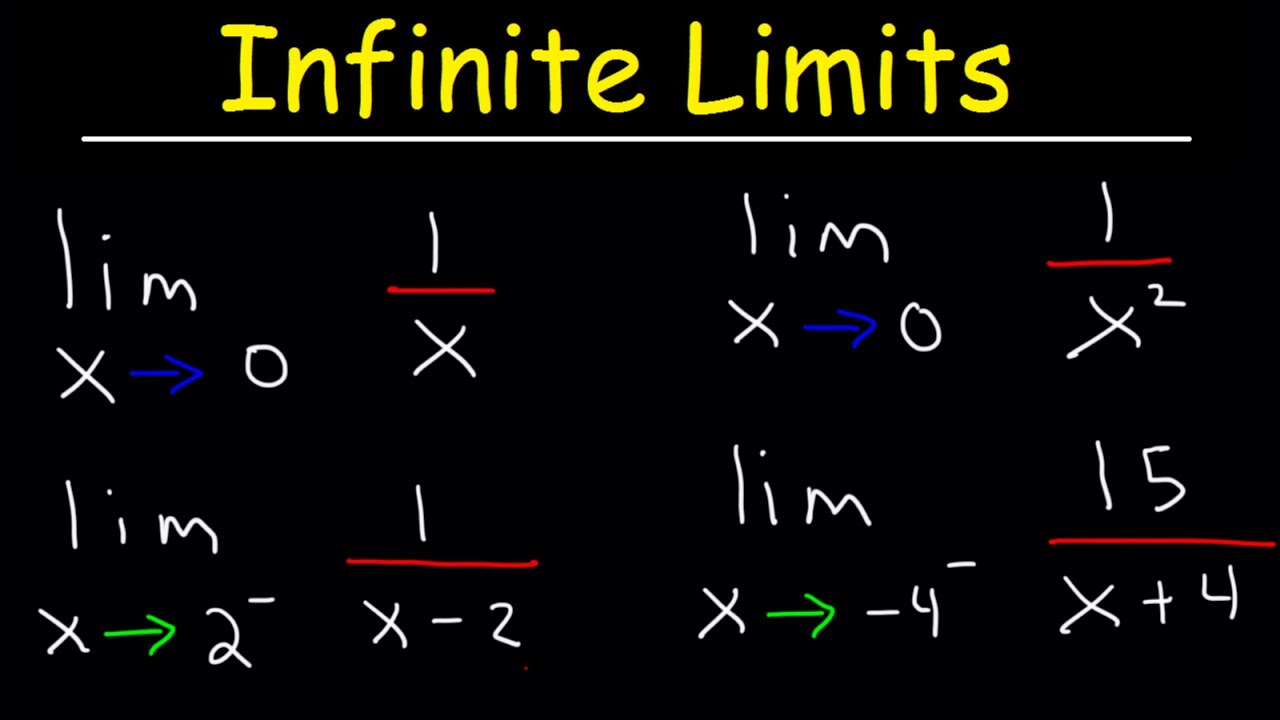
-The function 1/(x - 2)^2 is used as an example to illustrate a case where a limit does not exist. As x approaches 2, the function's value increases without bound, which is indicated by the notation 'infinity', although infinity is not a number but a symbol used to describe this special kind of behavior.
How does the concept of a vertical asymptote relate to the limits discussed in the session?
-A vertical asymptote is a line on the graph of a function where the function approaches infinity or negative infinity as the variable approaches a certain value. It is related to the limits discussed in the session as it represents a point at which the function is not defined or does not have a limit.
What is the significance of the epsilon-delta definition in the context of limits?
-The epsilon-delta definition provides a strict, mathematical way to define what a limit is. It is mentioned in the transcript as a concept that could be explored for a deeper understanding of the limits, particularly in the context of one-sided limits and vertical asymptotes.
How does the session address the concept of limits at infinity?
-The session addresses limits at infinity by examining functions that approach a certain value or zero as the variable increases or decreases without bound. This is done by looking at numerical and graphical representations of the functions, and by discussing the behavior of these functions at infinity.
What is the role of technology in exploring limits as discussed in the session?
-Technology, as represented by graphing software like Mathematica, is used to visualize the behavior of functions as they approach certain values or infinity. It helps in understanding the concepts of vertical and horizontal asymptotes, and in exploring the limits at infinity.
What is the significance of the horizontal asymptote in the context of the functions discussed in the session?
-A horizontal asymptote is a horizontal line on a graph that a function approaches as the variable increases or decreases without bound. It is significant in the session as it represents the long-term behavior of a function and is used to understand the limit of a function as the variable approaches infinity or negative infinity.
How does the session handle the concept of a limit not existing due to differing behaviors on either side of a point?
-The session explains that if a function behaves differently as it approaches a point from the left and from the right, the limit at that point does not exist. This is demonstrated with the function 3x/(x - 1), which approaches positive infinity from the right and negative infinity from the left as x approaches 1.
What is the significance of the one-sided limits in the context of the session?
-One-sided limits are significant in the session as they provide insight into the behavior of a function as it approaches a certain point from either the left or the right. These limits are used to determine the presence of vertical asymptotes and to understand the behavior of functions at points where the standard limit may not exist.
Outlines
📚 Introduction to Monday Night Calculus
The video begins with an introduction to the Monday Night Calculus session, hosted by Curtis Brown, Steve Kakoska, and Tom Dick. Curtis expresses excitement for the participants joining the session and mentions that Steve and Tom have prepared problem sets for the group. He explains that these sessions will occur every two weeks and that problem sets will be made available for download. Curtis also highlights that solutions will be posted on a bulletin board and that the session will cover topics such as infinite limits and limits at infinity.
🔢 Understanding Limits and Infinite Behavior
The paragraph delves into the concept of limits, particularly focusing on situations where the limit does not exist due to the function's value increasing without bound. The speaker uses the function 1/(x-2)^2 as an example to illustrate this concept. He explains that as x approaches 2, the function's value grows without limit, which is numerically and graphically demonstrated. The speaker emphasizes that while infinity is not a number, it is used as a symbol in calculus to describe the behavior of functions near certain points.
📈 Graphing and Table Analysis of Limits
This section continues the discussion on limits by examining the behavior of functions through graphs and tables of values. The speaker describes observing symmetry in the function and notes that the values of the function can be made arbitrarily large by taking x close to 2. A graph is used to support the observation that the function's value increases without bound as x approaches 2 from both sides. The concept of a vertical asymptote is introduced, with the speaker explaining how it is represented on a graph and how to determine its presence.
🤔 Evaluating Asymptotes and Limit Definitions
The speaker engages the audience with questions and examples to explore the definitions of vertical asymptotes and one-sided limits. Using a function with a 'hole' at x=1, the speaker discusses whether it has a vertical asymptote. The conversation involves the audience, with participants offering insights and answers. The speaker also addresses a question about oscillating functions and clarifies the definition of a vertical asymptote, emphasizing that the graph of the function cannot cross the vertical asymptote.
📊 Analytical and Graphical Exploration of Limits
The speaker presents a detailed analytical approach to determining limits, using the function 3x/(x-1) as an example. He explains how to calculate one-sided limits and what to conclude when the behavior on either side of a point is different. The speaker also discusses the concept of a function approaching positive or negative infinity and how to express this mathematically. The conversation then turns to a discussion about necessary conditions for evaluating limits and the importance of understanding function behavior at infinity.
🌐 Limits in Infinity and Infinite Limits
The speaker shifts focus to limits in infinity, examining the behavior of functions as x increases or decreases without bound. Using the function 1/(x^2+1) as an example, the speaker numerically and graphically demonstrates how the function approaches zero as x increases, indicating a horizontal asymptote. The speaker explains the notation and intuitive understanding of limits at infinity and discusses the possibility of a function having different horizontal asymptotes depending on the direction from which the limit is approached.
📝 Analytical Techniques for Finding Limits
The speaker provides a step-by-step analytical method for finding limits of rational functions, emphasizing the importance of these techniques for AP Calculus students. He demonstrates how to divide the numerator and denominator by the highest power of x in the denominator to simplify the expression and find the limit. The speaker also suggests exercises for students to practice finding both horizontal and vertical asymptotes of functions and to understand the different behaviors of functions at infinity.
💡 Limit Evaluations and Technology Aids
The speaker discusses the use of technology in evaluating limits, particularly at infinity. He suggests techniques such as zooming out on graphs and replacing x with 1/x to examine behavior near zero. The speaker also highlights the capabilities of computer algebra systems in evaluating limits and demonstrates this with an example. The conversation includes a problem involving the composition of functions and how to determine limits in such cases, emphasizing the need for caution and understanding of function domains.
🔍 Final Thoughts and Future Sessions
In the concluding paragraph, the hosts wrap up the session by expressing gratitude for the participants' engagement and sharing of expertise. They remind the audience to look for solutions to the problem sets on the posted link and announce the date for the next session. The hosts encourage the audience to send in questions and express eagerness for the continuation of the series, highlighting the value of revisiting calculus concepts.
Mindmap
Keywords
💡Calculus
💡Limits
💡Infinite Limits
💡Technology Tips
💡Asymptotes
💡Functions
💡Composite Functions
💡Continuity
💡Graphing
💡Problem Sets
Highlights
Introduction to Monday Night Calculus with Steve Kakoska and Tom Dick
Discussion on problem sets and technology tips for calculus
Explanation of infinite limits and limits at infinity
Illustration of limit behavior through a special case where a limit does not exist
Use of numerical and graphical methods to investigate limits
Definition and identification of vertical asymptotes
Distinguishing between different types of limits through examples
Discussion on the concept of limits in infinity for functions
Analytical techniques for finding limits of rational functions
Investigation of limits at infinity using technology and graphing
Exploration of horizontal asymptotes and their properties
Challenges and subtleties in evaluating limits of composite functions
Use of computer algebra systems to evaluate limits
Strategies for dealing with limits at infinity in a computational setting
Importance of continuity in functions when evaluating limits of composite functions
Conclusion and wrap-up of the calculus session with future problem sets and topics
Transcripts
Browse More Related Video

AP Calculus AB - 1.14 Connecting Infinite Limits and Vertical Asymptotes

AP Calculus AB - 1.15 Connecting Limits at Infinity and Horizontal Asymptotes
Math 133 Lecture 2 6

Fall 2023 MNC: Take it to the limit with numerical, graphical, algebraic representations
Infinite Limits and Vertical Asymptotes

Calculus AB Homework 1.2 Understanding the Limit
5.0 / 5 (0 votes)
Thanks for rating: