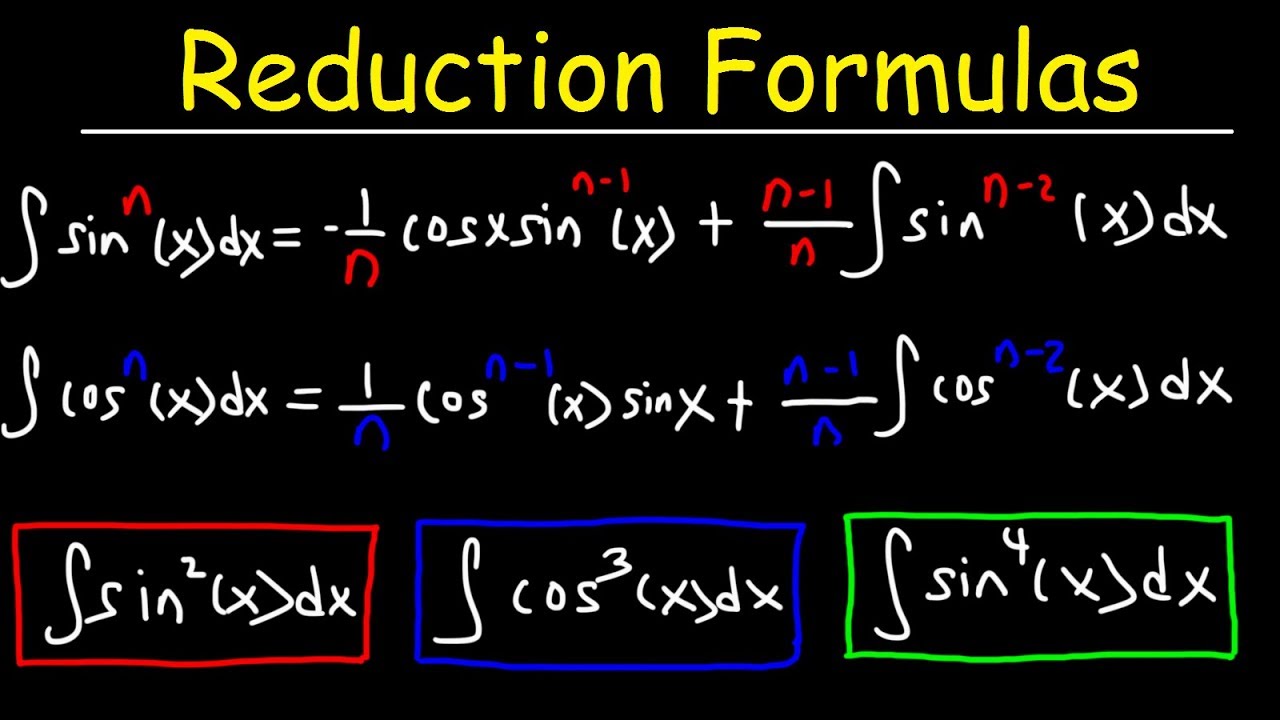
Indefinite Integral

The Organic Chemistry Tutor
22 Dec 201905:14
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a step-by-step walkthrough of finding the indefinite integral of the function x times the cosine squared of x times the tangent of x. The process involves simplifying the expression, utilizing the double angle formula for sine, and applying integration by parts. The final result is derived as negative one quarter times x times the cosine squared of 2x, plus one eighth times the sine of 2x, plus a constant of integration, effectively solving the original calculus problem.

Takeaways
  • πŸ“š The problem involves finding the indefinite integral of a complex function, specifically x * cos^2(x) * tan(x).
  • πŸ” The first step is to simplify the expression by recognizing that tan(x) = sin(x)/cos(x) and cos^2(x) can be reduced by one cos(x).
  • πŸ“ The double angle formula for sine, sin(2x) = 2sin(x)cos(x), is applied to further simplify the integrand to x * sin(2x)/2.
  • 🎯 Integration by parts is the chosen method for solving the integral, with the formula ∫udv = uv - ∫vdu.
  • 🌟 Assigning x as the u variable and sine(2x)dx as dv, we proceed with the integration by parts process.
  • πŸ”„ Derivative of u (x) is 1, and the antiderivative of dv (sine(2x)dx) is -cos(2x)/2 after accounting for the 2x term in its derivative.
  • πŸ“Š Plugging the values back into the integration by parts formula yields an expression involving x and the remaining integral of cosine(2x).
  • 🧩 The constant 1/2 is distributed to both terms resulting from the integration by parts process.
  • 🌈 The antiderivative of cosine(2x) is sine(2x)/2, which is then incorporated into the final expression.
  • πŸ“ The final answer is derived as -1/4 * x * cosine(2x) + 1/8 * sine(2x) + C, where C is the constant of integration.
  • πŸŽ“ The solution provided is for the indefinite integral of the given function, emphasizing the use of trigonometric identities and integration techniques.
Q & A

  • What is the first step in solving the indefinite integral of x*cos^2(x)*tan(x)?

    -The first step is to simplify the expression if possible. In this case, we simplify cos^2(x) to cos(x)*cos(x) and recognize that tan(x) is sin(x)/cos(x).

  • How can we simplify the expression further after identifying the components of x*cos^2(x)*tan(x)?

    -By canceling out one cos(x) term, we are left with the integral of x*sin(x)*cos(x).

  • What is the double angle formula for sine that is used in the script?

    -The double angle formula for sine is sin(2x) = 2*sin(x)*cos(x).

  • How does the script apply the double angle formula to the integral?

    -The script applies the double angle formula by multiplying both sides of sin(2x) = 2*sin(x)*cos(x) by 1/2, resulting in 1/2*sin(2x) = sin(x)*cos(x), and then replacing sin(x)*cos(x) with 1/2*sin(2x) in the integral.

  • What integration technique is used to solve the integral of the expression?

    -Integration by parts is used to solve the integral of the expression.

  • What is the formula for integration by parts?

    -The formula for integration by parts is ∫u dv = u*v - ∫v du.

  • How are the u and dv chosen in the integration by parts process for this problem?

    -In the integration by parts process, u is chosen to be x and dv is chosen to be sin(2x) dx.

  • What are the derivatives and antiderivatives used in the integration by parts formula for this problem?

    -The derivative of u (du) is 1*dx, and the antiderivative of dv (v) is -cos(2x)/2, considering the derivative of 2x is 2.

  • What is the final result of the indefinite integral of x*cos^2(x)*tan(x)?

    -The final result is -1/4*x*cos(2x) + 1/8*sin(2x) + C, where C is the constant of integration.

  • What is the significance of the constant of integration (C) in the final answer?

    -The constant of integration (C) is included in the final answer to account for the arbitrary constant that arises when integrating a function.

  • How does the script demonstrate the process of solving the integral?

    -The script demonstrates the process by first simplifying the expression, applying the double angle formula, and then using integration by parts to find the antiderivative of the resulting expression.

Outlines
00:00
πŸ“š Solving the Indefinite Integral of a Trigonometric Expression

This paragraph walks through the process of finding the indefinite integral of a complex trigonometric expression, specifically ∫x*cos^2(x)*tan(x) dx. It begins by simplifying the expression, using the double angle formula for sine and the definition of tangent. The integral is then reformulated using integration by parts, with x as the u variable and sine(2x) as dv. The paragraph explains the steps to find the antiderivative of sine(2x) and how to apply the integration by parts formula to obtain the final result, which includes the antiderivative of cosine(2x) and a constant of integration.

05:03
πŸŽ“ Final Answer to the Indefinite Integral Problem

This paragraph concludes the video script by summarizing the final answer to the indefinite integral problem presented in the previous paragraph. It reiterates that the integral of the given trigonometric expression is equivalent to -1/4*x*cos(2x) + 1/8*sin(2x) + C, where C represents the constant of integration. This concise summary provides the viewer with a clear and final answer to the problem discussed in the video.

Mindmap
Keywords
πŸ’‘indefinite integral
The indefinite integral is a fundamental concept in calculus that represents the reverse process of differentiation. It is used to find the original function whose derivative is given. In the context of the video, the indefinite integral of the expression involving x, cosine squared x, and tangent x is being sought. The process involves simplifying the expression and applying integration techniques such as integration by parts.
πŸ’‘cosine squared
Cosine squared is a trigonometric expression that represents the cosine function of an angle squared. In the video, cosine squared x is simplified as cosine x times cosine x. This simplification is crucial for further integrating the expression and is part of the process leading to the application of the double angle formula.
πŸ’‘tangent x
Tangent x, often abbreviated as tan x, is a trigonometric function that equals the sine of x divided by the cosine of x. In the video, the term tangent is used to represent the ratio of the sine function to the cosine function, which is later simplified to facilitate the integration process.
πŸ’‘double angle formula
The double angle formula is a trigonometric identity that expresses the sine of twice an angle in terms of the sine and cosine of the angle. Specifically, sine(2x) = 2sin(x)cos(x). This formula is essential in the video as it allows the transformation of the integrand into a more manageable form, which can then be integrated using standard techniques.
πŸ’‘integration by parts
Integration by parts is a technique used in calculus to evaluate integrals when the integrand is a product of two functions. The formula for integration by parts is ∫u dv = u*v - ∫v du. It is a powerful method for reducing complex integrals to simpler ones. In the video, integration by parts is applied to the integral of x times sine(2x) to find the indefinite integral.
πŸ’‘antiderivative
An antiderivative is a function whose derivative is the given function being integrated. In other words, it is the reverse process of differentiation. In the context of the video, finding the antiderivative of sine(2x) and cosine(2x) is crucial for completing the integration process.
πŸ’‘constant of integration
The constant of integration is an arbitrary constant, usually denoted as 'C', that is added to the antiderivative of a function to account for the infinite number of possible antiderivatives that exist for a given derivative. It is a fundamental concept in calculus, ensuring that the indefinite integral is correctly represented.
πŸ’‘sine function
The sine function is a trigonometric function that relates the ratio of the opposite side to the hypotenuse in a right triangle to the angle in that triangle. It is periodic with a period of 2Ο€ and oscillates between -1 and 1. In the video, the sine function is used in the expressions being integrated and is a key component in the final answer.
πŸ’‘cosine function
The cosine function is a trigonometric function that relates the ratio of the adjacent side to the hypotenuse in a right triangle to the angle in that triangle. It is also periodic with a period of 2Ο€ and oscillates between -1 and 1. In the video, the cosine function is used in the simplification and integration process of the given expression.
πŸ’‘trigonometric identities
Trigonometric identities are equations that relate different trigonometric functions to each other. They are essential tools for simplifying trigonometric expressions and solving trigonometric equations. In the video, the double angle formula is a specific trigonometric identity used to simplify the integrand before integration.
πŸ’‘integration techniques
Integration techniques are methods used to evaluate definite and indefinite integrals. They include basic rules like the power rule, substitution, integration by parts, and others. In the video, several integration techniques are discussed and applied to find the indefinite integral of a complex expression.
Highlights

The problem involves finding the indefinite integral of a complex trigonometric expression involving x, cosine squared x, and tangent x.

The first step is to simplify the expression by recognizing that cosine squared x is essentially cosine x times cosine x.

Tangent x is defined as sine x divided by cosine x, which allows for the cancellation of one cosine x in the expression.

After simplification, the integral becomes x times sine x times cosine x.

The double angle formula for sine, which is sine 2x = 2 sine x cosine x, is used to further simplify the expression.

By applying the double angle formula and dividing by 2, the expression is transformed into one half times x times sine 2x.

Integration by parts is the method used to solve the integral of the resulting expression.

The formula for integration by parts is the integral of u dv = u times v - the integral of v du.

In the integration by parts process, x is chosen as the u variable and sine 2x dx as the dv.

The derivative of u (du) is 1 times dx, and the antiderivative of dv (v) is negative cosine 2x divided by 2.

Plugging everything into the integration by parts formula yields one half times x times negative one half times cosine 2x minus the integral of negative one half times cosine 2x dx.

The integral of cosine 2x dx is the antiderivative of cosine 2x, which is sine 2x divided by 2.

The final answer includes terms of x cosine 2x and sine 2x, with respective coefficients and a constant of integration.

The solution is presented in a step-by-step manner, making it easy to follow and understand the process.

The problem-solving approach demonstrates a solid understanding of trigonometric identities and integration techniques.

The use of integration by parts shows adaptability in tackling more complex integrals.

The explanation is clear and methodical, highlighting the importance of simplifying expressions before integrating.

The final answer is a combination of trigonometric functions and their properties, showcasing the beauty of mathematical relationships.

Transcripts

Browse More Related Video

Integral of sinx cosx

Integration by parts: ºx____dx | AP Calculus BC | Khan Academy

Indefinite Integration (part 7)

_-substitution: definite integrals | AP Calculus AB | Khan Academy

Reduction Formulas For Integration

Product To Sum Identities and Sum To Product Formulas - Trigonometry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometricIntegrationMathematicsTutorialDoubleAngleFormulaIntegrationByPartsTrigonometricIdentitiesMathematicsEducationAdvancedCalculusOnlineLearningEducationalContentMathematicsCommunity