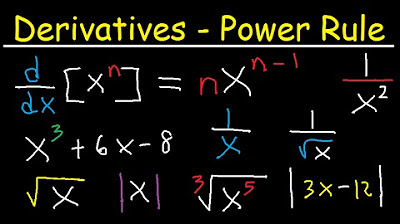Higher Order Derivatives

The Organic Chemistry Tutor

25 Feb 201810:50

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the concept of higher order derivatives in calculus, illustrating the process through several examples. It begins with finding the second derivative of a polynomial function, applying the power rule systematically. The script then progresses to more complex functions, such as the product rule for a function involving x squared and cosine x, and further differentiates to find the second derivative. It also tackles a case where the function is the square root of x, showing how to find the third derivative by repeatedly applying the power rule. Lastly, the script demonstrates finding the fourth derivative when given the second derivative, emphasizing the method of successive differentiation. The video is an informative guide for those looking to understand and apply higher order derivatives in their mathematical studies.

Takeaways

📚 The video focuses on finding higher order derivatives of functions.
🧮 To find the second derivative, first determine the first derivative of the function.
🌟 Example function: f(x) = 3x^5 + 2x^3 - 6x + 4.
📈 First derivative of the example: 15x^4 + 6x^2 - 6.
📈 Second derivative of the example: 60x^3 + 12x.
🤹‍♂️ For the function h(x) = x^2*cos(x), use the product rule to find derivatives.
📊 First derivative of h(x): 2x*cos(x) - x^2*sin(x).
📊 Second derivative of h(x) involves using the product rule again on the terms from the first derivative.
🔢 For 👉 f(x) = √x, find derivatives by rewriting the function as x^(1/2) and applying the power rule.
📝 Third derivative of 👉 f(x) = 3/8 * √x^5.
🔄 If given a higher derivative, like the second, find subsequent derivatives by differentiating the expression further.
🧠 The process of finding higher order derivatives involves a systematic application of differentiation rules.

Q & A

What is the main focus of the video?
-The main focus of the video is to demonstrate the process of finding higher order derivatives of given functions.
What is the first step in finding the second derivative of a function?
-The first step in finding the second derivative of a function is to determine the first derivative of the function.
What is the derivative of 3x to the fifth power using the power rule?
-The derivative of 3x to the fifth power using the power rule is 15x to the fourth power.
How do you find the derivative of a constant in a function?
-The derivative of a constant in a function is zero, as constants do not change with respect to the variable.
What is the product rule used for in differentiation?
-The product rule is used for differentiating the product of two functions. It states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.
What is the second derivative of the function h(x) = x squared cosine x?
-The second derivative of the function h(x) = x squared cosine x is 2 cosine x - 4x sine x - x squared sine x.
How can you rewrite the square root of x in exponential form?
-The square root of x can be rewritten in exponential form as x to the power of one half, which is the same as x^(1/2).
What is the third derivative of the function f(x) = the square root of x?
-The third derivative of the function f(x) = the square root of x, or f(x) = x^(1/2), is 3 times the square root of x divided by 8 times x cubed (3 sqrt(x) / (8x^3)).
If the second derivative of a function is 5/x squared, how do you find the fourth derivative?
-To find the fourth derivative from the second derivative 5/x squared, you need to differentiate it two more times. The fourth derivative in this case is 30 divided by x to the fourth power (30/x^4).
What is the significance of higher order derivatives in understanding a function?
-Higher order derivatives provide insights into the behavior of a function beyond just its rate of change. They can reveal information about the function's concavity, inflection points, and oscillatory behavior, which are crucial for a comprehensive understanding of the function's graph and properties.

Outlines

00:00

📚 Finding Higher Order Derivatives

This paragraph introduces the concept of higher order derivatives and demonstrates the process of finding the second derivative of a given polynomial function. The function f(x) = 3x^5 + 2x^3 - 6x + 4 is used as an example. The first derivative is calculated using the power rule, resulting in 15x^4 + 6x^2 + (-6). The second derivative is then found by differentiating the first derivative, yielding 60x^3 + 12x. The explanation emphasizes the importance of understanding the power rule and the process of repeated differentiation to find higher order derivatives.

05:04

📐 Applying the Product Rule for Derivatives

This section focuses on the application of the product rule for finding derivatives of functions that are the product of two other functions. The function h(x) = x^2 * cos(x) is used to illustrate the process. The first derivative h'(x) is calculated using the product rule, resulting in 2x * cos(x) - x^2 * sin(x). The explanation then proceeds to find the second derivative, h''(x), by applying the product rule again, leading to 2 * cos(x) - 4x * sin(x) - x^2 * cos(x). The summary emphasizes the importance of breaking down complex functions into simpler parts and applying the product rule iteratively to handle higher order derivatives.

10:06

🤔 Tackling Derivatives of Radical Functions

This paragraph discusses the process of finding higher order derivatives for radical functions. The function f(x) = √x, or x^(1/2), is used as an example to find the third derivative. The power rule is applied iteratively, leading to the first derivative of (1/2)x^(-1/2), the second derivative of -(1/4)x^(-3/2), and the third derivative of (3/8)x^(-5/2). The explanation then shows how to rewrite the third derivative with a positive exponent and in radical form, resulting in 3√x / (8x^3). The summary highlights the importance of understanding the power rule for radicals and the process of rewriting derivatives in simplified forms for better comprehension.

🔢 Deriving from Given Higher Order Derivatives

This final paragraph addresses the scenario where a higher order derivative is given, and the task is to find the next order derivative. Using the example of a second derivative of 5/x^2, the process of finding the fourth derivative is outlined. The derivative is calculated by applying the power rule, leading to a third derivative of -10x^(-3) and a fourth derivative of 30x^(-4). The explanation then shows how to rewrite the fourth derivative as 30/x^4, emphasizing the importance of understanding the power rule for negative exponents and the ability to manipulate expressions to find higher order derivatives.

Mindmap

Keywords

💡higher order derivatives

Higher order derivatives refer to the process of differentiating a function multiple times to find its higher derivatives. In the context of the video, this concept is central as it is used to determine the second, third, or even fourth derivative of a given function, which helps in understanding the function's behavior more comprehensively.

💡function f(x)

The function f(x) represents the mathematical relationship between an input variable x and an output. In the video, f(x) is used to define specific mathematical expressions such as 3x^5 + 2x^3 - 6x + 4, which is then differentiated to find its derivatives.

💡power rule

The power rule is a fundamental differentiation technique used when finding the derivative of a function that involves raising a variable to a power. According to the rule, the derivative of x^n (where n is a constant) is nx^(n-1). The video utilizes the power rule to find the first derivative of the initial polynomial function.

💡first derivative

The first derivative of a function represents the rate of change or the slope of the function at any given point. It is the result of the first differentiation of the original function. In the video, finding the first derivative is the initial step in the process of obtaining higher order derivatives.

💡second derivative

The second derivative is the derivative of the first derivative. It provides information about the concavity of a function and the rate of change of its slope. The second derivative is crucial for analyzing the function's curvature and inflection points. In the video, the second derivative is found by differentiating the first derivative of the given functions.

💡product rule

The product rule is a differentiation technique used when differentiating a product of two functions. It states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function. The product rule is essential for differentiating more complex functions involving multiplication, such as x^2*cos(x) in the video.

💡trigonometric functions

Trigonometric functions, such as sine and cosine, are mathematical functions that relate angles to real numbers. They are widely used in various fields, including mathematics, physics, and engineering. In the video, the derivative of the cosine function is used when applying the product rule to the function h(x) = x^2*cos(x).

💡chain rule

The chain rule is a differentiation technique used when differentiating a composite function, which is a function composed of other functions. The chain rule involves differentiating the outer function and then multiplying by the derivative of the inner function. Although not explicitly mentioned in the video, the chain rule is implied when differentiating expressions like the square root of x.

💡negative exponent

A negative exponent indicates the reciprocal of the base raised to the absolute value of the exponent. It is a concept used in algebra and calculus, particularly when dealing with derivatives that result in expressions with negative exponents. In the video, negative exponents appear when differentiating functions involving square roots and require simplification.

💡rationalize the denominator

Rationalizing the denominator is the process of eliminating the radicals in the denominator of a fraction by multiplying both the numerator and the denominator by a suitable expression to create a rational (non-radical) denominator. This technique is used to simplify expressions and prepare them for further calculation. In the video, the denominator is rationalized when expressing the third derivative of f(x) = sqrt(x).

💡inflection points

Inflection points are points on a curve where the concavity of the graph changes. They are significant in the study of calculus as they indicate a change in the nature of the function's curve without necessarily being extreme points. The second derivative test is often used to identify inflection points. Although not explicitly discussed in the video, understanding inflection points is crucial when analyzing higher order derivatives.

Highlights

The video focuses on finding higher order derivatives of functions.

The first example involves a polynomial function f(x) = 3x^5 + 2x^3 - 6x + 4.

The first derivative of the given polynomial is found using the power rule, resulting in f'(x) = 15x^4 + 6x^2 - 6.

The second derivative of the polynomial is calculated as f''(x) = 60x^3 + 12x.

The second example involves a product of functions, h(x) = x^2 * cos(x), where the product rule is applied to find the first derivative.

The first derivative of h(x) is h'(x) = 2x * cos(x) - x^2 * sin(x).

The second derivative of h(x) is found by applying the product rule again, resulting in h''(x) = 2 * cos(x) - 4x * sin(x) - x^2 * cos(x).

The third example deals with finding the third derivative of a function f(x) = √x, which is rewritten as x^(1/2) for differentiation purposes.

The first derivative of √x is f'(x) = (1/2) * x^(-1/2).

The second derivative of √x is f''(x) = (-1/4) * x^(-3/2).

The third derivative of √x, f'''(x), is calculated to be 3 * √x / (8 * x^(3/2)).

The fourth example involves finding the fourth derivative of a function given its second derivative, which is 5/x^2.

The third derivative of the function with the second derivative of 5/x^2 is found to be -10/x^3.

The fourth derivative is calculated as 30/x^4, which can be rewritten as 30/(x^4).

The process of finding higher order derivatives involves applying the power rule and product rule iteratively.

The video demonstrates the importance of step-by-step differentiation for complex functions and products of functions.

The examples provided in the video showcase the application of fundamental calculus rules in solving higher order derivative problems.

Transcripts

Browse More Related Video

Derivatives - Power Rule

Taking the derivative of two binomials using product and chain rule

Partial Derivatives - Multivariable Calculus

Product Rule For Derivatives

Higher Order Derivatives!

Lesson 6 - Higher Derivatives in Calculus (Calculus 1 Tutor)