AP Physics Workbook 6.I Changing Mass and Period of a Mass Spring SYstem

Mr.S ClassRoom
15 Apr 202015:53
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses a physics problem involving a mass-spring system, focusing on calculating the spring constant (k) and amplitude (a) given the system's total energy. It explains the conservation of energy principle and uses equations to derive the mass (M) from the initial kinetic energy, then the spring constant (k) from the period of oscillation. The differences in maximum speed and amplitude in two scenarios are attributed to changes in the system's mass and mechanical energy. The script emphasizes understanding the relationships between energy, period, and amplitude in simple harmonic motion.

Takeaways
  • 🌟 The spring system in question has 10 Joules of energy, which is used to calculate the mass (M), spring constant (k), and amplitude (a).
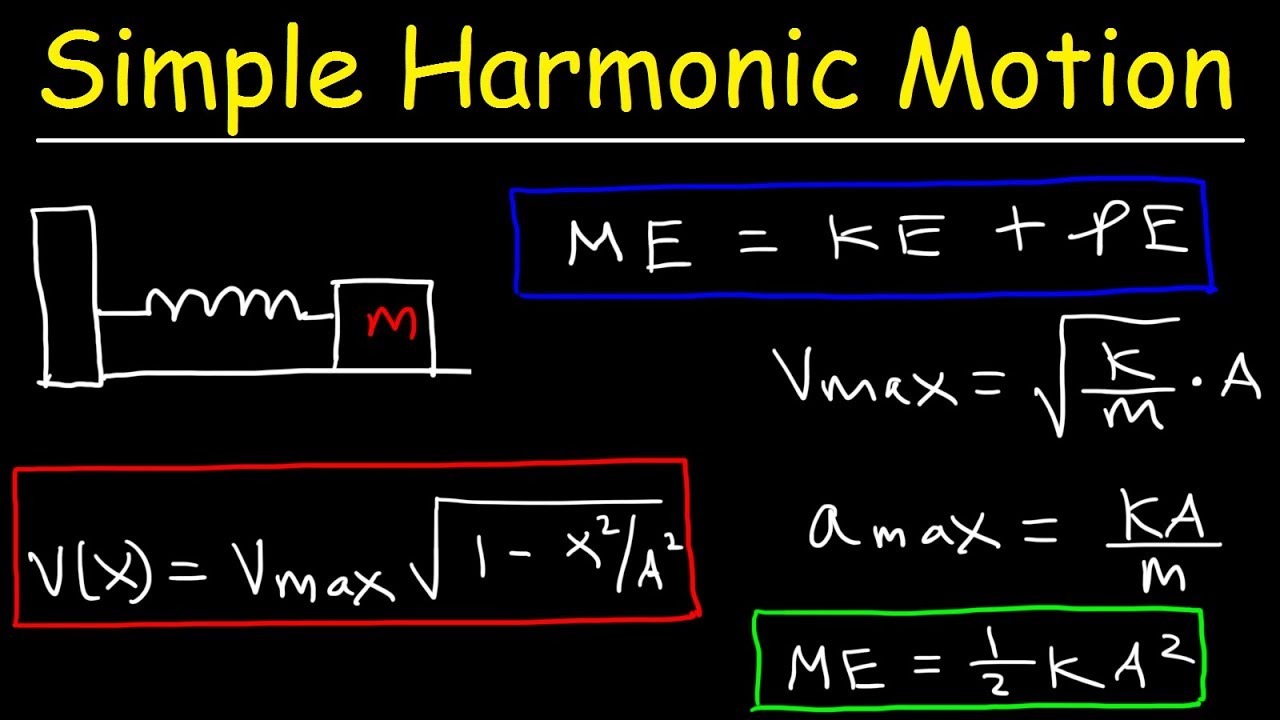
  • πŸ“š The total mechanical energy of a simple harmonic oscillator is the sum of gravitational potential energy, spring potential energy, and kinetic energy.
  • πŸ”„ At the equilibrium position, the gravitational potential energy and spring potential energy are both zero, leaving only the kinetic energy to contribute to the total energy.
  • πŸ‹οΈβ€β™‚οΈ The initial velocity of the system is given as 2 meters per second, which is used to calculate the mass (M) using the conservation of energy principle.
  • πŸ“ The period of oscillation is derived from the mass (M) and the spring constant (k) using the formula T = 2Ο€βˆš(M/k).
  • πŸ•’ The period is determined to be 4 seconds by observing one complete cycle of the system's motion.
  • πŸ“ˆ The spring constant (k) is calculated to be approximately 12.33 N/m using the period and the mass.
  • πŸ“Š The amplitude (a) is found by rearranging the energy equation and substituting the known values of energy (E) and spring constant (k), resulting in an amplitude of 1.27 meters.
  • πŸ”„ The total mechanical energy of a simple harmonic oscillator is proportional to the square of the amplitude (1/2 k a^2).
  • πŸš€ In the first case, the period increases due to an increase in mass when the dart and cart combine, resulting in an increase in amplitude.
  • πŸ”„ In the second case, the block and dart move in opposite directions after collision, leading to a decrease in mechanical energy and, consequently, a decrease in amplitude.
Q & A

  • What is the total energy of the spring system mentioned in the transcript?

    -The total energy of the spring system is 10 Joules.

  • What are the components of the system's energy?

    -The system's energy is composed of gravitational potential energy, spring potential energy, and kinetic energy.

  • How is the gravitational potential energy (ug) defined in the context of the problem?

    -The gravitational potential energy (ug) is defined as MGH, where M is the mass and H is the height.

  • What is the spring potential energy in the equilibrium position?

    -At the equilibrium position, the spring potential energy is zero because the displacement X is zero.

  • What is the initial velocity of the system, and how is it used in the calculations?

    -The initial velocity of the system is 2 meters per second. It is used to calculate the mass (M) by applying the conservation of energy principle.

  • How is the spring constant (K) determined from the given period (T)?

    -The spring constant (K) is determined using the period equation T = 2Ο€βˆš(M/K). By knowing the mass (M) and the period (T), K can be solved.

  • What is the amplitude (a) of the system, and how is it calculated?

    -The amplitude (a) is the maximum displacement of the mass from its equilibrium position. It is calculated using the energy equation K*a^2 = E, where E is the total energy of the system.

  • How does the total mechanical energy of a simple harmonic oscillator relate to the amplitude?

    -The total mechanical energy of a simple harmonic oscillator is proportional to the square of the amplitude, as given by the equation E = 1/2 * K * a^2.

  • What happens to the period when the mass of the system increases?

    -When the mass of the system increases, the period also increases, as seen in the case where the dart and the cart combine, increasing the total mass and thus the period T = 2Ο€βˆš(M/K).

  • How does the amplitude change when the system's mechanical energy increases?

    -When the system's mechanical energy increases, the amplitude also increases because E = 1/2 * K * a^2. More energy means a larger amplitude for the same spring constant.

  • What is the significance of the conservation of energy principle in solving this problem?

    -The conservation of energy principle is crucial in solving this problem as it allows us to relate the initial kinetic energy to the total mechanical energy of the system, which includes potential and kinetic energies, to find the mass and subsequently the spring constant and amplitude.

Outlines
00:00
πŸ“š Introduction to Simple Harmonic Motion

The video begins by introducing the topic of simple harmonic motion within the context of an AP Physics workbook. It outlines the focus on unit 6, specifically addressing the mass-spring system and its period. The scenario involves calculating the mass (M), spring constant (k), and amplitude (a) of the system, given that it has 10 joules of energy. The video script explains the method for solving these variables, starting with the energy equation (E = potential + kinetic) and emphasizing the conservation of energy principle. It clarifies that at equilibrium, the gravitational potential and spring potential are zero, simplifying the energy equation to E = 1/2 MV^2. The script then proceeds to solve for mass (M) using the given initial velocity and energy, and sets up the process for finding the spring constant (k) and amplitude (a).

05:02
πŸ•°οΈ Determining Period and Spring Constant

This paragraph delves into the concept of the period (T) in relation to a mass-spring system. It explains that the period represents one complete cycle of motion, using the sine and cosine graphs to illustrate a full cycle. The script then uses the given period of 4 seconds to derive the spring constant (k) through the period equation (T = 2Ο€βˆš(M/k)). By rearranging and solving the equation, the video demonstrates how to calculate k, and subsequently, the amplitude (a) using the energy equation (E = 1/2 k*a^2). The explanation includes a step-by-step walkthrough of the mathematical process, highlighting the importance of understanding the relationships between energy, mass, and the spring constant in a simple harmonic oscillator system.

10:08
πŸ“ˆ Analysis of Energy and Amplitude

The third paragraph focuses on analyzing the energy and amplitude of a simple harmonic oscillator in different scenarios. It explains the relationship between the total mechanical energy and the square of the amplitude (E ∝ a^2), and how changes in mass or energy can affect the period and amplitude. The script presents two cases: one where the mass of the system increases due to an elastic collision (dart and cart combining), leading to an increase in period and amplitude, and another where the block and dart move in opposite directions post-collision, resulting in a decrease in mechanical energy and thus a decrease in amplitude. The explanation emphasizes the importance of understanding how the physical properties and interactions within a system can influence its behavior and performance.

15:09
πŸŽ“ Summary of Key Equations and Concepts

The final paragraph wraps up the video by summarizing the key equations and concepts discussed. It reiterates the total mechanical energy equation, the period equation for a spring, and the energy equation in terms of amplitude. The script ensures that viewers understand the derivation and significance of these equations, and how they relate to the behavior of a mass-spring system in simple harmonic motion. It also provides a brief explanation of the concepts, reinforcing the learning objectives of the video and ensuring that viewers have a comprehensive understanding of the material covered.

Mindmap
Keywords
πŸ’‘Simple Harmonic Motion
Simple Harmonic Motion (SHM) is a type of periodic motion where an object moves back and forth along a straight line or an arc, experiencing a restoring force that is directly proportional to the displacement from its equilibrium position. In the video, SHM is the central theme, with the mass-spring system being a classic example. The motion of the mass-spring system is governed by the spring constant and the mass of the object, which are used to calculate the period and amplitude of the oscillation.
πŸ’‘Spring Constant (K)
The spring constant, denoted by K, is a measure of the stiffness of a spring. It is defined by Hooke's Law as the ratio of the force exerted by the spring to the displacement caused by the force. In the context of the video, the spring constant is a critical parameter in determining the period of oscillation and the amplitude. The video shows how to calculate K using the period and the mass of the system, with the formula K = (5m * E) / (12.3 * sqrt(2 * pi)).
πŸ’‘Amplitude (a)
Amplitude, symbolized by 'a', is the maximum displacement of an object from its equilibrium position in a periodic motion like SHM. It represents the extent of the oscillation and is directly proportional to the square root of the total mechanical energy in the system. In the video, the amplitude is calculated using the energy equation (E = 1/2 * K * a^2), which shows that an increase in energy leads to an increase in amplitude, and vice versa.
πŸ’‘Period (T)
The period of a motion is the time taken for one complete cycle of the motion. In the context of a mass-spring system undergoing SHM, the period is determined by the mass of the object and the spring constant. The formula for the period of a simple pendulum is T = 2 * pi * sqrt(M/K), where M is the mass and K is the spring constant. The video explains how changes in mass or spring constant affect the period, as seen when a dart is added to a moving cart, altering the system's mass and thus the period of oscillation.
πŸ’‘Conservation of Energy
The principle of conservation of energy states that the total amount of energy in a closed system remains constant, though it may change forms. In the context of the mass-spring system, this means that the total mechanical energy (potential plus kinetic) remains constant during the oscillation. The video uses this principle to solve for the mass M and the spring constant K, showing that the initial energy (10 Joules) is conserved throughout the system's motion.
πŸ’‘Mechanical Energy
Mechanical energy is the sum of potential energy and kinetic energy in a mechanical system. In the context of the video, the mechanical energy of the mass-spring system is related to the amplitude of the oscillation and is conserved during the motion. Changes in mechanical energy, such as when a dart is added to a cart, result in changes in the amplitude and period of the system's oscillation.
πŸ’‘Potential Energy
Potential energy is the stored energy an object has due to its position or condition. In the context of the mass-spring system, the potential energy is associated with the spring's deformation and is given by the formula 1/2 KX^2, where K is the spring constant and X is the displacement from the equilibrium position. The video explains that at the equilibrium position, the spring potential energy is zero since there is no deformation.
πŸ’‘Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. In the mass-spring system, the kinetic energy is given by the formula 1/2 MV^2, where M is the mass of the object and V is its velocity. The video uses the conservation of energy principle to relate the kinetic energy to the potential energy and to solve for the mass and other parameters of the system.
πŸ’‘Equilibrium Position
The equilibrium position is the position where the net force on an object is zero, and it has the lowest potential energy in the context of a mass-spring system. In the video, the equilibrium position is the starting point for the oscillation, and the potential energy of the spring is zero at this point because the spring is neither compressed nor stretched.
πŸ’‘Gravitational Potential Energy
Gravitational potential energy is the energy an object possesses due to its elevated position in a gravitational field. It is given by the formula MGH, where M is the mass of the object, G is the acceleration due to gravity, and H is the height above a reference point. In the context of the video, the gravitational potential energy is not relevant to the mass-spring system as the height does not change during the oscillation, thus it is considered zero.
πŸ’‘Velocity
Velocity is a vector quantity that describes the rate of change of an object's position. It has both magnitude (speed) and direction. In the context of the mass-spring system, the velocity of the mass is used to calculate its kinetic energy. The video provides an initial velocity value (2 m/s), which is crucial for determining the system's total mechanical energy and subsequently solving for the mass and other parameters.
Highlights

The spring system has 10 joules of energy.

The method to calculate the spring constant (k) and amplitude (a) is explained.

The total mechanical energy of the system is a sum of gravitational potential, spring potential, and kinetic energy.

At the equilibrium position, the gravitational potential and spring potential energies are zero.

The velocity of the mass at the initial position is given as 2 meters per second.

The mass (M) can be calculated using the conservation of energy principle.

The period (T) of the mass-spring system is related to the mass and spring constant through T = 2Ο€βˆš(M/K).

The period is determined by observing one complete cycle of the system's motion.

The spring constant (k) can be calculated using the period and mass.

The amplitude (a) is defined by the equation a = √(E/(1/2 * k))

The total mechanical energy of a simple harmonic oscillator is proportional to the square of the amplitude.

In one case, an increase in wave cycles per given time indicates an increase in the period and amplitude.

The mass of the system affects the period; an increase in mass results in an increased period.

The direction of motion of the mass and another object (like a dart) at the moment of collision affects the mechanical energy and amplitude.

An increase in mechanical energy post-collision results in an increase in amplitude.

A decrease in mechanical energy post-collision results in a decrease in amplitude.

Transcripts

Browse More Related Video

Simple Harmonic Motion - Complete Review of the Mass-Spring System

Energy In a Simple Harmonic Oscillator - Maximum Velocity & Acceleration Calculations

AP Physics Workbook 6.L Sine function of SHM

'AP Physics 2023 Exam Solutions|Q1. PART C ( i ) | Complete Step-by-Step Answers and Explanations"

2018 #5 Free Response Question - AP Physics 1 - Exam Solution

AP Physics Workbook 6.K Mass and Period of Mass Spring System

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationHarmonicMotionSpringConstantEnergyConservationMechanicalEnergyMassSpringSystemAmplitudeChangePeriodEquationElasticCollisionKineticPotentialEnergy