Torque, Basic Introduction, Lever Arm, Moment of Force, Simple Machines & Mechanical Advantage
TLDRThe transcript discusses the concept of torque, explaining its relationship with force and the moment arm. It illustrates how the ease of moving an object, such as a door, depends on the position where force is applied. The video covers various ways to calculate torque, including when force is applied at an angle. It also explains how torque influences the rotational movement of objects and its role in simple machines like seesaws and shovels, emphasizing the concept of mechanical advantage.
Takeaways
- 🚪 Torque is the key factor in determining the ease of moving an object, like a door, and is calculated as the product of the force applied and the moment arm (lever arm).
- 📐 The moment arm is the perpendicular distance from the axis of rotation to the line of action of the force, which is parallel to the force and extends from it.
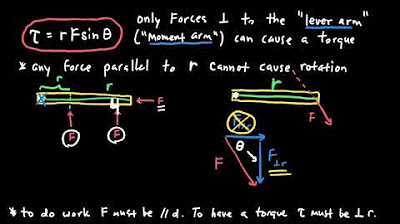
- 🔢 When the force is applied perpendicular to the object, the torque can also be calculated as the force times the distance (l) times the sine of the angle (θ).
- 🔄 The direction of the torque affects the rotation: a positive torque causes counterclockwise rotation, while a negative torque results in clockwise rotation.
- 📈 To calculate the net torque, sum the individual torques acting on the object, taking into account their direction (positive or negative).
- 🌀 The mechanical advantage of a simple machine, like a seesaw, is the ratio of the output force to the input force, which can be calculated as the input lever arm divided by the output lever arm.
- 🔨 The output force generated by a simple machine can be greater than the input force, depending on the mechanical advantage.
- 🍽️ Using a seesaw as an example, applying a force farther from the fulcrum (greater lever arm) results in a higher output force on the opposite side.
- 🛠️ The mechanical advantage of a shovel increases with the distance from the axis of rotation to where the force is applied; the greater the distance, the more force is generated.
- 🏗️ When using a shovel, placing your hand at the edge provides the best mechanical advantage, allowing you to lift heavier loads with less effort.
- ⚖️ The mechanical advantage and torque are fundamental concepts in understanding how simple machines like levers can amplify forces for easier work.
Q & A
What is the concept of torque and how does it relate to force and the moment arm?
-Torque is a measure of the force's effectiveness in causing an object to rotate around an axis. It is calculated as the product of the force applied and the moment arm (or lever arm), which is the perpendicular distance from the axis of rotation to the line of action of the force. A greater torque can be achieved either by applying a larger force or by increasing the moment arm.
Why is it easier to push a door at point A compared to point C as described in the script?
-It is easier to push the door at point A because the moment arm at point A is greater than at point C. This results in a larger torque when applying the same force at point A compared to point C, making it easier to move the door.
How does the angle between the force and the door affect the torque calculation?
-When there is an angle between the force and the door, the torque is calculated by considering the perpendicular component of the force. This is done by multiplying the force by the sine of the angle (θ) and the distance (l) from the axis of rotation, resulting in the equation: torque = force * l * sin(θ).
What is the significance of the direction of torque in relation to positive and negative values?
-A positive torque value indicates that the force will cause an object to rotate in a counterclockwise direction, while a negative torque value indicates a clockwise rotation. The direction of the torque is essential for understanding the rotational effect of the force applied.
How do you calculate the net torque acting on an object when multiple forces are applied?
-The net torque is calculated by summing the individual torques caused by each force. Each torque is found by multiplying the force by its respective moment arm and considering the direction (positive or negative). The net torque is the algebraic sum of all these torques.
What is the seesaw example in the script illustrating about mechanical advantage?
-The seesaw example demonstrates how a simple machine can use leverage (torque) to multiply the input force. By applying a downward force at a certain distance from the fulcrum (input force), an output force is generated on the other side. The mechanical advantage is the ratio of the output force to the input force, which in the example is 2, meaning the machine doubles the force applied.
How does the shovel example in the script show the concept of mechanical advantage?
-The shovel example shows that by applying a force at the edge of the shovel (longer lever arm), the output force generated is significantly greater than the input force, providing a high mechanical advantage of 10. This means that with less effort, one can lift a heavier load due to the leverage created by the shovel's design.
What happens to the mechanical advantage if the input force is applied at different points on the shovel?
-The mechanical advantage changes depending on where the input force is applied. If applied at the middle of the shovel, the mechanical advantage is reduced to half of its original value (from 10 to 5), and the output force is also reduced accordingly. However, applying the force at the edge maintains the maximum mechanical advantage and output force.
How does the concept of torque relate to simple machines and mechanical advantage?
-Torque is fundamental to simple machines as it allows them to multiply forces and provide mechanical advantage. By adjusting the lever arms (moment arms) in a machine, one can increase or decrease the torque, thereby altering the mechanical advantage and the effectiveness of the machine in performing work.
What is the ideal mechanical advantage of a machine and how is it calculated?
-The ideal mechanical advantage of a machine is the ratio of the output force to the input force when no energy is lost in the process. It is calculated by dividing the input lever arm (distance from the axis of rotation to the input force) by the output lever arm (distance from the axis of rotation to the output force).
How does the actual mechanical advantage differ from the ideal mechanical advantage?
-The actual mechanical advantage takes into account energy losses due to factors like friction and inefficiency in the machine's operation. While the ideal mechanical advantage assumes no energy loss, the actual mechanical advantage will often be slightly less than the ideal due to these real-world factors.
Outlines
🚪 Understanding Torque and its Calculation
This paragraph explains the concept of torque, which is the force applied to an object that causes it to rotate. It uses the analogy of pushing a door at different points (A, B, and C) to illustrate that the ease of moving the door depends on the position where the force is applied. Torque is defined as the product of the force and the moment arm (or lever arm), which is the perpendicular distance from the axis of rotation to the line of action of the force. The paragraph further explains how to calculate torque in different scenarios, such as when the force is applied perpendicular to the object and when there is an angle between the force and the object. The importance of understanding torque is emphasized as it is fundamental in various applications, including simple machines like levers.
📐 Calculating Torque with Components and Angles
This paragraph delves deeper into the calculation of torque, focusing on the components of force and the impact of angles on torque. It explains that a force can be broken down into parallel and perpendicular components relative to the object's surface. The perpendicular component is the one that contributes to torque. The paragraph also discusses how to calculate torque when an angle is introduced between the force and the object, using the sine of the angle in the calculation. It further clarifies the concept of positive and negative torque, depending on whether the force causes counterclockwise or clockwise rotation. The paragraph concludes with examples of calculating net torque when multiple forces are applied to an object, demonstrating how to determine if the object is in equilibrium or not.
🔄 Torque and Simple Machines: The Seesaw
This paragraph explores the application of torque in the context of simple machines, specifically the seesaw. It explains how torque can be used to multiply a force by adjusting the lever arm, thereby providing mechanical advantage. The paragraph uses an example where a downward force is applied to one end of a seesaw, and it calculates the output force on the other end. The seesaw is modeled as a lever with the fulcrum as the axis of rotation. By applying the concept of equal torques on both sides of the fulcrum, the paragraph demonstrates how the output force is twice the input force, resulting in a mechanical advantage of two. The ideal mechanical advantage is calculated as the ratio of the input lever arm to the output lever arm, highlighting the efficiency of the simple machine in multiplying forces.
🛠️ Leverage in Action: The Shovel
This paragraph examines another simple machine, the shovel, and its ability to multiply forces through leverage. It describes how the distance from the axis of rotation (the shovel's edge) and the point where the force is applied affects the output force. The paragraph provides a detailed example where an input force is applied to a shovel, and it calculates the resulting output force based on the input lever arm and the output lever arm. It emphasizes the mechanical advantage of the shovel, which is the ratio of the output force to the input force. The paragraph also discusses how the position of the hands on the shovel affects the mechanical advantage, advising that placing the hands at the edge of the shovel maximizes leverage and reduces the effort needed to lift heavy objects.
Mindmap
Keywords
💡Torque
💡Moment Arm
💡Line of Action
💡Mechanical Advantage
💡Rotational Motion
💡Force
💡Axis of Rotation
💡Counterclockwise and Clockwise Rotation
💡Simple Machines
💡Leverage
💡Right Triangle
Highlights
Exploring the concept of torque and its impact on the ease of moving an object, such as a door, at different points (position a, b, and c).
Discussing how the position of the applied force affects the moment arm and consequently the torque, with position a being the easiest to move due to the greater moment arm.
Describing the calculation of torque as the product of the force and the moment arm, emphasizing the importance of perpendicular distance from the axis of rotation.
Introducing the concept of the line of action and how it relates to the moment arm when an angle is introduced between the force and the door.
Explaining the use of trigonometry, specifically the sine function, to calculate the moment arm (r) when there is an angle (theta) between the force and the door.
Discussing the impact of the direction of the force on the sign of the torque, with positive torque resulting from counterclockwise rotation and negative torque from clockwise rotation.
Providing a practical example of calculating net torque with two forces applied at different distances and directions from the axis of rotation.
Explaining how to calculate the net torque when multiple forces are applied to an object, and how this relates to the object's rotation.
Discussing the application of torque in simple machines like the seesaw, and how it can be used to multiply forces through leverage.
Describing the calculation of output force in a seesaw scenario, and how the mechanical advantage is determined by the ratio of the lever arms.
Exploring the mechanical advantage of a shovel as another example of a simple machine, and how the position of the applied force affects the output force and mechanical advantage.
Highlighting the importance of applying force at the edge of the shovel to maximize mechanical advantage and lift heavy objects with less effort.
Discussing the theoretical and actual mechanical advantage of machines, and how they can differ in practical scenarios.
Providing a comprehensive understanding of torque, its calculation, and its application in real-world scenarios through the use of simple machines.
Emphasizing the importance of understanding the relationship between force, distance, and angle in calculating torque and mechanical advantage.
Demonstrating how the principles of torque and mechanical advantage can be applied to everyday tasks, such as moving objects or lifting heavy loads, to reduce the required effort.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: