Torque

Bozeman Science
22 Sept 201407:03
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Mr. Andersen explains the concept of torque in the context of physics, focusing on its role in rotational motion. He illustrates how torque is the product of force applied perpendicular to the lever arm, resulting in rotational acceleration. The importance of the lever arm's distance in determining mechanical advantage is highlighted, and practical examples like door knobs and seesaws are used to demonstrate the principles of torque and balanced systems. The video concludes with an interactive question about designing an experiment to explore balanced forces, encouraging viewers to apply their understanding of torque.

Takeaways
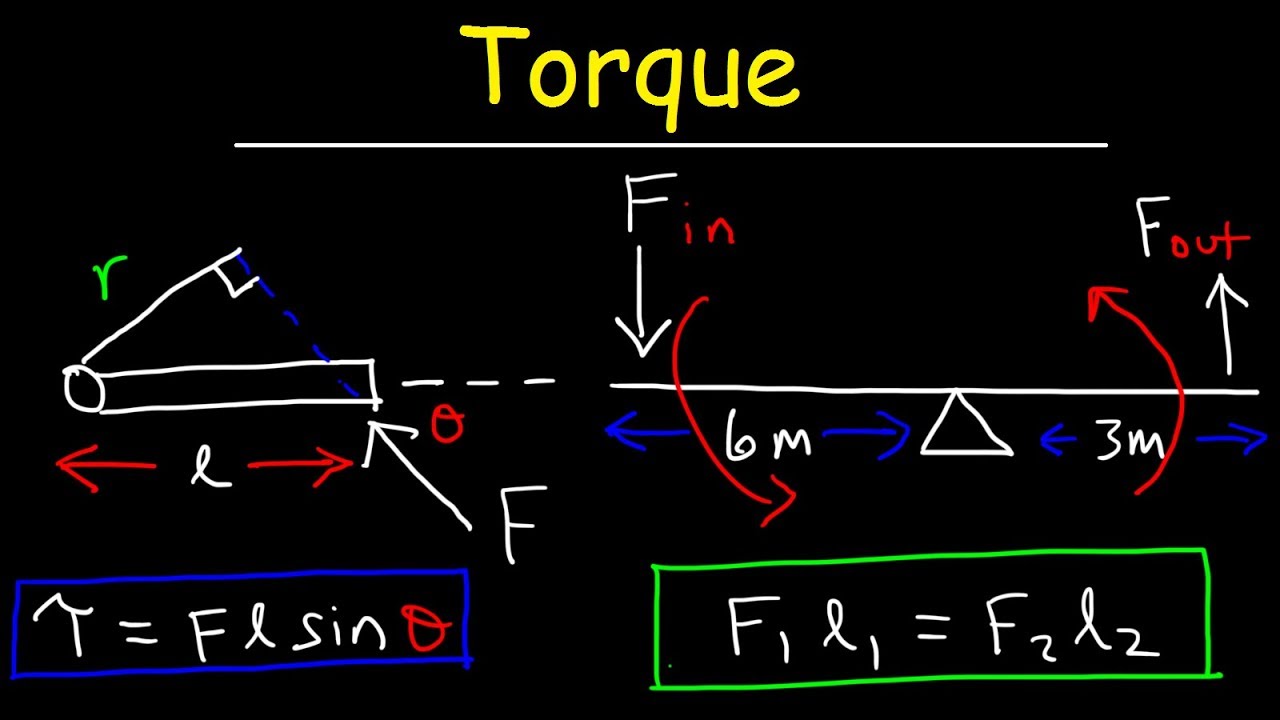
  • πŸ“ Torque is the product of force applied and the lever arm, which is perpendicular to the axis of rotation.
  • πŸŒ€ Rotational motion occurs when a force is applied off-center, causing both translational and rotational movement.
  • πŸ”§ The lever arm is the distance from the axis of rotation to the point where the force is applied, acting like a simple lever.
  • πŸ“ˆ Torque is measured in newton meters (NΒ·m), indicating both the magnitude of the force and the distance it is applied from the rotation axis.
  • πŸšͺ The position of the door knob relative to the hinge exemplifies the principle of torque, with the knob further from the hinge providing greater mechanical advantage.
  • πŸ”„ In a balanced system, the net torque is zero, meaning all torques acting on the system are balanced and no movement occurs.
  • βš–οΈ Changing the magnitude of the force or the length of the lever arm affects the torque, with greater force or longer arm resulting in higher torque.
  • πŸ”„ A see-saw is an example of a balanced system where torques on either side can be manipulated to achieve equilibrium.
  • πŸ”’ The relationship between force, lever arm, and torque can be used to solve for unknown distances or forces in a system.
  • πŸ§ͺ Experimental manipulation of balanced forces can be conducted using simple machines like a teeter-totter.
  • πŸ“Š Understanding the relationship between force and torque allows for the calculation of torque in two-dimensional systems.
Q & A

  • What is torque?

    -Torque is the product of the force applied and the lever arm, which is the perpendicular distance from the axis of rotation to the point where the force is applied. It is a measure of the rotational force and has units of newton meters.

  • What is the difference between linear motion and rotational motion?

    -Linear motion, also known as translational motion, occurs when a force is applied in one direction, resulting in acceleration in that direction. Rotational motion occurs when a force is applied off-center, causing an object to rotate around a fixed point.

  • How does the position of the force application affect torque?

    -The position of force application affects torque through the length of the lever arm. The further the force is applied from the axis of rotation, the longer the lever arm and the greater the torque produced for the same force.

  • What are the units of torque?

    -The units of torque are newton meters (NΒ·m), which reflect the product of the force in newtons and the lever arm in meters.

  • What is the relationship between torque and mechanical advantage?

    -Torque provides a mechanical advantage by allowing a smaller force applied at a greater distance from the axis of rotation (longer lever arm) to produce the same or greater rotational effect compared to a larger force applied closer to the axis.

  • How does the net torque on a system affect its motion?

    -If the net torque on a system is not zero, the system will experience rotational acceleration. If the net torque is zero, the system is in a balanced state and does not rotate.

  • What is the significance of the door knob placement in relation to the hinge?

    -The placement of the door knob further from the hinge increases the lever arm, which in turn increases the torque when the same force is applied, making it easier to open the door.

  • How can you calculate the torque in the given door example?

    -To calculate the torque, you multiply the force applied (in newtons) by the lever arm (in meters). For example, a 6.7 newton force applied at a distance of 0.75 meters (75 centimeters) from the hinge results in a torque of 5 newton meters.

  • What happens to the torque when you decrease the lever arm distance?

    -When you decrease the lever arm distance, the torque is reduced, which may not be sufficient to cause the desired rotational motion, such as opening a door.

  • How does the see-saw example illustrate balanced torque?

    -In the see-saw example, balanced torque occurs when the product of the force and lever arm on each side is equal, resulting in no net torque and thus no movement of the see-saw.

  • How can you balance a system with unequal weights on a see-saw?

    -To balance a system with unequal weights, you can adjust the distance of the weights from the axis of rotation so that the product of the force (weight) and lever arm is equal on both sides, resulting in a net torque of zero.

Outlines
00:00
πŸ”§ Introduction to Torque and Rotational Motion

This paragraph introduces the concept of torque, defined as the product of force applied perpendicular to the lever arm, and its significance in rotational motion. Mr. Andersen uses the analogy of a wrench to explain the difference between linear (translational) motion and rotational motion. He further clarifies that torque is relevant when a force is applied off-center, resulting in both translational and rotational movement. The importance of the lever arm in calculating torque is emphasized, and the units of torque are explained as newton meters. The concept is applied to everyday examples, such as door knobs and hinges, to illustrate how changes in the position of the force application (lever arm) affect torque and rotational acceleration.

05:05
πŸ“ Balancing Torques and Calculations

In this paragraph, Mr. Andersen discusses the balance of torques in a system, using the example of a balanced see-saw. He explains that if torques are balanced, there is no movement in the system. By adding different weights to each side of the see-saw, he demonstrates how changing the magnitude of force or the distance of the force application (lever arm) can disrupt balance and cause rotational motion. The paragraph concludes with a practical question about how to rebalance the system by adjusting the position of the mass, reinforcing the relationship between force, lever arm, and torque. Calculations are provided to show how torque is computed in a two-dimensional system, emphasizing the need for consistent units (SI units) and the impact of varying the lever arm on the resulting torque.

Mindmap
Keywords
πŸ’‘Torque
Torque is the product of the force applied and the lever arm, which is the perpendicular distance from the axis of rotation to the point where the force is applied. It is a measure of the rotational force and is crucial in understanding rotational motion. In the video, torque is illustrated by applying force to a wrench and a door knob, showing how it affects rotational acceleration.
πŸ’‘Rotational Motion
Rotational motion refers to the movement of an object around a fixed axis. In the context of the video, it is demonstrated by the wrench rotating around a nut and the door knob turning around the hinge. The video emphasizes that torque is directly related to the rotational motion and is responsible for causing it.
πŸ’‘Lever Arm
The lever arm is the distance from the axis of rotation to the point where the force is applied. It acts like a simple machine's lever, amplifying the effect of the force when it is perpendicular to the lever arm. In the video, the lever arm is used to explain how moving the door knob further from the hinge increases the torque and thus the ease of opening the door.
πŸ’‘Force
Force is any push or pull upon an object resulting from its interaction with another object. In the video, force is applied to the wrench and the door knob to generate torque and cause rotational motion. The magnitude and direction of the force, in relation to the lever arm, determine the effectiveness of the torque produced.
πŸ’‘Center of Gravity
The center of gravity is the point at which the total weight of the body is considered to be concentrated. In the video, it is mentioned in the context of applying force directly at the center of gravity of the wrench, which results in linear or translational motion rather than rotational motion.
πŸ’‘Translational Motion
Translational motion is when all points of an object move the same distance, in the same direction, and during the same time interval. In the video, it is contrasted with rotational motion. When force is applied at the center of gravity, the wrench undergoes translational motion instead of rotating.
πŸ’‘Newton Meters
Newton meters is the unit of torque in the International System of Units (SI). It is derived from the product of the force in newtons and the lever arm in meters. The video explains that when calculating torque, the units are not just newtons but newton meters, reflecting the combination of force and distance.
πŸ’‘Mechanical Advantage
Mechanical advantage is the ratio of output force to input force in a mechanical system. In the video, the lever arm provides a mechanical advantage by increasing the effectiveness of the applied force when trying to open a door. The longer the lever arm, the less force is needed to produce the same amount of torque.
πŸ’‘Balanced System
A balanced system is one where the net torque is zero, meaning all torques acting on the system are balanced. In the video, this is demonstrated using a seesaw, where placing equal weights at equal distances from the pivot point results in no movement, indicating a balanced system.
πŸ’‘Axis of Rotation
The axis of rotation is the imaginary line around which an object rotates. In the video, it is the point from which the lever arm is measured and is central to understanding how torque affects rotational motion. The force must be applied perpendicular to the axis of rotation to produce torque.
Highlights

Torque is defined as the product of force and the lever arm, which is perpendicular to the axis of rotation.

Rotational motion occurs when a force is applied off-center, causing both translational and rotational movement.

To isolate rotational motion, the object should be pinned down to rotate around a fixed point.

The lever arm is the distance from the axis of rotation to the point where the force is applied.

Torque has units of newton meters, indicating both force and rotational effect.

In a balanced system, the net torque is zero, meaning all torques are balanced.

The position of the door knob affects the torque and ease of opening the door.

Increasing the force or lever arm distance increases torque, leading to faster rotational acceleration.

Decreasing the lever arm distance with a constant force significantly reduces torque, potentially preventing movement.

The mechanical advantage in torque is determined by the ratio of lever arm distances.

Torque can be calculated using the formula Tau = r * F, where r is the lever arm and F is the force.

Changing the position of the force application on a lever can dramatically alter the resulting torque.

A balanced system, like a seesaw, can be used to demonstrate and understand the effects of torque.

By adjusting the distance of the force application, one can balance a system with unequal weights on a seesaw.

Understanding torque and its relationship with force and lever arm is crucial for designing systems that require controlled rotational motion.

Transcripts

Browse More Related Video

Torque, Basic Introduction, Lever Arm, Moment of Force, Simple Machines & Mechanical Advantage

AP Physics 1 - Torque

AP Physics Workbook 7.B Torque

Introduction to torque | Moments, torque, and angular momentum | Physics | Khan Academy

Torque = Force Times Lever Arm | Physics with Professor Matt Anderson | M12-06

How to solve Rotational Equilibrium Problems

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsConceptsTorqueExplainedRotationalMotionLeverArmsForceAnalysisMechanicalAdvantageBalancingTorqueTeeterTotterDoorKnobTorqueSIUnits