Exponential functions differentiation intro | Advanced derivatives | AP Calculus AB | Khan Academy

Khan Academy
22 Jul 201605:24
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into the concept of derivatives of exponential functions, highlighting the unique property of e^x and exploring how to find derivatives for exponential functions with bases other than e. By using logarithmic and exponent rules, the video demonstrates that the derivative of a^x is (natural log of a) * a^x, offering a practical approach to solving derivatives for a variety of bases.

Takeaways
  • 📚 The derivative of e to the power of x (e^x) is e^x, showcasing the unique property of the exponential function with base e.
  • 🔍 When exploring derivatives of exponential functions with different bases, the key lies in understanding how to express them in terms of the natural logarithm.
  • 🌟 The derivative of a function a^x, where a is any number, can be found using the natural logarithm, leading to the expression (ln(a)) * a^x.
  • 📈 By using algebra and exponent properties, we can rewrite a^x as e^( ln(a) * x ) to facilitate the calculation of its derivative.
  • 🤔 The natural logarithm of a (ln(a)) represents the power to which e must be raised to obtain a, which is crucial for understanding the derivative of a^x.
  • 📝 The derivative calculation involves applying the chain rule, taking the derivative of the outer function with respect to the inner function, and vice versa.
  • 🧮 The derivative of a^x simplifies to ln(a) * a^x, which is a significant result for exponential functions with any base other than e.
  • 🌈 To find the derivative of an expression with a different base, such as 8 * 3^x, multiply the coefficient (8) by the natural log of the base (ln(3)) and the base raised to the power of x.
  • 🔧 The process of differentiating exponential functions with various bases is a practical application of logarithms and the chain rule in calculus.
  • 🎓 Understanding the relationship between exponential functions, their derivatives, and the natural logarithm is fundamental for solving more complex calculus problems.
Q & A

  • What is the derivative of e to the x?

    -The derivative of e to the x with respect to x is equal to e to the x. This is a unique property of the exponential function with base e.

  • If the derivative of a function at any point is equal to the value of the function itself, what kind of function is it?

    -It is an exponential function with base e. This property is specific to the function with base e, making it stand out among other exponential functions.

  • How can we find the derivative of an exponential function with any base?

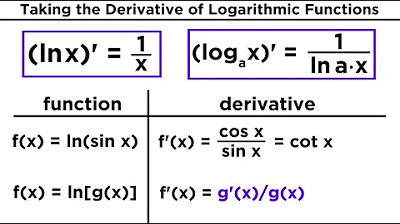
    -We can use the property of logarithms and exponents to rewrite the exponential function with base 'a' as e to the power of the natural log of 'a' times x, and then apply the chain rule to find the derivative, which will be the natural log of 'a' times 'a' to the power of x.

  • What is the role of logarithms in finding the derivative of an exponential function with a different base?

    -Logarithms are used to express the base 'a' in terms of 'e' by setting 'a' equal to 'e' to the power of the natural log of 'a'. This allows us to use the known derivative of e to the x to find the derivative of any exponential function.

  • What is the derivative of a to the x with respect to x?

    -The derivative of a to the x with respect to x is the natural log of 'a' times 'a' to the power of x, which can be expressed as ln(a) * a^x.

  • How can we apply the chain rule to find the derivative of an exponential function?

    -We apply the chain rule by first taking the derivative of the outer function (e to the natural log of a times x) with respect to the inner function (natural log of a times x), and then multiplying by the derivative of the inner function with respect to x (which is a constant, the natural log of a).

  • What is the derivative of 8 times 3 to the power of x?

    -The derivative of 8 times 3 to the power of x is 8 times the natural log of 3 times 3 to the power of x, which can be written as 8 * ln(3) * 3^x.

  • What property of exponents allows us to simplify the derivative of an exponential function?

    -The property that when raising a number to an exponent and then raising that result to another exponent, it is equivalent to raising the original base to the product of those exponents simplifies the derivative of an exponential function.

  • Why is understanding the derivative of exponential functions important?

    -Understanding the derivative of exponential functions is important as it provides insights into the rate of change of these functions, which is crucial in many areas of mathematics, physics, and engineering.

  • How does the derivative of an exponential function with base 'a' relate to the natural log of 'a'?

    -The derivative of an exponential function with base 'a' is directly proportional to the natural log of 'a', as seen in the expression ln(a) * a^x, which shows the close relationship between exponential and logarithmic functions.

  • What is the significance of the chain rule in calculus?

    -The chain rule is significant in calculus as it allows us to find the derivative of composite functions, which are functions made up of one or more functions. It is a fundamental tool for differentiating complex functions.

Outlines
00:00
📚 Derivatives of Exponential Functions

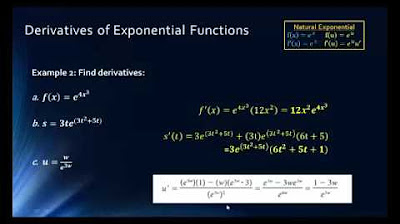
This paragraph delves into the concept of taking derivatives of exponential functions, starting with the special case of e to the power of x, whose derivative is the function itself. It then explores the general case of a to the power of x, where 'a' can be any number. By using algebra and properties of exponents, the voiceover explains how to express 'a' as e to the natural log of 'a', which allows the application of the chain rule to find the derivative. The result is the natural log of 'a' times a to the power of x, highlighting the relationship between the derivative and the original function.

05:00
🔢 Application of Derivative Rules to Specific Exponentials

Building on the previous explanation, this paragraph applies the derived rules to a specific example, calculating the derivative of 8 times 3 to the power of x. By using the natural log property and the rules for derivatives of exponential functions, it is shown that the derivative is 8 times the natural log of 3 times 3 to the power of x, demonstrating how the general rule can be applied to find derivatives of expressions with different bases.

Mindmap
Keywords
💡Derivative
In the context of the video, a derivative refers to the rate at which a function changes at any given point, essentially representing the slope of the tangent line at that point on the graph of the function. The video explores derivatives of exponential functions, highlighting how the derivative indicates the function's rate of change with respect to its variable, such as the derivative of e to the x being equal to e to the x itself. This fundamental concept in calculus is used to understand how functions behave and change, serving as a basis for more complex calculations and applications in various fields.
💡Exponential Functions
Exponential functions, as discussed in the video, are functions of the form a to the x, where 'a' is a constant base and 'x' is the variable exponent. The video specifically explores the properties of these functions when taking derivatives, particularly highlighting the unique case when the base is the mathematical constant e. The exploration of exponential functions with bases other than e, and how their derivatives are calculated, forms a central theme, illustrating the broader application of calculus concepts to various types of functions.
💡e (mathematical constant)
The constant e is a fundamental mathematical constant approximately equal to 2.71828, known as the base of the natural logarithm. It's unique because the derivative of e to the power of x with respect to x is itself e to the x. This property makes e especially important in calculus, finance, and other fields. The video emphasizes e's special role in the context of exponential functions and derivatives, serving as a foundational concept for understanding the behavior of exponential functions at a deeper level.
💡Natural Logarithm
The natural logarithm, denoted as ln, is the logarithm to the base e, where e is the mathematical constant approximately equal to 2.71828. It represents the power to which e must be raised to obtain a certain number. In the video, the natural logarithm is used as a tool to transform exponential functions with any base into a form that involves e, facilitating the derivation process. This concept is crucial for understanding how to generalize the derivative of e to the x to find the derivatives of exponential functions with any base.
💡Chain Rule
The chain rule is a formula in calculus for computing the derivative of the composition of two or more functions. It essentially allows one to break down the derivative of a complex function into simpler parts. The video employs the chain rule to find the derivative of an exponential function where the exponent itself is a function of x, demonstrating how to apply the rule in the context of exponential functions with bases other than e. This illustrates the chain rule's critical role in facilitating the differentiation of composite functions.
💡Logarithm Properties
Logarithm properties include rules that simplify the manipulation and transformation of logarithmic expressions, such as the property that the logarithm of a product equals the sum of the logarithms. The video leverages these properties to rewrite an exponential function with any base as an equivalent expression with base e, facilitating the differentiation process. This application underscores the usefulness of logarithm properties in solving calculus problems, particularly in finding derivatives of exponential functions.
💡Function's Slope
The slope of a function at any given point refers to the steepness or incline of the tangent line to the function at that point, effectively representing the rate at which the function's value changes with respect to changes in the variable. In the context of the video, understanding the slope of exponential functions is crucial for finding derivatives, which are essentially the mathematical representation of the function's slope. This concept is foundational in calculus and is directly related to the video's main theme of exploring the derivatives of exponential functions.
💡Algebra
Algebra refers to a branch of mathematics dealing with symbols and the rules for manipulating these symbols. In the video, algebraic manipulation and exponent properties are used to transform the expression of an exponential function with any base into a form involving the base e, enabling the application of known derivative rules. This highlights how algebraic techniques are essential for problem-solving in calculus, particularly in the context of finding derivatives.
💡Exponent Properties
Exponent properties are rules that govern the operations on expressions with exponents, such as the property that multiplying powers with the same base equates to adding their exponents. The video utilizes these properties to simplify and manipulate the expressions of exponential functions during the process of differentiation. This demonstrates the relevance of exponent properties in the context of calculus, especially in solving problems related to the derivatives of exponential functions.
💡Product Rule
Although not explicitly mentioned in the provided transcript, the product rule is an essential calculus rule used to differentiate functions that are products of two or more functions. The video's exploration of taking derivatives of exponential functions indirectly relates to this rule, as understanding how to differentiate products of functions is fundamental when dealing with exponential functions multiplied by coefficients or other functions. The product rule, together with the chain rule, exemplifies the core calculus principles needed to tackle a wide range of differentiation problems.
Highlights

Exploring the derivatives of exponential functions is the main focus of the video.

The derivative of e^x with respect to x is equal to e^x, showcasing the unique property of the exponential function with base e.

The derivative of an exponential function a^x can be determined using algebra and exponent properties.

a can be expressed as e^(natural log of a), which is a key step in finding the derivative of a^x.

The natural log of a is the power required to raise e to obtain a, which is fundamental in rewriting a^x in terms of e.

The derivative of a^x with respect to x is the natural log of a times a^x, derived using the chain rule.

The derivative of e^(natural log of a) is a^(natural log of a), simplifying back to a.

The video demonstrates the application of this result to find the derivative of expressions with bases other than e.

The derivative of 8*3^x is calculated as 8*(natural log of 3)*3^x, as an example of applying the derived rule.

Understanding the derivative of exponential functions is crucial for various mathematical and practical applications.

The video emphasizes the importance of not just accepting the results but understanding the underlying mathematical concepts.

The chain rule is used to evaluate the derivative of the exponential function with a different base.

The derivative of a^x reveals the relationship between the base a, the natural log of a, and the exponential function.

The video provides a clear and detailed explanation of the process to find the derivative of a^x.

The natural log plays a pivotal role in transforming the base of the exponential function to facilitate the derivative calculation.

The video demonstrates the power of logarithmic and exponential functions in mathematical analysis.

The ability to find derivatives of exponential functions with any base is a valuable skill in advanced mathematics.

Transcripts

Browse More Related Video

Math1325 Lecture 11 2 - Derivatives of Exponential

Derivatives of Exponential Functions

Differentiating Exponentials with Non-e Bases

2.2 - Derivatives of Exponential Functions

Derivatives of Exponential Functions

Derivatives of Logarithmic and Exponential Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Exponential FunctionsDerivativesAlgebraLogarithmsMathematicsEducationalCalculuse^xa^x