Derivatives of Exponential Functions
TLDRThis video tutorial delves into the process of finding derivatives of exponential functions, emphasizing the use of base 'e' and other bases with examples. It explains the derivative of e^x as e^x itself, and extends this concept to more complex expressions like e^(5x+3) and e^(x^2). The video also covers the general formula for different bases, a^u, using the derivative rule a^u * u' * ln(a). It progresses to more challenging problems, applying the product and quotient rules to functions like (x^3 * e^(4x)) and complex fractions involving e^x. The video concludes with a comprehensive example, simplifying a complex expression using the difference of squares method, demonstrating a thorough understanding of calculus concepts.
Takeaways
- 📚 The derivative of e to the x is e to the x times 1, simplifying to e to the x.
- 📈 For the derivative of e to the u, where u is a function, use the formula: e to the u times the derivative of u.
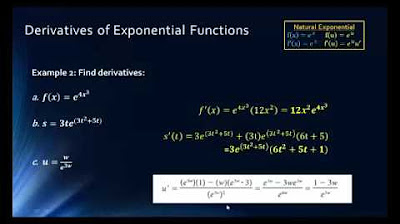
- 🌟 When differentiating e to the 5x plus 3, the result is 5 times e to the 5x plus 3.
- 🌠 For e to the x squared, the derivative is 2x times e to the x squared.
- 🔢 If the base of the exponential function is not e, use the formula a to the u times u prime times ln(a).
- 🏠 For a non-e base, like 3 to the x, the derivative involves using ln(a), in this case, ln(3).
- 📊 When differentiating more complex expressions like a to the 2x minus 5, use the chain rule and ln(a).
- 🔧 For the product rule, remember the formula: (f times g) prime equals f prime times g plus f times g prime.
- 🌐 For composite functions, use the chain rule to find the derivative of the inner function and then apply the outer function's derivative.
- 🔄 When dealing with fractions, apply the quotient rule: (f over g) prime equals g times f prime minus f times g prime over g squared.
- 🎯 For complex fractions, simplify by factoring and using algebraic identities, such as the difference of squares.
Q & A
What is the derivative of e to the x?
-The derivative of e to the x is simply e to the x, as the derivative of x is 1.
What is the general formula for the derivative of e to the u, where u is a function?
-The general formula for the derivative of e to the u is e to the u times the derivative of u.
How do you find the derivative of e to the 5x plus 3?
-You rewrite the expression as e to the 5x times the derivative of 5x plus 3, which is 5. So, the derivative is 5 times e to the 5x plus 3.
What is the derivative of e to the x squared?
-The derivative is 2x times e to the x squared, as the derivative of x squared is 2x.
How do you differentiate exponential functions with a base other than e?
-For a base other than e, the derivative of a raised to u is a to u times the derivative of u times the natural log of a.
What is the derivative of 3 raised to the x, using the general formula for non-e exponential functions?
-The derivative is 3 to the u times u prime times ln 3, where u is the exponent function, which in this case is x, so the derivative is 3 to the x times the derivative of x (which is 1) times ln 3.
How do you find the derivative of e raised to the sine x?
-The derivative is e to the sine x times the derivative of sine x, which is cosine x.
What is the derivative of x cubed e to the 4x using the product rule?
-The derivative is x squared e to the 4x plus 3 times e to the 4x times 4, which simplifies to x squared e to the 4x plus 12e to the 4x.
What is the quotient rule for finding the derivative of a fraction?
-The quotient rule states that the derivative of f over g is g times f prime minus f times g prime, all divided by g squared.
How do you simplify the expression for the derivative of e to the x plus e to the negative x divided by e to the x minus e to the negative x?
-After applying the quotient rule, the expression simplifies to negative four times e to the x minus e to the negative x, all divided by e to the x minus e to the negative x squared.
What method can be used to further simplify expressions in the form of a squared minus b squared?
-The difference of squares method can be used to factor and simplify expressions in the form of a squared minus b squared, which is a minus b times a plus b.
Outlines
📚 Derivatives of Exponential Functions
This paragraph introduces the concept of finding derivatives of exponential functions, particularly those with base e. It explains the fundamental formula for the derivative of e^u, where u is a function, which is e^u times the derivative of u. The paragraph provides examples of applying this rule to expressions like e^(5x) + 3 and e^(x^2), and demonstrates how to rewrite and simplify the expressions to find their derivatives. It also touches on the derivative of e^x, which is simply e^x times the derivative of x, equating to e^(3x) in the given example.
📈 Derivatives with Different Bases
This section delves into the derivatives of exponential functions with bases other than e, such as 3^x or 9^x. It introduces the general formula for the derivative of a^u, which is a^u times u' times ln(a), and contrasts it with the formula for e^u. The paragraph provides examples of applying this formula, including the derivative of 7^(2x - 5) and 9^(x^3), and emphasizes the use of the natural logarithm in these calculations. It also presents a problem involving the derivative of 5^u, where u is 2x - x^2, and explains the process of finding the derivative using the new formula.
🔢 Solving Complex Exponential Derivatives
This paragraph focuses on solving more complex problems involving exponential derivatives. It starts with the derivative of x^3 * e^(4x), using the product rule. The explanation details the process of finding the derivatives of the individual parts of the function and combining them according to the product rule. The paragraph then tackles a more challenging problem involving a fraction, where the quotient rule is applied. It explains the process of finding the derivatives of the numerator and the denominator, and then using the quotient rule to find the derivative of the entire expression. The paragraph concludes with a detailed example of simplifying the resulting expression using the difference of squares method.
Mindmap
Keywords
💡Derivative
💡Exponential Functions
💡Base e
💡Chain Rule
💡Product Rule
💡Quotient Rule
💡Natural Logarithm
💡Composite Functions
💡Simplifying Expressions
💡Difference of Squares
💡Exponential Growth and Decay
Highlights
The derivative of e to the x is e to the x times the derivative of x, which is 1.
The derivative of e to the 5x plus 3 is 5 times e to the 5x plus 3.
The derivative of e to the x squared is 2x times e to the x squared.
For exponential functions with a base other than e, the derivative is a to u times u prime times ln(a).
The derivative of 3 to the x is 3 times ln(3) times x to the x minus 1.
The derivative of 9 to the x cubed is 3 times 9 to the x cubed times the derivative of x cubed, which is 3x squared times ln(9).
The derivative of 5 to the 2x minus x squared is 2 minus 2x times ln(5) times 5 to the 2x minus x squared.
The derivative of e to the sine x is e to the sine x times the derivative of sine, which is cosine.
The derivative of 4 to the tangent x is 4 times the derivative of tangent, which is secant squared, times ln(4).
For the function x cubed e to the 4x, the derivative is found using the product rule, resulting in x squared e to the 4x plus 3 times e to the 4x.
The derivative of the fraction (e to the x plus e to the negative x) divided by (e to the x minus e to the negative x) is found using the quotient rule and simplifying the expression.
The final simplified form of the derivative of the fraction is negative four times e to the negative x plus two times e to the x, divided by e to the x minus e to the negative x squared.
The video provides a comprehensive overview of differentiating exponential functions with various bases and exponents.
The use of the product and quotient rules is demonstrated for more complex exponential function derivatives.
The video emphasizes the importance of understanding the natural logarithm when differentiating exponential functions with bases other than e.
The process of simplifying derivatives through factoring and recognizing patterns, such as the difference of squares, is highlighted.
The video serves as a valuable resource for learners looking to understand the calculus of exponential functions.
The examples provided in the video cover a range of scenarios, from basic to more advanced problems, offering a solid foundation in the topic.
The video's structured approach to teaching the derivative of exponential functions makes it accessible to a wide range of learners.
The video concludes with a summary of the key points and a thank you message to the viewers.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: